Sliding Mode Controller with Generalized Extended State Observer for Single Link Flexible Manipulator
Abstract
:1. Introduction
2. Preliminaries and Problem Statement
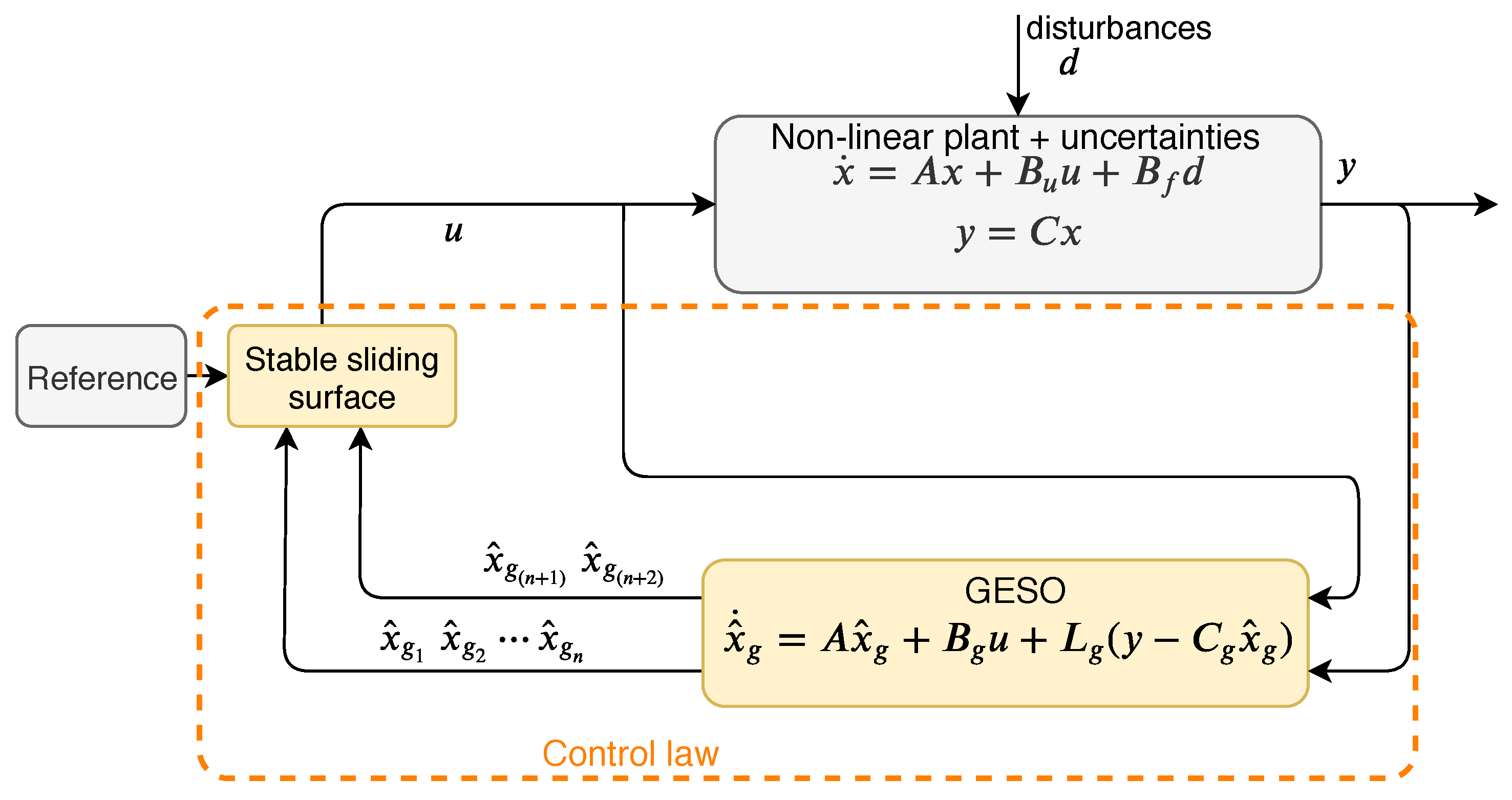
3. Proposed Control Scheme
3.1. Criteria for EGESO
- , where A and are any general system and control matrices respectively. See numerical examples in results section for more understanding.
- , , with , , denotes zeros in the matrix triple of , where C is output matrix. For more information, see numerical examples in the results section.
- denotes the multiplicity of .
- The zeros , and their respective multiplicities , are split into: minimum phase zero, , = 1, non-minimum phase zero, , and zeros at the imaginary axis, = 1, satisfying .
- The total number of zeros in the matrix triple is indicated by . Furthermore, in the same way: = , = , and = are the total number of minimum phase zeros, non-minimum phase zeros, and zeros at the imaginary axis, respectively.
- The matrix riple uses the same notation as defined in (2)–(5), replacing the subindexes ‘u’ by ‘f’, where is a disturbance matrix. See numerical examples in results section for more understanding.
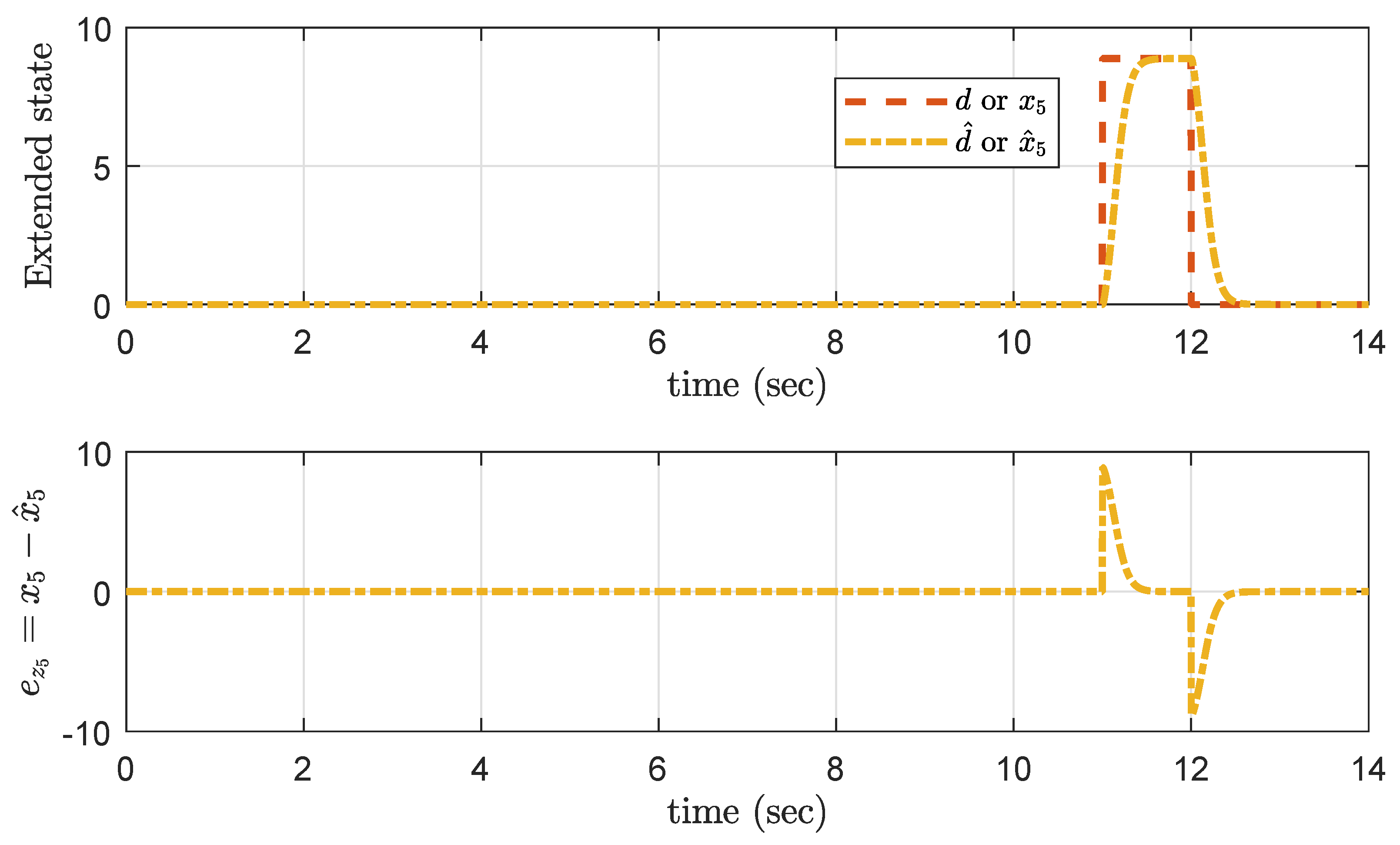
3.2. Design of Enhanced Generalized Extended State Observer
3.3. Design of Stable Sliding Surface
3.4. Control Law Using Estimated States
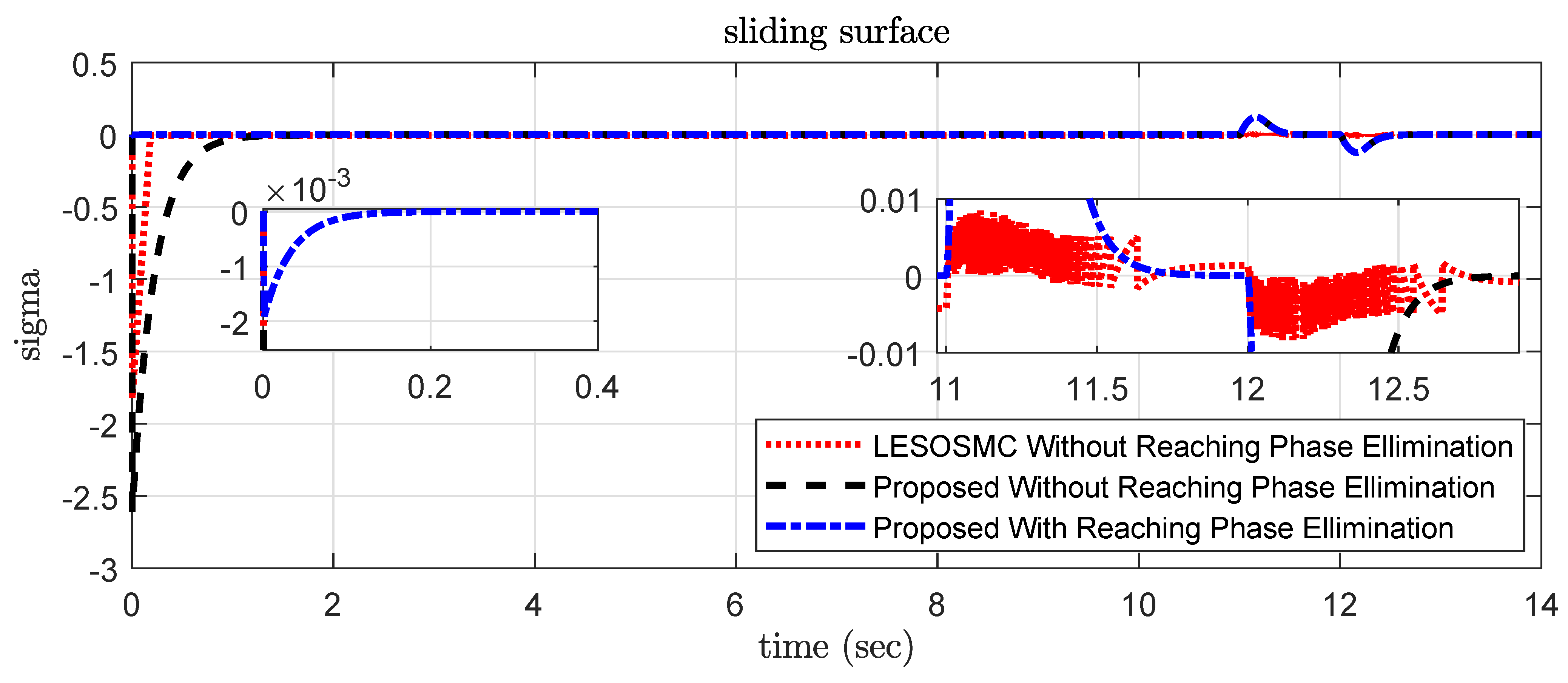
3.5. Reaching Phase Elimination
4. Stability
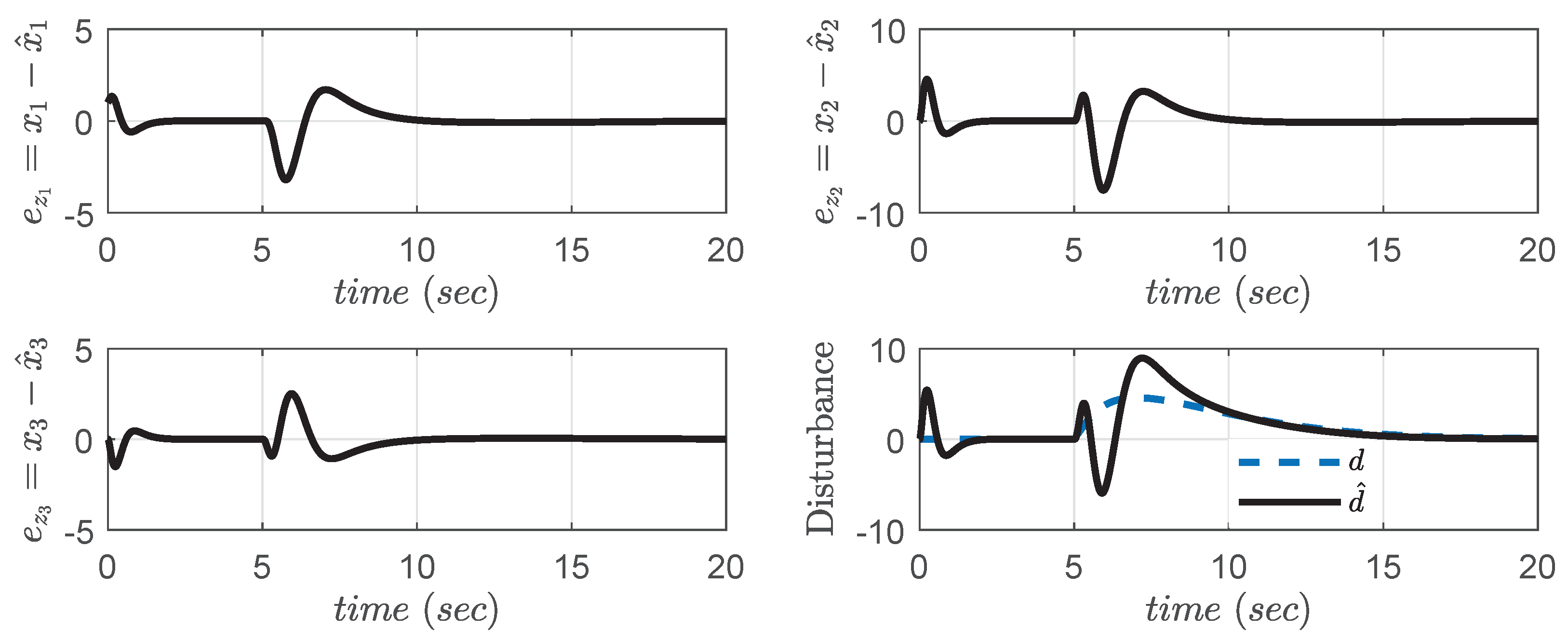
5. Simulation and Experimental Results
5.1. Study Example 1
5.2. Study Example 2
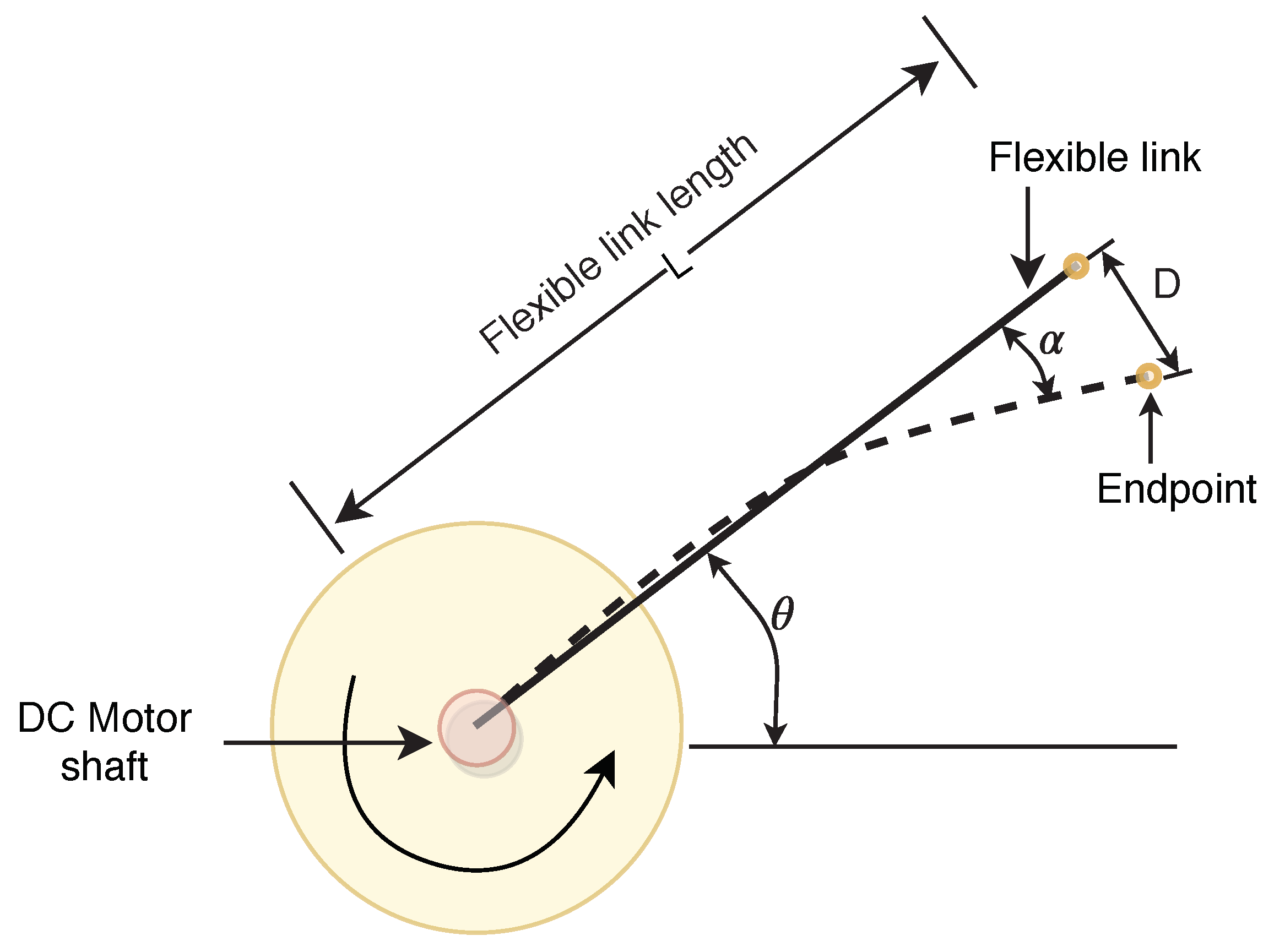
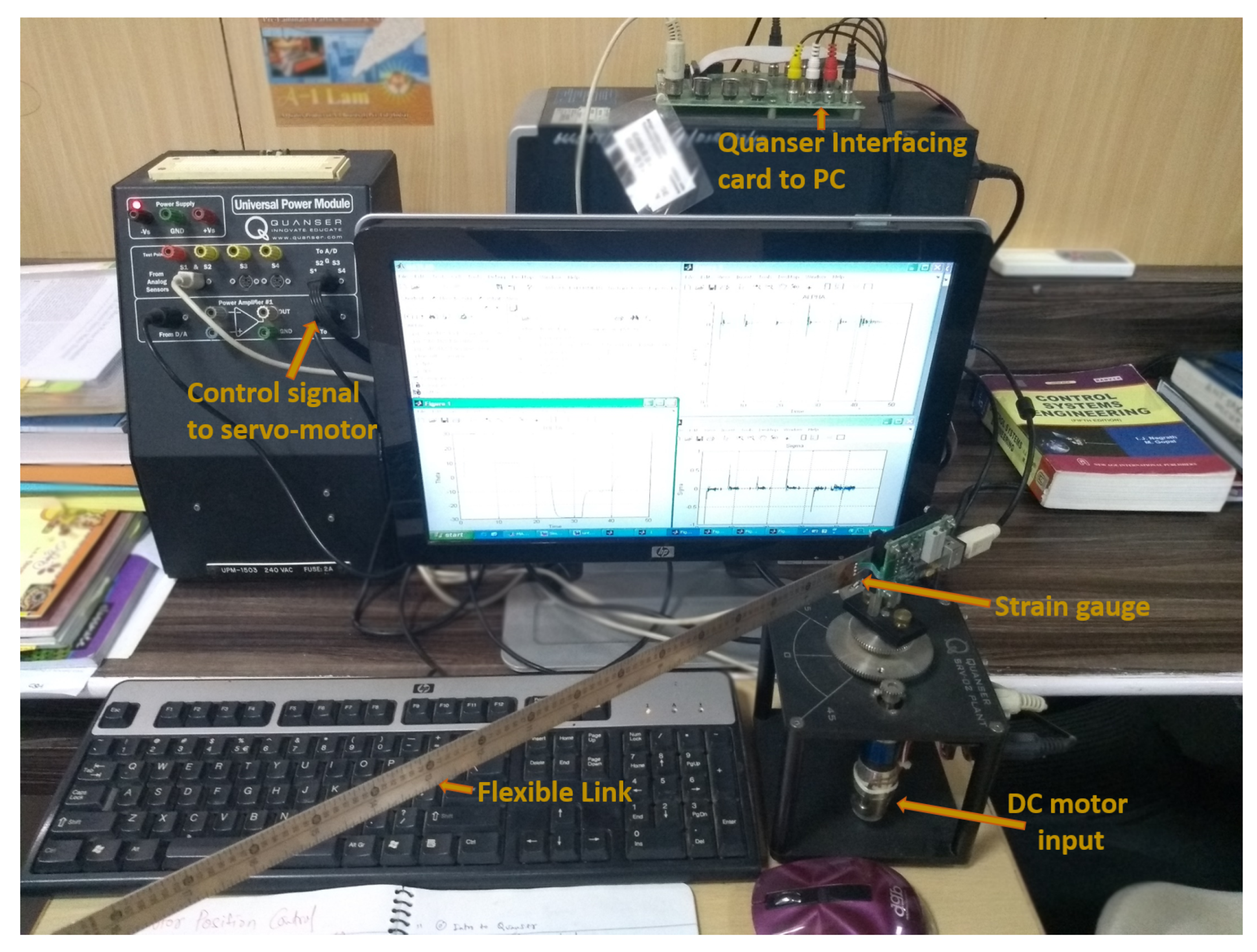
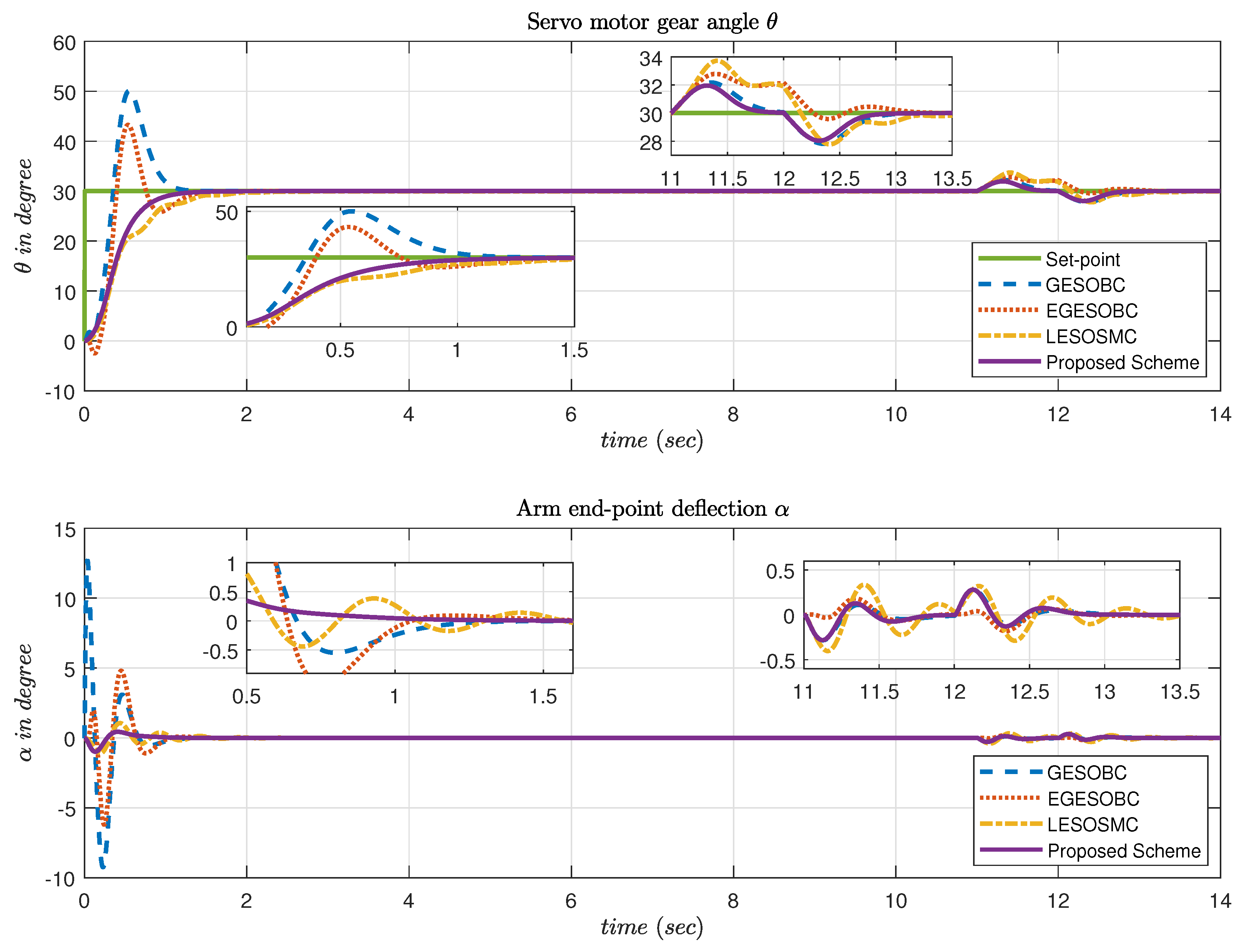
5.3. Experimental Model
5.3.1. System Model Dynamics
5.3.2. Simulation Results for SLFM
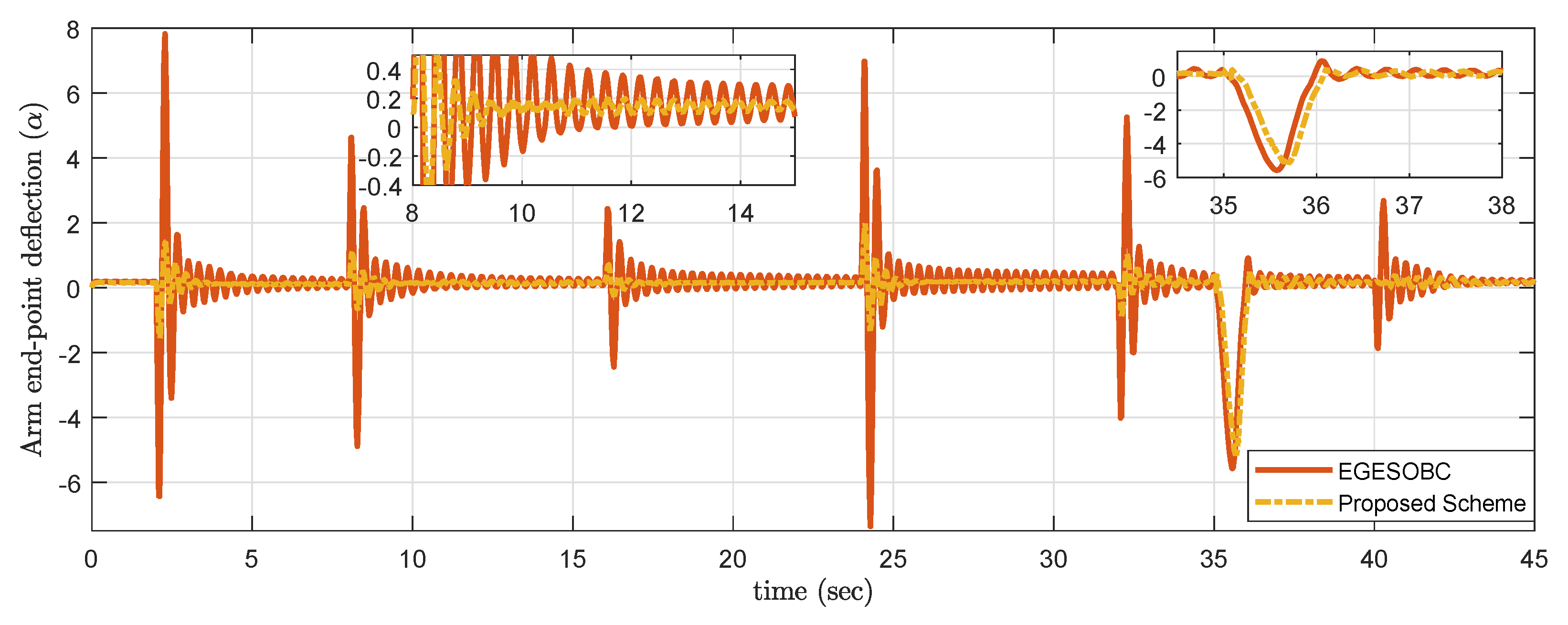
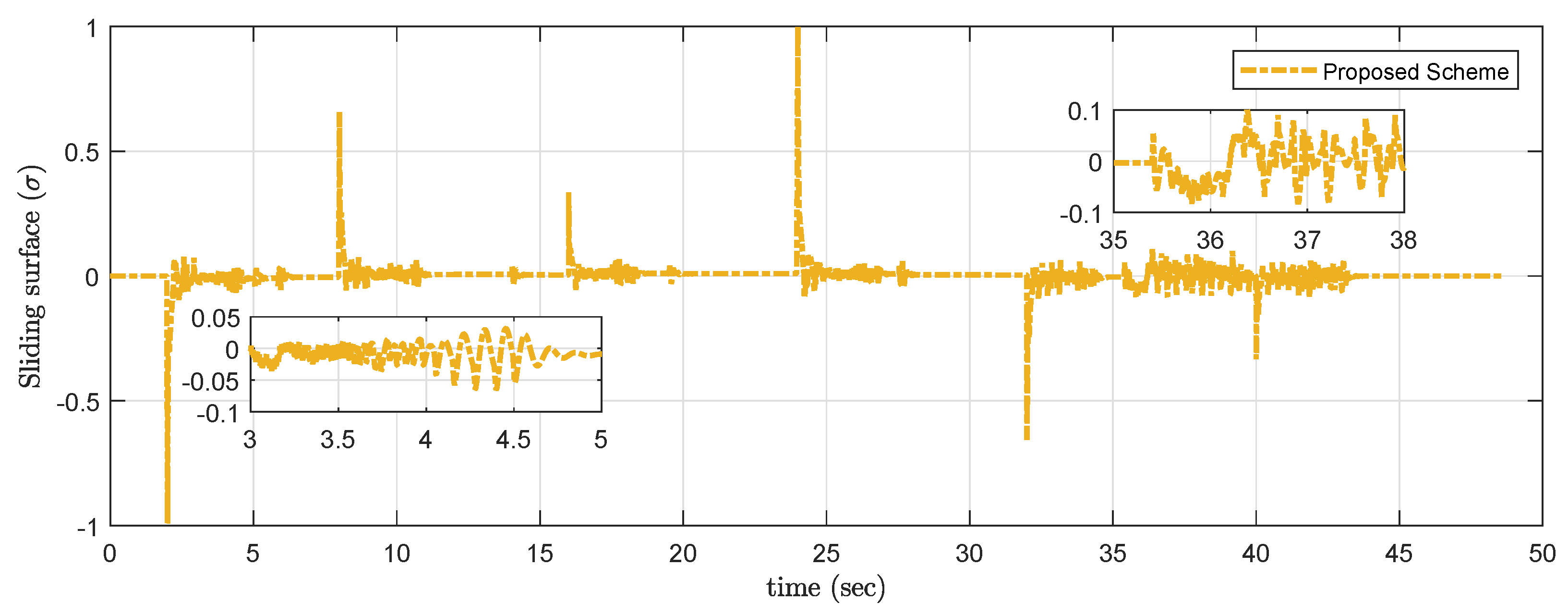
5.3.3. Experimentation Result for SLFM
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Gao, Z. On the centrality of disturbance rejection in automatic control. ISA Trans. 2014, 53, 850–857. [Google Scholar] [CrossRef] [Green Version]
- Rezvani, A.; Gandomkar, M. Simulation and control of intelligent photovoltaic system using new hybrid fuzzy-neural method. Neural Comput. Appl. 2016, 28, 2501–2518. [Google Scholar] [CrossRef]
- Gupta, M.; Hawari, H.F.; Kumar, P.; Burhanudin, Z.A.; Tansu, N. Functionalized Reduced Graphene Oxide Thin Films for Ultrahigh CO2 Gas Sensing Performance at Room Temperature. Nanomaterials 2021, 11, 623. [Google Scholar] [CrossRef]
- Aole, S.; Elamvazuthi, I.; Waghmare, L.; Patre, B.; Bhaskarwar, T.; Meriaudeau, F.; Su, S. Active Disturbance Rejection Control Based Sinusoidal Trajectory Tracking for an Upper Limb Robotic Rehabilitation Exoskeleton. Appl. Sci. 2022, 12, 1287. [Google Scholar] [CrossRef]
- Ohishi, K.; Nakao, M.; Ohnishi, K.; Miyachi, K. Microprocessor-Controlled DC Motor for Load-Insensitive Position Servo System. IEEE Trans. Ind. Electron. 1987, 34, 44–49. [Google Scholar] [CrossRef]
- She, J.; Fang, M.; Ohyama, Y.; Hashimoto, H.; Wu, M. Improving Disturbance-Rejection Performance Based on an Equivalent-Input-Disturbance Approach. IEEE Trans. Ind. Electron. 2008, 55, 380–389. [Google Scholar] [CrossRef]
- Zhong, Q.C.; Rees, D. Control of Uncertain LTI Systems Based on an Uncertainty and Disturbance Estimator. J. Dyn. Syst. Meas. Control 2004, 126, 905–910. [Google Scholar] [CrossRef]
- Gao, Z.; Huang, Y.; Han, J. An alternative paradigm for control system design. In Proceedings of the 40th IEEE Conference on Decision and Control (Cat. No.01CH37228), Orlando, FL, USA, 4–7 December 2001; Volume 5, pp. 4578–4585. [Google Scholar] [CrossRef]
- Han, J. The extended state observer of a class of uncertain systems. Control Decis. 1995, 10, 19–23. (In Chinese) [Google Scholar]
- Chen, W.; Yang, J.; Guo, L.; Li, S. Disturbance-Observer-Based Control and Related Methods—An Overview. IEEE Trans. Ind. Electron. 2016, 63, 1083–1095. [Google Scholar] [CrossRef] [Green Version]
- Johnson, C. Optimal control of the linear regulator with constant disturbances. IEEE Trans. Autom. Control 1968, 13, 416–421. [Google Scholar] [CrossRef]
- Ginoya, D.; Shendge, P.D.; Phadke, S.B. State and Extended Disturbance Observer for Sliding Mode Control of Mismatched Uncertain Systems. J. Dyn. Syst. Meas. Control 2015, 137. [Google Scholar] [CrossRef]
- Li, S.; Yang, J.; Chen, W.; Chen, X. Generalized Extended State Observer Based Control for Systems With Mismatched Uncertainties. IEEE Trans. Ind. Electron. 2012, 59, 4792–4802. [Google Scholar] [CrossRef] [Green Version]
- Castillo, A.; García, P.; Sanz, R.; Albertos, P. Enhanced extended state observer-based control for systems with mismatched uncertainties and disturbances. ISA Trans. 2018, 73, 1–10. [Google Scholar] [CrossRef] [Green Version]
- Zhou, L.; She, J. Generalized-Extended-State-Observer-Based Repetitive Control for MIMO Systems With Mismatched Disturbances. IEEE Access 2018, 6, 61377–61385. [Google Scholar] [CrossRef]
- Han, J. From PID to Active Disturbance Rejection Control. IEEE Trans. Ind. Electron. 2009, 56, 900–906. [Google Scholar] [CrossRef]
- Aole, S.; Elamvazuthi, I.; Waghmare, L.; Patre, B.; Meriaudeau, F. Non-linear active disturbance rejection control for upper limb rehabilitation exoskeleton. Proc. Inst. Mech. Eng. Part I J. Syst. Control. Eng. 2021, 235, 606–632. [Google Scholar] [CrossRef]
- Aole, S.; Elamvazuthi, I.; Waghmare, L.; Patre, B.; Meriaudeau, F. Improved active disturbance rejection control for trajectory tracking control of lower limb robotic rehabilitation exoskeleton. Sensors 2020, 20, 3681. [Google Scholar] [CrossRef]
- Guo, B.-Z.; Wu, Z.-H. Output tracking for a class of nonlinear systems with mismatched uncertainties by active disturbance rejection control. Syst. Control Lett. 2017, 100, 21–31. [Google Scholar] [CrossRef]
- Hung, J.Y.; Gao, W.; Hung, J.C. Variable structure control: A survey. IEEE Trans. Ind. Electron. 1993, 40, 2–22. [Google Scholar] [CrossRef] [Green Version]
- Rezvani, A.; Ali Esmaeily, H.E.; Mohammadinodoushan, M. Intelligent hybrid power generation system using new hybrid fuzzy-neural for photovoltaic system and RBFNSM for wind turbine in the grid connected mode. Front. Energy 2019, 13, 131–148. [Google Scholar] [CrossRef]
- Sun, H.; Li, S.; Sun, C. Finite time integral sliding mode control of hypersonic vehicles. Nonlinear Dyn. 2013, 73, 229–244. [Google Scholar] [CrossRef]
- Lu, K.; Xia, Y. Adaptive attitude tracking control for rigid spacecraft with finite-time convergence. Automatica 2013, 49, 3591–3599. [Google Scholar] [CrossRef]
- Edward, C.; Spurgeon, S. Sliding Mode Control: Theory and Applications; CRC Press: London, UK, 1998. [Google Scholar]
- Edwards, C.; Spurgeon, S.K. Sliding mode stabilization of uncertain systems using only output information. Int. J. Control 1995, 62, 1129–1144. [Google Scholar] [CrossRef]
- Abidi, K.; Sabanovic, A. Sliding-Mode Control for High-Precision Motion of a Piezostage. IEEE Trans. Ind. Electron. 2007, 54, 629–637. [Google Scholar] [CrossRef]
- Slotine, J.J.E.; Li, W. Applied Nonlinear Control; Prentice-Hall: Englewood Cliffs, NJ, USA, 1991; Volume 199. [Google Scholar]
- Mohamed, G.; Sofiane, A.A.; Nicolas, L. Adaptive super twisting extended state observer based sliding mode control for diesel engine air path subject to matched and unmatched disturbance. Math. Comput. Simul. 2018, 151, 111–130. [Google Scholar] [CrossRef]
- Qian, J.; Xiong, A.; Ma, W. Extended State Observer-Based Sliding Mode Control with New Reaching Law for PMSM Speed Control. Math. Probl. Eng. 2016, 2016, 1–10. [Google Scholar] [CrossRef] [Green Version]
- Wang, J.; Li, S.; Yang, J.; Wu, B.; Li, Q. Extended state observer-based sliding mode control for PWM-based DC–DC buck power converter systems with mismatched disturbances. IET Control Theory Appl. 2015, 9, 579–586. [Google Scholar] [CrossRef]
- Prasad, S.; Purwar, S.; Kishor, N. Load frequency regulation using observer based non-linear sliding mode control. Int. J. Electr. Power Energy Syst. 2019, 104, 178–193. [Google Scholar] [CrossRef]
- Zhao, L.; Cheng, H.; Wang, T. Sliding mode control for a two-joint coupling nonlinear system based on extended state observer. ISA Trans. 2018, 73, 130–140. [Google Scholar] [CrossRef]
- Mujumdar, A.; Tamhane, B.; Kurode, S. Observer-Based Sliding Mode Control for a Class of Noncommensurate Fractional-Order Systems. IEEE/ASME Trans. Mechatronics 2015, 20, 2504–2512. [Google Scholar] [CrossRef]
- Fareh, R.; Al-Shabi, M.; Bettayeb, M.; Ghommam, J. Robust Active Disturbance Rejection Control For Flexible Link Manipulator. Robotica 2020, 38, 118–135. [Google Scholar] [CrossRef]
- Hisseine, D.; Lohmann, B. Sliding Mode Tracking Control for a Single-Link Flexible Robot Arm. IFAC Proc. Vol. 2000, 33, 333–338. [Google Scholar] [CrossRef]
- Yang, H.J.; Tan, M. Sliding Mode Control for Flexible-link Manipulators Based on Adaptive Neural Networks. Int. J. Autom. Comput. 2018, 15, 239–248. [Google Scholar] [CrossRef]
- Ordaz, P.; Ordaz, M.; Cuvas, C.; Santos, O. Reduction of matched and unmatched uncertainties for a class of nonlinear perturbed systems via robust control. Int. J. Robust Nonlinear Control 2019, 29, 2510–2524. [Google Scholar] [CrossRef]
- Talole, S.; Kolhe, J.; Phadke, S. Extended-state-observer-based control of flexible-joint system with experimental validation. IEEE Trans. Ind. Electron. 2010, 57, 1411–1419. [Google Scholar] [CrossRef]
- S. Link Flexible Model. SRV02-Series Flexgauge Rotary Flexible Link User Manual Workbook, Quanser. 2019. Available online: https://www.quanser.com/products/rotary-flexible-link/ (accessed on 8 December 2019).
- Denker, A.; Ohnishi, K. Robust tracking control of mechatronic arms. IEEE/ASME Trans. Mechatronics 1996, 1, 181–188. [Google Scholar] [CrossRef]
- MathWorks. MATLAB. 2019. Available online: https://in.mathworks.com/ (accessed on 8 December 2019).
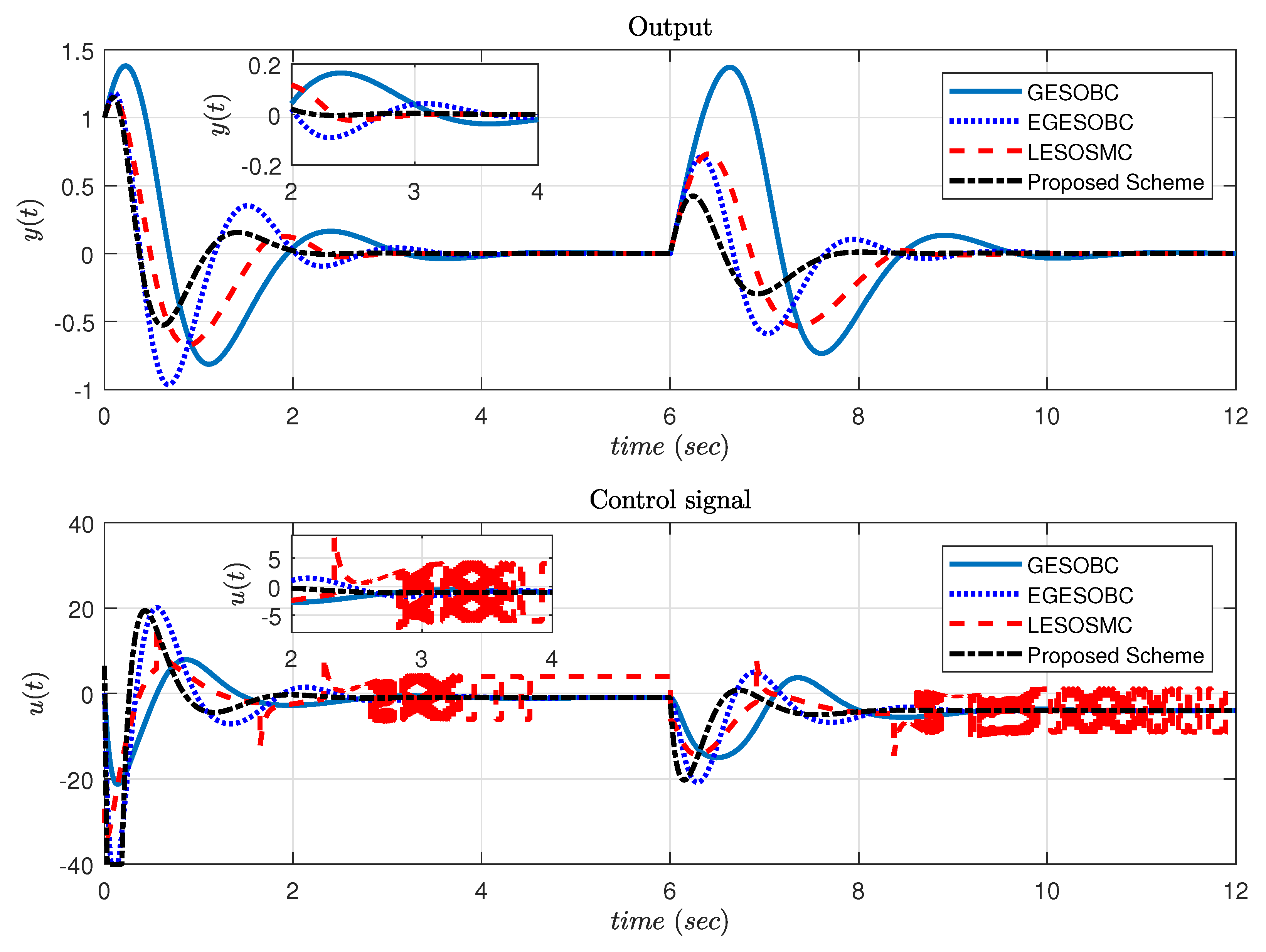


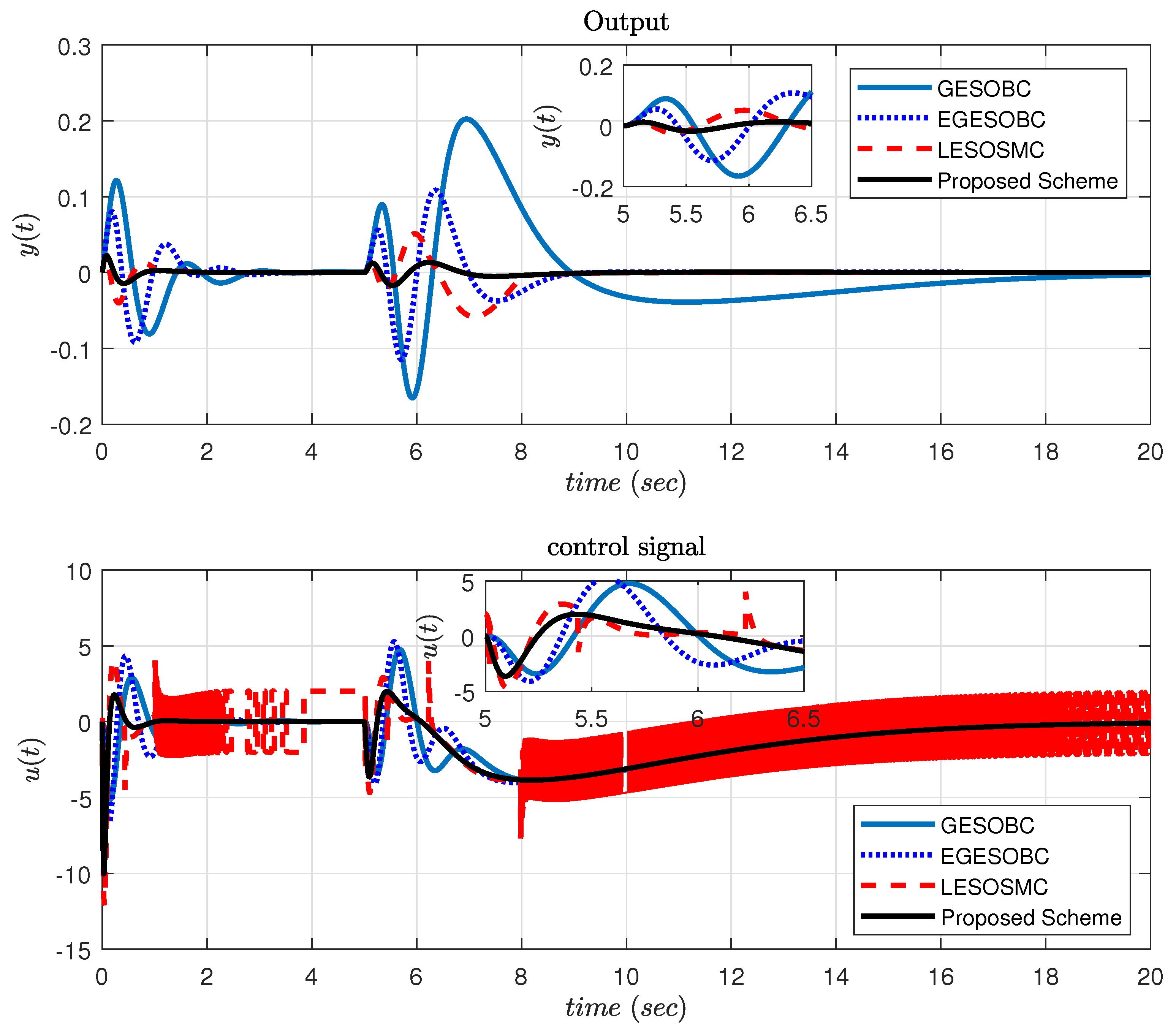
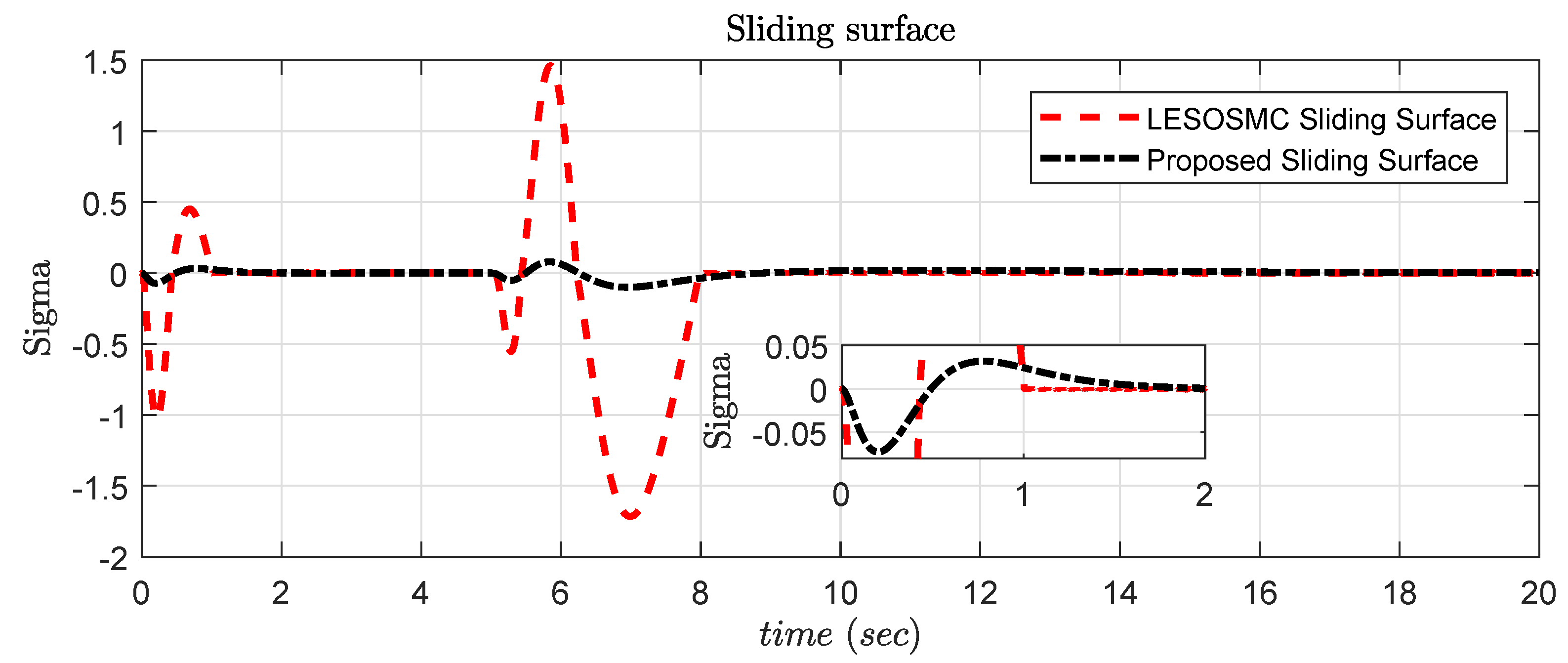




| Controller | Set-Point (r) | Error Indices | ||||
|---|---|---|---|---|---|---|
| Output (y) | IAE (10−2) | ISE (10−2) | ITAE | ITSE (10−2) | Control Efforts (u) in ITAE | |
| GESOBC | error = y − 0 | 311.5 | 256.1 | 13.47 | 1030 | 280.5 |
| EGESOBC | error = y − 0 | 175.5 | 103.9 | 5.827 | 254.4 | 284.4 |
| LESOSMC | error = y − 0 | 179.4 | 101.8 | 6.910 | 322.3 | 355.5 |
| Proposed scheme | error = y − 0 | 96.66 | 47.43 | 2.736 | 71.81 | 259.4 |
| Controller | Set-Point (r) | Error Indices | ||||
|---|---|---|---|---|---|---|
| Output (y) | IAE (10−3) | ISE (10−3) | ITAE (10−2) | ITSE (10−3) | Control Efforts (u) in ITAE | |
| GESOBC | error = y − 0 | 712.9 | 67.52 | 569.8 | 456.7 | 246.5 |
| EGESOBC | error = y − 0 | 233.3 | 14.50 | 113.7 | 67.89 | 248.2 |
| LESOSMC | error = y − 0 | 113.3 | 4.173 | 66.9 | 25.97 | 455.3 |
| Proposed scheme | error = y − 0 | 35.95 | 0.3193 | 19.60 | 1.314 | 238.1 |
| Parameter | Description | Unit |
|---|---|---|
| Arm end-point deflection | degree | |
| Servo motor gear angle | degree | |
| L | Flexible link length | cm |
| Load torque | Nm | |
| D | Link end-point deflection (Arc length) | cm |
| Viscous damping coefficient | ||
| Total stiffness of model | Nm/deg | |
| Moment of inertia of link | Kg-m2 | |
| Equivalent moment of inertia of the model | Kg-m2 |
| Controller | Set-Point (r) Radian | Error Indices | ||||
|---|---|---|---|---|---|---|
| Output (y) | IAE (10−2) | ISE (10−3) | ITAE (10−2) | ITSE (10−3) | Control Efforts (u) in ITAE | |
| GESOBC | error = y − 0.523 rad | 29.50 | 88.51 | 54.59 | 37.66 | 1.885 |
| EGESOBC | error = y − 0.523 rad | 28.69 | 91.29 | 60.27 | 34.91 | 1.663 |
| LESOSMC | error = y − 0.523 rad | 34.22 | 91.02 | 89.72 | 47.24 | 4.825 |
| Proposed scheme | error = y − 0.523 rad | 25.11 | 78.97 | 42.06 | 23.29 | 1.650 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bhaskarwar, T.; Hawari, H.F.; Nor, N.B.M.; Chile, R.H.; Waghmare, D.; Aole, S. Sliding Mode Controller with Generalized Extended State Observer for Single Link Flexible Manipulator. Appl. Sci. 2022, 12, 3079. https://doi.org/10.3390/app12063079
Bhaskarwar T, Hawari HF, Nor NBM, Chile RH, Waghmare D, Aole S. Sliding Mode Controller with Generalized Extended State Observer for Single Link Flexible Manipulator. Applied Sciences. 2022; 12(6):3079. https://doi.org/10.3390/app12063079
Chicago/Turabian StyleBhaskarwar, Tushar, Huzein Fahmi Hawari, Nursyarizal B. M. Nor, Rajan Hari Chile, Dhammaratna Waghmare, and Sumit Aole. 2022. "Sliding Mode Controller with Generalized Extended State Observer for Single Link Flexible Manipulator" Applied Sciences 12, no. 6: 3079. https://doi.org/10.3390/app12063079
APA StyleBhaskarwar, T., Hawari, H. F., Nor, N. B. M., Chile, R. H., Waghmare, D., & Aole, S. (2022). Sliding Mode Controller with Generalized Extended State Observer for Single Link Flexible Manipulator. Applied Sciences, 12(6), 3079. https://doi.org/10.3390/app12063079







