Safety Analysis and Condition Assessment of Corroded Energy Pipelines under Landslide Disasters
Abstract
:1. Introduction
2. Mechanism of Pipeline across Landslides
3. Numerical Modeling
- Both the soil and the pipeline are composed of uniform materials.
- The soil thickness in the landslide area is consistent, with cracks penetrating the landslide wall.
- The soil displacement in the landslide area follows a fourth-power parabolic distribution.
- Temperature effects on pipeline stress are neglected.
- Landslide displacement is applied from the rear of the model.
- The corrosion defects are considered as regular metal losses with uniform depth.
4. Results and Discussion
4.1. Model Validation
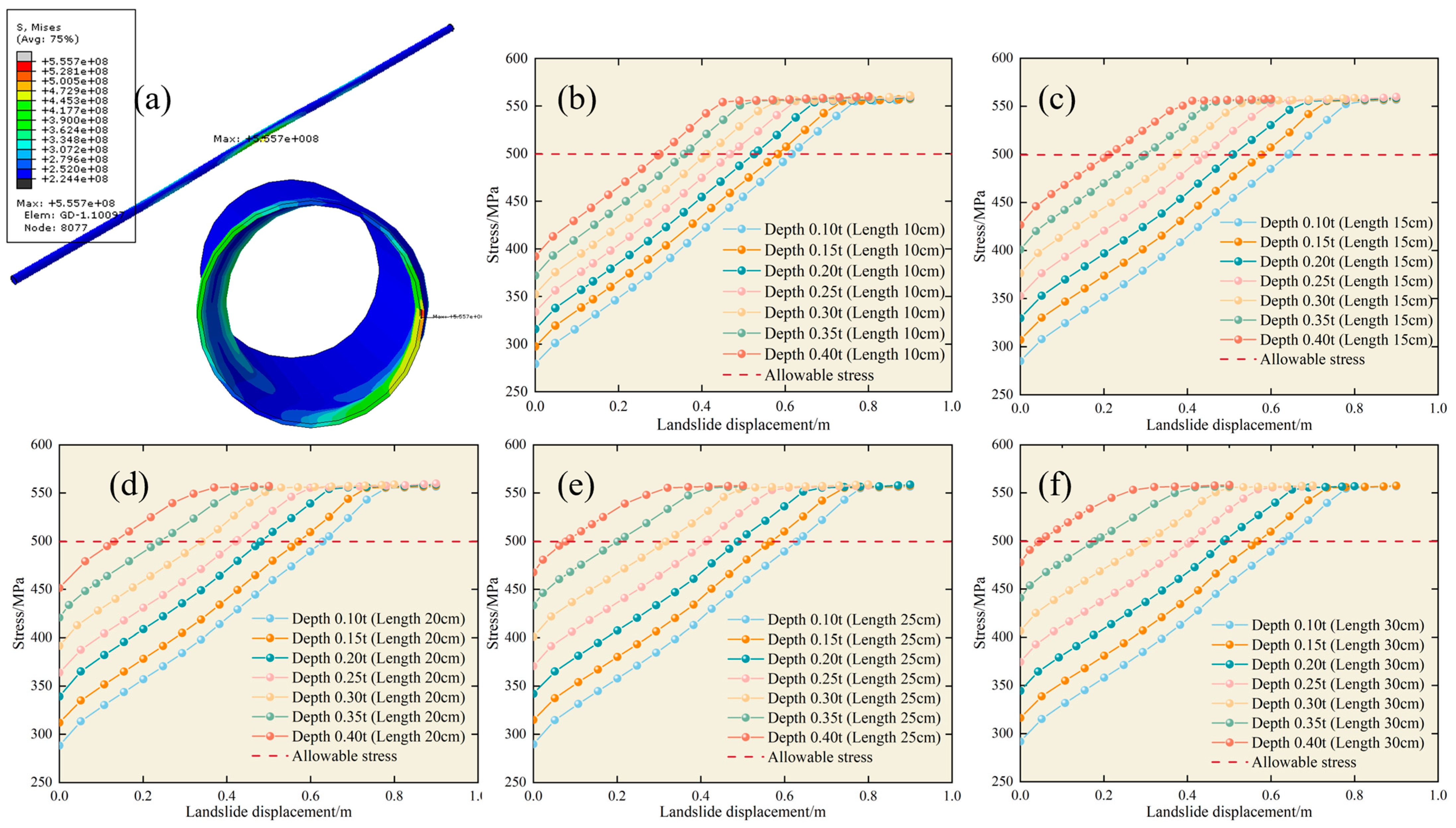
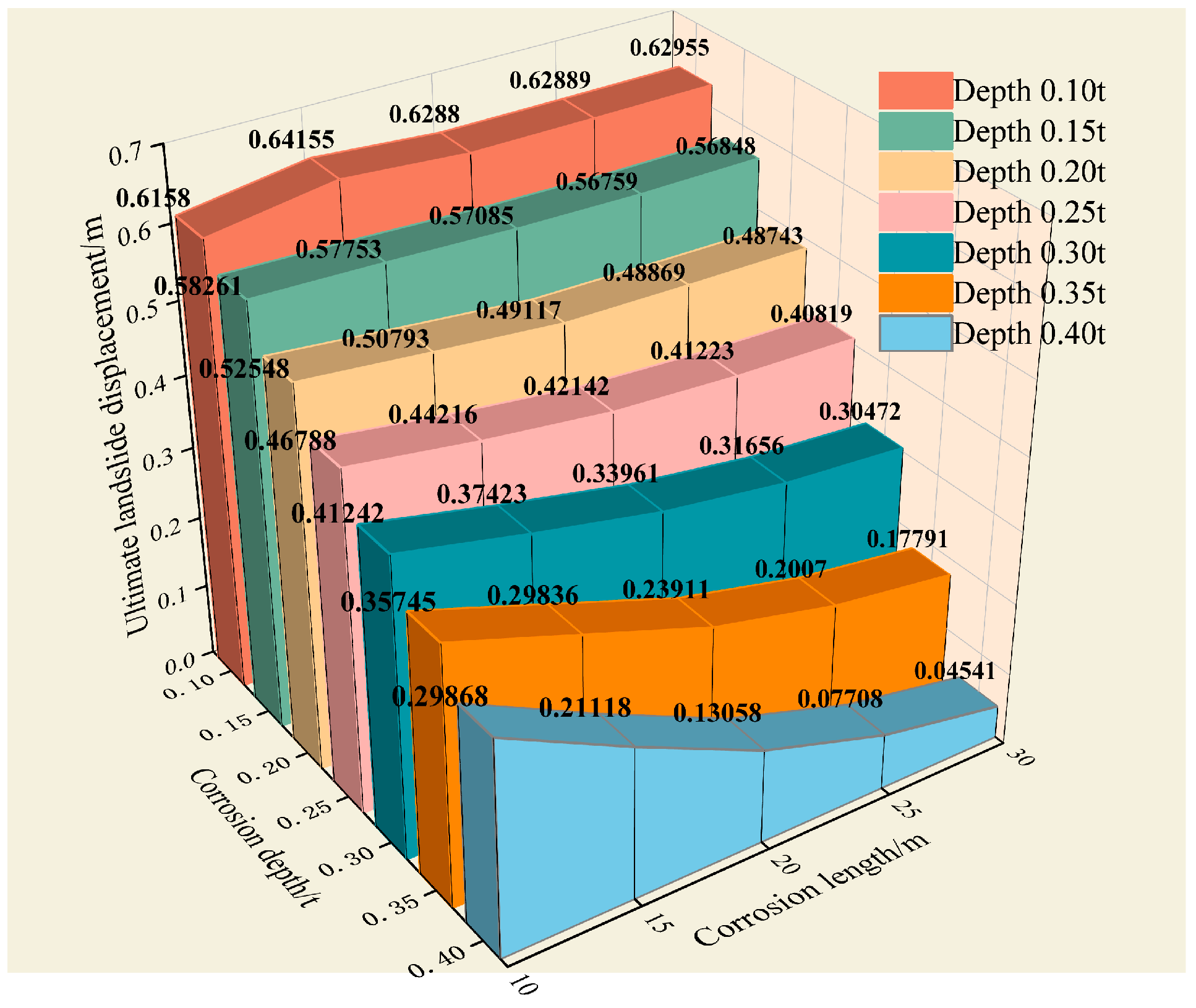
4.2. Effect of Corrosion Dimension
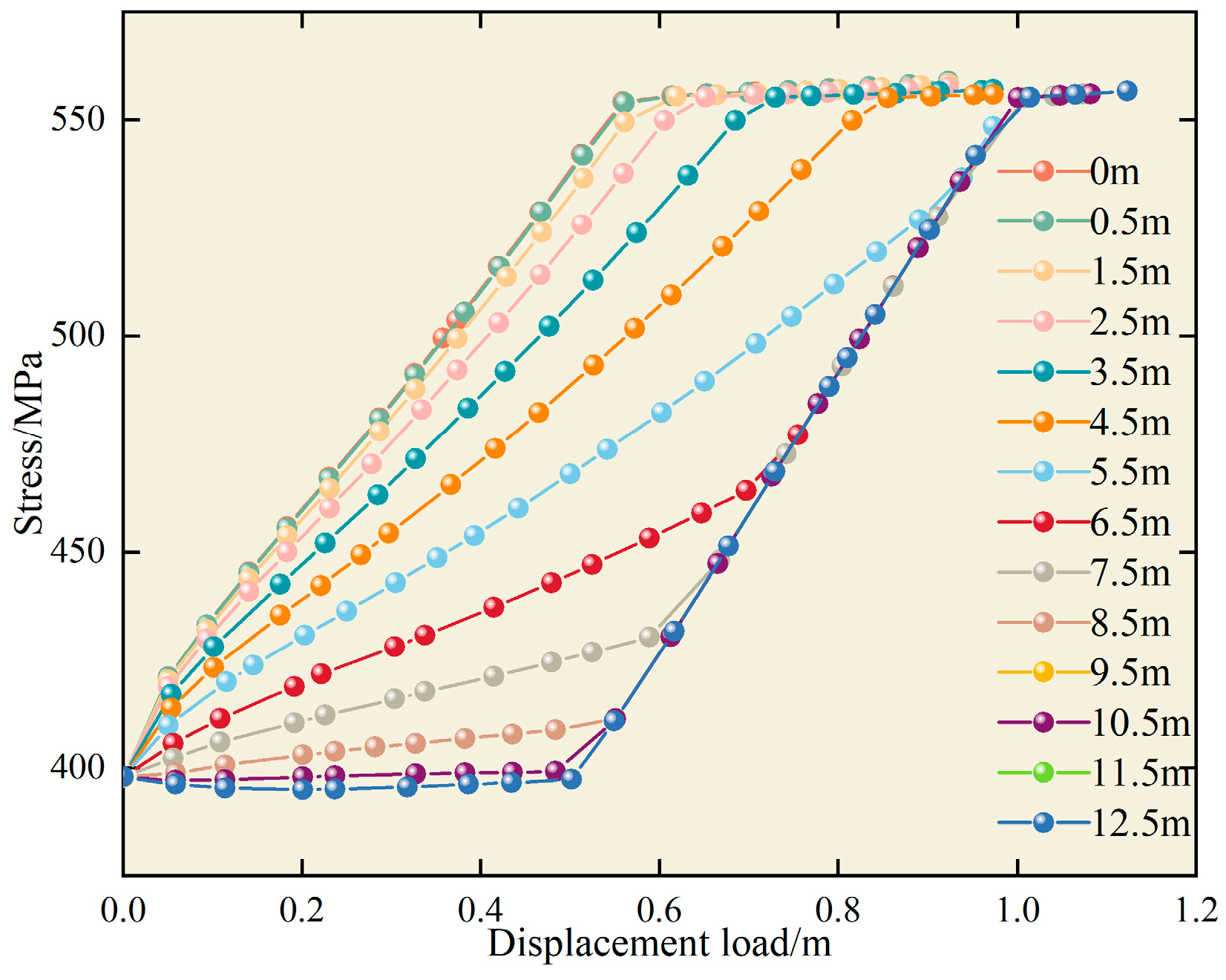
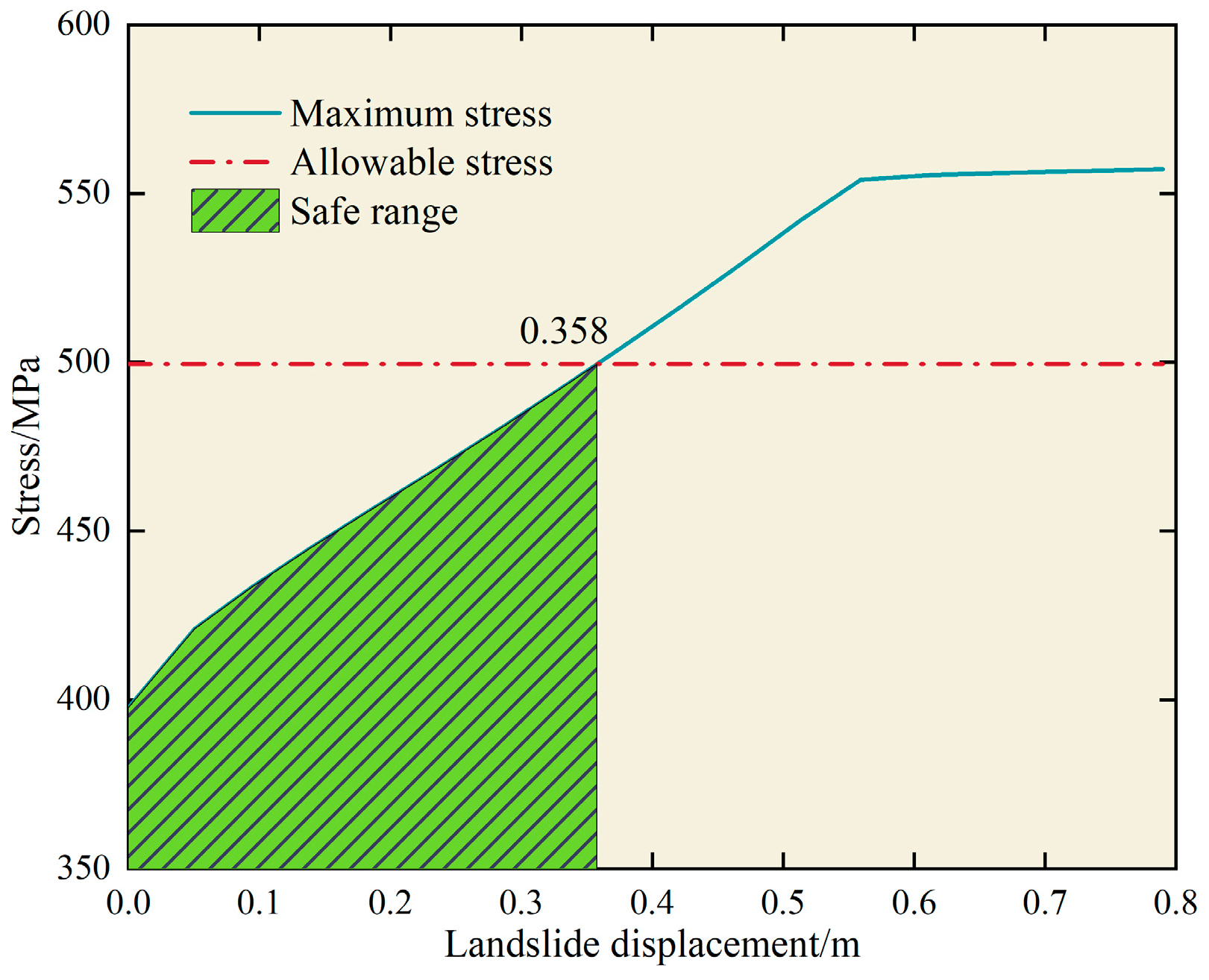
- Given a constant corrosion length and depth, with increasing landslide displacement, the maximum equivalent stress in the pipeline notably rises, stabilizing close to the yield stress of 555 MPa.
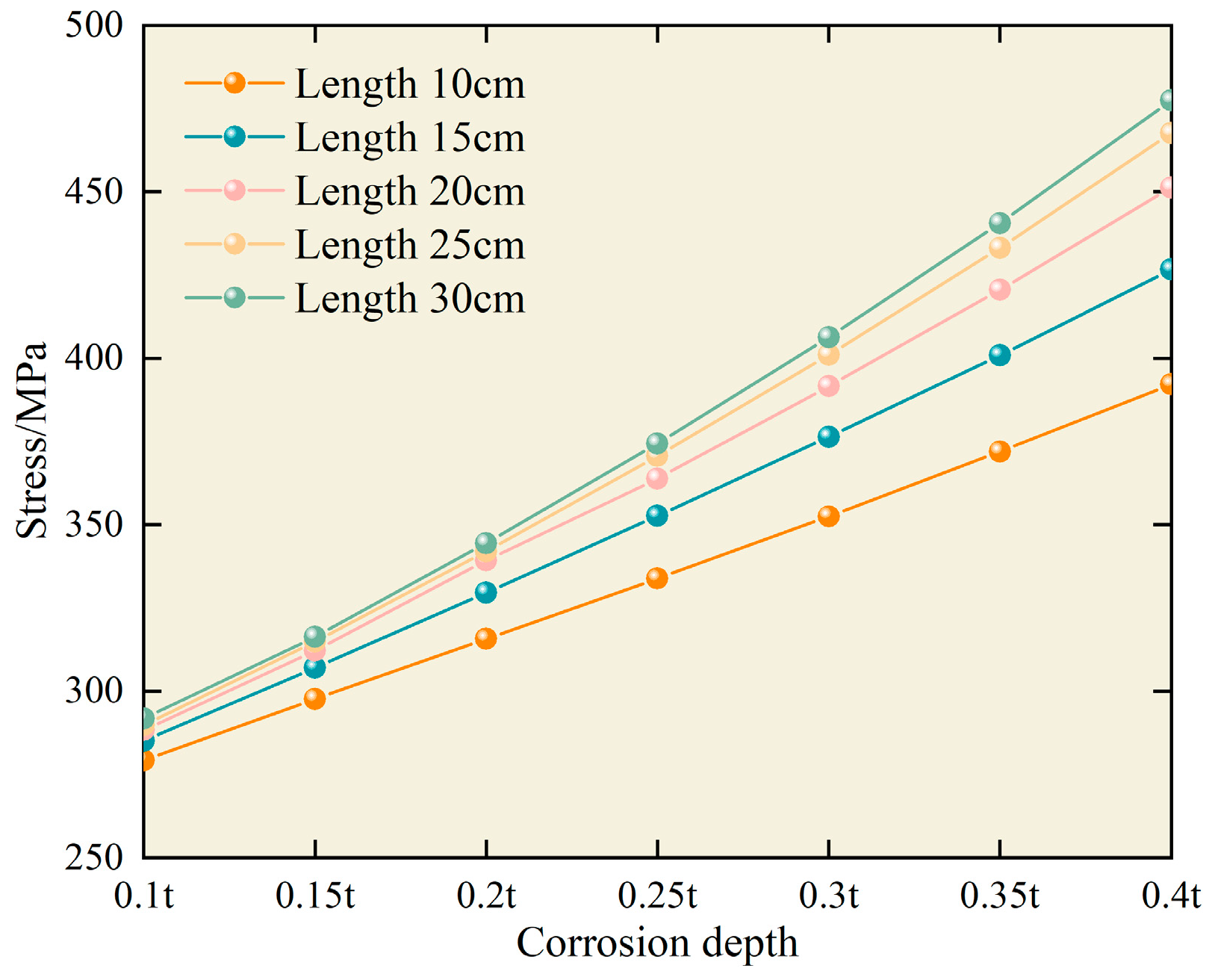
- Given a constant corrosion length and landslide displacement, an increase in the corrosion depth significantly elevates the maximum equivalent stress in the pipeline.
- Given a constant corrosion depth and landslide displacement, the corrosion length positively correlates with the maximum equivalent stress in the pipeline, albeit the correlation magnitude is not pronounced.
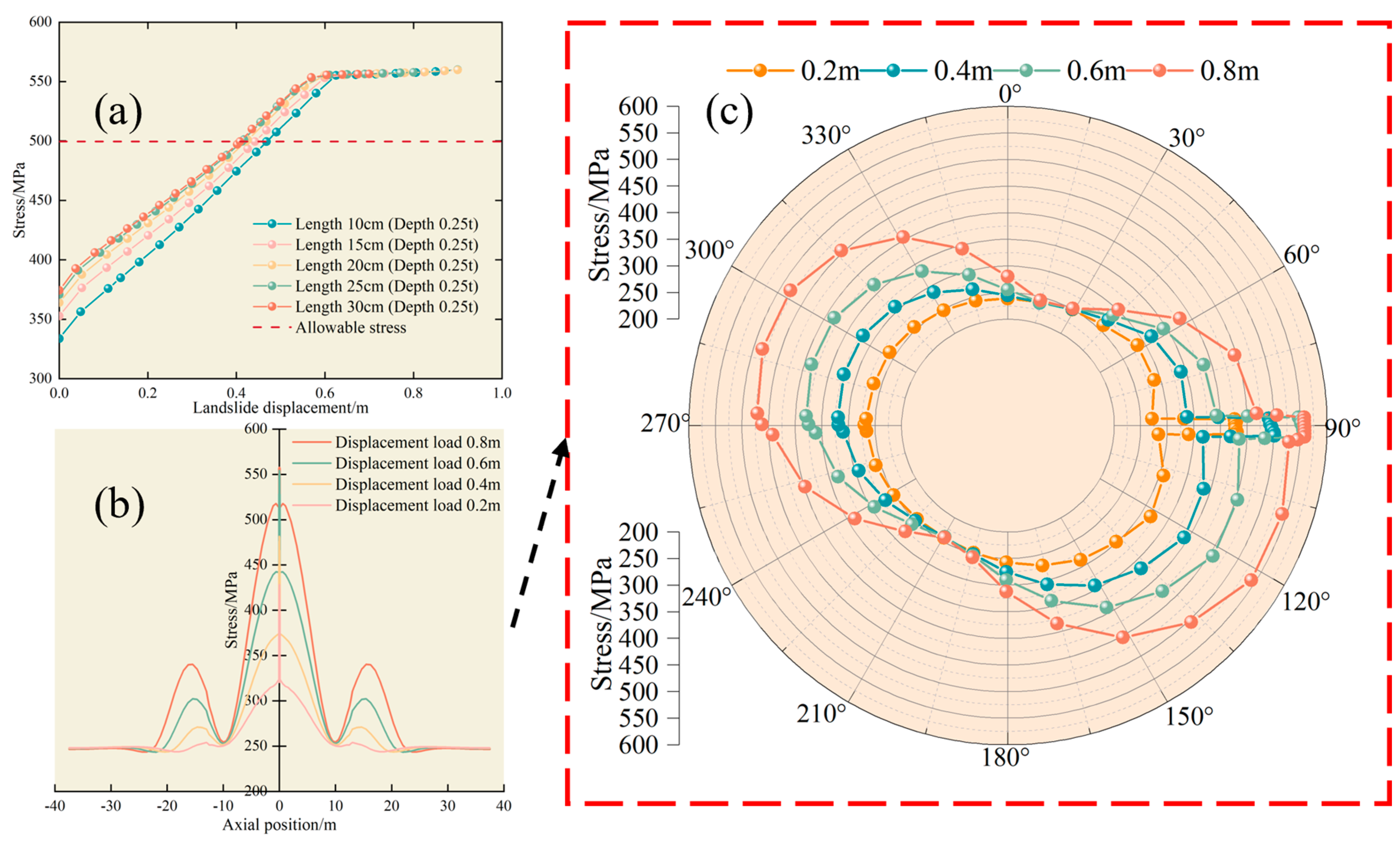
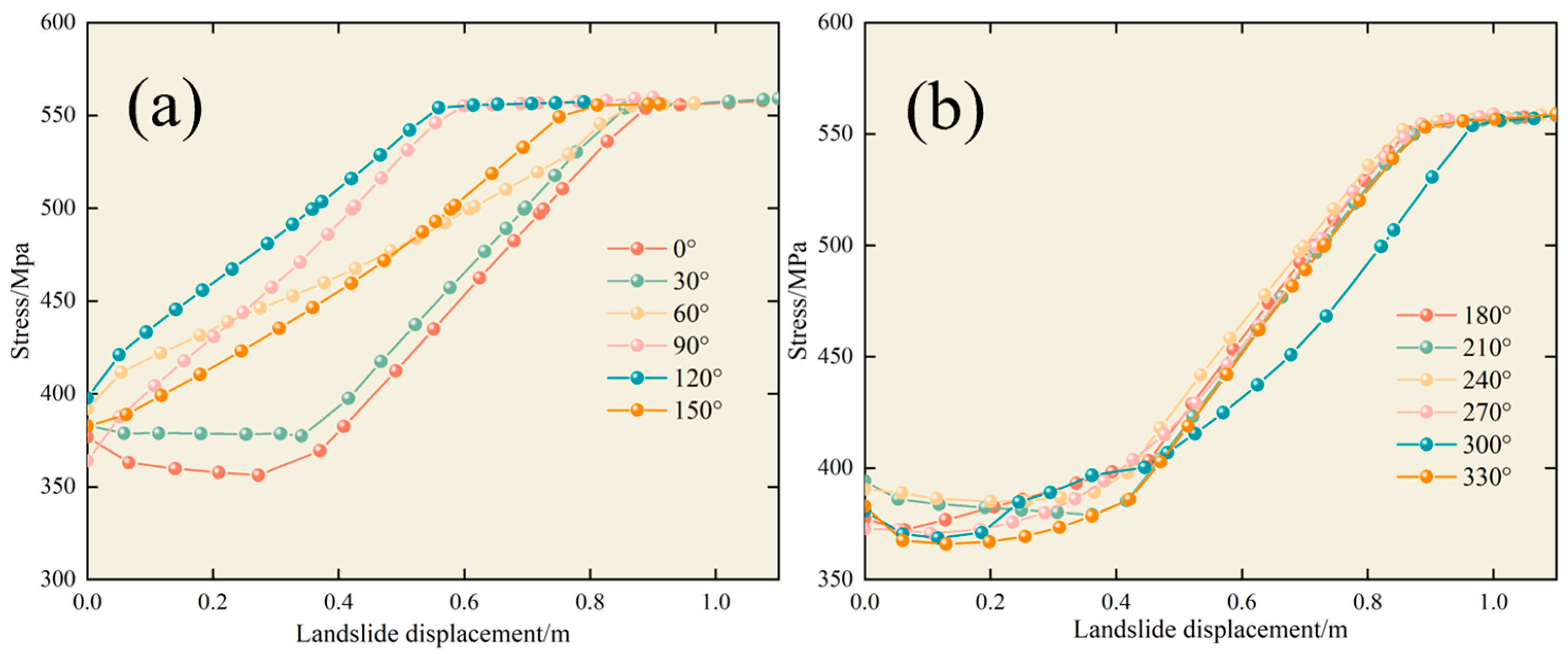
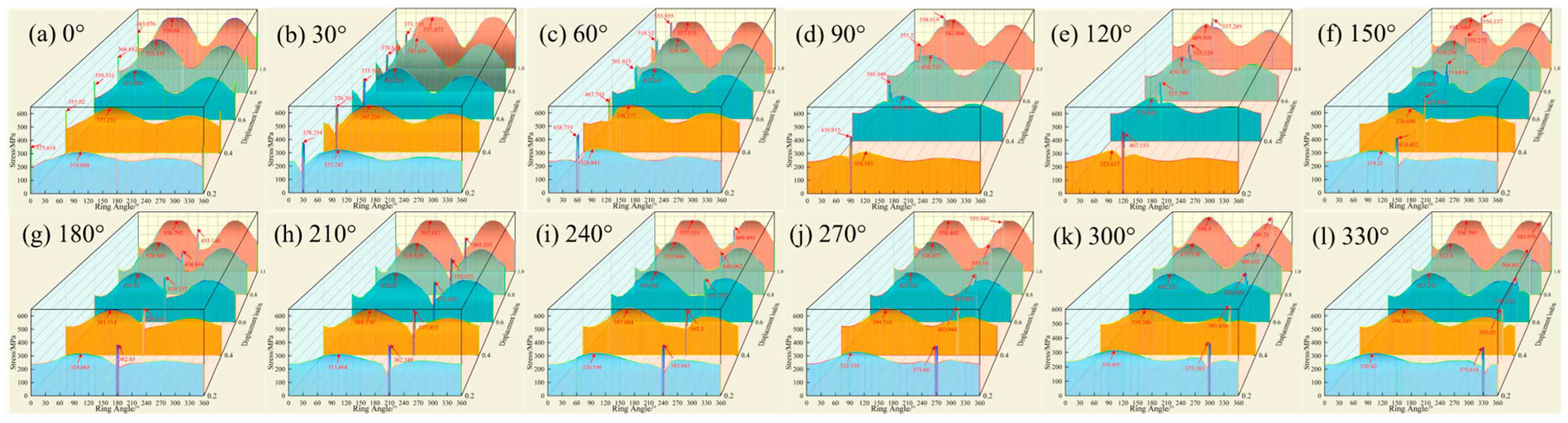
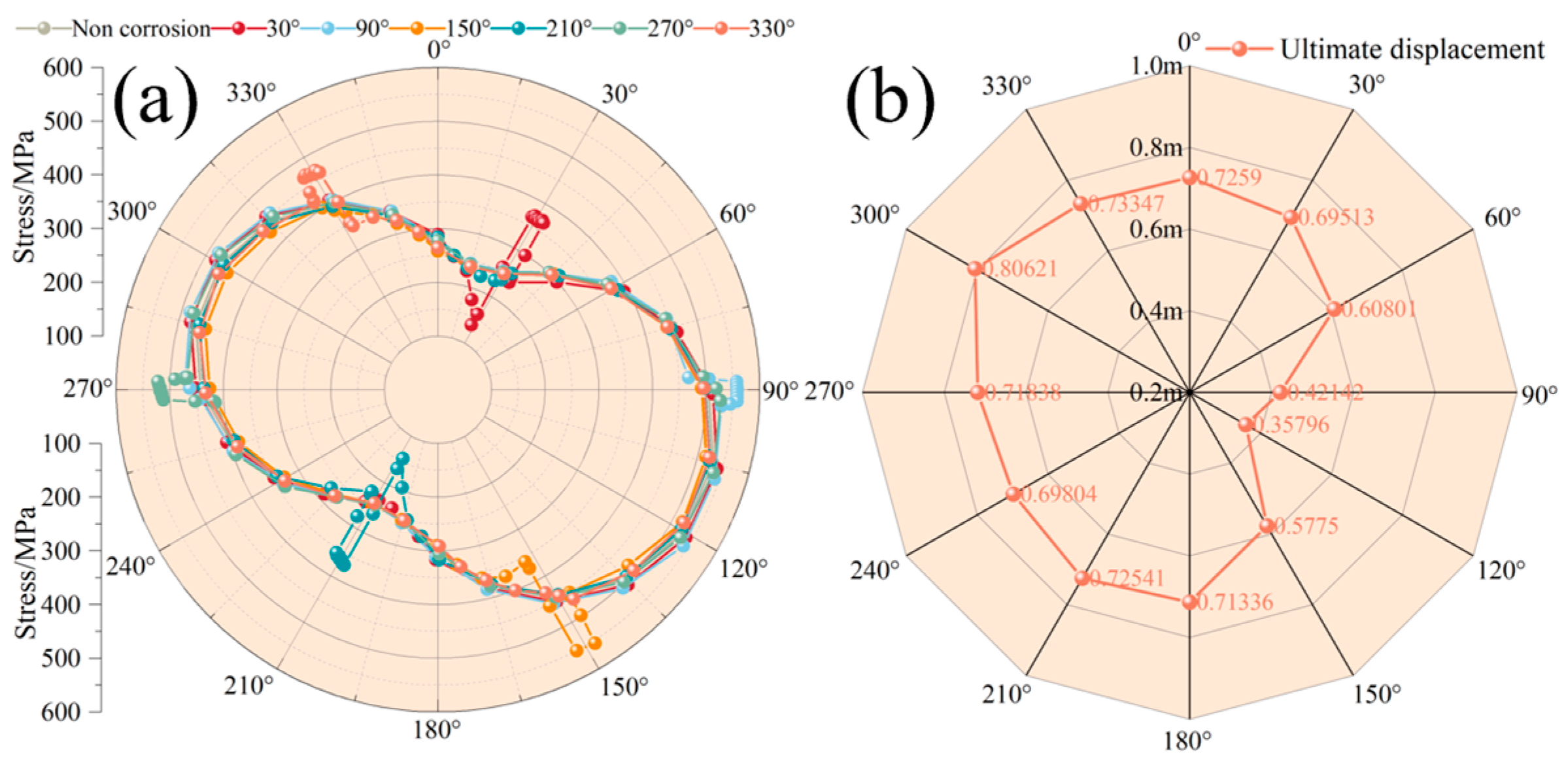
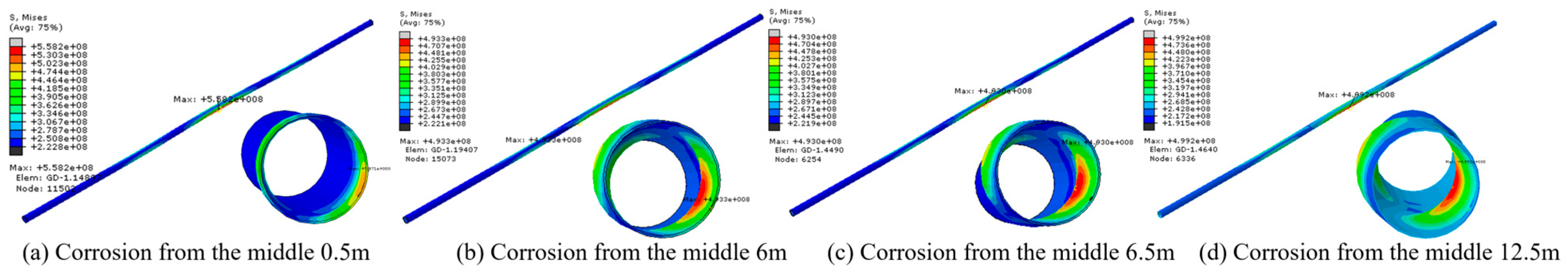
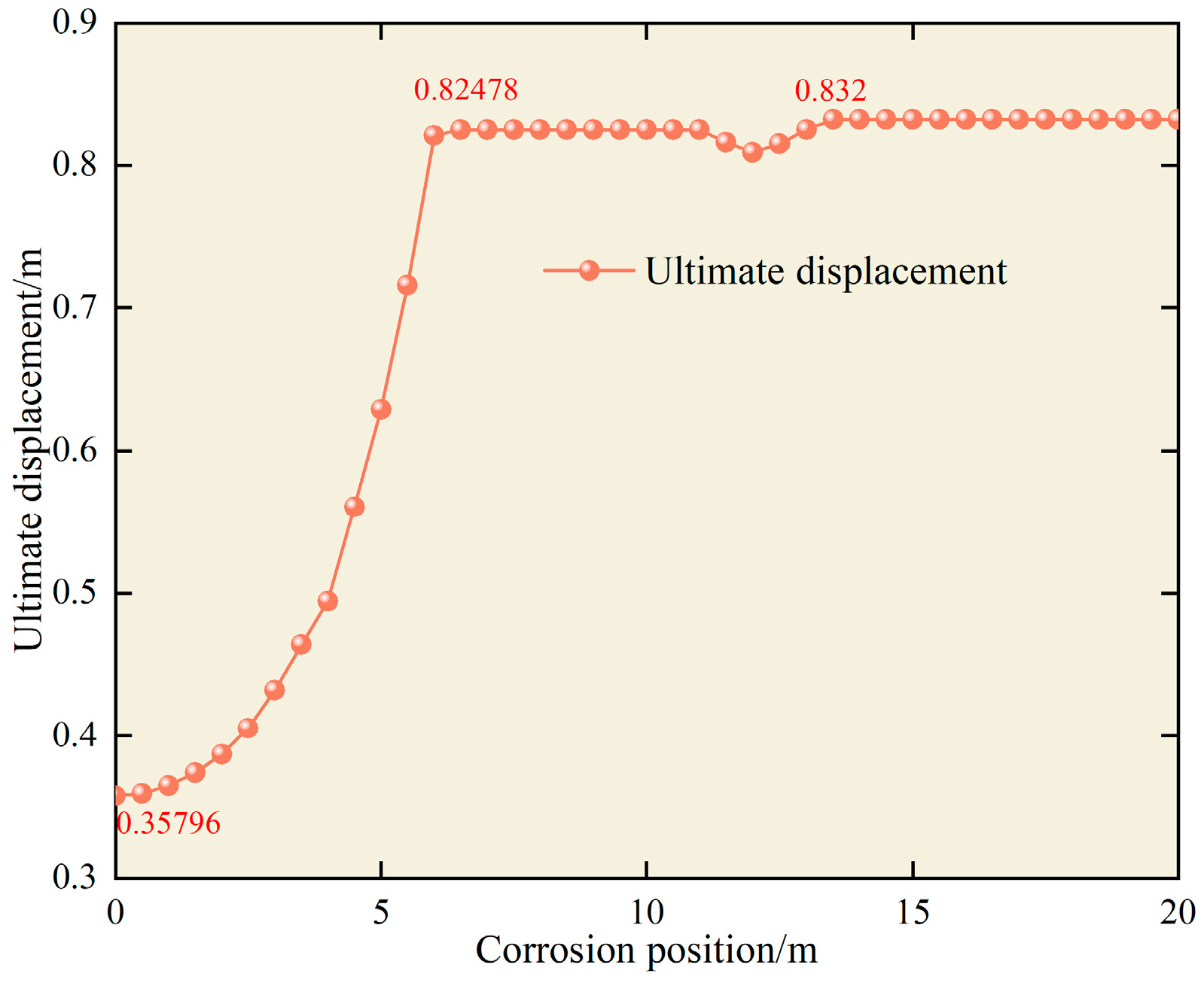
4.3. Effect of Corrosion Location
- An initial decrease followed by a consistent growth rate (at 0°, 30°, 180°, 210°, 240°, 270°, and 330°).
- An initial decrease then a gradual increase (at 300°).
- Continuous growth (at 60°, 90°, 120°, and 150°).
5. Equation Prediction
6. Conclusions
- Under landslide conditions, the maximum stress of the pipeline increases with the growth of corrosion length or depth, albeit marginally. The order of the influence of each factor on the pipeline is landslide displacement > defect depth > defect length.
- As displacement increases, the initial single-peak axial stress gradually transforms into a tri-peak stress profile (edge–center–edge) due to the combined effects of the landslide-induced soil thrust and soil shear at the edge. The angle facing the slide becomes the most vulnerable part of the pipeline, warranting close attention in engineering projects.
- There is an interplay between the stress concentration and the increasing corroded area when the corrosion defect is small in depth and length. This interplay can lead to a short-term increase in the limited landslide displacement, followed by a gradual decrease.
- For the defect at different circumferential positions in the pipeline, the maximum stress location alternates between the slide-facing side and the defect. When the defect is near the slide-facing angle, this side becomes the most critical, when situated between the slide-facing and slide-backing sides, the maximum stress remains on the slide-facing side, and when the defect is located near or at the slide-backing side, the stress at the defect exceeds that of the slide-facing side.
- When the defect is not central within the landslide, the stress at the defect exceeds that at the landslide center. Yet, when the defect shifts a certain distance from the center, the landslide’s central influence prevails, making the defect location the most hazardous point of maximum stress. Moreover, its stress decreases as the defect distance from the center increases, but in edge areas, shearing effects cause some increases in stress.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Dx | The soil displacement at position x from the landslide center, m |
| Dc | The soil displacement at the landslide center, m |
| w1 and w2 | The distances from the two ends of the landslide to its center, m |
| γ1 | The deflection at position x from the landslide center, m |
| q(x) | The lateral soil pressure at that position, KN |
| T | The axial tension, KN |
| E | Elastic modulus, MPa |
| I | The moment of inertia, m4 |
| μ | Poisson ratio |
| D | The X80 pipeline outside diameter, mm |
| t | The X80 pipeline wall thickness, mm |
| [σ] | The allowable stress of X80 pipeline, MPa |
| σs | The yield stress of X80 pipeline, MPa |
| γ | The bulk density of soil, KN/m3 |
| Φ | The internal friction angle of soil, ° |
| C | The cohesion of soil, KPa |
| xc | The length of the corrosion defect, mm |
| xs | The depth of the corrosion defect, mm |
| σ | The equivalent stress of the pipeline, MPa |
| σ0 | The stress value of the intact pipeline under normal operation, MPa |
| x | The landslide displacement, m |
| Lc | The distance of the pipeline from the landslide crack, m |
| R | The correlation coefficient |
| R2 | The coefficient of determination |
| MSE | Mean squared error |
| F-test | The joint hypotheses test |
References
- Qin, G.; Cheng, Y.F. Modeling of mechano-electrochemical interaction at a corrosion defect on a suspended gas pipeline and the failure pressure prediction. Thin-Walled Struct. 2021, 160, 107404. [Google Scholar] [CrossRef]
- Yan, Y.; Zhou, J.; Xie, C.; Yin, S.; Hu, S.; Wang, R. Quantitative estimation of pipeline slope disaster risk in China. Int. J. Disaster Risk Sci. 2023, 14, 298–312. [Google Scholar] [CrossRef]
- Liang, G.; Zhang, X.; Ling, X.; Zhou, H.; Lin, W. Analysis of temporal-spatial characteristics of geological disasters in China from 2009 to 2019. J. Disaster Prev. Reduct. 2021, 37, 58–64. [Google Scholar] [CrossRef]
- Wang, Y.; Xia, A.; Qin, G. Probabilistic modeling for reliability analysis of buried pipelines subjected to spatiotemporal earthquakes. Probabilistic Eng. Mech. 2022, 69, 103315. [Google Scholar] [CrossRef]
- Wang, Y.; Zhang, P.; Hou, X.Q.; Qin, G. Failure probability assessment and prediction of corroded pipeline under earthquake by introducing in-line inspection data. Eng. Fail. Anal. 2020, 115, 104607. [Google Scholar] [CrossRef]
- Kozhaeva, K.V.; Azmetov, K.A.; Pavlova, Z.K. Analysis of the general stability of buried pipelines in the longitudinal direction taking into account the peculiarities of their construction and operation. IOP Conf. Ser. Earth Environ. Sci. 2022, 988, 052001. [Google Scholar] [CrossRef]
- Rowe, R.K.; Davis, E.H. The behaviour of anchor plates in clay. Geotechnique 1982, 32, 9–23. [Google Scholar] [CrossRef]
- Rajani, B.B.; Robertson, P.K.; Morgenstern, N.R. Simplified design methods for pipelines subject to transverse and longitudinal soil movements. Can. Geotech. J. 1995, 32, 309–323. [Google Scholar] [CrossRef]
- O’Rourke, M.J.; Liu, X.; Flores-Berrones, R. Steel pipe wrinkling due to longitudinal permanent ground deformation. J. Transp. Eng. 1995, 121, 443–451. [Google Scholar] [CrossRef]
- Chan, P.D. Soil: Pipeline Interaction in Slopes. Master’s Thesis, University of Calgary, Calgary, AB, Canada, 1999. Available online: http://hdl.handle.net/1880/42245 (accessed on 11 November 2022).
- Trifonov, O.V.; Cherniy, V.P. A semi-analytical approach to a nonlinear stress–strain analysis of buried steel pipelines crossing active faults. Soil Dyn. Earthq. Eng. 2010, 30, 1298–1308. [Google Scholar] [CrossRef]
- Trifonov, O.V.; Cherniy, V.P. Elastoplastic stress–strain analysis of buried steel pipelines subjected to fault displacements with account for service loads. Soil Dyn. Earthq. Eng. 2012, 33, 54–62. [Google Scholar] [CrossRef]
- Yuan, F.; Wang, L.; Guo, Z.; Shi, R. A refined analytical model for landslide or debris flow impact on pipelines. Part I: Surface pipelines. Appl. Ocean Res. 2012, 35, 95–104. [Google Scholar] [CrossRef]
- Li, C.; Wang, L.; Jing, H.; Liu, Q. Protection control scheme and evaluation of effects on pipeline crossing beneath landslide area. J. Pipeline Syst. Eng. Pract. 2013, 4, 41–48. [Google Scholar] [CrossRef]
- Wu, X.; Lu, H.; Huang, K.; Wu, S.; Qiao, W. Frequency spectrum method-based stress analysis for oil pipelines in earthquake disaster areas. PLoS ONE 2015, 10, 0115299. [Google Scholar] [CrossRef] [PubMed]
- Liu, J.; Tian, J.; Yi, P. Impact forces of submarine landslides on offshore pipelines. Ocean Eng. 2015, 95, 116–127. [Google Scholar] [CrossRef]
- Liu, P.; Zheng, J.; Zhang, B.; Shi, P. Failure analysis of natural gas buried X65 steel pipeline under deflection load using finite element method. Mater. Des. 2010, 31, 1384–1391. [Google Scholar] [CrossRef]
- Chaudhuri, C.H.; Choudhury, D. Buried pipeline subjected to seismic landslide: A simplified analytical solution. Soil Dyn. Earthq. Eng. 2020, 134, 106155. [Google Scholar] [CrossRef]
- Li, H.-J.; Zhu, H.-H.; Zhang, C.-X.; Zhang, W. Modelling and analysing failure modes of buried pipelines perpendicularly crossing landslide boundaries. Soil Dyn. Earthq. Eng. 2022, 162, 107447. [Google Scholar] [CrossRef]
- Audibert, J.M.; Kenneth, J.N. Soil restraint against horizontal motion of pipes. J. Geotech. Eng. Div. 1977, 103, 1119–1142. [Google Scholar] [CrossRef]
- Calvetti, F.; Claudio, D.P.; Roberto, N. Experimental and numerical analysis of soil–pipe interaction. J. Geotech. Geoenvironmental Eng. 2004, 130, 1292–1299. [Google Scholar] [CrossRef]
- Wijewickreme, D.; Karimian, H.; Honegger, D. Response of buried steel pipelines subjected to relative axial soil movement. Can. Geotech. J. 2009, 46, 735–752. [Google Scholar] [CrossRef]
- Lin, D.; Lei, Y.; Xu, K.; Huang, R.; Zhu, Y.; Luo, M.; Tao, H. An experiment on the effect of a transverse landslide on pipelines. Acta Pet. Sin. 2011, 32, 728–732. [Google Scholar] [CrossRef]
- Sarvanis, G.C.; Karamanos, S.A.; Vazouras, P.; Mecozzi, E.; Lucci, A.; Dakoulas, P. Permanent earthquake-induced actions in buried pipelines: Numerical modeling and experimental verification. Earthq. Eng. Struct. Dyn. 2018, 47, 966–987. [Google Scholar] [CrossRef]
- Huang, Y.; Qin, G.; Hu, G. Failure pressure prediction by defect assessment and finite element modelling on pipelines containing a dent-corrosion defect. Ocean Eng. 2022, 266, 112875. [Google Scholar] [CrossRef]
- Li, N. Principle and direct assessment of internal corrosion of gas pipelines. Corros. Prot. 2013, 34, 362–366. [Google Scholar]
- Chen, Y.; Hou, F.; Dong, S.; Guo, L.; Xia, T.; He, G. Reliability evaluation of corroded pipeline under combined loadings based on back propagation neural network method. Ocean Eng. 2022, 262, 111910. [Google Scholar] [CrossRef]
- Liang, P.; Li, X.; Du, C.; Chen, X. Stress corrosion cracking of X80 pipeline steel in simulated alkaline soil solution. Mater. Des. 2009, 30, 1712–1717. [Google Scholar] [CrossRef]
- Wasim, M.; Djukic, M.B. External corrosion of oil and gas pipelines: A review of failure mechanisms and predictive preventions. J. Nat. Gas Sci. Eng. 2022, 100, 104467. [Google Scholar] [CrossRef]
- Huang, Y.; Qin, G.; Yang, M. A risk-based approach to inspection planning for pipelines considering the coupling effect of corrosion and dents. Process Saf. Environ. Prot. 2023, 180, 588–600. [Google Scholar] [CrossRef]
- Netto, T.; Ferraz, U.; Estefen, S. The effect of corrosion defects on the burst pressure of pipelines. J. Constr. Steel Res. 2005, 61, 1185–1204. [Google Scholar] [CrossRef]
- Shuai, Y.; Wang, X.-H.; Cheng, Y.F. Modeling of local buckling of corroded X80 gas pipeline under axial compression loading. J. Nat. Gas Sci. Eng. 2020, 81, 103472. [Google Scholar] [CrossRef]
- Ma, H.; Zhang, W.; Wang, Y.; Ai, Y.; Zheng, W. Advances in corrosion growth modeling for oil and gas pipelines: A review. Process Saf. Environ. Prot. 2023, 171, 71–86. [Google Scholar] [CrossRef]
- Wang, W.; Zhang, Y.; Shuai, J.; Shuai, Y.; Shi, L.; Lv, Z.-Y. Mechanical synergistic interaction between adjacent corrosion defects and its effect on pipeline failure. Pet. Sci. 2023, 20, 2452–2467. [Google Scholar] [CrossRef]
- Qin, G.; Huang, Y.; Wang, Y.; Cheng, Y.F. Pipeline condition assessment and finite element modeling of mechano-electrochemical interaction between corrosion defects with varied orientations on pipelines. Tunn. Undergr. Space Technol. 2023, 136, 105101. [Google Scholar] [CrossRef]
- Zhang, P.; Tang, X.; Li, H.; Liu, S. Limit width analysis of X80 corroded pipeline pass through landslide. Chin. J. Geol. Hazard Control 2022, 33, 47–54. [Google Scholar] [CrossRef]
- Arumugam, T.; Karuppanan, S.; Ovinis, M. Finite element analyses of corroded pipeline with single defect subjected to internal pressure and axial compressive stress. Mar. Struct. 2020, 72, 102746. [Google Scholar] [CrossRef]
- Kang, J.; Frazie, P.; Chai, H.Y. Soil–structure interaction for deeply buried corrugated steel pipes Part I: Embankment installation. Eng. Struct. 2008, 30, 384–392. [Google Scholar] [CrossRef]
- Gu, X.; Zhang, Y.; Huang, C.; Luo, X.; Zhang, H.; Zhou, R.; Qiu, Y. Sensitivity Analysis of Influencing Factors of Gas Pipelines with Corrosion Defects under the Action of Landslides. Energies 2022, 15, 6640. [Google Scholar] [CrossRef]
- Zhu, X.; Chen, J.; Du, Y. Limit load prediction analysis of X80 pipeline containing corrosion in mountainous landslide section. Geoenergy Sci. Eng. 2023, 229, 212107. [Google Scholar] [CrossRef]
- Liu, S.; Zhang, P.; Tang, Q.; Wu, S.; Huang, Y. A novel safety early warning methodology for pipelines under landslide geological hazard. J. Pipeline Syst. Eng. Pract. 2024, 15, 04023050. [Google Scholar] [CrossRef]


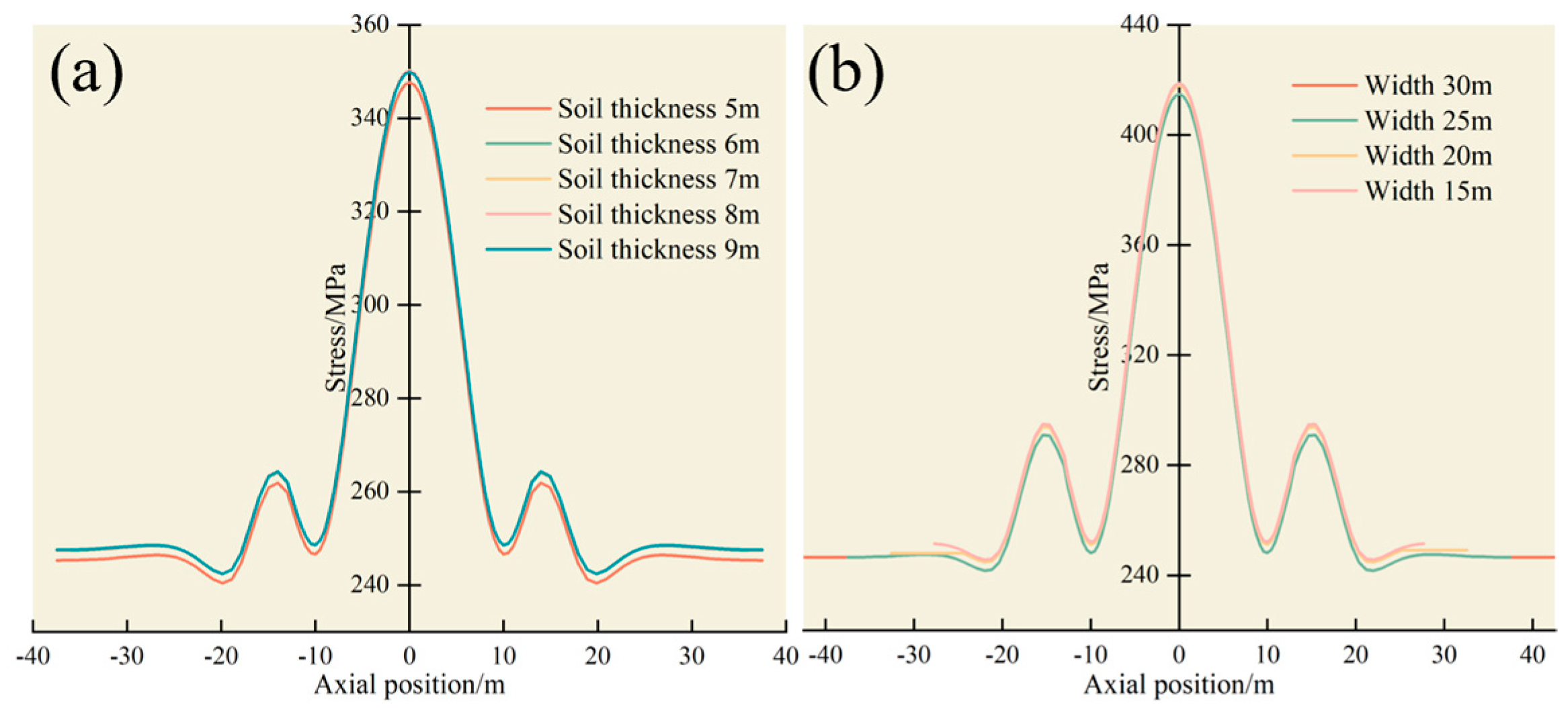
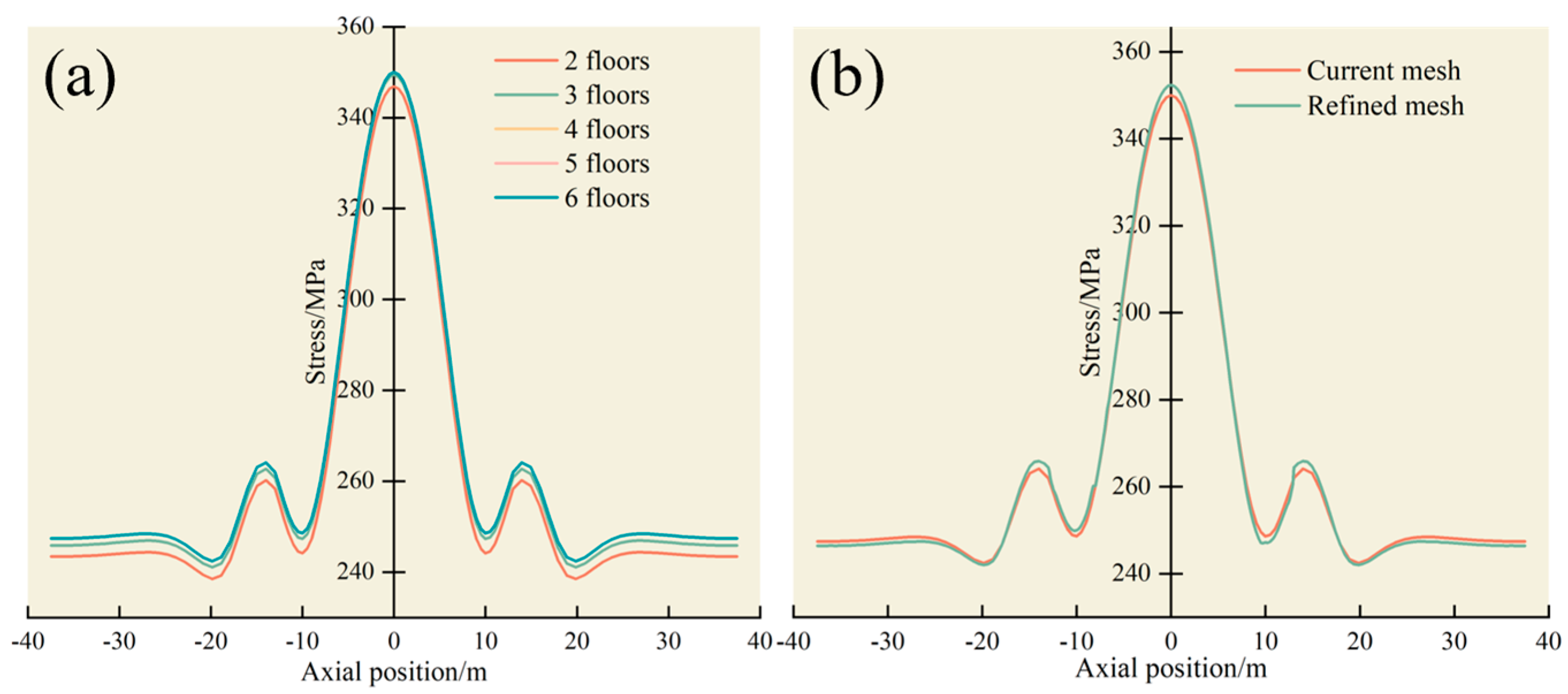






| ρ (kg/m3) | E (MPa) | μ | D (mm) | T (mm) | [σ] (MPa) | σs (MPa) |
|---|---|---|---|---|---|---|
| 7850 | 207,000 | 0.3 | 1016 | 18.2 | 499 | 555 |
| Parameters | E (MPa) | μ | γ (kN/m3) | Φ (°) | C (kPa) |
|---|---|---|---|---|---|
| Landslide zone | 32.5 | 0.4 | 20 | 10 | 15 |
| Non-landslide zone | 32.5 | 0.35 | 20 | 25 | 20 |
| Model | R | R2 | MSE | F-Test |
|---|---|---|---|---|
| Test value | 0.9928 | 0.9679 | 9.88 | 321.95 |
| Model | R | R2 | MSE | F-Test |
|---|---|---|---|---|
| Test value | 0.9943 | 0.9732 | 4.7977 | 49.7363 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, P.; Liu, W.; Liu, S.; Xu, T.; Li, Y.; Huang, Y. Safety Analysis and Condition Assessment of Corroded Energy Pipelines under Landslide Disasters. Appl. Sci. 2023, 13, 12880. https://doi.org/10.3390/app132312880
Zhang P, Liu W, Liu S, Xu T, Li Y, Huang Y. Safety Analysis and Condition Assessment of Corroded Energy Pipelines under Landslide Disasters. Applied Sciences. 2023; 13(23):12880. https://doi.org/10.3390/app132312880
Chicago/Turabian StyleZhang, Peng, Wei Liu, Siming Liu, Tian Xu, Yimiao Li, and Yunfei Huang. 2023. "Safety Analysis and Condition Assessment of Corroded Energy Pipelines under Landslide Disasters" Applied Sciences 13, no. 23: 12880. https://doi.org/10.3390/app132312880
APA StyleZhang, P., Liu, W., Liu, S., Xu, T., Li, Y., & Huang, Y. (2023). Safety Analysis and Condition Assessment of Corroded Energy Pipelines under Landslide Disasters. Applied Sciences, 13(23), 12880. https://doi.org/10.3390/app132312880






