Role of Piezoelectric Elements in Finding the Mechanical Properties of Solid Industrial Materials
Abstract
:1. Introduction
2. Using Piezoelectricity to Calculate Solid-Material Properties
2.1. Creep
2.2. Fracture Toughness
2.3. Hardness
2.4. Impact Toughness
2.5. Longitudinal Velocity of Sound
2.6. Modulus of Elasticity
2.7. Poisson’s Ratio
2.8. Shear Modulus of Elasticity
2.9. Tensile Strength
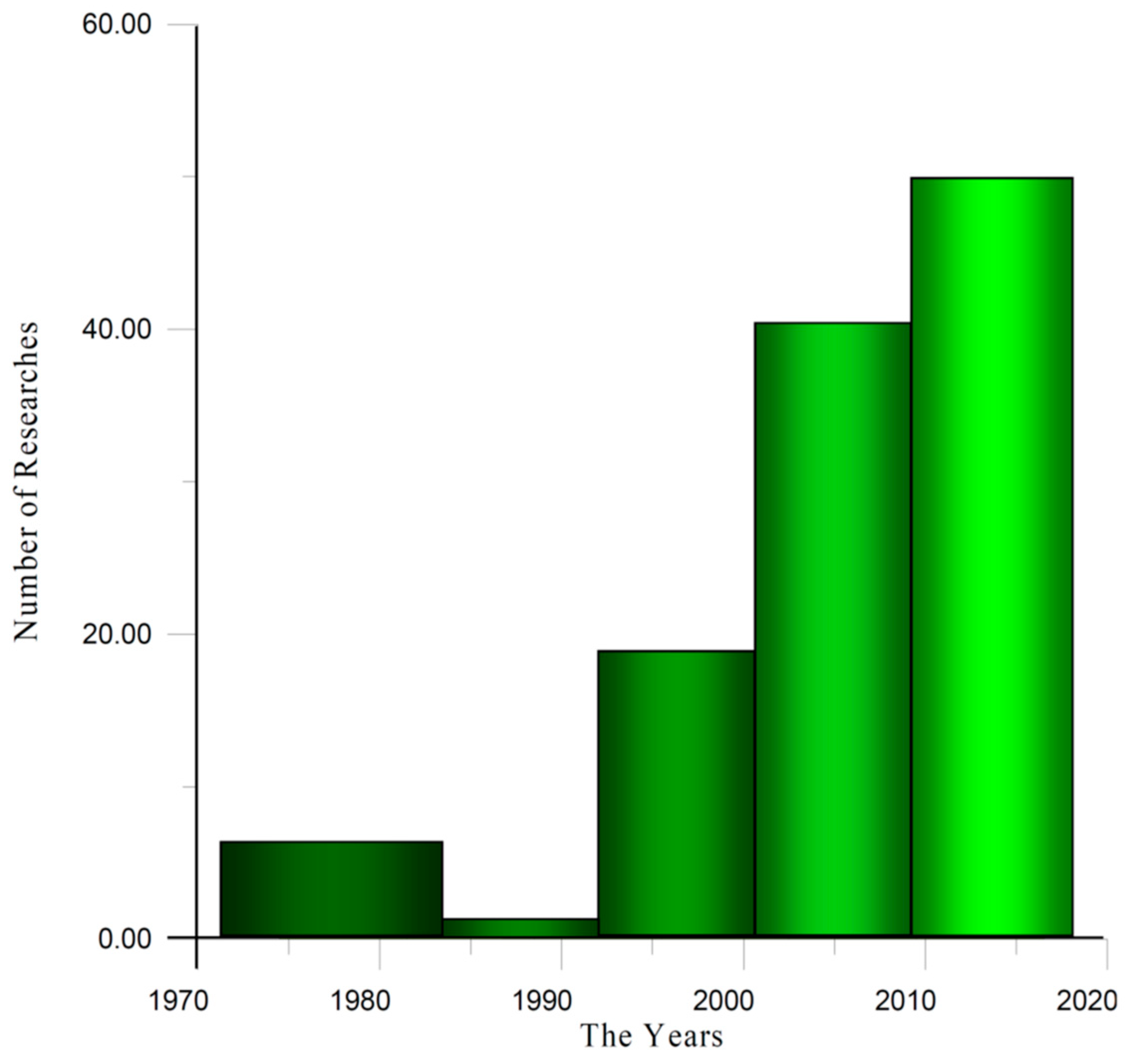
3. Statistical Study and Discussion
4. Conclusions
- Most of the ultrasonic creep tests have preferred of using PVDF because it can sense: (a) the low and high vibrations; (b) the slight changes in stress for more than 10 years and 100 million pressure cycles; (c) and can be used in a wide temperature range (−40 °C to +160 °C).
- PE can eliminate any inertia effects associated with the higher velocity; therefore, many applications focus on using the PEs as a load cell, instead of any type of actuators, in quasi-static dynamic fracture toughness tests.
- PE could be used as a strain gage to obtain real-time information such as force-versus-time behavior for the material and the magnitude of peak force, which Charpy and Izod cannot provide us in impact tests.
- The advantages of using piezoelectric accelerometers over other types of accelerometers in impact tests are the broad frequency range, good linearity in dynamic applications and performance in a wider range of environmental conditions, and the ability of data to be integrated.
- There is an inverse relationship between the dimensions of PE and the longitudinal velocity generated from it.
- The resonant piezoelectric shunts can be used to affect the equivalent mechanical characteristics of an elastic waveguide and suggests their application for enhancing the tunable band gaps.
- Tests of the shear modulus of elasticity, putting the PEs at the opposite corners for specimens that have cube shapes or at opposite edges for the short cylinders, show (a) weakness in elastically coupling for the piezoelectric transducers (b) reducing the perturbation of the vibration (c) minimal shift in resonant frequency, and finally (d) minimum parasitic damping. The main challenge of the use of PE in finding mechanical properties are that these tests do not include all mechanical properties such as yield stresses, ultimate tensile stress, and melting point etc. In addition, there are some of difference in results, for some of the special materials such as radiation materials, between the result coming from these tests (PE tests) from one side, and from traditional tests from the other side. Therefore, these tests need more study and research.
5. Recommendations
- Study using PE in finding other properties of materials such as yield stress, tensile strength and melting point.
- Study using PE in finding the materials properties in high temperature.
Author Contributions
Funding
Conflicts of Interest
References
- Weifang Jude Electronic Co., Ltd. The Piezoelectric Effect in Ceramic Materials. Available online: http://www.pztceramics.com/The-piezoelectric-effect-in-ceramic-materials-id508854.html (accessed on 15 March 2017).
- Ussef, H.; Uhlemeyer, R.; Nch, R. Development of an Apparatus for Static and Dynamic Creep Testing of Ice and Frozen Soils. Available online: http://www.docstoc.com/docs/72471918/Development-of-an-apparatus-for-static-and-dynamic-creep-testing (accessed on 21 September 2018).
- Vinogradov, A.M. Creep-Fatigue Interaction in Polymers. Rubber. Chem. Technol. 1994, 1–6. [Google Scholar]
- Shirinov, A.V.; Schomburg, W.K. Pressure sensor from a PVDF film. Sens. Actuators A Phys. 2008, 142, 48–55. [Google Scholar] [CrossRef]
- Seminara, L.; Capurro, M.; Cirillo, P.; Cannata, G.; Valle, M. Electromechanical characterization of piezoelectric PVDF polymer films for tactile sensors in robotics applications. Sens. Actuators A Phys. 2011, 169, 49–58. [Google Scholar] [CrossRef]
- Vinogradov, A.M.; Hugo Schmidt, V.; Tuthill, G.F.; Bohannan, G.W. Damping and electromechanical energy losses in the piezoelectric polymer PVDF. Mech. Mater. 2004, 36, 1007–1016. [Google Scholar] [CrossRef]
- Seminara, L.; Valle, M.; Capurro, M.; Cirillo, P.; Cannata, G. Piezoelectric polymer films for tactile sensors. In Sensors and Microsystems; D’Amico, A., Di Natale, C., Mosiello, L., Zappa, G., Eds.; Springer: Boston, MA, USA, 2012; Volume 109, pp. 169–173. [Google Scholar]
- Gyekenyesi, A.L.; Kautz, H.E.; Shannon, R.E. Quantifying creep damage in a metallic alloy using acousto-ultrasonics. J. Mater. Eng. Perform. 2002, 11, 205–210. [Google Scholar] [CrossRef]
- Ueno, K.; Nagakawa, J.; Murase, Y.; Yamamoto, N. Effect of cold work on the irradiation creep of SUS 316L. J. Nucl. Mater. 2004, 329, 602–606. [Google Scholar] [CrossRef]
- Ann, H.K.; Kim, C.S.; Shin, Y.H.; Jeon, Y.C.; Park, I.K. Nondestructive evaluation of the creep damaged Ni-based superalloy using ultrasonic wave method. In Proceedings of the Asia-Pacific Conference on NDT, Auckland, New Zealand, 5–10 November 2006. [Google Scholar]
- Rosti, J.; Koivisto, J.; Traversa, P.; Illa, X.; Grasso, J.-R.; Alava, M.J. Line creep in paper peeling. Int. J. Fract. 2008, 151, 281–297. [Google Scholar] [CrossRef]
- Virkar, A.V.; Johnson, D.L. Fracture behavior of ZrO2-Zr composites. Am. Cerr. Soc. 1977, 60, 514–519. [Google Scholar] [CrossRef]
- Tomaszewski, H.; Boniecki, M.; Weglarz, H. Toughness-curve behaviour of alumina-SiC and ZTA-SiC composites. J. Eur. Ceram. Soc. 2000, 20, 1215–1224. [Google Scholar] [CrossRef]
- Tomaszewski, H.; Strzeszewski, J.; Gȩbicki, W. The role of residual stresses in layered composites of Y–ZrO2 and Al2O3. J. Eur. Ceram. Soc. 1999, 19, 255–262. [Google Scholar] [CrossRef]
- He, J.; Clarke, D.R. Determination of the piezospectroscopic coefficients for chromium-doped sapphire. J. Am. Ceram. Soc. 1995, 78, 1347–1353. [Google Scholar] [CrossRef]
- Ramírez-Rico, J.; Martínez-Fernandez, J.; Peña, J.I.; Singh, D.; Routbort, J. Residual stresses in Al2O3–ZrO2 (3 MOL.% Y2O3) directionally solidified eutectic ceramics as a function of temperature. Mater. Sci. Eng. A 2012, 541, 61–66. [Google Scholar] [CrossRef]
- Jungk, J.M.; Boyce, B.L.; Buchheit, T.E.; Friedmann, T.A.; Yang, D.; Gerberich, W.W. Indentation fracture toughness and acoustic energy release in tetrahedral amorphous carbon diamond-like thin films. Acta Mater. 2006, 54, 4043–4052. [Google Scholar] [CrossRef]
- Félix-Bautista, R.; Hernández-Hernández, C.; Zendejas-Leal, B.E.; Fragoso, R.; Golzarri, J.I.; Vázquez-López, C.; Espinosa, G. Evolution of etched nuclear track profiles of alpha particles in CR-39 by atomic force microscopy. Radiat. Meas. 2013, 50, 197–200. [Google Scholar] [CrossRef]
- Hoffmann, J.; Schuler, A.; Weckenmann, A. Construction and evaluation of a traceable metrological scanning tunnelling microscope. Measurement 2009, 42, 1324–1329. [Google Scholar] [CrossRef]
- Starbuck, J.M.; Warren, C.D.; Carpenter, J.A.; Sklad, P.S.H. Crash Analysis of Adhesively-Bonded Structures (CAABS); Oak Ridge National Laboratory (ORNL): Oak Ridge, TN, USA, 2006.
- Salerno, M. Improved estimation of contact compliance via atomic force microscopy using a calibrated cantilever as a reference sample. Measurement 2012, 45, 2103–2113. [Google Scholar] [CrossRef]
- Belda, P.M.; Mielck, J.B. The tabletting machine as an analytical instrument: Qualification of the measurement devices for punch forces and validation of the calibration procedures. Eur. J. Pharm. Biopharm. 1998, 46, 381–395. [Google Scholar] [CrossRef]
- Schuurmans, J.; Scibetta, M. Cost-effective precracking of charpy V-notch specimens for fracture-toughness testing using a piezoelectric actuator. J. Test. Eval. 2016, 45, 1476–1483. [Google Scholar] [CrossRef]
- Mall, S. Integrity of graphite/epoxy laminate embedded with piezoelectric sensor/actuator under monotonic and fatigue loads. Smart Mater. Struct. 2002, 11, 527. [Google Scholar] [CrossRef]
- Mall, S.; Hsu, T.L. Electromechanical fatigue behavior of graphite/epoxy laminate embedded with piezoelectric sensor/actuator. Smart Mater. Struct. 2002, 9, 78. [Google Scholar] [CrossRef]
- Murri, G.B. Effect of embedded piezoelectric sensors on fracture toughness and fatigue resistance of composite laminates under mode I loading. In American Society of Composite 21st Annual Technical; NASA Langley Research Center: Hampton, VA, USA, 2006. [Google Scholar]
- Jayakumar, K.; Yadav, D.; Nageswara Rao, B. Moderately large deflection analysis of simply supported piezo-laminated composite plates under uniformly distributed transverse load. Int. J. Non-Linear Mech. 2013, 49, 137–144. [Google Scholar] [CrossRef]
- Miau, J.J.; Wu, C.W.; Hu, C.C.; Chou, J.H. A study on signal quality of a vortex flowmeter downstream of two elbows out-of-plane. Flow Meas. Instrum. 2002, 13, 75–85. [Google Scholar] [CrossRef]
- Sturm, D.; Heilmaier, M.; Schneibel, J.H.; Jéhanno, P.; Skrotzki, B.; Saage, H. The influence of silicon on the strength and fracture toughness of molybdenum. Mater. Sci. Eng. A 2007, 463, 107–114. [Google Scholar] [CrossRef]
- Patel, Y.; Blackman, B.R.K.; Williams, J.G. Determining fracture toughness from cutting tests on polymers. Eng. Fract. Mech. 2009, 76, 2711–2730. [Google Scholar] [CrossRef]
- Weerasooriya, T.; Moy, P.; Casem, D.; Cheng, M.; Chen, W. Determination of dynamic fracture toughness for brittle aterials with a modified SHPB. In Determination of Dynamic Fracture Toughness for SiC-N; The University of Arizona: Tucson, AZ, USA, 2010. [Google Scholar]
- Keshavanarayana, S.; Tan, K.Y.; Siddiqui, M.; Elyas, S.F. Crashworthiness of Composites—Material Dynamic Properties; Joint Advanced Materials & Structures Center of Excellence: San Diego, CA, USA, 20–21 April 2011. [Google Scholar]
- Deubener, J.; Höland, M.; Höland, W.; Janakiraman, N.; Rheinberger, V.M. Crack tip fracture toughness of base glasses for dental restoration glass-ceramics using crack opening displacements. J. Mech. Behav. Biomed. Mater. 2011, 4, 1291–1298. [Google Scholar] [CrossRef] [PubMed]
- Vary, A. Correlations among ultrasonic propagation factors and fracture toughness properties of metalic matterials. Mater. Eval. 1978, 36, 55–64. [Google Scholar]
- Vary, A.; Hull, D.R. Ultrasonic ranking of toughness of tungsten carbide. In National Aeronauticsand Space Administration; TM-83358; Lewis Research Center: Cleveland, OH, USA, 1983. [Google Scholar]
- Gopichand, A.; Rao, M.M.; Sharma, A.V.N.L.; Tony, V.S. Analysis and estimation of attenuation coefficient of aging en-19 steel. J. Eng. Res. Appl. (IJERA) 2012, 1, 590–595. [Google Scholar]
- Thompson, M.; Palaghian, L. Nitrogen influence on indentation fracture toughness of stainless steel coatings. In Proceedings of the International Conference on Tribology, Bucharest, Romania, 8–10 November 2007; pp. 78–82. Available online: http://www.om.ugal.ro/AnnalsFasc8Tribology/pdf/2008/067_Thompson_Romania%20xx.pdf (accessed on 26 September 2018).
- Randall, N.X.; Christoph, R.; Droz, S.; Julia-Schmutz, C. Localised micro-hardness measurements with a combined scanning force microscope/nanoindentation system. Thin Solid Films 1996, 290, 348–354. [Google Scholar] [CrossRef]
- Iwabuchi, A.; Shimizu, T.; Yoshino, Y.; Abe, T.; Katagiri, K.; Nitta, I.; Sadamori, K. The development of a vickers-type hardness tester for cryogenic temperatures down to 4.2 K. Cryogenics 1996, 36, 75–81. [Google Scholar] [CrossRef]
- Sansoz, F.; Gang, T. A force-matching method for quantitative hardness measurements by atomic force microscopy with diamond-tipped sapphire cantilevers. Ultramicroscopy 2010, 111, 11–19. [Google Scholar] [CrossRef] [PubMed]
- Vasauskas, V.; Čapas, V. Particular aspects in the calibration and application dynamic and static hardness. XVII IMEKO World Congr. 2003, TC5, 1009–1014. [Google Scholar]
- Ueda, T.; Huda, M.A.; Yamada, K.; Nakayama, K. Temperature measurement of CBN tool in turning of high hardness steel. Ann. CIRP 1999, 48, 63–66. [Google Scholar] [CrossRef]
- Mezlini, S.; Kapsa, P.; Abry, J.C.; Henon, C.; Guillemenet, J. Effect of indenter geometry and relationship between abrasive wear and hardness in early stage of repetitive sliding. Wear 2006, 260, 412–421. [Google Scholar] [CrossRef]
- Frank, S.; Schubert, H. Portable hardness testing—Principles and applications. J. Nondestruct. Test. 2002, 7, 8. [Google Scholar]
- Gutt, G.; Gutt, S.; Vasilache, V.; Severin, T.L. Research regarding the investigation of steel hardness. Roznov. Pod. Radhostem. 2004, 5, 18–20. [Google Scholar]
- Frank, S. Mobile Hardness Testing: Application Guide for Hardness Testers; GE Inspections Technologies: Skaneateles Falls, NY, USA, 2008. [Google Scholar]
- Tiniest Olsen Testing Machine Company. Universal Hardness Testing Machines. Available online: https://www.tiniusolsen.com/tinius-olsen-products/indentation-hardness-testers/univers (accessed on 21 September 2018).
- Ensminger, D.; Bond, L.J. Ultrasonics Fundamentals, Technologies and Applications, 3rd ed.; CRC Press: Boca Raton, FL, USA, 2011; p. 723. [Google Scholar]
- Qi, X.; Chen, W.; Liu, Y.; Tang, X.; Shi, S. A novel well drill assisted with high-frequency vibration using the bending mode. Sensors 2018, 18, 1167. [Google Scholar] [CrossRef] [PubMed]
- Hay, T.R. Rail Surface Characterization; IDEA: Washington, DC, USA, 2008; pp. 1–29. [Google Scholar]
- National Security Technologies, LLC. Strategic Opportunity Research Exploratory Research Site-Directed Research & Development; U.S. Department of Energy, and supported by the Site-Directed Research and Development Program; National Security Technologies, LLC: Laramie, WY, USA, 2017. [Google Scholar]
- Gutt, G.; Gutt, S.; Vasilache, V.; Severin, T.L. Research on universal dynamic hardness test. Roznov. Pod. Radhostem. 2010, 5, 1–8. [Google Scholar]
- Elahi, H.; Eugeni, M.; Gaudenzi, P.; Gul, M.; Swati, R.F. Piezoelectric thermo electromechanical energy harvester for reconnaissance satellite structure. In Microsystem Technologies; Springer: Berlin/Heidelberg, Germany, 2018. [Google Scholar]
- Elahi, H.; Eugeni, M.; Gaudenzi, P.; Qayyum, F.; Swati, R.F.; Khan, H.M. Response of piezoelectric materials on thermomechanical shocking and electrical shocking for aerospace applications. Microsyst. Technol. 2018, 24, 3791–3798. [Google Scholar] [CrossRef]
- Gaudenzi, P. Physical Behaviour, Mathematical Modelling and Applications; John-Wiley-and-Sons: Hoboken, NJ, USA, 2009. [Google Scholar]
- Korde, N.; Kundu, T. Material hardness and ageing measurement using guided ultrasonic waves. Ultrasonics 2013, 53, 506–510. [Google Scholar] [CrossRef] [PubMed]
- Chinchanikar, S.; Choudhury, S.K. Effect of work material hardness and cutting parameters on performance of coated carbide tool when turning hardened steel: An optimization approach. Measurement 2013, 46, 1572–1584. [Google Scholar] [CrossRef]
- Lendze, T.; Wojtyra, R.; Guillaumat, L.; Biateau, C.; Imielińska, K. Low velocity impact damage in glass/polyester composite sandwich panels. Adv. Mater. Sci. 2006, 6, 27–34. [Google Scholar]
- Scott, K.P. New instrumented pendulum impact system for testing plastics. Mater. Test. Prod. Technol. News 2001. [Google Scholar] [CrossRef]
- Du, G.; Li, Z.; Song, G. A PVDF-based sensor for internal stress monitoring of a concrete-filled steel tubular (CFST) column subject to impact loads. Sensors 2018, 18, 1682. [Google Scholar] [CrossRef] [PubMed]
- Ang, S.F. Evaluation Impact Strength on Particulate Reinforced (25% and 33%) Vinyl Ester Composite Cured under Microwave Conditions University of Southern Queensland; USQ: Darling Heights, Australia, 2004. [Google Scholar]
- Badri, A.E.; Sinha, J.K.; Albarbar, A. A typical filter design to improve the measured signals from mems accelerometer. Measurement 2010, 43, 1425–1430. [Google Scholar] [CrossRef]
- Medeiros, K.A.R.; Barbosa, C.R.H.; de Oliveira, E.C. Flow measurement by piezoelectric accelerometers: Application in the oil industry. Pet. Sci. Technol. 2015, 33, 1402–1409. [Google Scholar] [CrossRef]
- Vasconcelos, P.V.; Lino, F.J.; Magalhães, A.; Neto, R.J.L. Impact fracture study of epoxy-based composites with aluminium particles and milled fibres. J. Mater. Process. Technol. 2005, 170, 277–283. [Google Scholar] [CrossRef]
- Gururaja, T.R.; Schulze, W.A.; Cross, L.E.; Newnham, R.E.; Auld, B.A.; Wang, Y.J. Piezoelectric composite materials for ultrasonic transducer applications. Part I: Resonant modes of vibration of PZT rod-polymer composites. IEEE Trans. Sonics Ultrason 1985, 32, 481–498. [Google Scholar] [CrossRef]
- Lees, S.; Davidson, C.L. Ultrasonic measurement of some mineral filled plastics. IEEE Trans. Sonics Ultrason. 1977, 24, 222–225. [Google Scholar] [CrossRef]
- Alves, J.M.; Valleˆra, A.M. High temperature sound velocity measurement with piezoelectric transducers. Am. Inst. Phys. 1997, 69, 130–131. [Google Scholar] [CrossRef]
- Zhang, R.; Jiang, B.; Caoa, W. Influence of sample size on ultrasonic phase velocity measurements in piezoelectric ceramics. Appl. Phys. 2002, 91, 10194–10198. [Google Scholar] [CrossRef]
- Wen, Y.C.; Chou, L.C.; Lin, H.H.; Lin, K.H.; Kao, T.F.; Sun, C.K. Compositional dependence of longitudinal sound velocities of piezoelectric (111) InxGa(1−x) as measured by picosecond ultrasonics. J. Appl. Phys. 2006, 100, 103516. [Google Scholar] [CrossRef]
- Kambara, T.; Kageyama, K.; Kanai, Y.; Kojima, T.M.; Nanai, Y.; Yoneda, A.; Yamazaki, Y. Elastic wave from fast heavy ion irradiation on solids. Nucl. Instrum. Methods Phys. Res. Sect. Beam Interact. Mater. Atoms. 2002, 193, 371–375. [Google Scholar] [CrossRef]
- Golding, B.; Haemmerle, W.H.; Schneemeyer, L.F.; Waszczak, J.V. Gigahertz ultrasound in single crystal superconducting YBa2 Cu3O7. In Proceedings of the IEEE 1988 Ultrasonics Symposium, Chicago, IL, USA, 2–5 October 1988; Volume 1072, pp. 1079–1083. [Google Scholar]
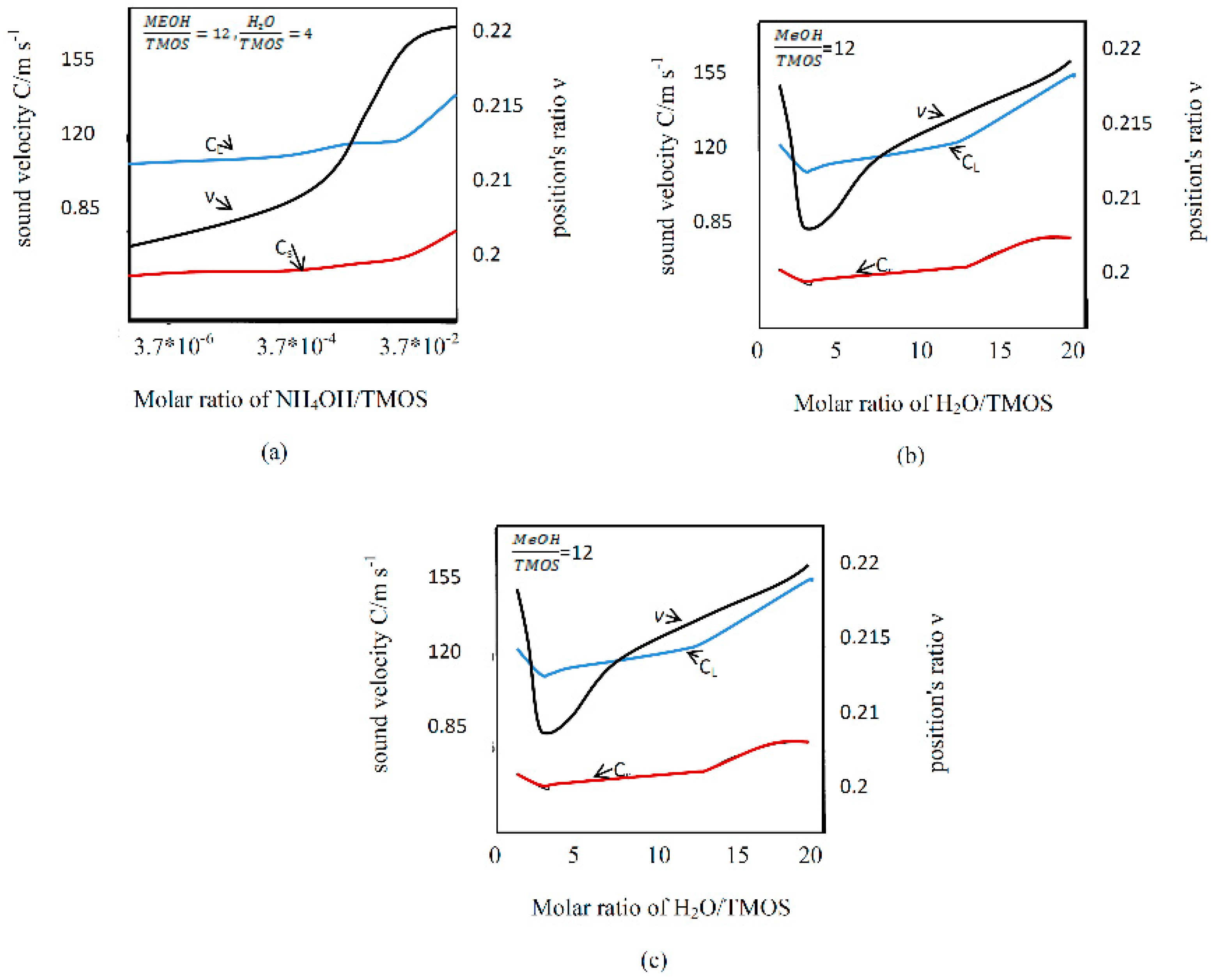
- Haranath, D.; Wagh, P.B.; Pajonk, G.M.; Rao, A.V. Influence of sol-gel processing parameters on the ultrasonic sound velocities in silica aerogels. Mater. Res. Bull. 1997, 32, 1079–1089. [Google Scholar] [CrossRef]
- Gomez, T.E.; Gonzalez, B.; Montero, F. Paper characterization by measurement of thickness and plate resonances using air-coupled ultrasound. In Proceedings of the 2002 IEEE Ultrasonics Symposium, Munich, Germany, 8–11 October 2002; Volume 861, pp. 865–868. [Google Scholar]
- Fariñas, M.D.; Álvarez-Arenas, T.E.G.; Cummins, G.; Desmulliez, M.P.Y.; Seetohul, V.; Cochran, S. Assessment of the ultrasonic properties of additive manufactured materials for passive components of piezoelectric transducers. In Proceedings of the 2016 IEEE International Ultrasonics Symposium (IUS), Tours, France, 18–21 September 2016. [Google Scholar]
- Garcia-Rodriguez, M.; Yañez, Y.; Garcia-Hernandez, M.J.; Salazar, J.; Turo, A. Experimental velocity measurements of the low-order anti-symmetric lambwave generated-detected in metallic plates using air-coupled concave array transducers. Nondestruct. Eval. 2011, 30, 50–58. [Google Scholar] [CrossRef]
- Kozhevnikov, V.F.; Payne, W.B.; Olson, J.K.; Allen, A.; Taylor, P.C. Sound velocity in liquid and glassy selenium. J. Non-Cryst. Solids 2007, 353, 3254–3259. [Google Scholar] [CrossRef] [Green Version]
- Greve, D.W.; Oppenheim, I.J.; Zhengc, P. Lamb waves and nearly-longitudinal waves in thick plates. Sens. Smart Struct. Technol. Civ. Mech. Aerosp. Syst. 2008, 6932, 1–10. [Google Scholar]
- Greve, D.W.; Zheng, P.; Oppenheim, I.J. 12D-1 excitation of longitudinal and lamb waves in plates by edge-mounted transducers. In Proceedings of the 2007 IEEE Ultrasonics Symposium Proceedings, New York, NY, USA, 28–31 October 2007; pp. 1120–1123. [Google Scholar]
- Zhang, L.; Rogl, G.; Grytsiv, A.; Puchegger, S.; Koppensteiner, J.; Spieckermann, F.; Kabelka, H.; Reinecker, M.; Rogl, P.; Schranz, W.; et al. Mechanical properties of filled antimonide skutterudites. Mater. Sci. Eng. B 2010, 170, 26–31. [Google Scholar] [CrossRef]
- Chen, S.; Wang, G.; Wen, J.; Wen, X. Wave propagation and attenuation in plates with periodic arrays of shunted piezo-patches. J. Sound Vib. 2013, 332, 1520–1532. [Google Scholar] [CrossRef]
- Rouzaud, A.; Barbier, E.; Ernoult, J.; Quesnel, E. A method for elastic modulus measurements of magnetron sputtered thin films dedicated to mechanical applications. Thin Solid Films 1995, 270, 270–274. [Google Scholar] [CrossRef]
- Khoury, M.; Tourtollet, G.E.; Schröder, A. Contactless measurement of the elastic young’s modulus of paper by an ultrasonic technique. Ultrasonics 1999, 37, 133–139. [Google Scholar] [CrossRef]
- Luukkala, M.; Heikkila, P.; Surakka, J. Plate wave resonance—A contactless test method. Ultrasonics 1971, 9, 201–208. [Google Scholar] [CrossRef]
- Osamura, K.; Nyilas, A.; Shin, H. Estimation of uncertainty with the modulus of elasticity measured by means of tensile test for bscco tapes. Cryogenics 2010, 50, 660–665. [Google Scholar] [CrossRef]
- Vendra, L.; Rabiei, A. Evaluation of modulus of elasticity of composite metal foams by experimental and numerical techniques. Mater. Sci. Eng. A 2010, 527, 1784–1790. [Google Scholar] [CrossRef]
- Diaz, C.A.; Afrifah, K.A.; Jin, S.; Matuana, L.M. Estimation of modulus of elasticity of plastics and wood plastic composites using a taber stiffness tester. Compos. Sci. Technol. 2011, 71, 67–70. [Google Scholar] [CrossRef]
- Genga, R.M.; Cornish, L.A.; Akdogan, G. Effect of Mo2C additions on the properties of SPS manufactured WC–TiC–Ni cemented carbides. Int. J. Refract. Met. Hard Mater. 2013, 41, 12–21. [Google Scholar] [CrossRef]
- Stasiak, M.; Molenda, M.; Horabik, J. Determination of modulus of elasticity of cereals and rapeseeds using acoustic method. J. Food Eng. 2007, 82, 51–57. [Google Scholar] [CrossRef]
- Dzbeński, W.; Wiktorski, T. Ultrasonic evaluation of mechanical properties of wood in standing trees. In Proceedings of the COST E 53 Conference—Quality Control for Wood and Wood Products, Warsaw, Poland, 15–17 October 2007. [Google Scholar]
- Río, L.M.D.; López, F.; Calleja, B.; Tejado, J.J.; Mota, M.I.; González, I.; San Emeterio, J.L.; Ramos, A. Resonance-based acoustic technique applied to the determination of young’s modulus in granites. In Proceedings of the 19th International Congress on Acoustics, Madrid, Spain, 2–7 September 2007. [Google Scholar]
- Elastic Modulus Measurment. Olympus: Tokyo, Japen. Available online: http://www.olympus-ims.com/en/applications/elastic-modulus-measurement/ (accessed on 14 September 2018).
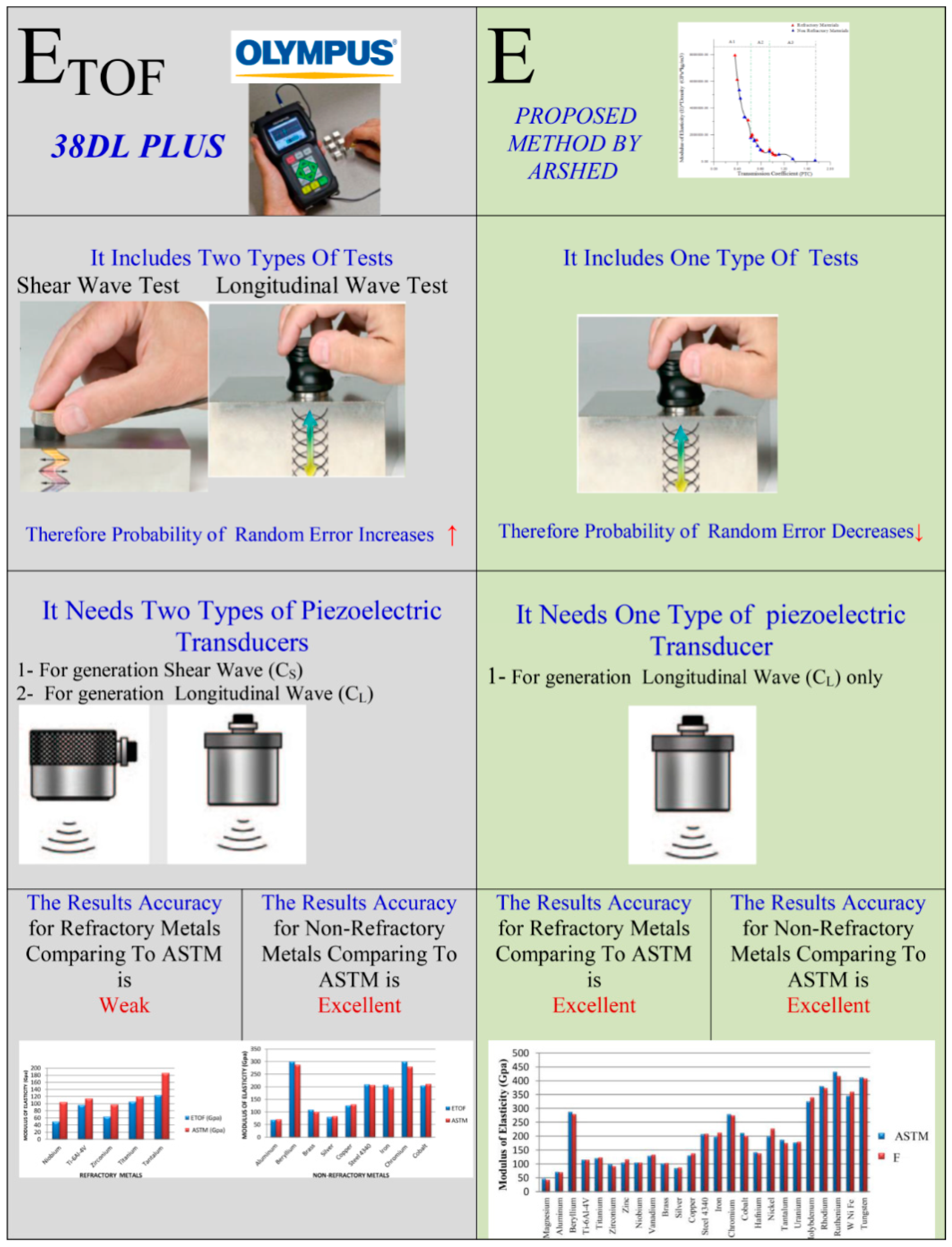
- Mohammed, A.A.; Haris, S.M.; Nuawi, M.Z. Using the pressure transmission coefficient of a transmitted wave to evaluate some of the mechanical properties of refractory metals. Ultrasonics 2015, 55, 133–140. [Google Scholar] [CrossRef] [PubMed]
- Mohammed, A.A. Design of a Piezoelectric Ceramic Transducer-Based Mechanical Properties Test for Refractory Metals. Universiti Kebangsaan Malaysia (UKM); Universiti Kebangsaan Malaysia (UKM): Bangi, Malaysia, 2015. [Google Scholar]
- Mohammed, A.A.; Haris, S.M.; Nuawi, M.Z. Utilizing hilbert–huang transform in detection some of mechanical properties of the refractory metals. Mech. Syst. Signal Process. 2016, 68, 449–461. [Google Scholar] [CrossRef]
- Boumiz, A.; Vernet, C.; Tenoudji, F.C. Mechanical properties of cement pastes and mortars at early ages: Evolution with time and degree of hydration. Adv. Cem. Based Mater. 1996, 3, 94–106. [Google Scholar] [CrossRef]
- Khandelwal, M.; Singh, T.N. Correlating static properties of coal measures rocks with P-wave velocity. Int. J. Coal Geol. 2009, 79, 55–60. [Google Scholar] [CrossRef]
- Sharpe, W.N., Jr.; Bin, Y.; Vaidyanathan, R.; Edwards, R.L. Measurements of young’s modulus, poisson’s ratio, and tensile strength of polysilicon. In Proceedings of the Tenth IEEE International Workshop on Microelectromechanical Systems, Nagoya, Japan, 26–30 January 1997; pp. 424–429. [Google Scholar]
- Jackson, K.M. Fracture strength, elastic modulus and poisson’s ratio of polycrystalline 3C thin-film silicon carbide found by microsample tensile testing. Sens. Actuators A Phys. 2005, 125, 34–40. [Google Scholar] [CrossRef]
- Bamber, M.J.; Cooke, K.E.; Mann, A.B.; Derby, B. Accurate determination of young’s modulus and poisson’s ratio of thin films by a combination of acoustic microscopy and nanoindentation. Thin Solid Films 2001, 398, 299–305. [Google Scholar] [CrossRef]
- Ganguly, P.; Poole, W.J. In situ measurement of reinforcement stress in an aluminum-alumina metal matrix composite under compressive loading. Mater. Sci. Eng. A 2003, 352, 46–54. [Google Scholar] [CrossRef]
- Lee, S.J.; Han, S.W.; Hyun, S.M.; Lee, H.J.; Kim, J.H.; Kim, Y.I. Measurement of young’s modulus and poisson’s ratio for thin au films using a visual image tracing system. Curr. Appl. Phys. 2009, 9, S75–S78. [Google Scholar] [CrossRef]
- Zhu, J.; Kee, S.-H. Monitoring early age microstructure development of cement paste using bender elements. Nondestruct. Charact. Compos. Mater. 2010, 7649, 76491R. [Google Scholar]
- Dano, C.; Hicher, P.-Y. Evolution of elastic shear modulus in granular materials along isotropic and deviatoric stress paths. In ASCE Engineering Mechanics; Columbia University: New York, NY, USA, 2002. [Google Scholar]
- Yoo, J.-K.; Park, D.; Baxter, C.D.P. Estimation of drained shear strength of granular soil from shear wave velocity and confining stress. J. Geotech. Geoenviron. Eng. 2018, 144, 04018027. [Google Scholar] [CrossRef]
- Wang, Y.C.; Lakesa, R.S. Resonant ultrasound spectroscopy in shear mode. Rev. Sci. Instrum. 2003, 74, 1371–1373. [Google Scholar] [CrossRef] [Green Version]
- Tanaka, K.; Ichitsubo, T.; Matsubara, E. Elasticity and anelasticity of metallic glass near the glass transition temperature. Mater. Sci. Eng. A 2006, 442, 278–282. [Google Scholar] [CrossRef]
- Builes, M.; Maqbool, S.; Sato, T.; Koseki, J. Dynamic and static measurements of small strain moduli of toyoura sand. In Proceedings of the Sixth International Summer Symposium, Saitama, Japan, 31 July 2004; pp. 229–232. [Google Scholar]
- Builes, M.; Garcia, E.; Riveros, C.A. Dynamic and static measurements of small strain moduli of toyoura sand. GIGA Group Fac. Eng. Univ. Antioq. 2008, 43, 86–101. [Google Scholar]
- Mulmi, S.; Sato, T.; Kuwano, R. Performance of plate type piezo-ceramictransducers for elastic wave measurements in laboratory soil specimens. Seisan Kenkyu 2008, 60, 565–569. [Google Scholar]
- Stanzl-Tschegg, S. Very high cycle fatigue measuring techniques. Int. J. Fatigue 2013, 60, 2–17. [Google Scholar] [CrossRef]
- Marines-Garcia, I.; Doucet, J.-P.; Bathias, C. Development of a new device to perform torsional ultrasonic fatigue testing. Int. J. Fatigue 2007, 29, 2094–2101. [Google Scholar] [CrossRef]
- Lashin, I.; Hussien, M.; Karray, M.; Chekired, M. Assessment of conventional interpretation methods of RC results based on 3D numerical simulations. Int. J. Geomech. 2018, 18, 04018160. [Google Scholar] [CrossRef]
- Clauer, A.H.; Fairand, B.P.; Wilcox, B.A. Laser shock hardening of weld zones in aluminum alloys. Met. Mater. Trans. A 1977, 8, 1871–1876. [Google Scholar] [CrossRef]
- Sikarwar, S.; Singh, S.; Yadav, B.C. Review on pressure sensors for structural health monitoring. Photonic Sens. 2017, 7, 294–304. [Google Scholar] [CrossRef] [Green Version]
- Labuz, J.F.; Biolzi, L. Characteristic strength of quasi-brittle materials. Int. J. Solids Struct. 1998, 35, 4191–4203. [Google Scholar] [CrossRef]
- Brown, E.N.; Davis, A.K.; Jonnalagadda, K.D.; Sottos, N.R. Effect of surface treatment on the hydrolytic stability of E-glass fiber bundle tensile strength. Compos. Sci. Technol. 2005, 65, 129–136. [Google Scholar] [CrossRef]
- Dent, A.C.E.; Bowen, C.R.; Stevens, R.; Cain, M.G.; Stewart, M. Tensile strength of active fibre composites—Prediction and measurement. Ferroelectr. Taylor Fr. Group LLC. 2008, 368, 209–215. [Google Scholar] [CrossRef]
- Chaki, S.; Bourse, G. Non-destructive evaluation of the stress levels in prestressed steel strands using acoustoelastic effect. In NDTCE’09, Non-Destructive Testing in Civil Engineering; Spring: Nantes, France, 2009. [Google Scholar]
- Waller, J.M.; Saulsberry, R.L.; Andrade, E. Use of acoustic emission to monitor progressive damage accumulation in kevlar® 49 composites. Qunt. Nondestruct. Eval. (QNDE) Kingst. 2009. [Google Scholar] [CrossRef]
- Silva, F.D.A.; Zhu, D.; Mobasher, B.; Soranakom, C.; Filhoc, R.D.T. High speed tensile behavior of sisal fiber cement composites. Mater. Sci. Eng. A 2010, 527, 544–552. [Google Scholar] [CrossRef] [Green Version]
- Kim, J.-H.; Kim, D.; Han, H.N.; Barlat, F.; Lee, M.-G. Strain rate dependent tensile behavior of advanced high strength steels: Experiment and constitutive modeling. Mater. Sci. Eng. A 2013, 559, 222–231. [Google Scholar] [CrossRef]
- Spronk, S.W.F.; Verboven, E.; Gilabert, F.A.; Sevenois, R.D.B.; Garoz, D.; Kersemans, M.; Paepegem, W.V. Dynamic tensile testing of brittle composites using a hydraulic pulse machine: Stress-strain synchronization and strain rate limits. Proceedings 2018, 2, 405. [Google Scholar] [CrossRef]


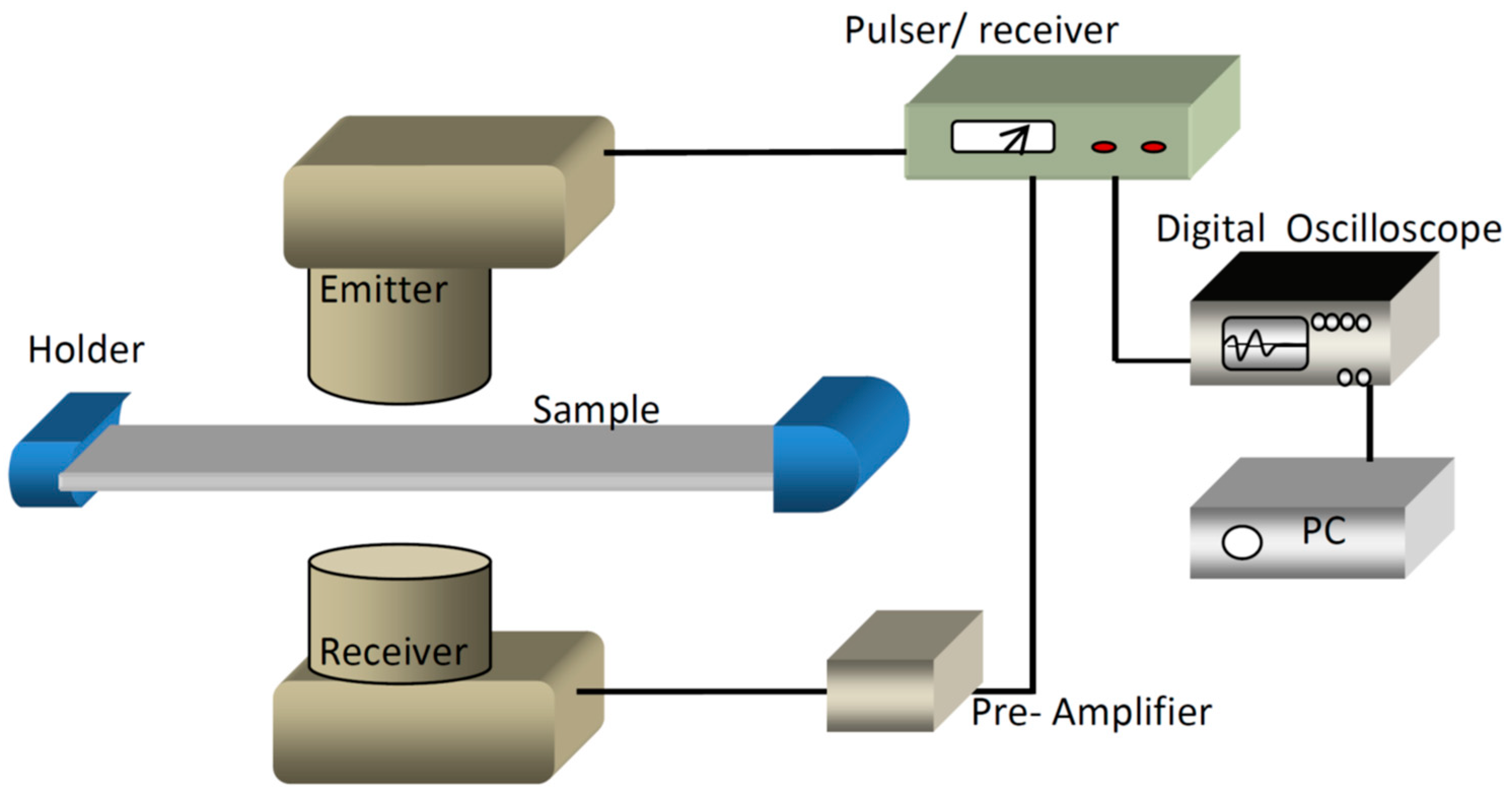
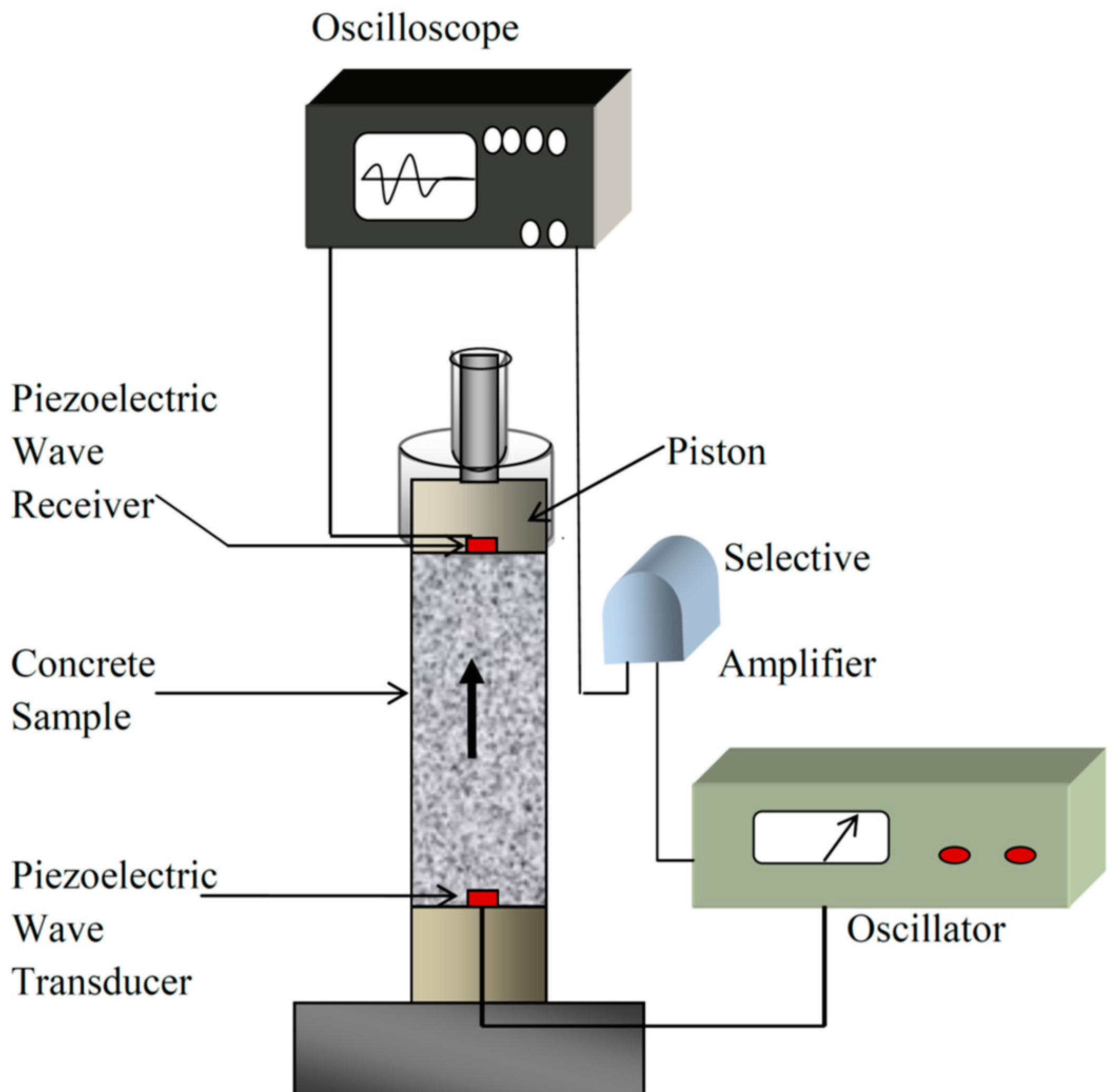
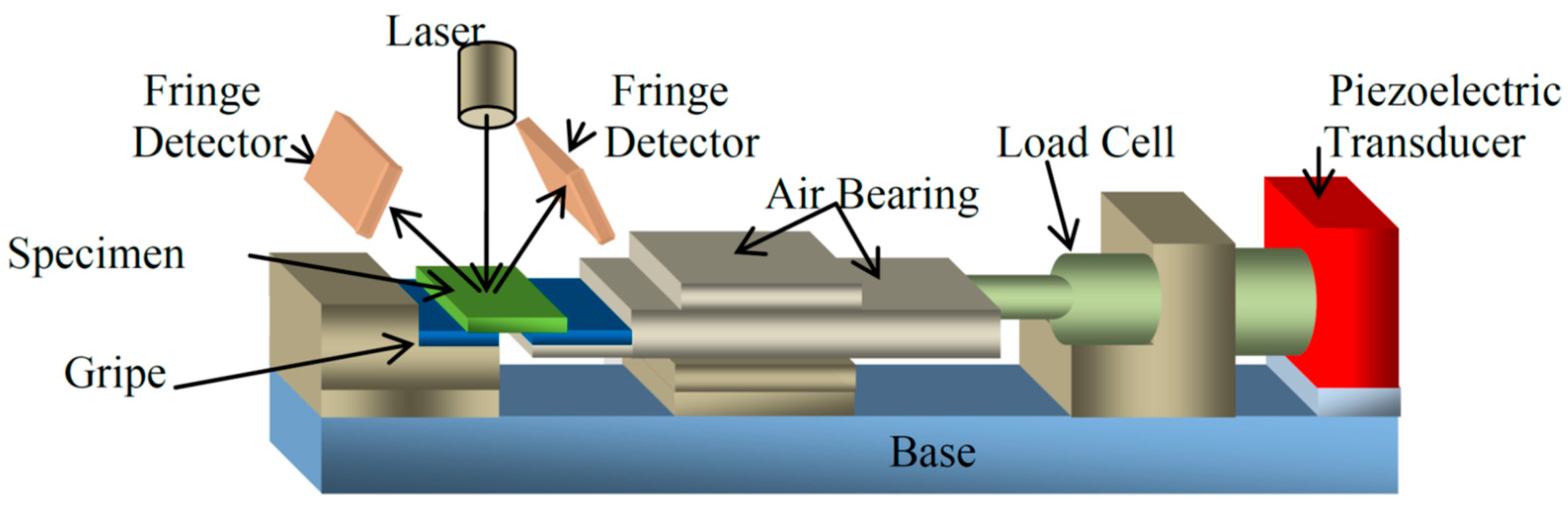



| Description of PE | Role of PE | Description of the Advantage of PE |
|---|---|---|
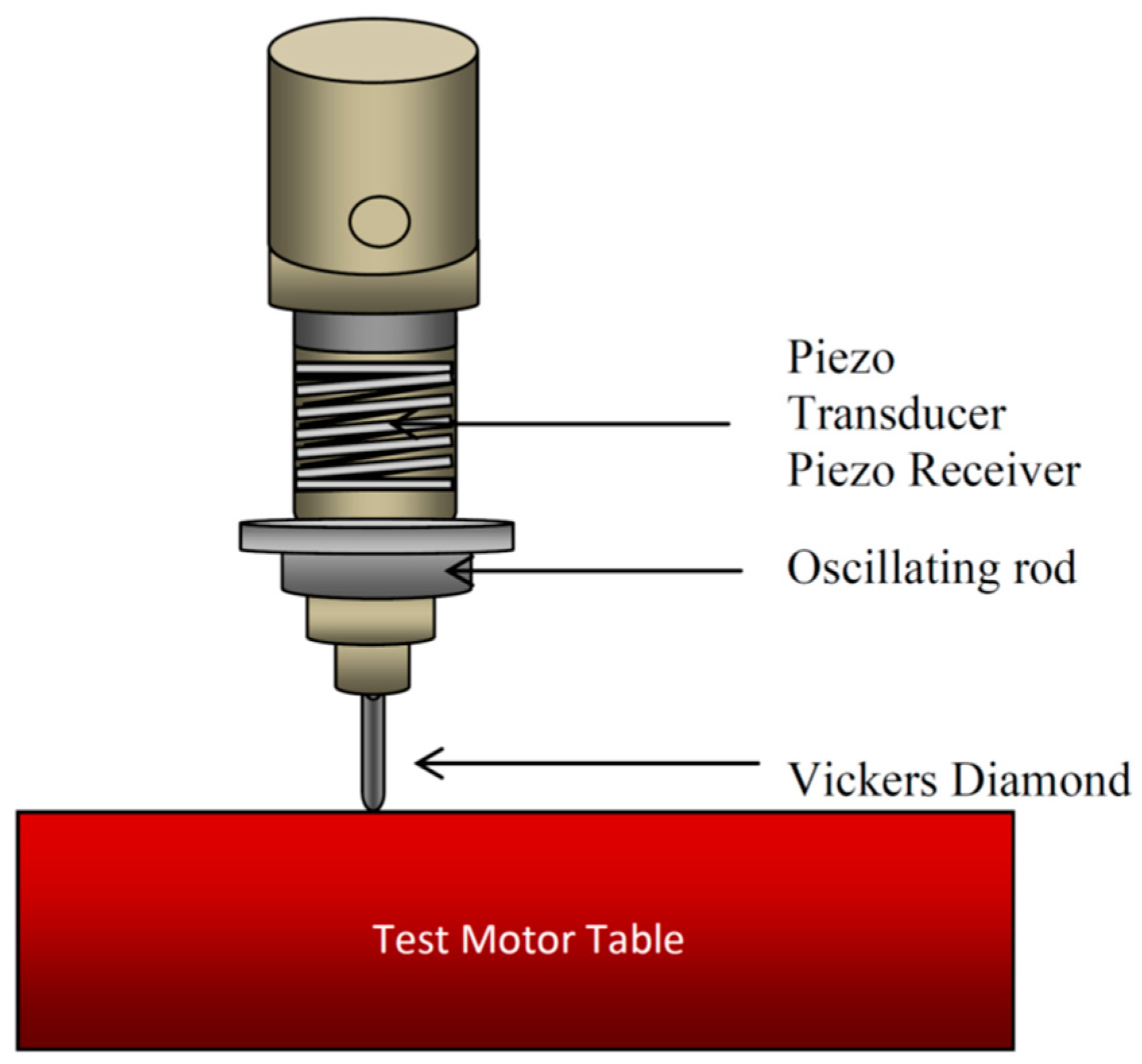
| (100 kHz to 2000 kHz) | Piezo-scanner. | An indenter tip was employed to obtain a convenient nanometer-resolution imaging of test surface for measuring the slim membrane toughness of the tetrahedral amorphous carbon of diamond [17]. More details on the piezo-scanner is available in the literature [18,19]. |
| 5 MHz | Load cell in quasi-static dynamic fracture toughness tests. | PE can eliminate any inertia effects associated with the higher velocity (5000 mm/s) test rates. Results show the magnitudes of K1C within the range () at an applied load rate of 1 m/s [20]. More details on using PE as a load cell has been previously reported [21,22,23]. |
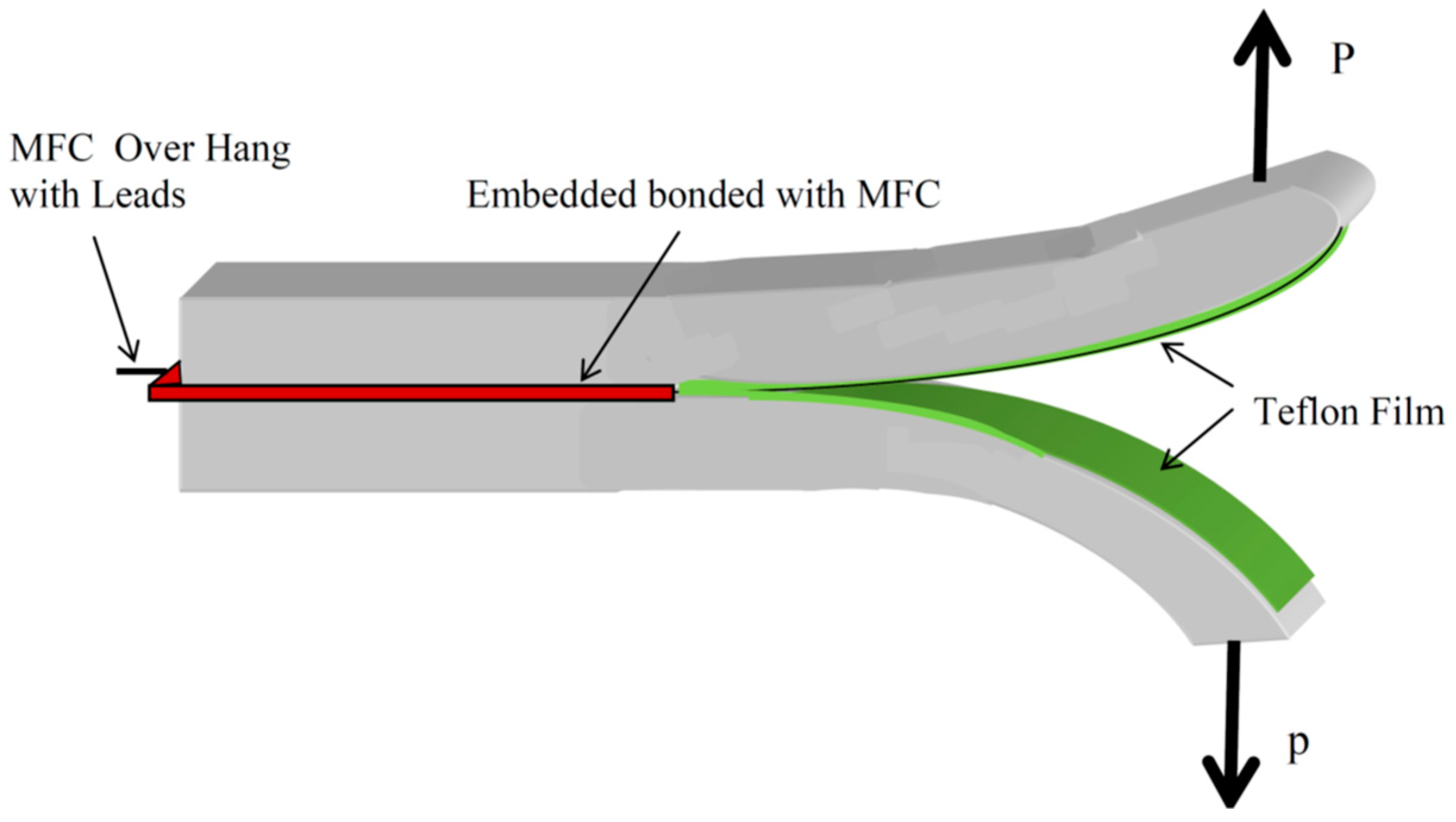
| Lead zirconate titanate Pb[ZrxTi1-x]O3 (PZT) 0.79 in × 3.7 in (width× length) and thickness 0.0115 in to 0.0063 in | Embedded sensor for vibration control and health monitoring of aircraft structure. | PE was used as a sensor for load frequencies from 1 Hz to 10 Hz in epoxy and to study the effect of embedded materials on fracture toughness. Piezoelectric microfiber composite (MFC) (embedded piezoelectric sensors) was covered with a thin polymer film on its two sides. Then, the sandwich was inserted as an embedded material in glass/epoxy composite material (12-in2 unidirectional panels), as shown in Figure 2 to function as a sensor [24,25,26]. More details on embedded sensors have been reported [27,28]. |
| Emitter and receiver. | PE was used as a detector to determine the characteristics of several molybdenum alloys (10 mm × 10 mm × 55 mm) at various resonance frequencies [29]. | |
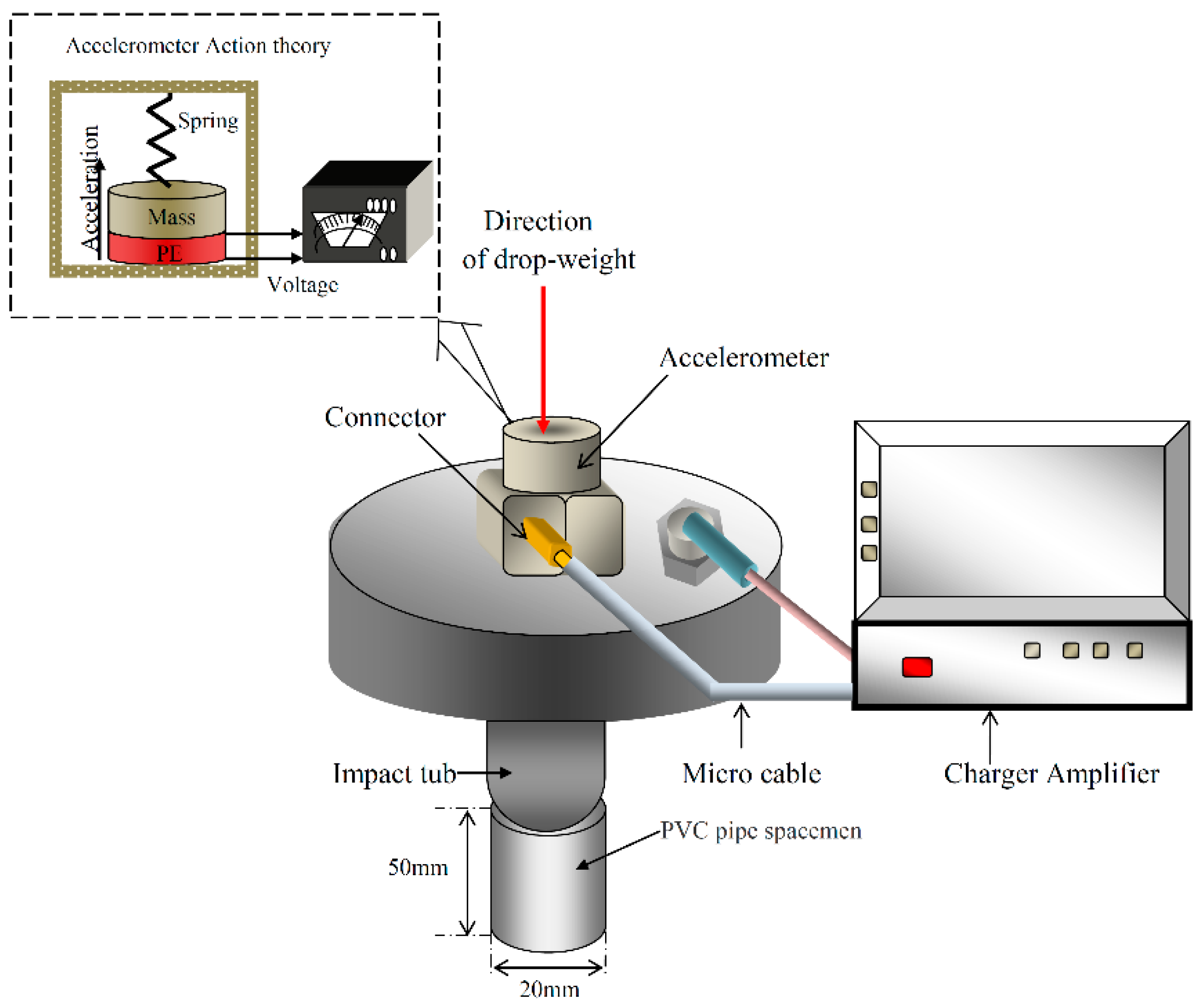
| Load cell for tool cutting. | PE was used to measure cutting force in the x-direction and transverse force in the y-direction to calculate the fracture toughness of polymers with cutting widths of 3 mm to 6 mm, depths of 0.025 mm to 0.25 mm, and velocity of 10 mm/s [30]. | |
| Monitor for the constant loading rate and equilibrium of the dynamic load on a pre-cracked specimen. | The new technology reported in references [31] proved the practicability of using the loading rate as a function of the dynamic fracture. Another article reported a similar finding [32]. | |
| Thick mode 10 MHz and 4 MHz shear mode | Sender and receiver for longitudinal and shear waves. | A thickness mode vibration PE was used for creating longitudinal waves and shear mode vibration for creating shear waves, these two types of PE were used in a crack-opening displacement method to determine the toughness of base glasses for dental restoration glass-ceramics [33]. |
| Material Properties | Emitter and Receiver Frequency Range | Rough Dimension Sample Size in mm, L = Length, W = Width, D = Depth |
|---|---|---|
| Creep | 2.25 MHz to 15 MHz | 150, 22–30, - |
| Fracture toughness | 4 MHz to 10 MHz | 10, 10, - |
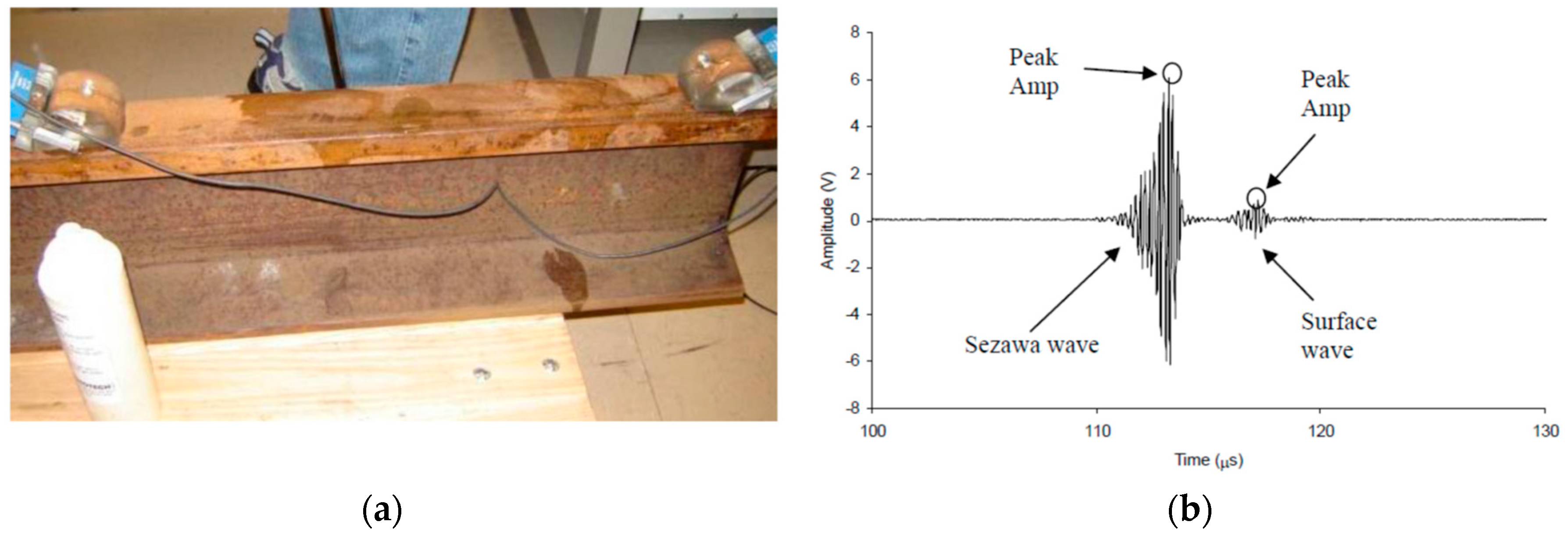
| Hardness | Sezawa wave (1 MHz to 5 MHz) | -, -, 1.9–5.65 |
| Longitudinal velocity | Guide wave (1 MHz to 3.5 MHz) | 152, 10~20, 30 |
| 405 kHz to 15 MHz | 19–100, 10, 305 | |
| Modulus of elasticity | Air Coupled 750 KHz to 1.7 MHz | Paper-, -, 1–2; metal -, -, 0.1~0.5 |
| 1 kHz to 1 MHz | 60–150, 13~70, 5 | |
| Poisson’s ratio | Air coupled 250 kHz to 1 MHz | paper 60–700, -, - |
| 500 kHz | ||
| Shear modulus | 60 kHz to 10 MHz | Diameter = 50, 100 |
| Tensile strength | 1 kHz to 2 MHz | Rock 200–800 span = 75–300; Metal 25, 1.4, 1.1 |
| Frequency (fr) Range in MHz | Type of Application | Rough Description of the Samples |
|---|---|---|
| Less than 0.5 | Piezo-spectroscope, shaker load cell, filter for interface noise, emitter only, pickup sensor only, shock wave generator, active and passive velocity transducer, polyvinylidene fluoride (PVDF), Piezo-scanner, load cell, monitor to the constant load, dynamometer, strain gauge, accelerometers | Most dimensions for length, width and depth are less than 100 mm. Weight loads ranged between 4 N to 200 Grain size (0.3 µm to 1.5 µm) |
| 0.5 < fr < 2 | PVDF, Piezo-electroscope, and pickup sensor | |
| 2 < fr < 5 | Shaker load cell, pickup sensor only, monitor for constant load and PVDF. | |
| 5 < fr < 15 | High-speed actuator, PVDF. |
| Material Type | Range of Frequency of PE | Dimension of Samples (mm) |
|---|---|---|
| Plexiglas, glass, metallic glass | 300 kHz to 8 MHz | |
| Polycrystaline metals Aluminium, copper, Al2O3 | 10 Hz to 2000 kHz (bulk wave), 1 MHz to 3.5 MHz (surface wave) | 200 × 25 × 2, 152 × 50 × 4 |
| Ice | 1 Hz to 1000 Hz | 394 × 76 |
| Polymer | 1 Hz to 20 Hz | 8 to 20 (circle diameter) |
| IN738IC nickel base | 2.25~15 MHz | 150 × (22.5–30) |
| Stainless steel 316L | 100 Hz to 15 MHz | |
| Tetrahedral amorphous carbon diamond | 100 kHz to 2000 kHz | |
| Rail specimen | 1 MHz to 5 MHz | 1.9–5.65 |
| Ceramic | 1 MHz to 10 MHz | |
| Silica aerogels | 405 kHz | |
| Paper of writing | 0.9 MHz to 1.7 MHz | 80–300 g/m3 |
| Fe4Sb12, Co4Sb12, didymium akaline | 10 MHz | 10 (diameter) and 10 (height) |
| Seismogenic rock, concert, bulk of cereal grains | 75 kHz to 1 MHz | 60 × 13 × 5 |
| Epoxy-impregnated Kevlar | 1 kHz to 1.5 kHz | 25 × 1.4 × 1.1 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mohammed, A.A.; Haris, S.M.; Nuawi, M.Z. Role of Piezoelectric Elements in Finding the Mechanical Properties of Solid Industrial Materials. Appl. Sci. 2018, 8, 1737. https://doi.org/10.3390/app8101737
Mohammed AA, Haris SM, Nuawi MZ. Role of Piezoelectric Elements in Finding the Mechanical Properties of Solid Industrial Materials. Applied Sciences. 2018; 8(10):1737. https://doi.org/10.3390/app8101737
Chicago/Turabian StyleMohammed, Arshed Abdulhamed, Sallehuddin Mohamed Haris, and Mohd Zaki Nuawi. 2018. "Role of Piezoelectric Elements in Finding the Mechanical Properties of Solid Industrial Materials" Applied Sciences 8, no. 10: 1737. https://doi.org/10.3390/app8101737
APA StyleMohammed, A. A., Haris, S. M., & Nuawi, M. Z. (2018). Role of Piezoelectric Elements in Finding the Mechanical Properties of Solid Industrial Materials. Applied Sciences, 8(10), 1737. https://doi.org/10.3390/app8101737





