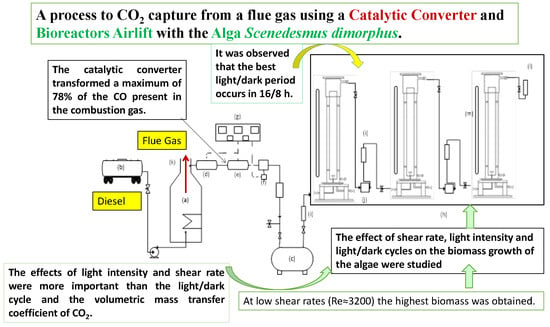
CO2 Capture of the Gas Emission, Using a Catalytic Converter and Airlift Bioreactors with the Microalga Scenedesmus dimorphus
Abstract
1. Introduction
2. Materials and Methods
2.1. Materials
2.1.1. Steam Boiler
2.1.2. Catalytic Converter
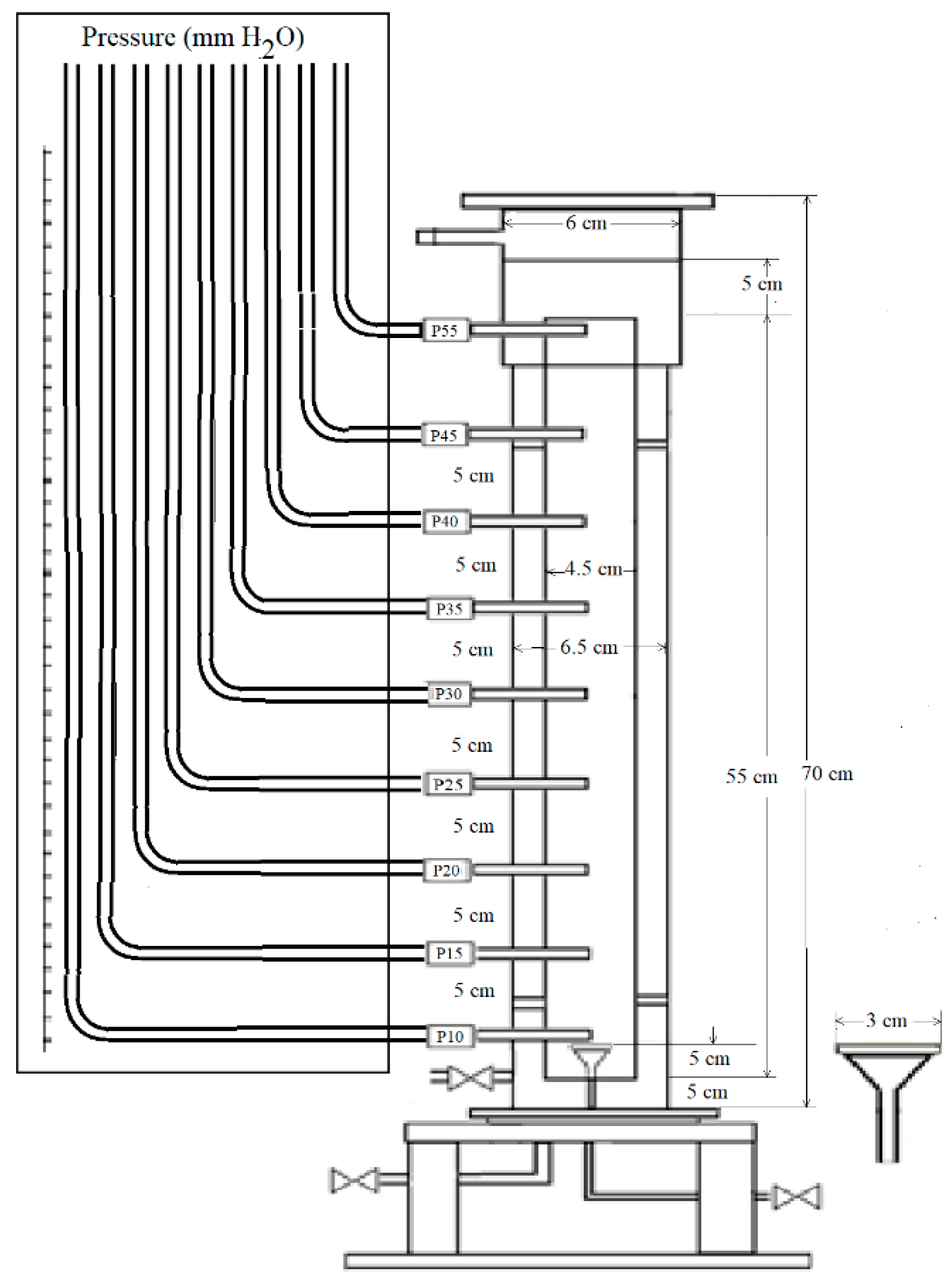
2.1.3. Airlift Bioreactors
2.1.4. Microorganism and Inoculum
2.1.5. Culture Media
2.2. Methods
2.2.1. Composition of the Gas Emission
2.2.2. Procedure to Convert CO, NO, and Hydrocarbons
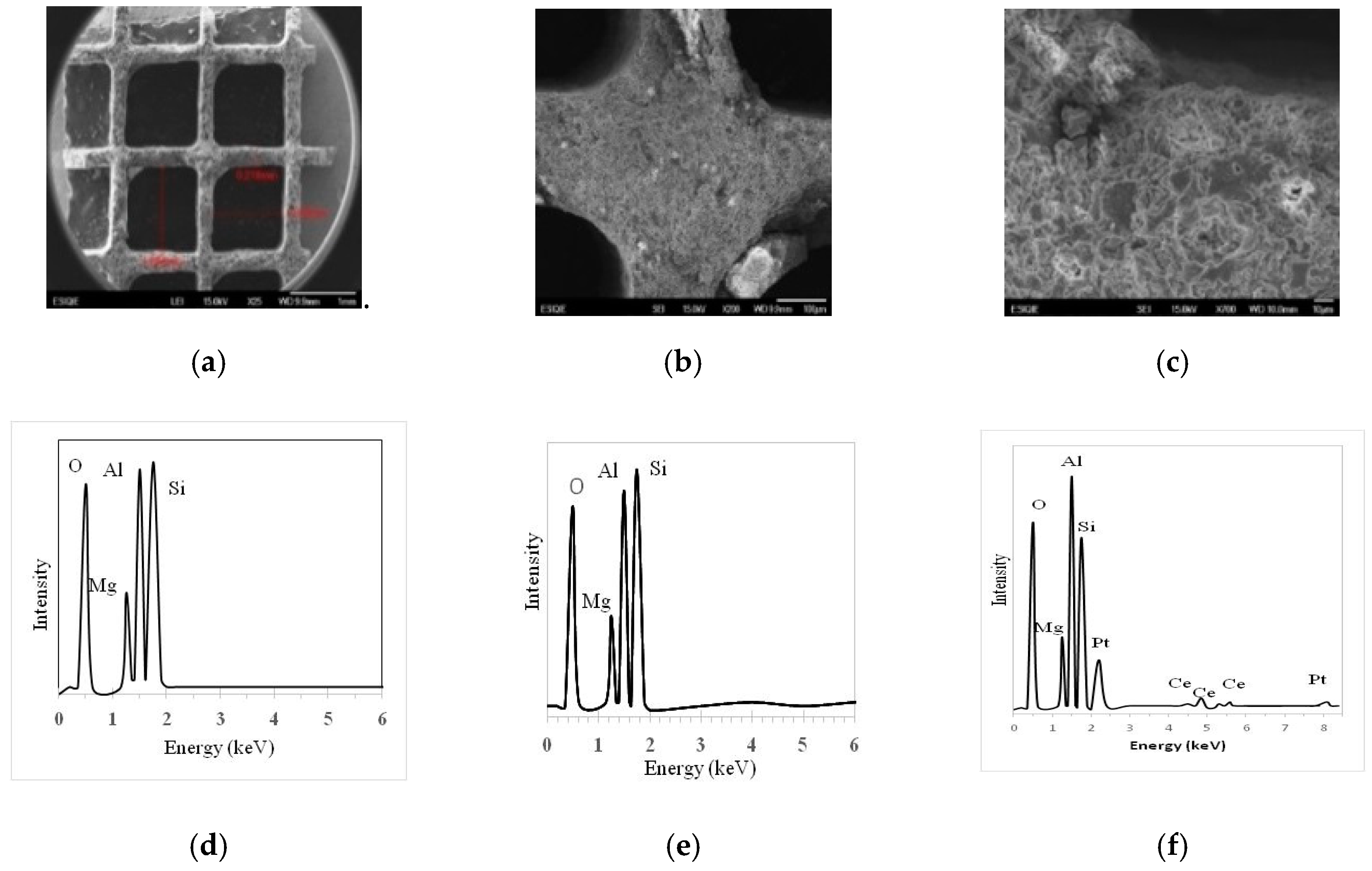
2.2.3. Characterization of the Catalytic Converter by SEM and EDS
2.2.4. Cultures
2.2.5. Calculation of Gas Holdup (φi)
2.2.6. Calculation of Superficial Liquid Velocity Ulr and Uld
2.2.7. Calculation of Shear Rate (γ)
2.2.8. Mass Balance of CO2 in the Culture
2.3. Determination of Biomass by Optical Density
2.4. Determination of Maximum Specific Growth Rate
2.5. Determination of CO2 Removal Efficiency
2.6. pH, Alkalinity, and Concentration of Dissolved Inorganic Carbon (CT)
2.7. Determination of the Overall Volumetric Mass Transfer Coefficient for CO2 (KLa)
3. Results and Discussion
3.1. Performance of the Catalytic Converter
3.2. Characterization of the Catalytic Converter
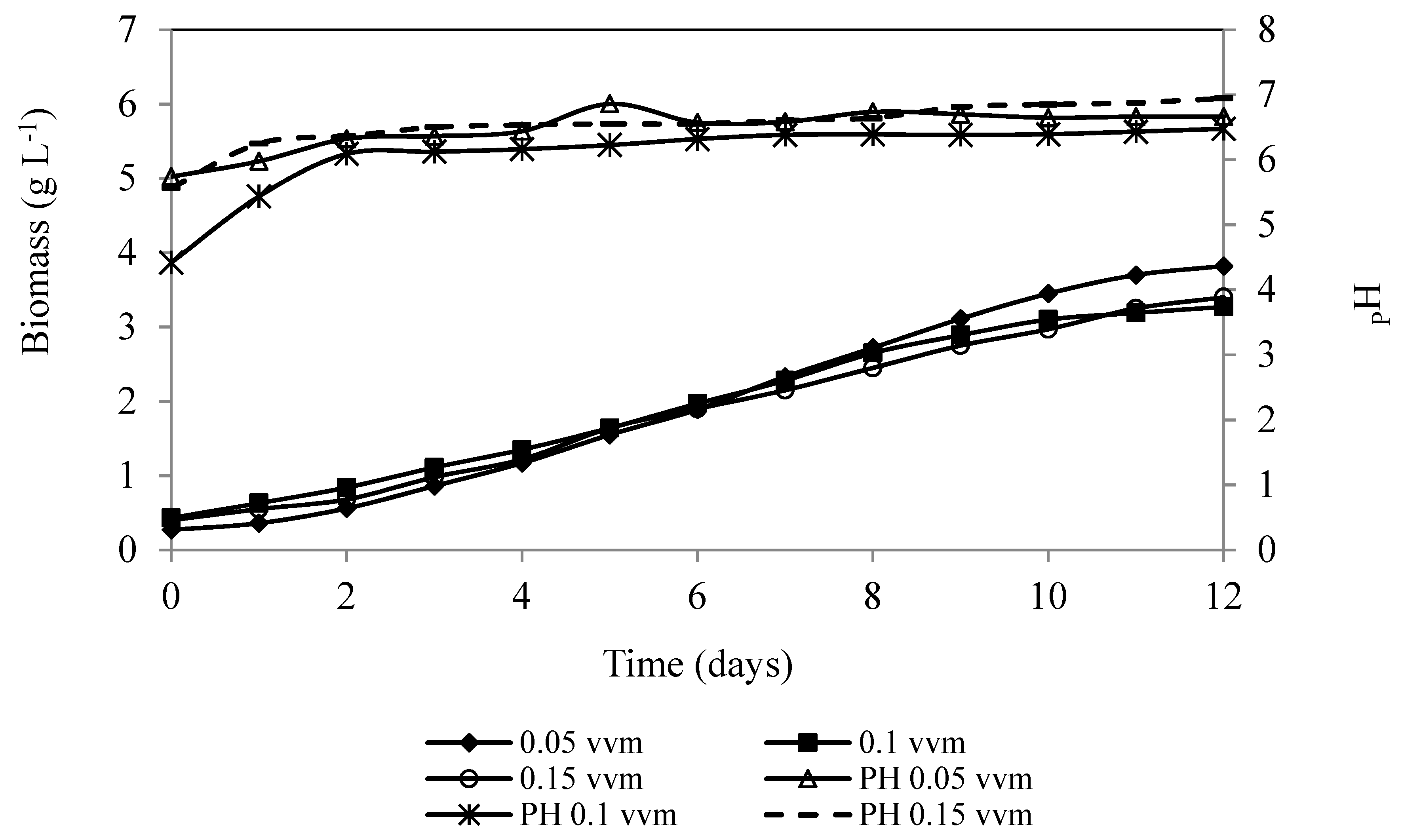
3.3. Effect of the Hydrodynamics of the Reactor Airlift on the Microalga Growth
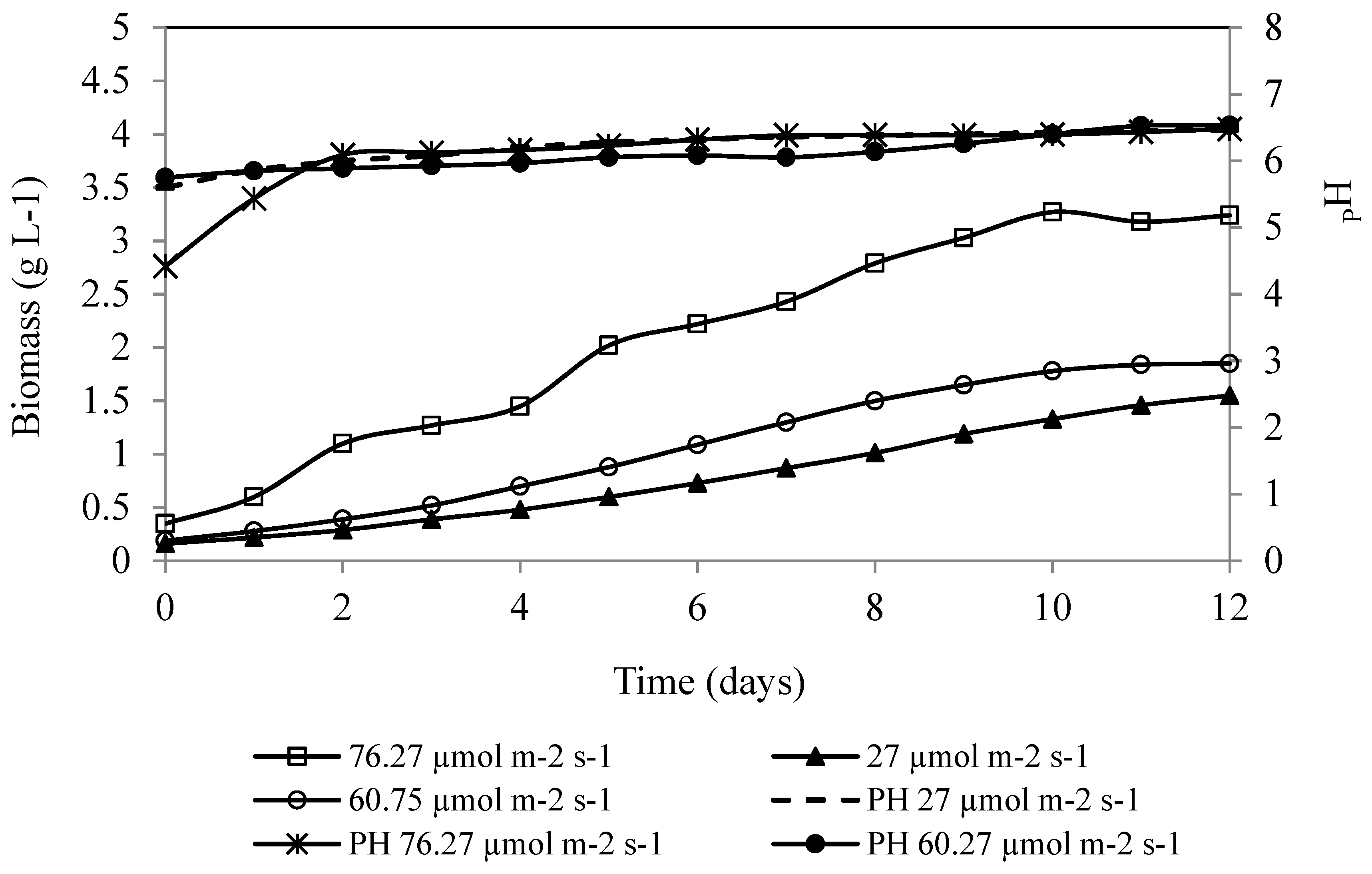
3.4. Effect of the Light Intensity on the Growth of Scenedesmus dimorphus
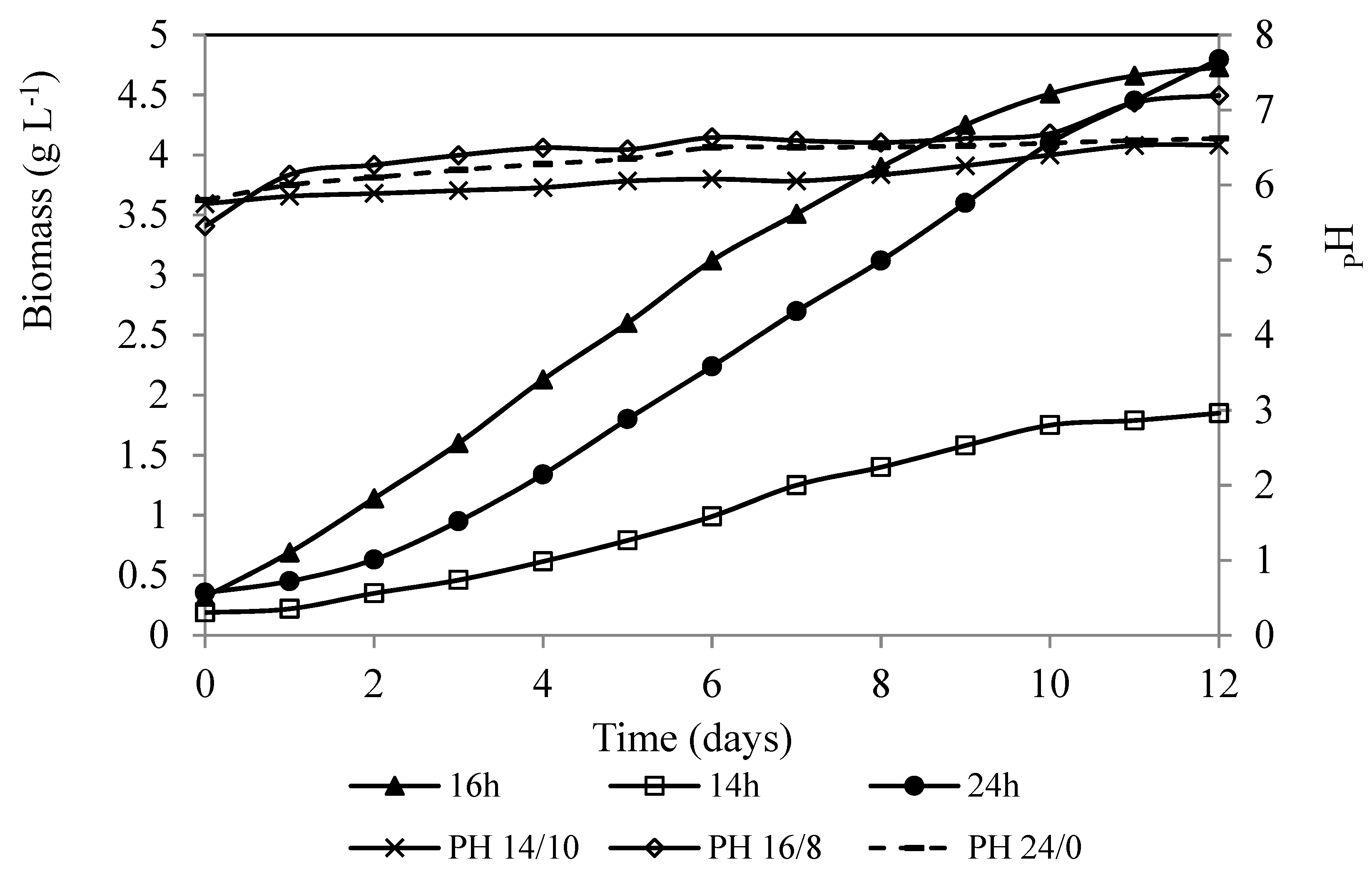
3.5. Effect of the Light/Darkness Cycle on the Growth of S. dimorphus
3.6. Determination of CO2 Removal Efficiency
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- López, M.T.; Zuk, M.; Garibay, V.; Tzintzun, G.; Iniestra, R.; Fernández, A. Health impacts from plant emissions in Mexico. Atmos. Environ. 2005, 39, 1199–1209. [Google Scholar] [CrossRef]
- Ministry of the Environment and Natural Resources. National Inventory of Pollutant Emissions Criteria. 2016. Available online: https://www.gob.mx/semarnat/ documentos/documentos-del-inventario-nacional-de-emisiones (accessed on 10 June 2019).
- National Institute of Ecology and Climate Change. National Inventory of Gas Emissions and Greenhouse Compounds. 2018. Available online: https://www.gob.mx/inecc/acciones-yprogramas/inventario-nacional-de-emisiones-de-gases-y-compuestos-de-efecto-invernadero (accessed on 10 June 2019).
- Wang, B.; Li, Y.Q.; Wu, N.; Lan, C.Q. CO2 bio-mitigation using microalgae. Appl. Microb. Biotech. 2008, 79, 707–718. [Google Scholar] [CrossRef]
- Chiang, C.L.; Lee, C.M.; Chen, P.C. Utilization of the cyoanobacteria Anabaena sp. CH1 in biological carbon dioxide mitigation processes. Bioresour. Technol. 2011, 102, 5400–5405. [Google Scholar] [CrossRef]
- Kaithwas, A.; Prasad, M.; Kulshreshtha, A.; Verma, S. Industrial waste derived solid adsorbents for CO2 capture: A mini review. Chem. Eng. Res. 2012, 90, 1632–1641. [Google Scholar] [CrossRef]
- Perry, R.H.; Chilton, C.H. Perry’s Chemical Engineer’s Handbook, 5th ed.; McGraw-Hill Kogakusha, LTD: Tokyo, Japan, 1973; pp. 3–18. [Google Scholar]
- Tilman, W.; Beutel, J.C.; Dettling, D.O.; Hollobaugh, W.; Torsten, W.; Mueller-Stach, P.P. Diesel Oxidation Catalysts with CO/HC Light Off and HC Storage Function. U.S. Patent No. 20090320449A1, 31 December 2009. [Google Scholar]
- Heo, I.; Wiebenga, M.H.; Gaudet, J.R.; Nam, I.S.; Li, W.; Kim, C.H. Ultra low temperature CO and HC oxidation over Cu-based mixed oxides for future automotive applications. Appl. Catal. B Environm. 2014, 160–161, 365–373. [Google Scholar] [CrossRef]
- Arroyo Gómez, C.A. Biofixation of CO2, NO and SO2 Present in the Gases of Thermoelectric Plants Using a Microalgae. Master’s Thesis, Metropolitan Autonomous University, Azcapotzalco, Mexico, 2015. [Google Scholar]
- Kasiri, S.; Ulrich, A.; Prasad, V. Optimization of CO2 fixation by Chlorella kassleri cultivated in a closed raceway pohotobioreactor. Bioresour. Thechnol. 2015, 194, 144–155. [Google Scholar] [CrossRef]
- Razzak, S.A.; Hossain, M.M.; Lucky, R.A.; Bassi, A.S.; De Lasa, H. Integrated CO2 capture, wastewater treatment and biofuel production by microalgae culturing—A review. Renew. Sustain. Energy Rev. 2013, 27, 622–653. [Google Scholar] [CrossRef]
- Chisti, Y. Airlift Bioreactors; Elsevier Applied Science: New York, NY, USA, 1989. [Google Scholar]
- Chen, Z.; Jiang, Z.; Zhang, J.; Zhang, J. Numerical and experimental study on the CO2 gas-liquid mass transfer in flat-plate airlift photobioreactor with different baffles. Biochem. Eng. J. 2016, 106, 129–138. [Google Scholar] [CrossRef]
- Chisti, Y. Pneumatically agitated bioreactors in industrial and environmental bioprocessing: Hydrodynamics, hydraulics, and transport phenomena. Appl. Mech. Rev. 1998, 51, 33–112. [Google Scholar] [CrossRef]
- Bitog, J.P.P.; Lee, I.B.; Oh, H.M.; Hong, S.W.; Seo, I.H.; Kwon, K.S. Optimized hydrodynamic parameters for the design of photobioreactors using computational fluid dynamics and experimental validation. Biosyst. Eng. 2014, 122, 42–61. [Google Scholar] [CrossRef]
- Nayac, B.K.; Roy, S.; Das, D. Biohydrogen production from algal biomass (Anabaena sp. PCC7120) cultivated in airlift photobioreactor. Int. J. Hydrogen Energy. 2014, 39, 7553–7560. [Google Scholar] [CrossRef]
- Kumar, K.; Dasgupta, C.N.; Nayak, B.; Lindblad, P.; Das, D. Review. Development of suitable photobioreactors for CO2 sequestration addressing global warming using Green algae and cyanobacteria. Bioresour. Technol. 2011, 102, 4945–4953. [Google Scholar] [CrossRef]
- Chisti, Y. Biodiesel from microalgae. Biotechnol. Adv. 2007, 24, 294–306. [Google Scholar] [CrossRef]
- Cheng, J.; Huang, Y.; Feng, J.; Sun, J.; Zhou, J.; Cen, K. Improving CO2 fixation efficiency by optimizing Chlorella PY-ZU1 culture conditions in sequential bioreactors. Bioresour. Thechnol. 2013, 144, 321–327. [Google Scholar] [CrossRef]
- Chang, E.H.; Yang, S.S. Some characteristics of microalgae isolated in Taiwan for biofixation of carbon dioxide. Bot. Bull. Acad. 2003, 44, 43–52. [Google Scholar]
- Jiang, Y.; Zhang, W.; Wang, J.; Chen, Y.; Shen, S.; Liu, T. Utilization of simulated flue gas for cultivation of Scenedesmus dimorphus. Bioresourse Technol. 2013, 128, 359–364. [Google Scholar] [CrossRef]
- Olguín, E.J. International Training Course. In the Use of Microalgae for the Removal of Pollutants and for the Production of Biofuels; Xalapa: Veracruz, Mexico, 2013. [Google Scholar]
- American Public Health Association, American Water Works Association, Water Pollution Control Federation. Standard Methods for Examination of Water and Wastewater; American Public Health Association: Washington, DC, USA, 1992. [Google Scholar]
- Merchuk, J.C.; Gluz, M. Bioreactors, Air Lift Reactors; Wiley Online Library: Hoboken, NJ, USA, 2002; pp. 320–352. [Google Scholar]
- Chisti, M.Y.; Halard, L.B.; Moo-Young, M. Liquid circulation in airlift reactors. Chem. Eng. Sci. 1988, 43, 451–457. [Google Scholar] [CrossRef]
- Shi, L.K.; Riba, J.P.; Angelino, H. Estimation of effective shear rate for aerated non-Newtonian liquids in airlift bioreactor. Chem. Eng. Comm. 1990, 89, 25–35. [Google Scholar] [CrossRef]
- Merchuk, J.C.; Ben-Zvi, S. A novel approach to the correlation of mass transfer in bubble columns with non-Newtonian liquids. Chem. Eng. Sci. 1992, 47, 3517–3523. [Google Scholar] [CrossRef]
- Shumpe, A.; Deckwer, W.D. Gas holdups, specific interfacial area, and mass transfer coefficients of aerated carboxy methyl cellulose solutions in a bubble column. Ing. Eng. Chem. Process Des. Dev. 1982, 21, 706–711. [Google Scholar] [CrossRef]
- Valdés, F.J.; Hernández, M.R.; Catalá, L.; Marcilla, A. Estimation of CO2 stripping/CO2 microalgae consumption ratios in a bubble column photobioreactor using the analysis of the pH profiles. Application to Nannochloropsis oculata microalgae culture. Bioresour. Technol. 2012, 119, 1–6. [Google Scholar] [CrossRef]
- Royce, P.N.C.; Thornhill, N.F. Estimation of dissolved carbon dioxide concentrations in aerobic fermentations. AICHE J. 1991, 37, 1680–1686. [Google Scholar] [CrossRef]
- Brune, D.E.; Novak, J.T. The use of Carbonate Equilibrium Chemistry in Quantifying Algal Carbon Uptake Kinetics. Eur. J. Appl. Microbiol. Biotechnol. 1981, 13, 71–76. [Google Scholar] [CrossRef]
- Camacho, R.F.; Acién Fernández, F.G.; Sánchez Pérez, J.A.; García Camacho, F.; Molina Grima, E. Prediction of disolved Oxigen and Carbon Dioxide Concentration Profiles in Tubular Photobioreactors for Microalgal Culture. Biotechnol. Bioeng. 1999, 62, 71–86. [Google Scholar] [CrossRef]
- Livansky, K. Losses of CO2 in outdoor mass algal cultures: Determination of the mass transfer coefficient KL by means of measured pH course in NaHCO3 solution. Algol. Stud. 1990, 58, 87–97. [Google Scholar]
- Kargi, F.; Moo-Young, M. Transport Phenomena in Bioprocesses; Moo-Young, M., Ed.; Comprehensive Biotechnology, Pergamon Press: Oxford, UK, 1985; pp. 5–55. [Google Scholar]
- Per-Ander, C.; Skoglundh, M. Low–Temperture oxidation of carbón monoxide and methane over alumina and ceria supported platinum Catalysts. Appl. Catal. B Environ. 2011, 101, 669–675. [Google Scholar]
- Graham, L.E.; Y Wilcox, L.W. Algae; Prentice Hall: Upper Saddle River, NJ, USA, 2000. [Google Scholar]
- Contreras, E.M. Carbon dioxide stripping in bubble columns. Ind. Eng. Chem. Res. 2007, 46, 6332–6337. [Google Scholar] [CrossRef]
- Landau, L.D. Fluid Mechanics; Pergamon: Oxford, UK, 1975; Volume 6, pp. 115–123. [Google Scholar]
- Zaidi, A.; Bourziza, H.; Echihabi, L. Warmeubergang und effektives Schergefalle in Blasensaule-Bioreaktoren mit Xanthan-Losungen. Chem. Ing. Tech. 1987, 59, 748–749. [Google Scholar] [CrossRef]
- Nishikawa, M.; Kato, H.; Hashimoto, K. Heat transfer in aerated tower filled with non-Newtonian Liquids. Ind. Eng. Chem. Process Des. Dev. 1977, 16, 133–137. [Google Scholar] [CrossRef]
- Pirouzi, A.; Nosrati, M.; Shojaosadati, S.; Shakhesi, S. Improvement of mixing time, mass transfer, and power consumption in an external loop airlift photobioreactor for microalgae culures. Biochem. Eng. J. 2014, 87, 25–32. [Google Scholar] [CrossRef]
- Loubiere, K.; Pruvost, J.; Alour, F.; Legrand, J. Investigations in an external-loop airlift photobioreactor wih annular light chambers and swirling flow. Chem. Eng. Res. Des. 2011, 89, 164–171. [Google Scholar] [CrossRef][Green Version]
- Reyna-Velarde, R.; Cristiani-Urbina, E.; Hernández-Melchor, D.J.; Thalasso, F.; Cañizares-Villanueva, R.O. Hydrodinamic and mass transfer characterization of a flat-panel airlift photobioreactor with high light path. Chem. Eng. Process. 2010, 49, 97–103. [Google Scholar] [CrossRef]
- Contreras, A.; García, F.; Molina, E.; Merchuk, J.C. Interaction between CO2-mass transfer, light availability, and hydrodynamic stress in the growth of Phaeodactylum tricornutum in a concentric tube airlift photobioreactor. Biotechnol. Bioeng. 1998, 60, 317–325. [Google Scholar] [CrossRef]
- Kazbar, A.; Cogne, G.; Urbain, B.; Marec, H.; Le-Gounic, B.; Tallec, J.; Takache, H.; Ismail, A.; Pruvost, J. Effect of dissolved oxygen concentration on microalgal culture in photobioreactors. Algal Res. 2019, 39, 101432. [Google Scholar] [CrossRef]
- Roustan, M. Transferts Gas-Liquide dans les Procédes de Traitement des eaux et des Effluents Gazeux [WWW Document]. Lithium Bromide. Lavoisier. 2003. Available online: https://www.lavoisier.fr/libre/enviornnement/transferts-gaz-liquide-dans-les-procedes-de-traitment-des-eaux-et-des-effluents-gazeux/roustan/descriptif-9782743006051 (accessed on 15 August 2017).
- Xue, S.; Su, Z.; Cong, W. Growth of Spirulina platensis enhanced under intermitent illumination. J. Biotechnol. 2011, 151, 271–277. [Google Scholar] [CrossRef]
- Basu, S.; Roy, A.S.; Mohanty, K.; Ghoshal, A.K. Enhanced CO2 sequestration by a novel microalga: Scenedesmus obliquus SA1 isolated from bio-diversity hotspot region of Assam, India. Bioresour. Technol. 2013, 143, 369–377. [Google Scholar] [CrossRef]
- Thawechai, T.; Cheirsilp, B.; Louhasakul, Y.; Boonsawang, P.; Prasertsan, P. Mitigation of carbon dioxide by oleaginous microalgae for lipids and pigments production: Effect of light illumination and carbon dioxide feeding strategies. Bioresour. Technol. 2016, 219, 139–149. [Google Scholar] [CrossRef]




| Culture | Light Intensity (μmol·m−2·s−1) | Light/Dark Cycle (h) | Gas Flow (vvm) |
|---|---|---|---|
| C1 | 76.27 | 12/12 | 0.05 |
| C2 | 76.27 | 12/12 | 0.10 |
| C3 | 76.27 | 12/12 | 0.15 |
| C4 | 27 | 14/10 | 0.1 |
| C5 | 60.75 | 14/10 | 0.1 |
| C6 | 76.27 | 14/10 | 0.1 |
| C7 | 60.75 | 16/8 | 0.1 |
| C8 | 60.75 | 24/0 | 0.1 |
| Chemical Element | In the Monolith Structure (wt.%) | In the Supported Active Phase (wt.%) |
|---|---|---|
| O2 | 50.04 | 41.26 |
| Mg | 7.32 | 5.45 |
| Al | 17.73 | 18.59 |
| Si | 24.91 | 15.18 |
| Ce | 0 | 16.2 |
| Pt | 0 | 3.33 |
| Culture | Qg (L·min−1) | Ugr (m·s−1) | Ulr (m·s−1) | Uld (m·s−1) | Ub (m·s−1) | φr | Rer | Red | (γ) (1/s) |
|---|---|---|---|---|---|---|---|---|---|
| C1 | 0.137 | 0.0018 | 0.00078 | 0.00032 | 0.367 | 0.0049 | 3121 | 902 | 8.07 |
| C2 | 0.275 | 0.0036 | 0.0015 | 0.00065 | 0.439 | 0.0082 | 6241 | 1805 | 9.42 |
| C3 | 0.412 | 0.0054 | 0.00234 | 0.00098 | 0.337 | 0.016 | 9362 | 2708 | 10.5 |
| Culture | Biomass (g·L−1) | μmax (d−1) | P (gcel L−1·d−1) | Fc (gCO2 L−1 ·h−1) |
|---|---|---|---|---|
| C1 | 3.8 | 0.22 | 0.29 | 0.53 |
| C2 | 3.27 | 0.19 | 0.27 | 0.49 |
| C3 | 3.4 | 0.13 | 0.22 | 0.41 |
| C4 | 1.55 | 0.15 | 0.09 | 0.23 |
| C5 | 1.85 | 0.22 | 0.16 | 0.28 |
| C6 | 3.25 | 0.29 | 0.25 | 0.46 |
| C7 | 4.73 | 0.24 | 0.44 | 0.80 |
| C8 | 4.79 | 0.21 | 0.37 | 0.69 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Arroyo, C.A.; Contreras, J.L.; Zeifert, B.; Ramírez C., C. CO2 Capture of the Gas Emission, Using a Catalytic Converter and Airlift Bioreactors with the Microalga Scenedesmus dimorphus. Appl. Sci. 2019, 9, 3212. https://doi.org/10.3390/app9163212
Arroyo CA, Contreras JL, Zeifert B, Ramírez C. C. CO2 Capture of the Gas Emission, Using a Catalytic Converter and Airlift Bioreactors with the Microalga Scenedesmus dimorphus. Applied Sciences. 2019; 9(16):3212. https://doi.org/10.3390/app9163212
Chicago/Turabian StyleArroyo, Citlalli Adelaida, José Luis Contreras, Beatriz Zeifert, and Clementina Ramírez C. 2019. "CO2 Capture of the Gas Emission, Using a Catalytic Converter and Airlift Bioreactors with the Microalga Scenedesmus dimorphus" Applied Sciences 9, no. 16: 3212. https://doi.org/10.3390/app9163212
APA StyleArroyo, C. A., Contreras, J. L., Zeifert, B., & Ramírez C., C. (2019). CO2 Capture of the Gas Emission, Using a Catalytic Converter and Airlift Bioreactors with the Microalga Scenedesmus dimorphus. Applied Sciences, 9(16), 3212. https://doi.org/10.3390/app9163212