Deformations of FRP–Concrete Composite Beam: Experiment and Numerical Analysis
Abstract
:Featured Application
Abstract
1. Introduction
2. Test Program
2.1. Material Properties
2.2. Bending Test Specimens
2.3. Test Apparatus
2.4. Test Results
3. Deformation Analysis
3.1. Tension-Stiffening Model
3.2. Numerical Simulations
4. Discussion
5. Conclusions
- The tension-stiffening approach was not capable of predicting the deformation response of GFRP–concrete elements. The adequate application of this approach is limited to the FE models with reinforcement simulated as embedded truss elements. Contrarily, the tension-softening effect is predominant for the modelling of FRP–concrete specimens.
- A detail FE model is capable of representing deformation behaviour and failure mechanisms of GFRP–concrete composite elements. However, engineering applications of such an approach can be problematic because of the existing hardware limitations.
- A simplified model is proposed to solve the above problem. The model assumes a perfectly elastic model of the compressive concrete; the contribution of the tensile concrete and adhesive are neglected. The predictions are in a satisfactory agreement with the experimental results. The prediction error, corresponding to the service load, is approximately equal to 2%.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Zuo, Y.; Mosallam, A.; Xin, H.; Liu, Y.; He, J. Flexural performance of a hybrid GFRP-concrete bridge deck with composite T-shaped perforated rib connectors. Compos. Struct. 2018, 194, 263–278. [Google Scholar] [CrossRef]
- Siwowski, T.; Rajchel, M.; Kulpa, M. Design and field evaluation of a hybrid FRP composite—Lightweight concrete road bridge. Compos. Struct. 2019, 230, 111504. [Google Scholar] [CrossRef]
- Sapuan, S.M.; Mansor, M.R. Concurrent engineering approach in the development of composite products: A review. Mater. Des. 2014, 58, 161–167. [Google Scholar] [CrossRef]
- Meier, U. Structural tensile elements made of advanced composite materials. Struct. Eng. Int. 1999, 9, 281–285. [Google Scholar] [CrossRef]
- Czaderski, S.; Meier, U. EBR strengthening technique for concrete, long-term behaviour and historical survey. Polymers 2018, 10, 77. [Google Scholar] [CrossRef] [PubMed]
- Correia, J.R. Pultrusion of advanced fibre-reinforced polymer (FRP) composites. In Advanced Fibre-Reinforced Polymer (FRP) Composites for Structural Applications; Woodhead Publishing Limited: Cambridge, UK, 2013; pp. 207–251. [Google Scholar]
- Custódio, J.; Cabral-Fonseca, S. Advanced fibre-reinforced polymer (FRP) composites for the rehabilitation of timber and concrete structures: Assessing strength and durability. In Advanced Fibre-Reinforced Polymer (FRP) Composites for Structural Applications; Woodhead Publishing Limited: Cambridge, UK, 2013; pp. 814–882. [Google Scholar]
- Wan, B. Using fiber-reinforced polymer (FRP) composites in bridge construction and monitoring their performance: An overview. In Advanced Composites in Bridge Construction and Repair; Woodhead Publishing Limited: Cambridge, UK, 2014; pp. 3–29. [Google Scholar]
- Akbarzadeh, H.; Maghsoudi, A.A. Experimental and analytical investigation of reinforced high strength concrete continuous beams strengthened with fiber reinforced polymer. Mater. Des. 2010, 31, 1130–1147. [Google Scholar] [CrossRef]
- Saadatmanesh, H.; Ehsani, M.R. RC beams strengthened with GFRP plates. I: Experimental study. J. Struct. Eng. ASCE 1991, 117, 3417–3433. [Google Scholar] [CrossRef]
- Taheri, F.; Shahni, K.; Widiarsa, I. On the parameters influencing the performance of reinforced concrete beams strengthened with FRP plates. Compos. Struct. 2002, 58, 217–226. [Google Scholar] [CrossRef]
- Gribniak, V.; Ng, P.L.; Tamulenas, V.; Misiūnaitė, I.; Norkus, A.; Šapalas, A. Strengthening of fibre reinforced concrete elements: Synergy of the fibres and external sheet. Sustainability 2019, 11, 4456. [Google Scholar] [CrossRef]
- Wu, Z.J.; Davies, J.M. Mechanical analysis of a cracked beam reinforced with an external FRP plate. Compos. Struct. 2003, 62, 139–143. [Google Scholar] [CrossRef]
- Achintha, M.; Burgoyne, C.J. Fracture mechanics of plate debonding: Validation against experiment. Constr. Build. Mater. 2011, 25, 2961–2971. [Google Scholar] [CrossRef]
- Wroblewski, L.; Hristozov, D.; Sadeghian, P. Durability of bond between concrete beams and FRP composites made of flax and glass fibers. Constr. Build. Mater. 2016, 15, 800–811. [Google Scholar] [CrossRef]
- Hollaway, L.C. A review of the present and future utilisation of FRP composites in the civil infrastructure with reference to their important in-service properties. Constr. Build. Mater. 2010, 24, 2419–2445. [Google Scholar] [CrossRef]
- Li, Z.; Khennane, A.; Hazell, P.J.; Remennikov, A.M. Performance of a hybrid GFRP-concrete beam subject to low-velocity impacts. Compos. Struct. 2018, 206, 425–438. [Google Scholar] [CrossRef]
- Attari, N.; Amziane, S.; Chemrouk, M. Flexural strengthening of concrete beams using CFRP, GFRP and hybrid FRP sheets. Constr. Build. Mater. 2012, 37, 746–757. [Google Scholar] [CrossRef]
- Hawileh, R.A.; Rasheed, H.A.; Abdalla, J.A.; Al-Tamimi, A.K. Behaviour of reinforced concrete beams strengthened with externally bonded hybrid fiber reinforced polymer systems. Mater. Des. 2014, 53, 972–998. [Google Scholar] [CrossRef]
- Gribniak, V.; Cervenka, V.; Kaklauskas, G. Deflection prediction of reinforced concrete beams by design codes and computer simulation. Eng. Struct. 2013, 56, 2175–2186. [Google Scholar] [CrossRef]
- Kaklauskas, G.; Ghaboussi, J. Stress-strain relations for cracked tensile concrete from RC beam tests. J. Struct. Eng. ASCE 2001, 127, 64–73. [Google Scholar] [CrossRef]
- Torres, L.; López-Almansa, F.; Bozzo, L.M. Tension-Stiffening model for cracked flexural concrete members. J. Struct. Eng. ASCE 2004, 130, 1242–1251. [Google Scholar] [CrossRef]
- Gribniak, V.; Sokolov, A.; Rimkus, A. A new approach to quantification of residual flexural stiffness of reinforced concrete. In Proceedings of the International Conference on Sustainable Materials, Systems and Structures (SMSS 2019)—Novel Methods for Characterization of Materials and Structures, Rovinj, Croatia, 20–22 March 2019; RILEM Publications SARL: Paris, France, 2019; Volume 5, pp. 232–239. [Google Scholar]
- Gribniak, V.; Sokolov, A.; Rimkus, A.; Sultani, H.A.; Tuncay, M.C.; Torres, L. A novel approach to residual stiffness of flexural concrete elements with composite reinforcement. In Proceedings of the IABSE Symposium—Towards a Resilient Built Environment Risk and Asset Management, Guimarães, Portugal, 27–27 March 2019; IABSE: Zurich, Switzerland, 2019; pp. 46–51. [Google Scholar]
- Gribniak, V.; Kaklauskas, G.; Juozapaitis, A.; Kliukas, R.; Meskenas, A. Efficient technique for constitutive analysis of reinforced concrete flexural members. Inverse Probl. Sci. Eng. 2017, 25, 27–40. [Google Scholar] [CrossRef]
- International Federation for Structural Concrete (FIB). Model Code for Concrete Structures 2010; Wilhelm Ernst & Sohn: Berlin, Germany, 2013; 434p. [Google Scholar]
- Bažant, Z.P.; Oh, B.H. Crack band theory for fracture of concrete. Mater. Struct. 1983, 16, 155–177. [Google Scholar] [CrossRef]
- Hordijk, D.A. Local Approach to Fatigue of Concrete. Ph.D. Thesis, Delft University of Technology, Delft, The Netherlands, 2013; 210p. [Google Scholar]
- Gribniak, V.; Jakubovskis, R.; Rimkus, A.; Ng, P.-L.; Hui, D. Experimental and numerical analysis of strain gradient in tensile concrete prisms reinforced with multiple bars. Constr. Build. Mater. 2018, 187, 572–583. [Google Scholar] [CrossRef] [Green Version]




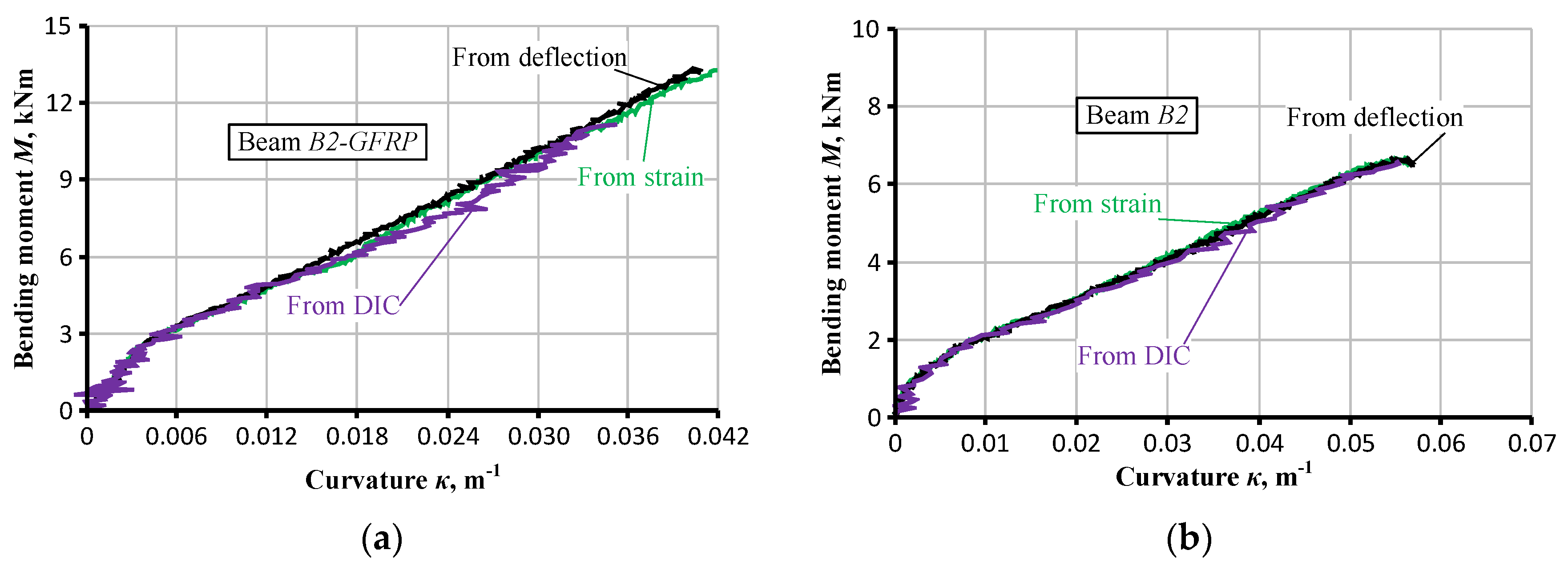
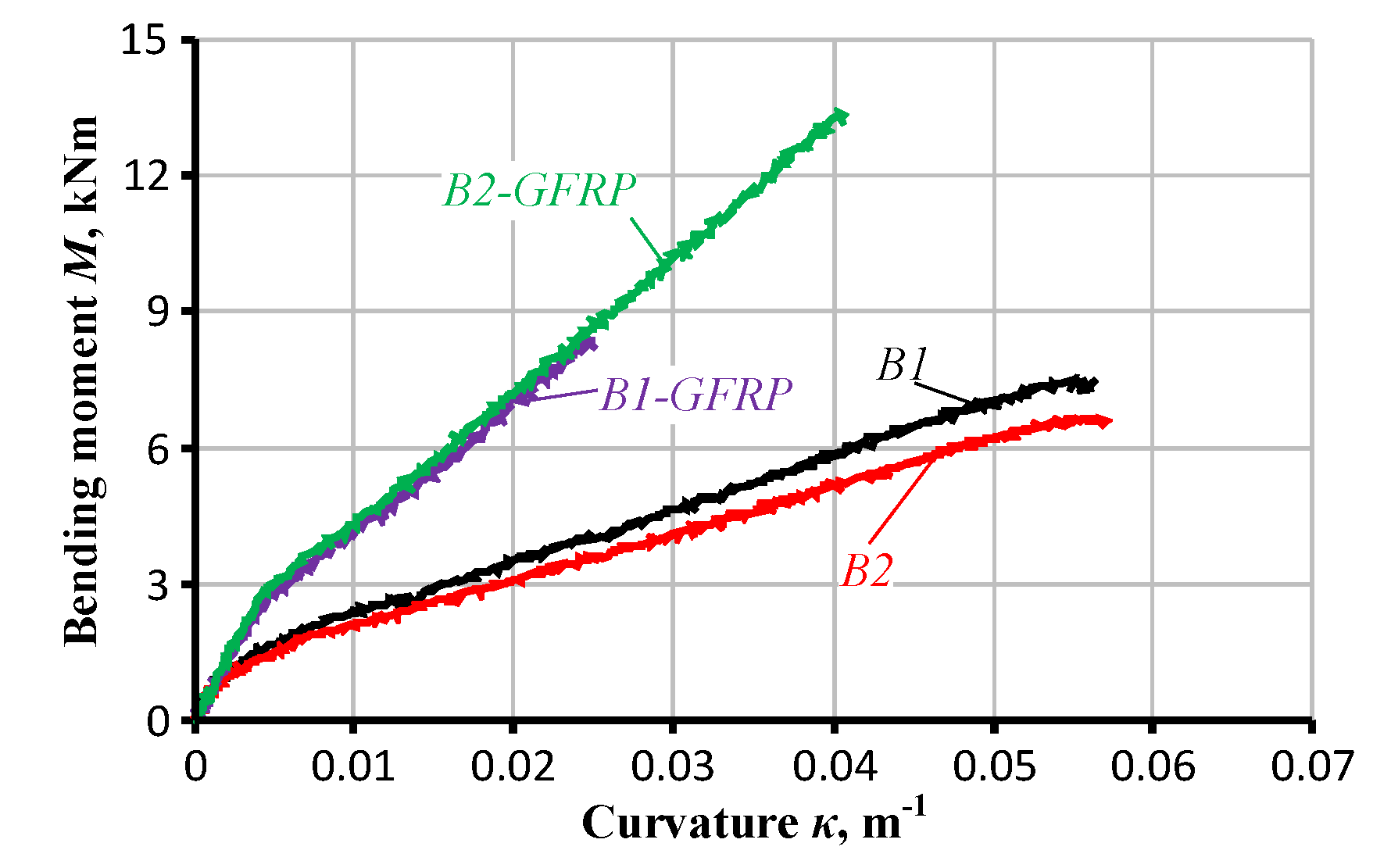

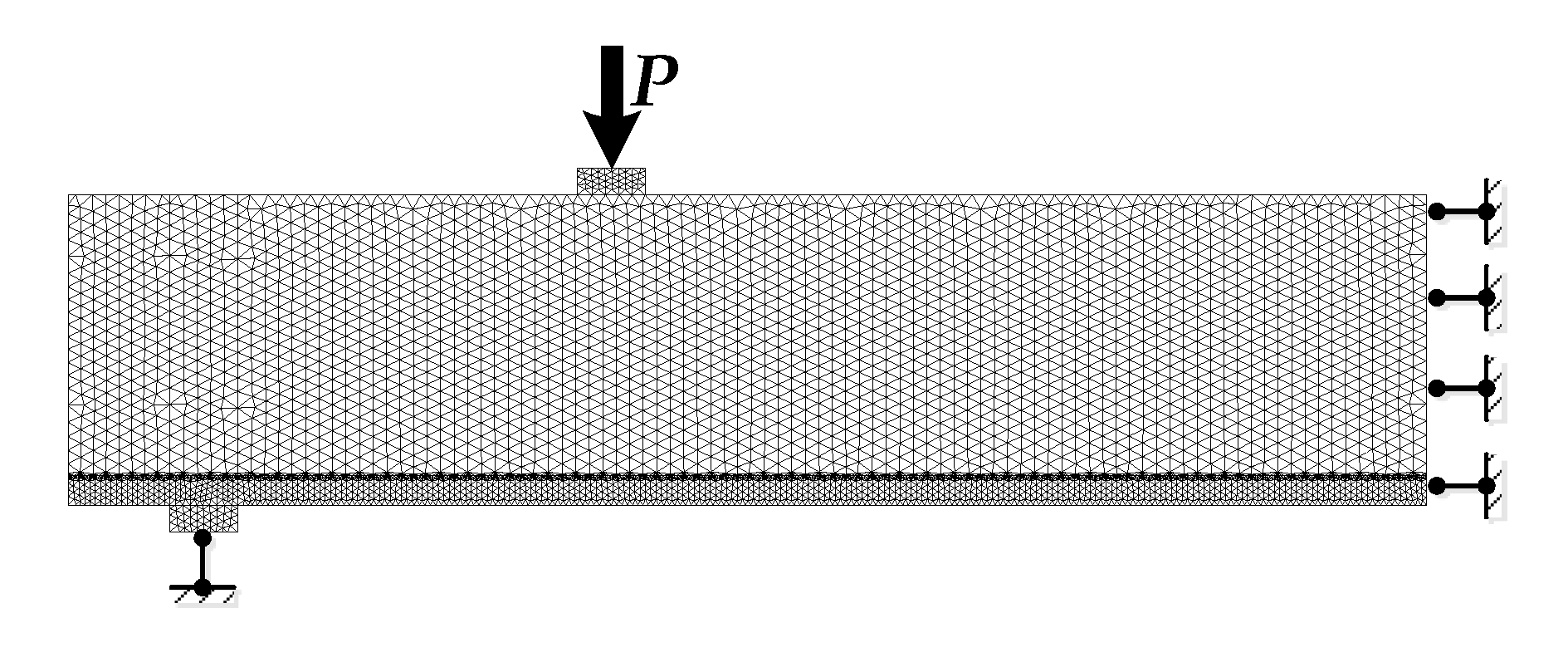


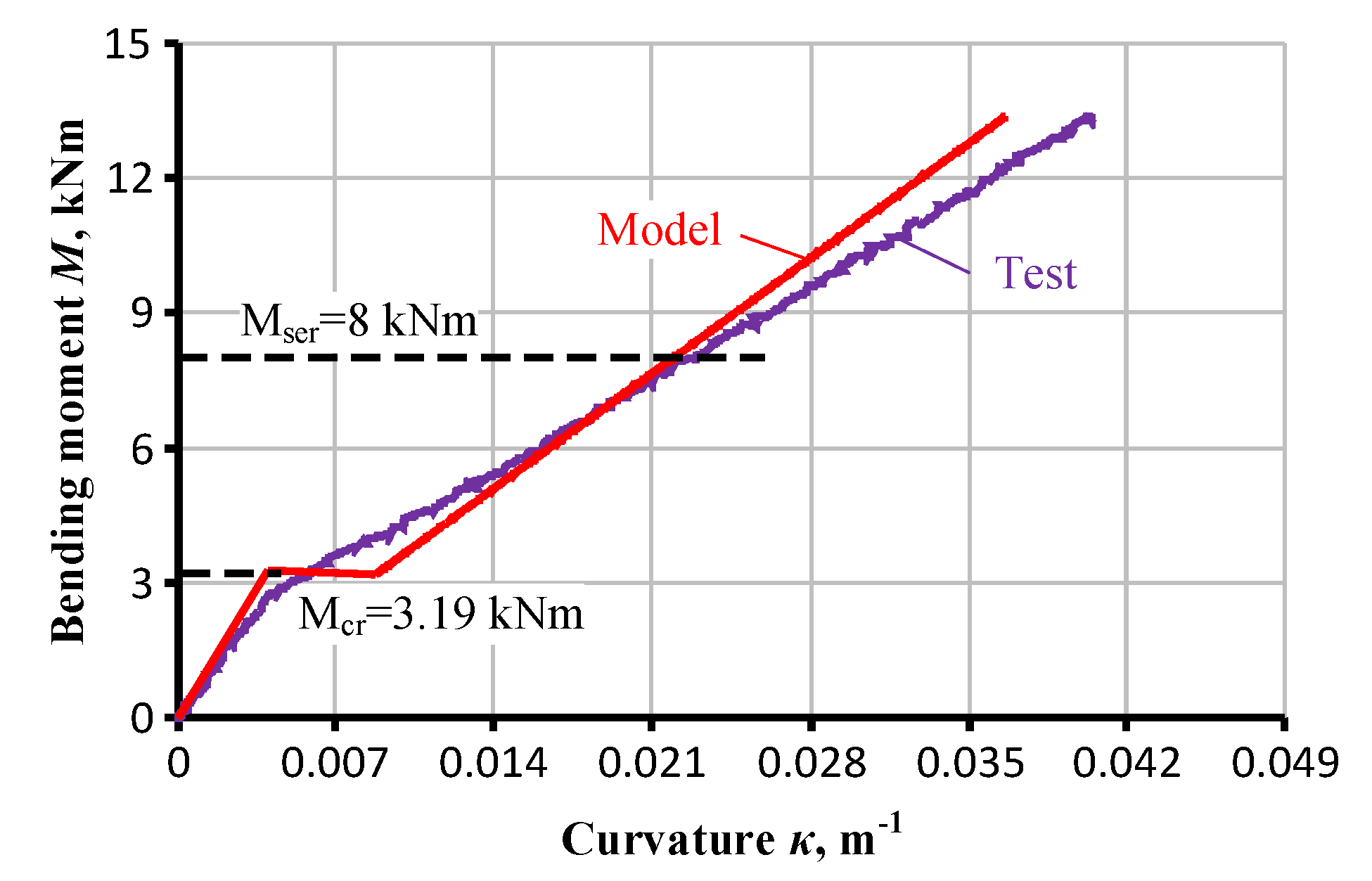
| Specimen | h, mm | b, mm | d (*), mm | t, mm | Ar, mm2 | Er, GPa | Ea, GPa |
|---|---|---|---|---|---|---|---|
| B1-GFRP | 104 | 197 | 109 | 1.0 | 800.0 | 58.1 | 7.47 |
| B2-GFRP | 106 | 200 | 111 | 1.0 | 800.0 | 58.1 | 7.47 |
| B1 | 105 | 201 | 80 | – | 150.8 | 196 | – |
| B2 | 102 | 198 | 75 | – | 150.8 | 196 | – |
| FE Model | Model for Concrete | Adhesive | |
|---|---|---|---|
| Tension | Compression | ||
| #1 | 3D Non Linear ementitious 2 User | Elastic | – |
| #2 | Sbeta, Gf = 46 N/m | Elastic | Elastic, 1 mm depth |
| #3 | Sbeta, Gf = 46 N/m | Nonlinear | Elastic, 1 mm depth |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gribniak, V.; Misiūnaitė, I.; Rimkus, A.; Sokolov, A.; Šapalas, A. Deformations of FRP–Concrete Composite Beam: Experiment and Numerical Analysis. Appl. Sci. 2019, 9, 5164. https://doi.org/10.3390/app9235164
Gribniak V, Misiūnaitė I, Rimkus A, Sokolov A, Šapalas A. Deformations of FRP–Concrete Composite Beam: Experiment and Numerical Analysis. Applied Sciences. 2019; 9(23):5164. https://doi.org/10.3390/app9235164
Chicago/Turabian StyleGribniak, Viktor, Ieva Misiūnaitė, Arvydas Rimkus, Aleksandr Sokolov, and Antanas Šapalas. 2019. "Deformations of FRP–Concrete Composite Beam: Experiment and Numerical Analysis" Applied Sciences 9, no. 23: 5164. https://doi.org/10.3390/app9235164
APA StyleGribniak, V., Misiūnaitė, I., Rimkus, A., Sokolov, A., & Šapalas, A. (2019). Deformations of FRP–Concrete Composite Beam: Experiment and Numerical Analysis. Applied Sciences, 9(23), 5164. https://doi.org/10.3390/app9235164







