RETRACTED: The Effect of Structural Phase Transitions on Electronic and Optical Properties of CsPbI₃ Pure Inorganic Perovskites

Abstract

Hybrid inorganic perovskites (HIPs) have been developed in recent years as new high-efficiency semiconductors with a wide range of uses in various optoelectronic applications such as solar cells and light-emitting diodes (LEDs). In this work, we used a first-principles theoretical study to investigate the effects of phase transition on the electronic and optical properties of CsPbI₃ pure inorganic perovskites. The results showed that at temperatures over 300 °C, the structure of CsPbI₃ exhibits a cube phase (pm3m) with no tilt of PbI₆ octahedra (distortion index = 0 and bond angle variance = 0). As the temperature decreases (approximately to room temperature), the PbI₆ octahedra is tilted, and the distortion index and bond angle variance increase. Around room temperature, the CsPbI₃ structure enters an orthorhombic phase with two tilts PbI₆ octahedra. It was found that changing the halogens in all structures reduces the volume of PbI₆ octahedra. The tilted PbI₆ octahedra causes the distribution of interactions to vary drastically, which leads to a change in band gap energy. This is the main reason for the red and blue shifts in the absorption spectrum of CsPbI₃. In general, it can be said that the origin of all changes in the structural, electronic, and optical properties of HIPs is the changes in the volume, orientation, and distortion index of PbI₆ octahedra.

1. Introduction

Lead halide-based perovskites have been widely studied as absorbing materials for thin film solar cells with a power conversion efficiency of about 20%, [1,2,3,4,5,6,7,8,9,10,11,12,13,14]. These compounds can also be used as a promising emitter in light-emitting diodes (LEDs) [15,16,17,18,19,20,21,22,23,24,25,26,27,28]. The general chemical formula for HIPs is ABX₃, where A is a monovalent inorganic cation, similar to Cs⁺: cesium; X is a halogen anion including F⁻, I⁻, Br⁻, and Cl⁻, and B is a divalent metallic cation such as Pb²⁺, Sn²⁺, Cu²⁺, etc. [29,30,31,32,33,34,35,36,37,38,39,40,41,42]. Inorganic hybrid perovskites have a semiconducting nature. In all types of hybrid perovskites, divalent metallic cations form a BX₆ octahedral with halogen anions [43,44,45,46,47,48,49,50,51,52,53,54,55,56]. Most of the electronic and optical properties of hybrid perovskites depend on the BX₆ framework [57,58,59,60,61,62,63,64,65,66,67,68,69,70]. These materials are found in an orthorhombic phase at low temperatures (space group: Pnma) [71,72,73,74,75,76,77,78,79,80,81,82,83,84]. As the temperature increases to about 50 °C, the structure of HIPs takes a tetragonal phase with the space group P4/mbm, and at temperatures above 300 °C, they are in the original cubic phase with the space group Pm3m. In hybrid perovskite structures, by changing the halogens from iodine to bromine and then chlorine, the BX₆ octahedral volume decreases, resulting in a reduction in total volume of the structures [85,86,87,88,89,90].To acquire a comprehensive knowledge of the electrical and optical properties of semiconductor compounds, the band structure, electron density, charge carrier mobility, density of states, and dielectric function must be investigated [91,92,93,94,95].

The study of the characteristics of organic/inorganic hybrid perovskites suggests that these materials might be a viable alternative to semiconductor materials such as silicon, as well as semiconductor compounds such as Cu-InGeS (CIGS) and CuZnSnS (CZTS), which are extensively used in various optoelectronic devices [96,97,98,99,100]. Recent advances in the synthesis of inorganic hybrid perovskite nanostructures have increased the importance of HOIPs [101,102,103,104,105,106,107,108].

Due to their special properties such as broadband infrared absorption, narrow PL emission bandwidth, high photoluminescence (PL), quantum yield (QY) ΦPL, adjustable emission across the visible range, and desirable optical gain combined with low-threshold spontaneous emission, inorganic cesium lead halide perovskite colloidal quantum dots (CsPbX₃ QDs, X = Cl, Br, I) have attracted considerable attention in many applications of solar cells, LEDs, lasers, and photodetectors [109,110,111]. However, the chemical stability of CsPbX₃ QDs remains a serious issue, particularly for infrared applications. Indeed, the low chemical stability of APbX₃ (where A = (CH3NH)³⁺, or HC (NH₂)²⁺; X = Br⁻, I⁻, and/or Cl⁻) is the major impediment to the industrialization of corresponding devices as well as fundamental research into these compounds [112,113]. Obviously, more research into the structural and electrical properties, phase, and single crystals of these appealing semiconductor compounds can lead to the best use of their benefits in diverse applications while also improving their disadvantages [114,115].

In this work, we investigated the behavior of PbI₆ inorganic octahedral upon changing the structural phases of CsPbI₃ and assessed the contribution of the pb-I framework to the electronic and optical properties of CsPbI₃.

2. Computational Details

The PWSCF code, as implemented in the Quantum-Espresso package, was used for all computations [58]. Within a generalized gradient approximation, the Perdew–Burke–Ernzerhof exchange-correlation functional [59] was employed (GGA). The cubic and orthorhombic phases of pure inorganic perovskite materials with the chemical structure CsPbI₃ were the focus of our research. The density of valence electrons and wave functions were represented using scalar relativistic ultra-soft and plane-wave basis set pseudo-potentials. Wave functions and electron density were also represented using energy cutoffs of 35 and 330 Rydberg, respectively. A 12 × 12 × 12 Monkhorst–Pack grid [115,116,117,118,119] was selected for sampling the Brillouin zone (BZ) of the cubic systems. The structure was totally relaxed until each atom’s force was less than 0.0025 eV·A⁻¹. We initially computed the frequency-dependent complex dielectric function to assess the optical properties [116].

$$\epsilon \left(\omega \right)=1+\frac{8\pi }{\mathsf{\Omega }{N}_k}{\displaystyle \sum }_{k,v,c}\frac{{|\langle {\phi }_{kv}\left|\widehat{v}\right|{\phi }_{kc}\rangle |}^2}{{\left(E_{kc}-E_{kv}\right)}^2\left(E_{kc}-E_{kv}-\omega -i\eta \right)}$$

(1)

where Ω is the cell volume, $N_k$ is the total number of k-points in the BZ, $\widehat{v}$ is the operator of velocity, and η is an opportune broadening factor. The indices v and c represent the occupied and unoccupied states, respectively.

It is feasible to acquire the whole dielectric tensor calculated on the imaginary frequency axe $\epsilon \left(i\omega \right)$ by applying a London transformation on ${\epsilon }_i\left(\omega \right)$ [58].

$$\epsilon \left(i\omega \right)=1+\frac{2}{\pi }{{\displaystyle \int }}_0^{\infty }\frac{{\omega }^{\prime }{\epsilon }_i\left({\omega }^{\prime }\right)}{{\omega }^{\prime 2}+{\omega }^2}d\omega$$

(2)

The LOSS spectrum is proportional to the imaginary part of the inverse dielectric tensor [58]:

$$\mathrm{Imm}\left\{\frac{1}{\epsilon \left(\omega \right)}\right\}=\frac{{\epsilon }_i\left(\omega \right)}{{\epsilon }_r^2\left(\omega \right)+{\epsilon }_i^2\left(\omega \right)}$$

(3)

The refractive index, n(ω) was calculated using the following formula:

$$n\left(\omega \right)=\omega \sqrt{\frac{{\epsilon }_r\left(\omega \right)+\sqrt{{\epsilon }_r^2\left(\omega \right)+{\epsilon }_i^2\left(\omega \right)}}{2}}$$

(4)

The frequency dependent absorption coefficient, α(ω), was also computed using the following relationship:

$$\alpha \left(\omega \right)=\omega \sqrt{\frac{-{\epsilon }_r\left(\omega \right)+\sqrt{{\epsilon }_r^2\left(\omega \right)+{\epsilon }_i^2\left(\omega \right)}}{2}}$$

(5)

3. Results

Figure 1 schematically illustrates the three-dimensional structure of the CsPbI₃ inorganic perovskite in the cubic and orthorhombic phases. At temperatures above 300 °C, the CsPbI₃ structure is in the cubic phase (Figure 1a). However, as the temperature decreases to around room temperature, the structure of the inorganic perovskite undergoes a phase transition and finds an orthorhombic phase at 25 °C (Figure 1b). The lattice parameters, band gaps, total structure volumes, and optimization energies are provided for both cubic and orthorhombic phases in

Table 1. Lattice parameters, structure volumes, band gap values, and optimization energies of CsPbI₃ for both cubic and orthorhombic phases.

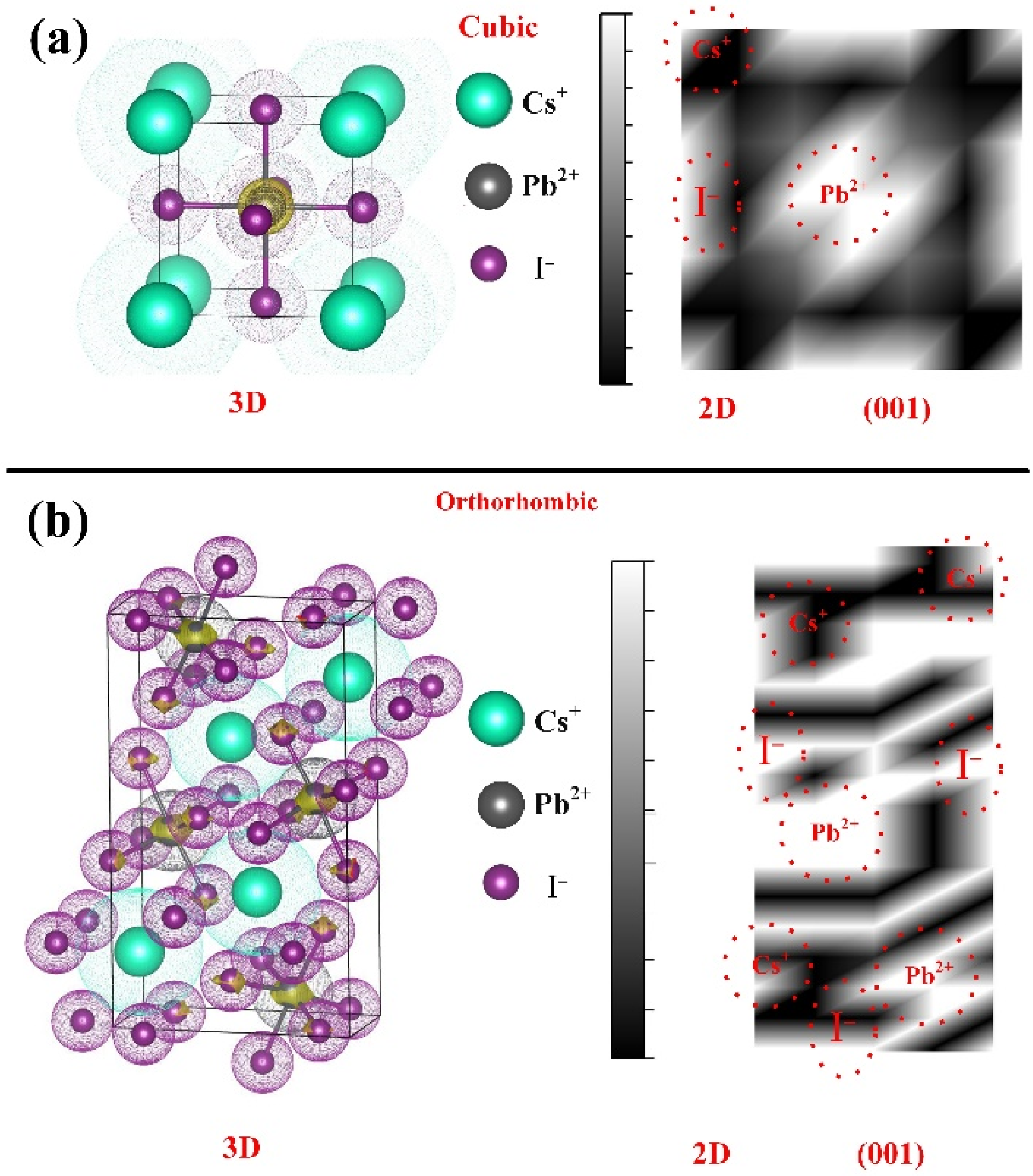
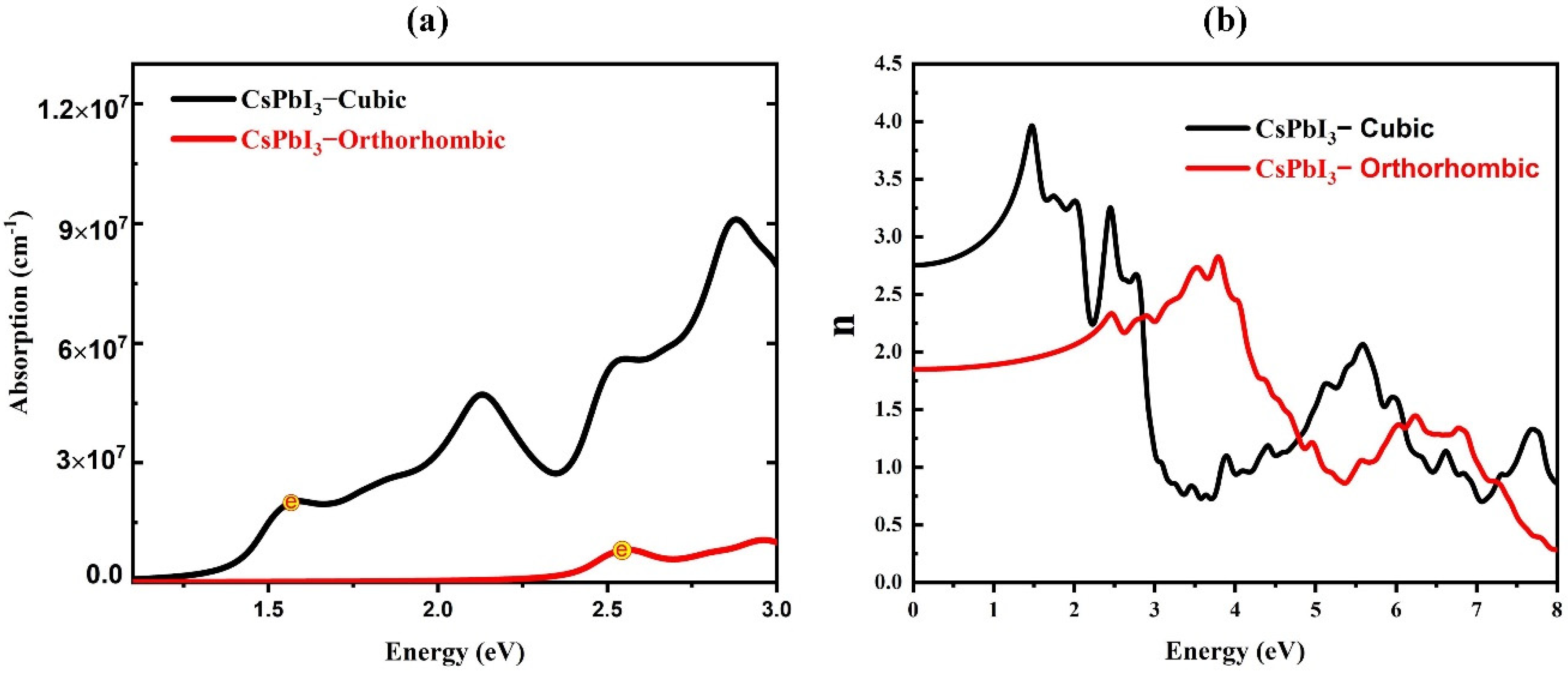
Component	Lattice Parameter (Å)	Band Gap (eV)	Volume (Å)3	Optimization Energy (eV)
CsPbI3−Cubic	a = b = c = 6.40	1.62	262.97	−5265.40
CsPbI3−Orthorhombic	a = 10.53, b = 4.87, c = 18.07	2.45	927.092	−4223.486

. As can be seen, the volume of the CsPbI₃ structure increases several times when the structural phase is transformed from cubic into orthorhombic. According to Table 1, the semiconductor nature of the pure inorganic perovskite is preserved during the structural phase transition caused by the temperature change. This can be attributed to the appropriate ratio of the lead atoms to the cesium and iodine atoms in each structural phase.

The band structure was calculated for both structural phases and is shown in Figure 2. When the CsPbI₃ structure is in the original cubic phase, we have a direct band gap at transition points R and M of the Brillouin zone (Figure 2a). Unlike conventional semiconductors such as GaAs, which have a dual degeneracy in the valence band maximum (VBM) range, in pure inorganic perovskite semiconductors, band degeneracy occurs in the conduction band minimum (CBM) range. This is a unique feature for these materials, which can be very useful in optoelectronic applications. Most electron transitions in the cubic phase are from the direct transition point R. When the structural phase changes to the orthorhombic, the band gap increases to about 2.50 eV. This is due to the increased interactions of cesium atoms with PbI₆ octahedra within the structure. Due to the change of the band structure pathway, the electron transitions in the orthorhombic phase occur at points X, B, and G. There is dual degeneracy at the G transition point, but at the B and X points, there is a small band splitting in the CBM range.

To determine the contribution of each atomic orbital to the interactions, the partial density of states (PDOS) was calculated for both cubic and orthorhombic phases and shown in Figure 3. From Figure 3a, it is clear that for the cubic phase, the 5p orbital of iodine atoms has the largest contribution to the DOS in the VBM range, and the 6p orbital of lead atoms has the most contribution in the CBM range. The cesium atom also plays a major role in the middle of the conduction band. The PDOS diagram shows that by changing the structural phase from the cubic to orthorhombic, the p orbital of iodine in VBM and the p orbital of the lead atom in CBM have still the most contribution to the DOS (Figure 3b). However, due to the increased interactions between the Cs atom and the PbI₆ inorganic octahedra, the band gap value increases (see Table 1).

To further investigate the structural phase transition of CsPbI₃ as well as the interactions between the inorganic Cs cation and the PbI₆ framework, the electron density was calculated in two and three dimensions, as shown in Figure 4. The ionic radii, atomic radii, Van Der Waals radii, and atomic masses of the atoms Cs, Pb, and I are listed in

Table 2. Ionic radii, atomic radii, Van Der Waals radii, and atomic masses of I, Pb, and Cs.

Atom Name	Ionic Radius (Å)	Atomic Radius (Å)	Van der Waals Radius (Å)	Atomic Mass
I	2.2	1.33	1.98	126.90
Pb	1.19	1.75	2.16	207.20
Cs	1.74	2.72	3.31	132.90

.

According to Figure 4a, in the cubic structural phase, the lead atom is located in the center of the PbI₆ framework and interacts with the cesium cation. From

Table 3. Distance between the atoms inside the CsPbI₃ pure inorganic perovskite.

Cubic Phase				Orthorhomic Phase
Atom Name	I (Å)	Pb (Å)	Cs (Å)	Atom Name	I (Å)	Pb (Å)	Cs (Å)
I	4.53	3.20	4.53	I	4.87	3.10	3.52
Pb	3.20	0	5.60	Pb	3.10	7.84	5.44
Cs	4.53	5.60	6.40	Cs	3.93	5.44	5.97

, it is expected that the cesium atom interacts more with the PbI₆ framework due to its larger interaction radius than the other atoms. However, from Figure 4, it can be seen that the cation Cs has little interaction with the inorganic octahedra. This is because of the type of interactions between the cation Cs and PbI₆, which is of the Van der Waals type. Therefore, Cs interactions do not have a large contribution to the DOS in VBM and CBM ranges, but they play the largest role in the high energy levels of the conduction band. Figure 4a shows that most of the interactions here occur between the lead and iodine atoms.

The distortion index, bond angle variance, and PbI₆ octahedral volume are represented in Table 3 for the two structural phases of CsPbI₃. According to Table 3 and Figure 5, the distortion index and bond angle variance of the structure are zero in the cubic structural phase at high temperatures. This is due to the symmetry of the structure. As shown in Figure 5, as the temperature decreases, octahedra PbI₆ is tilted, and a bond angle variance of 19.0306° and a distortion index of 0.01817 Å are created. In fact, the main reasons for the structural phase transition caused by temperature changes are two important factors: the distortion index and bond angle variance, which affect the PbI₆ inorganic octahedra. From Figure 4b, it can be seen that the PbI₆ octahedra is tilted, which causes some disorders in the distribution of interactions between the CS cation and the PbI₆ framework. According to the two-dimensional electron density (001), it is observed that similar to the cubic phase, the lead cation and iodine anion have the most contribution in the interactions. According to Figure 5 and Table 3, it is found that with changing the structural phase of CsPbI₃ from cubic to orthorhombic, the PbI₆ volume increases. The distance between the atoms comprising the structure is given in

Table 4. Volume, distortion index, and bond angle variance of PbI₆−Octahedral within the CsPbI₃ structure.

Component	Distortion Index (Å)	Bond Angle Variance (deg2)	Volume (Å3)
CsPbI3−Cubic	0	0	43.82
CsPbI3−Orthorhombic	0.01817	19.306	46.25

for both phases. However, this structural phase transition has increased the interaction of CS cation with PbI₆ octahedra. This can be related to the increased volume and consequently the increased interaction radius of the Pb-I framework. From Table 4, it is evident that increasing the interaction radius of the Pb-I octahedra leads to a decrease in the distance between the cesium cation and the Pb-I framework. Therefore, the Van der Waals-type interactions between the cation Cs and the PbI₆ and consequently the band gap of the CsPbI₃ inorganic perovskite increase.

To investigate the optical properties of the CsPbI₃ structure, the real and imaginary parts of the dielectric function were calculated for both structural phases and shown in Figure 6. The computed static dielectric constant is reported in

Table 5. Static dielectric constant of CsPbI₃ for the cubic and orthorhombic phases.

Perovskite Structures	${\mathit{\epsilon }}_0$
CsPbI3−Cubic	7.58
CsPbI3−Orthorhombic	3.42

. As mentioned in the previous section, the band gap of the CsPbI₃ structure increases following the structural phase transition caused by a temperature change. In turn, this increase in the band gap value reduces the static dielectric constant value and also causes a blue shift in the first peak of the imaginary part of the dielectric function, which is also the location of the first electron transition. Indeed, this is due to the increased interactions between the cesium cation and inorganic PbI₆ octahedra. To confirm the accuracy of the static dielectric constant calculations, the ε(iω) spectrum was evaluated for both structural phases and shown in Figure 6b. It is clear that the starting point of this spectrum actually corresponds to the static dielectric constant. By changing the structural phase from cube to orthorhombic, this point drops to 3.42. According to the spectrum of electron energy loss, it is observed that at the location of the first peak, there is the least amount of electron energy loss, which is strong confirmation for the results obtained for the dielectric function.

Absorption and refractive spectra were also calculated from the dielectric function for both structural phases of CsPbI₃ and represented in Figure 7. The values obtained for the wavelength of the absorption edge and refraction index are given in

Table 6. Wavelength of absorption edge and refraction index of CsPbI₃ structure for the cubic and orthorhombic phases.

Perovskite Structures	n0	α (nm)
CsPbI3−Cubic	2.75	779
CsPbI3−Orthorhombic	1.82	506

. As can be seen, the structural phase transition of pure inorganic CsPbI₃ perovskite causes a decrease in the absorption edge wavelength and also in the refractive index. The origin of these reductions in the optical parameters is the changes in the volume, distortion index, and bond angle variance of inorganic Pb-I octahedra, which affects the distribution of cesium cation interactions with the Pb-I framework.

4. Conclusions

The present first-principles theoretical study of pure CsPbI₃ inorganic perovskite at two temperatures of 300 °C and 25 °C revealed that a change in the structure of Pb6 inorganic octahedra substantially influences the electronic properties of CsPbI₃. Lowering the temperature causes a change in the volume, distortion index, and bond angle variance of the PbI₆, which in turn changes the structural phase of the inorganic perovskite. The tilted inorganic octahedra with an increased volume gives rise to the Van der Waals interactions between the cesium cation and PbI₆ framework. This increases the band gap value and causes a blue shift in the wavelength of absorption edge. Investigation of the effect of temperature change on the structural, electronic, and optical properties of hybrid inorganic perovskite is essential for a better understanding of these unrivaled materials.