Operator-Based Fractional-Order Nonlinear Robust Control for the Spiral Heat Exchanger Identified by Particle Swarm Optimization ^†

Abstract

Fractional-order calculus and derivative is extended from integral-order calculus and derivative. This paper investigates a nonlinear robust control problem using fractional order and operator theory. In order to improve the tracking performance and antidisturbance ability, operator- and fractional-order-based nonlinear robust control for the spiral counter-flow heat exchanger described by the parallel fractional-order model (PFOM) is proposed. The parallel fractional-order model for the spiral counter-flow heat exchanger was identified by particle swarm optimization (PSO) and the parameters of a fractional-order PID (FOPID) controller were optimized by the PSO. First, the parallel fractional-order mathematical model for a spiral counter-flow heat exchanger plant was identified by PSO. Second, a fractional-order PID controller and operator controller for the spiral heat exchanger were designed under the identified parallel fractional-order mathematical model. Third, the parameters of the operator and fractional-order PID were optimized by PSO. Then, tracking and antidisturbance performance of the control system were analyzed. Finally, comparisons of two control schemes were performed, and the effectiveness illustrated.

1. Introduction

The first appearance of fractional-order calculus was in a letter from Leibniz in 1695 which expanded integral order calculus. However, fractional-order calculus has attracted great interest in the last several decades or so [1]. Some researchers have found that it allows them to present a real object more accurately than the conventional integer-order derivative and calculus in some fields such as the voltage–current relation of a semi-infinite lossy transmission line [2], economic systems [3], electric power systems [4], thermal systems [5], and the diffusion of heat through a semi-infinite solid, where heat flow is equal to the half-derivative of the temperature [6].

In real industries, proportional–integral–derivative(PID) control is considered to be the best control in the control system family for good control performance and robustness in linear or approximately linear systems [7]. By calculating and controlling three parameters—the proportional, integral, and derivative of how much a process variable deviates from the desired set point value—we can achieve different control actions for specific work. Therefore, the tuning of a PID controller’s parameters is very important. Fractional-order PID (FOPID) control extended from the conventional PID control is extensively used by many scientists in order to achieve the most robust performance in control systems. The fractional PID controller was introduced by Podlubny, I. in 1994 for a fractional-order system [6]. A FOPID controller has five parameters to tune. Thus, it is more flexible than a classical PID controller [8]. However, it is more difficult to tune. In references [9,10,11,12], different designs and tuning methods were presented. In this paper, different design methods along with available software tools and tuning methods are described. Basic definitions of fractional order are introduced with their use in control systems (or in engineering applications).

A spiral heat exchanger is widely used because it needs a smaller space for installation than traditional heat exchanger solutions and it has excellent heat transfer efficiency [13,14,15]. However, the spiral heat exchanger is a nonlinear system with uncertainties influenced by the input temperatures and flow rates of cold-fluid side and hot-fluid side, and other factors [16]. Therefore, it is difficult to control for the heated or cooled output temperature in variable working conditions.

Nonlinear robust control has been considered by many researchers in different methods in different fields [17,18,19]. In reference [20,21,22], the coprime right factorization was considered to compensate the nonlinearity of the system and the robust control performance of the system was improved. The coprime factorization is suited to not only linear feedback control but also nonlinear feedback control. It provides a convenient approach to study the input–output stability of nonlinear feedback control systems. The papers [23,24,25,26] studied the robust right coprime factorization of a nonlinear system with perturbations. A spiral heat exchanger mathematical model is described by integer differential equations in traditional methods. Reference [5] demonstrated a spiral heat exchanger mathematical model constructed by fractional differential equations was more accurate than traditional integer differential equations. In reference [27], Operator Based Fractional Order Control System for a spiral heat exchanger with uncertainties is proposed. However, the order of the fractional-order derivative was difficult to determine by a theoretical method, so the parameters of the fractional-order model were determined by a testing method. Therefore, motivated by the previous references, in order to improve its tracking performance and antidisturbance ability, an operator- and fractional-order-based nonlinear robust control for the spiral counter-flow heat exchanger described by a parallel fractional-order model (PFOM) is proposed. The parallel fractional-order model for the spiral counter-flow heat exchanger is identified by particle optimization (PSO) and the parameters of the fractional-order PID (FOPID) controller are optimized by PSO. A fractional-order PID controller and operator controller for the spiral heat exchanger are designed under the identified parallel fractional-order model. Comparisons of two control schemes are performed, and the effectiveness is illustrated.

The remainder of this paper is structured as follows. In Section 2, preliminaries and problem statement are presented. Section 3 introduces the parallel fractional-order model for the spiral counter-flow heat exchanger. The parallel fractional-order model for the spiral counter-flow heat exchanger is identified by PSO, with the least squares method as the performance index. In Section 4, operator- and fractional-order-based nonlinear robust control for the spiral counter-flow heat exchanger described by the identified parallel fractional-order model is proposed. Then, the parallel fractional-order operator controller and compensator are designed, with the compensator aiming to reduce disturbance. Section 5 optimizes the parameters of the fractional-order PID controller by PSO. Comparisons of two control schemes are performed to analyze the tracking and antidisturbance performance for the spiral counter-flow heat exchanger, and the effectiveness is verified in Section 6. Finally, a conclusion is given.

2. Preliminaries and Problem Statement

In this section, several definitions of fractional-order calculus, operator theory, and the modified swarm optimization are introduced.

2.1. The Definitions of Fractional Order

2.1.1. The Caputo Definition of Fractional Order

$$\begin{array}{c}\hfill {}_a^C{D}_t^{q}y\left(t\right)=\frac{1}{\mathsf{\Gamma }(n-q)}{\int }_a^t{\displaystyle \frac{y^{\left(n\right)}\left(t\right)}{(t-\tau )}}d\tau ,n-1\le q\le n\end{array}$$

(1)

where $\mathsf{\Gamma }(·)$ is Gamma function defined by $\mathsf{\Gamma }\left(ϵ\right)={\int }_0^{\infty }{e}^{-t}{t}^{ϵ-1}$ and n is a positive integer number.

2.1.2. Grunwald–Letnikov’s Definition of Fractional Order

$$\begin{array}{c}\hfill {}_a^{GL}{D}_t^{q}y\left(t\right)=\underset{h\to 0}{lim}{h}^{-q}\sum _{j=0}^{\left[{\displaystyle \frac{t-a}{h}}\right]}{(-1)}^j\frac{\mathsf{\Gamma }(q+1)}{\mathsf{\Gamma }(j+1)\mathsf{\Gamma }(q-j+1)}\\ \hfill y(t-jh)\end{array}$$

(2)

where $[·]$ means the integer part. (1) and (2) are equivalent if y( ·) is differentiable. Grunwald–Letnikov’s definition of fractional order is a discrete form of the fractional order definition, which is implemented easily on a CPU. Therefore, Grunwald–Letnikov’s fractional order was used in this paper.

2.2. Particle Swarm Optimization

Particle swarm optimization (PSO) is a population-based stochastic optimization technique developed by Eberhart and Kennedy in 1995, inspired by the social behavior of bird flocking or fish schooling [28]. PSO shares many similarities with evolutionary computation techniques such as genetic algorithms (GA) and ant colony optimization (ACO) algorithms [29]. The system is initialized with a population of random solutions and searches for optima by updating iterations. However, unlike GA, PSO has no evolution operators such as crossover and mutation [30]. In PSO, potential solutions, called particles, fly through the problem space by following the current optimum particles.

It has been successfully applied to many problems in several fields such as biomedicine and energy conversion. Image analysis is one of the most frequent applications and it has been performed on biomedical images, microwave imaging, among others.

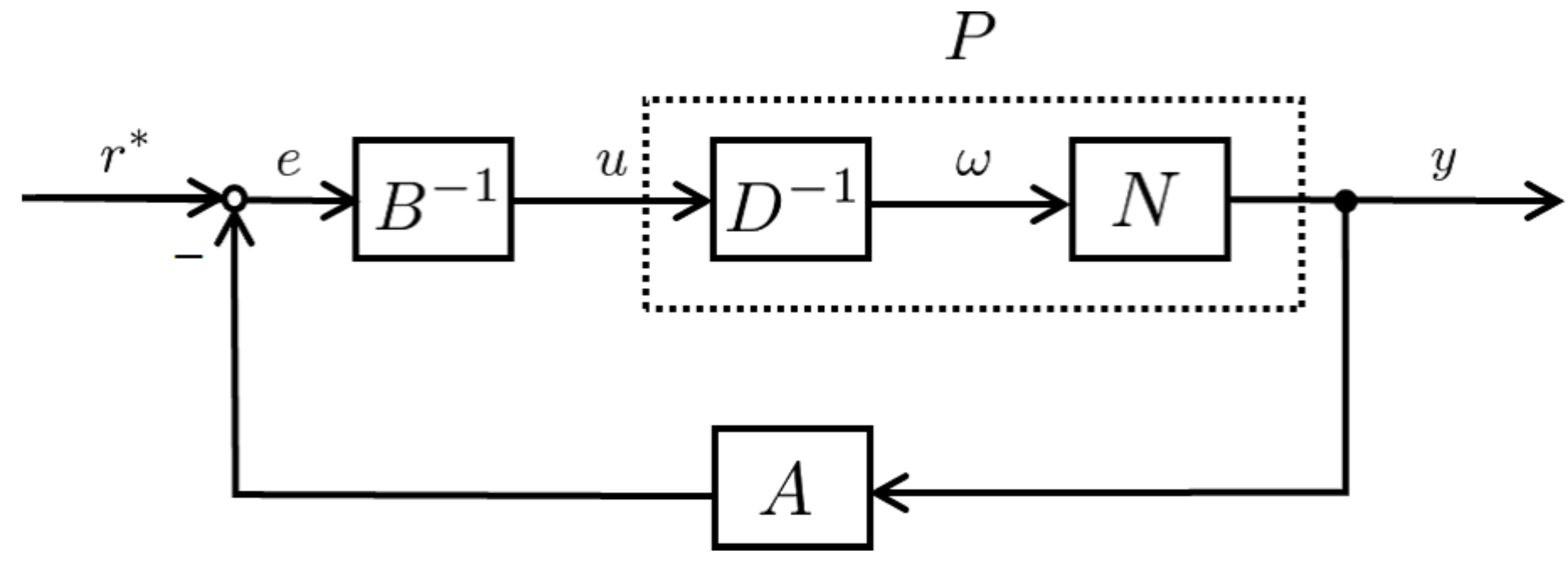
2.3. Operator Theory

In this section, operator theory is described. The robust stability analysis can be performed even with uncertainties that are difficult to model in mathematics. Here, a nonlinear control system with uncertainties can be designed in the time domain by operator theory and does not convert into the frequency domain by using a transfer function. A nonlinear feedback system based on operator theory is shown in Figure 1.

2.3.1. Right Factorization

Let the plant be the operator $P:U\to Y:y\left(t\right)=P\left(u\left(t\right)\right)$. If spaces U and Y of the input and output signals are two different extended linear spaces, plant P is unstable. A stable operator $N:w\left(t\right)\in W\to y\left(t\right)\in Y$ and stable operator $D:w\left(t\right)\in W\to u\left(t\right)\in U$ can be invertible. At this time, plant P is said to have a right factorization of N, $D^{-1}$.

$$\begin{array}{c}\hfill P=N{D}^{-1}\end{array}$$

(3)

2.3.2. Right Coprime Factorization

Suppose there is a right factorization operator N,D in plant P. The Bezout equation is obtained as follows.

$$\begin{array}{c}\hfill AN+BD=M,\forall M\in \mathcal{U}\left(W,U\right)\end{array}$$

(4)

If a stable operator A and a stable and invertible operator B satisfies the above Bezout Equation (4), then plant P is said to have a right coprime factorization of A, B, N, and D as shown in Figure 2. The stability of the control system can be guaranteed.

2.3.3. Robust Right Coprime Factorization

In general, there are many uncertainties in an actual nonlinear control system that are difficult to express in a mathematical model. So, the stability of the nonlinear control system with uncertainties is no longer guaranteed. Therefore, by constructing a nonlinear feedback system with robust right coprime factorization, it is possible to analyze robust stability with uncertainties. The nonlinear feedback system with uncertainties is shown in Figure 2. Plant P without uncertainties is the nominal plant, and the actual plant with uncertainties $\mathsf{\Delta }P$ is $\tilde{P}=P+\mathsf{\Delta }P$.

$$\begin{array}{c}\hfill \tilde{P}=P+\mathsf{\Delta }P=\left(N+\mathsf{\Delta }N\right)D^{-1}\end{array}$$

(5)

$$\begin{array}{c}\hfill A\left(N+\mathsf{\Delta }N\right)+BD=\tilde{M}\end{array}$$

(6)

$$\begin{array}{c}\hfill A\left(N+\mathsf{\Delta }N\right)+BD=AN+BD=M\end{array}$$

(7)

$$\begin{array}{c}\hfill \parallel \left(A\left(N+\mathsf{\Delta }N\right)-AN\right)M^{-1}{\parallel }_{Lip}<1\end{array}$$

(8)

If the following inequality (8) is satisfied, $\tilde{M}$ is unimodular. In other words, the nonlinear feedback system with uncertainties can be said to have robust stability only if (8) is satisfied.

2.4. Statement Problem

A spiral heat exchanger is a nonlinear system with uncertainties. It is used widely in industry. In real application, the heated or cooled output temperature should track a timely reference input temperature in variable load conditions. However, the output temperature of the spiral heat exchanger is difficult to control for nonlinearity, uncertainties, and many disturbances. In conventional control methods, a PID controller is used to derive a good control performance in slow or no disturbances. In real application, there are many disturbances for the spiral heat exchanger, such as input temperature and flow rate on the hot-fluid side, input temperature on the cold-fluid side. Thus, due to the disturbances, control performance is poor in conventional control methods.

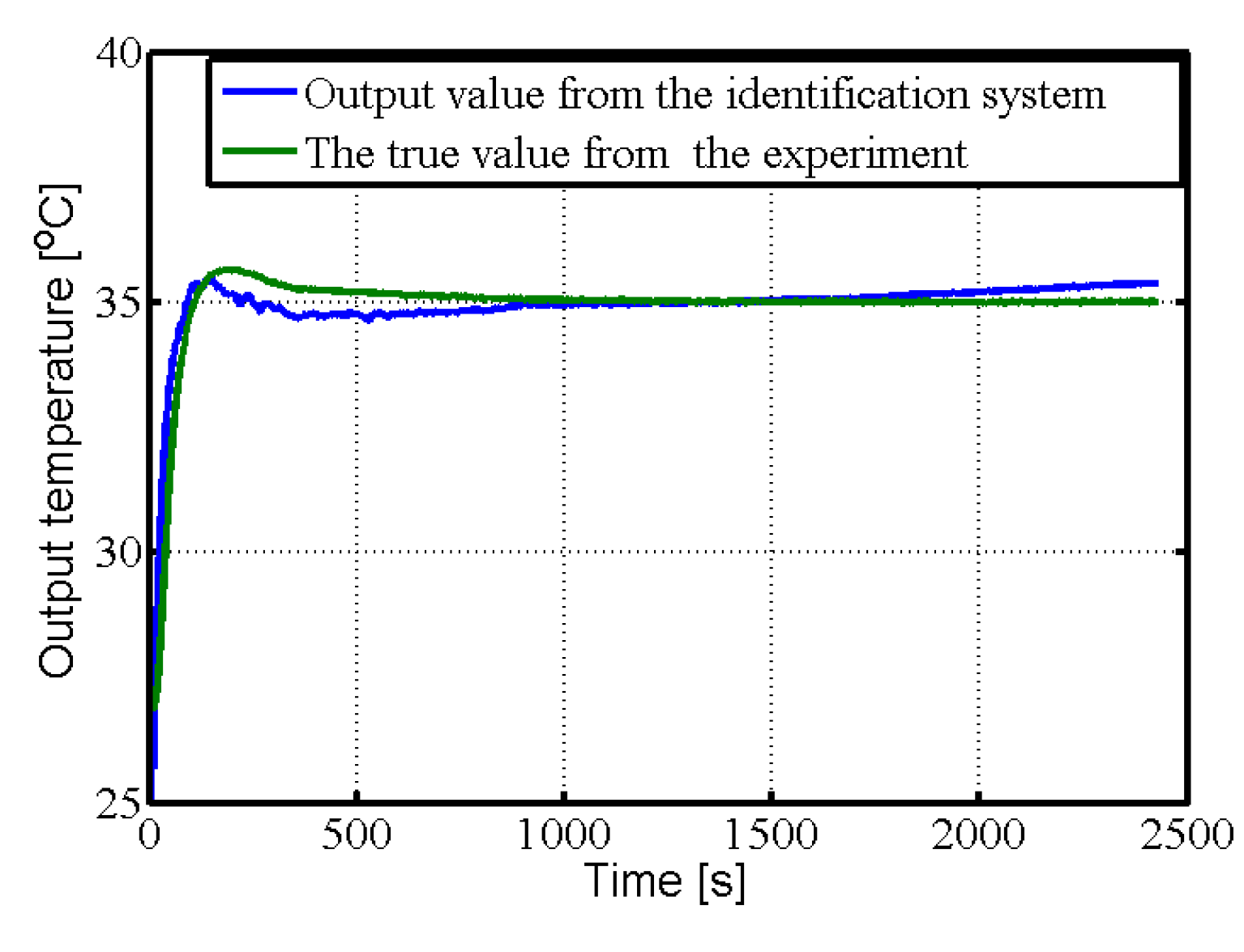
3. Fractional-Order System Identification for a Spiral Heat Exchanger by PSO

3.1. Parallel Fractional-Order Model for the Spiral Counter-Flow Heat Exchanger

3.1.1. Fractional-Order Model for the Spiral Counter-Flow Heat Exchanger

According to the law of heat energy balance of two fluids, the fractional-order derivative model for the spiral-plate counter-flow heat exchanger (See Appendix A) is derived as follows [31].

$$\begin{array}{c}\hfill \left\{\begin{array}{cc}\hfill & \hfill D_{\theta }^{q_1}{T}_h(\theta ,t)={\displaystyle \frac{(k+\mathsf{\Delta }k)FAZ}{Q{L}_1{c}_h{\rho }_h}}(T_c(\theta ,t)-T_h(\theta ,t)\hfill \\ \hfill & \hfill D_{\theta }^{q_2}{T}_c(\theta ,t)={\displaystyle \frac{(k+\mathsf{\Delta }k)FAZ}{Q{L}_2{c}_c{\rho }_c}}(T_h(\theta ,t)-T_c(\theta ,t)\hfill \\ \hfill & \hfill \theta \in [0,11\pi ]\hfill \end{array}\right.\end{array}$$

(9)

where $k=\frac{{\delta }_s}{\lambda }$, $k+\mathsf{\Delta }k=\frac{1}{h_h}+\frac{{\delta }_s}{\lambda }+\frac{1}{h_c}$, $k_c$ = $c_c{\rho }_c$, $k_h$ = $c_h{\rho }_h$, and $\mathsf{\Delta }T(\theta ,t)$ = $T_c(\theta ,t)-T_h(\theta ,t)$. $A=\sqrt{a^2+b^2}$, and $A+\mathsf{\Delta }A$= $\sqrt{a^2+{(b+a*11\pi )}^2}$ $F\in [1,2]$, which is related to the plant of the spiral-plate heat exchanger. ${\rho }_c,{\rho }_h$ are the densities of the cold fluid and the hot fluid, respectively. $c_c,c_h$ are the specific heat capacities of the cold fluid and the hot fluid, respectively.

3.1.2. Parallel Fractional-Order Model for the Spiral Counter-Flow Heat Exchanger

The parallel fractional-order model for the spiral-plate counter-flow heat exchanger is described as follows.

$$\begin{array}{c}\hfill \left\{\begin{array}{c}\hfill T_{hk}={\left(\mathsf{\Delta }\theta \right)}^{q_1}{D}_{hfrac}+B_h{T}_{hk-1}\hfill \\ \hfill T_{ck}={\left(\mathsf{\Delta }\theta \right)}^{q_2}{D}_{cfrac}+B_c{T}_{ck-1}\hfill \end{array}\right.\end{array}$$

(10)

where $T_{hk},T_{hk-1},D_{hfrac}\in R^N$, $B_h\in R^{N\times N}$, $T_{ck},T_{ck-1},D_{cfrac}\in R^N$, and $B_c\in R^{N\times N}$

$$\begin{array}{c}\hfill T_{hk}=\left(\begin{array}{c}{T}_h\left(\mathsf{\Delta }\theta \right)\\ T_h\left(2\left(\mathsf{\Delta }\theta \right)\right)\\ ⋮\\ T_h\left(N\left(\mathsf{\Delta }\theta \right)\right)\end{array}\right)\end{array}$$

(11)

$$\begin{array}{c}\hfill B_h=\left(\begin{array}{cccc}\frac{-\mathsf{\Gamma }(q_1+1)}{\mathsf{\Gamma }\left(2\right)\mathsf{\Gamma }\left(q_1\right)}& 0& \dots & 0\\ \frac{\mathsf{\Gamma }(q_1+1)}{\mathsf{\Gamma }\left(3\right)\mathsf{\Gamma }(q_1-1)}& \frac{-\mathsf{\Gamma }(q_1+1)}{\mathsf{\Gamma }\left(2\right)\mathsf{\Gamma }\left(q_1\right)}& \dots & 0\\ ⋮& ⋮& ⋮& ⋮\\ \frac{{(-1)}^N\mathsf{\Gamma }(q_1+1)}{\mathsf{\Gamma }(N+1)\mathsf{\Gamma }(q_1-N+1)}& \frac{{(-1)}^{(N-1)}\mathsf{\Gamma }(q_1+1)}{\mathsf{\Gamma }\left(N\right)\mathsf{\Gamma }((q_1-N+1)}& \dots & \frac{-\mathsf{\Gamma }(q_1+1)}{\mathsf{\Gamma }\left(2\right)\mathsf{\Gamma }\left(q_1\right)}\end{array}\right)\end{array}$$

(12)

$$\begin{array}{c}\hfill T_{hk-1}=\left(\begin{array}{c}{T}_h\left(0\right)\\ T_h\left(\mathsf{\Delta }\theta \right)\\ ⋮\\ T_h\left((N-1)\left(\mathsf{\Delta }\theta \right)\right)\end{array}\right)\end{array}$$

(13)

$$\begin{array}{c}\hfill B_c=\left(\begin{array}{cccc}\frac{-\mathsf{\Gamma }(q_2+1)}{\mathsf{\Gamma }\left(2\right)\mathsf{\Gamma }\left(q_2\right)}& 0& \dots & 0\\ \frac{\mathsf{\Gamma }(q_2+1)}{\mathsf{\Gamma }\left(3\right)\mathsf{\Gamma }(q_2-1)}& \frac{-\mathsf{\Gamma }(q_2+1)}{\mathsf{\Gamma }\left(2\right)\mathsf{\Gamma }\left(q_2\right)}& \dots & 0\\ ⋮& ⋮& ⋮& ⋮\\ \frac{{(-1)}^N\mathsf{\Gamma }(q_2+1)}{\mathsf{\Gamma }(N+1)\mathsf{\Gamma }(q_2-N+1)}& \frac{{(-1)}^{(N-1)}\mathsf{\Gamma }(q_2+1)}{\mathsf{\Gamma }\left(N\right)\mathsf{\Gamma }((q_2-N+1)}& \dots & \frac{-\mathsf{\Gamma }(q_2+1)}{\mathsf{\Gamma }\left(2\right)\mathsf{\Gamma }\left(q_2\right)}\end{array}\right)\end{array}$$

(14)

$$\begin{array}{c}\hfill T_{ck}=\left(\begin{array}{c}{T}_c\left(\mathsf{\Delta }\theta \right)\\ T_c\left(2\mathsf{\Delta }\theta \right)\\ ⋮\\ T_c\left(N\left(\mathsf{\Delta }\theta \right)\right)\end{array}\right)\end{array}$$

(15)

$$\begin{array}{c}\hfill T_{ck-1}=\left(\begin{array}{c}{T}_c\left(0\right)\\ T_c\left(\mathsf{\Delta }\theta \right)\\ ⋮\\ T_c\left((N-1)\left(\mathsf{\Delta }\theta \right)\right)\end{array}\right)\end{array}$$

(16)

Therefore, the parallel fractional-order derivative model for the spiral-plate counter-flow heat exchanger is obtained.

$$\begin{array}{c}\hfill \left\{\begin{array}{c}\hfill T_{hk}={\left(\mathsf{\Delta }\theta \right)}^{q_1}{\displaystyle \frac{FkZA}{Q{L}_1{c}_h{\rho }_h}}(H{T}_{cK-1}-T_{hK-1})+B_h{T}_{hk-1}\hfill \\ \hfill T_{ck}={\left(\mathsf{\Delta }\theta \right)}^{q_2}{\displaystyle \frac{FkZA}{Q{L}_2{c}_c{\rho }_c}}(H{T}_{hK-1}-T_{cK-1})+B_c{T}_{ck-1}\hfill \\ \hfill T_{cout}=C{T}_{ck}\hfill \end{array}\right.\end{array}$$

(17)

where

$$\begin{array}{c}\hfill H=\left(\begin{array}{cccccc}0& 0& 0& \dots & 0& 1\\ 0& 0& 0& \dots & 1& 0\\ ⋮& ⋮& ⋮& ⋮& ⋮& ⋮\\ 1& 0& 0& \dots & 0& 0\end{array}\right)\end{array}$$

(18)

$$\begin{array}{c}\hfill C=\left(\begin{array}{cccccc}0& 0& 0& 0& \dots & 1\end{array}\right)\end{array}$$

(19)

where $C\in R^{1\times N}$ and $H\in R^{N\times N}.$ The parallel fractional-order model for the spiral counter-flow heat exchanger is a model with parallel input data.

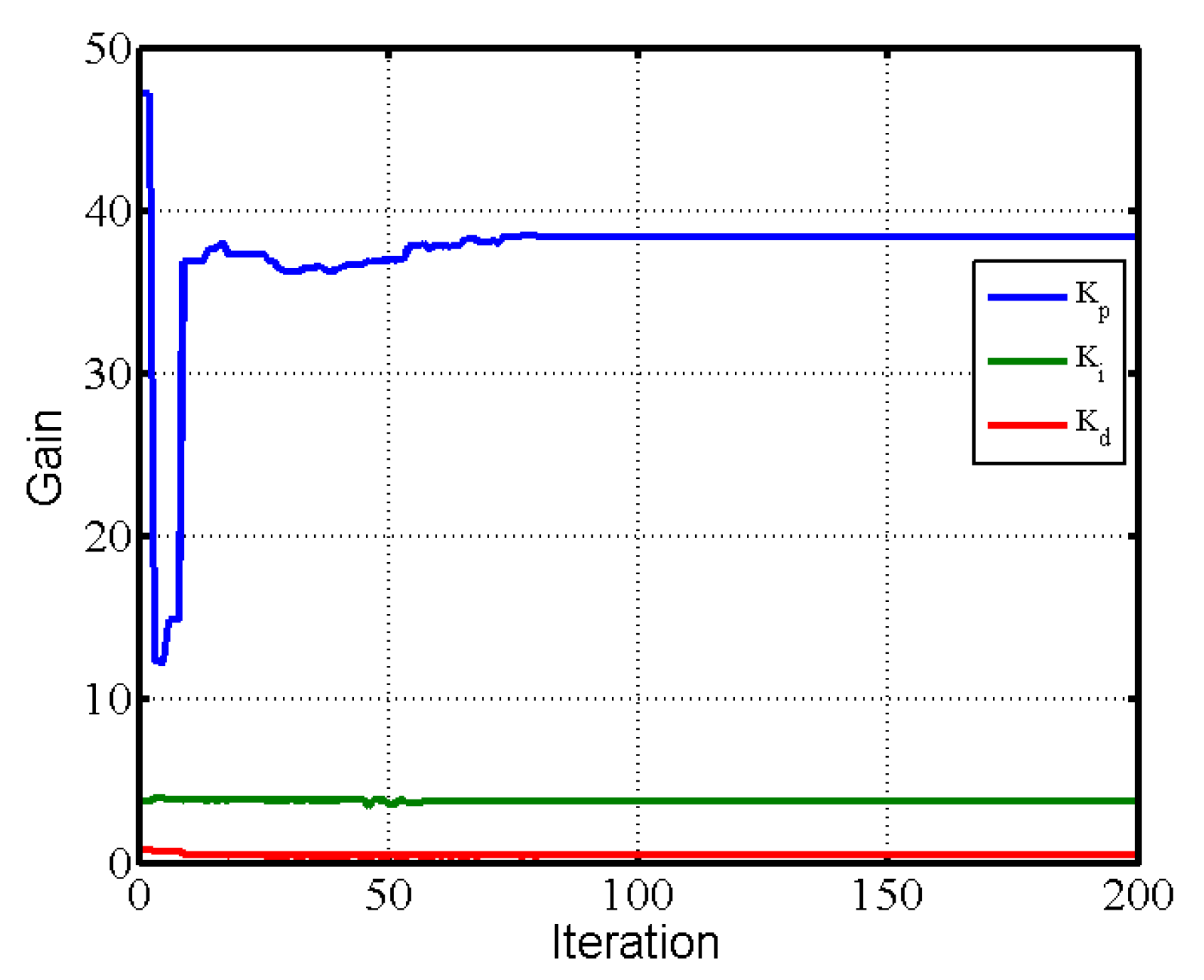
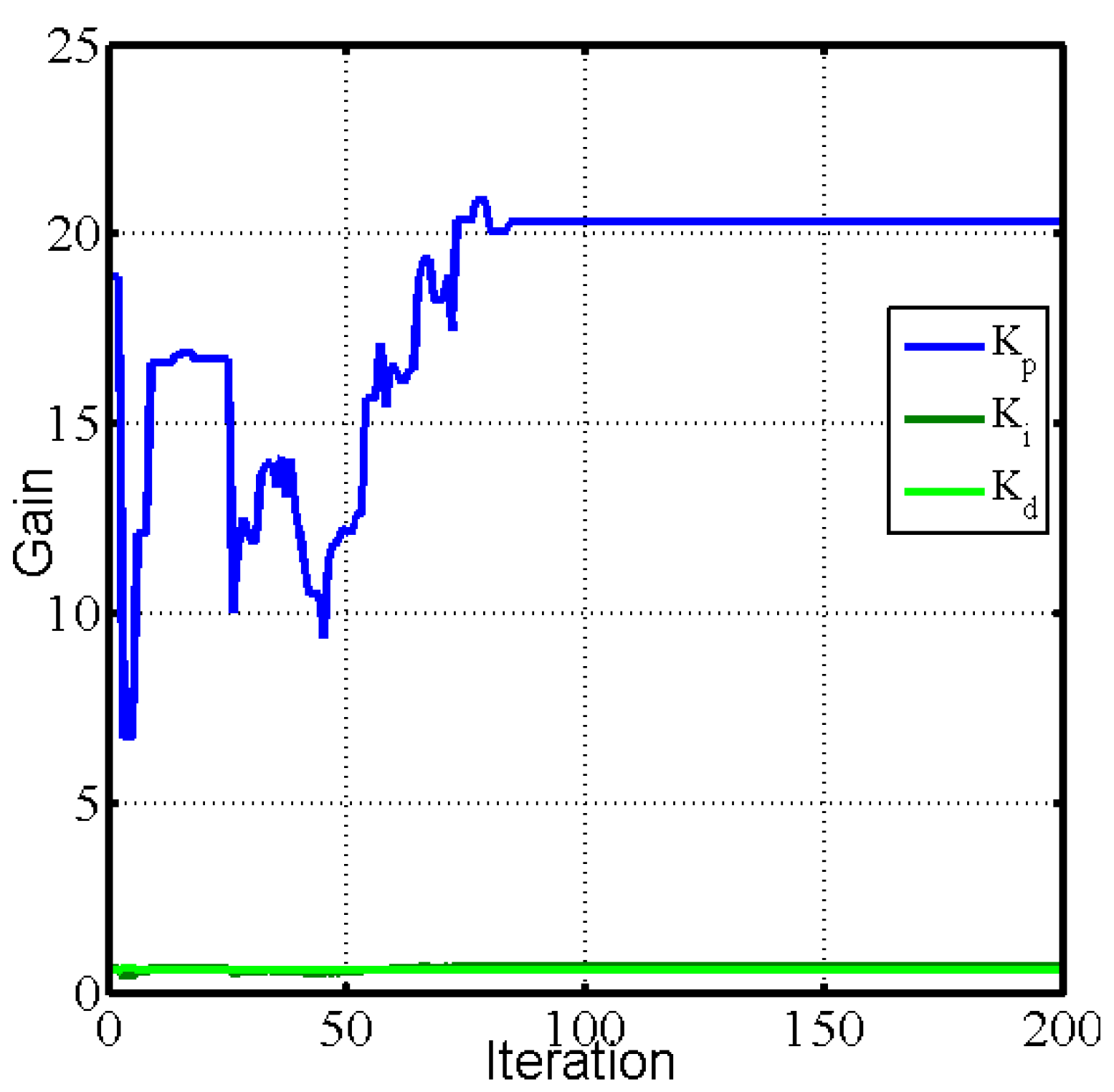
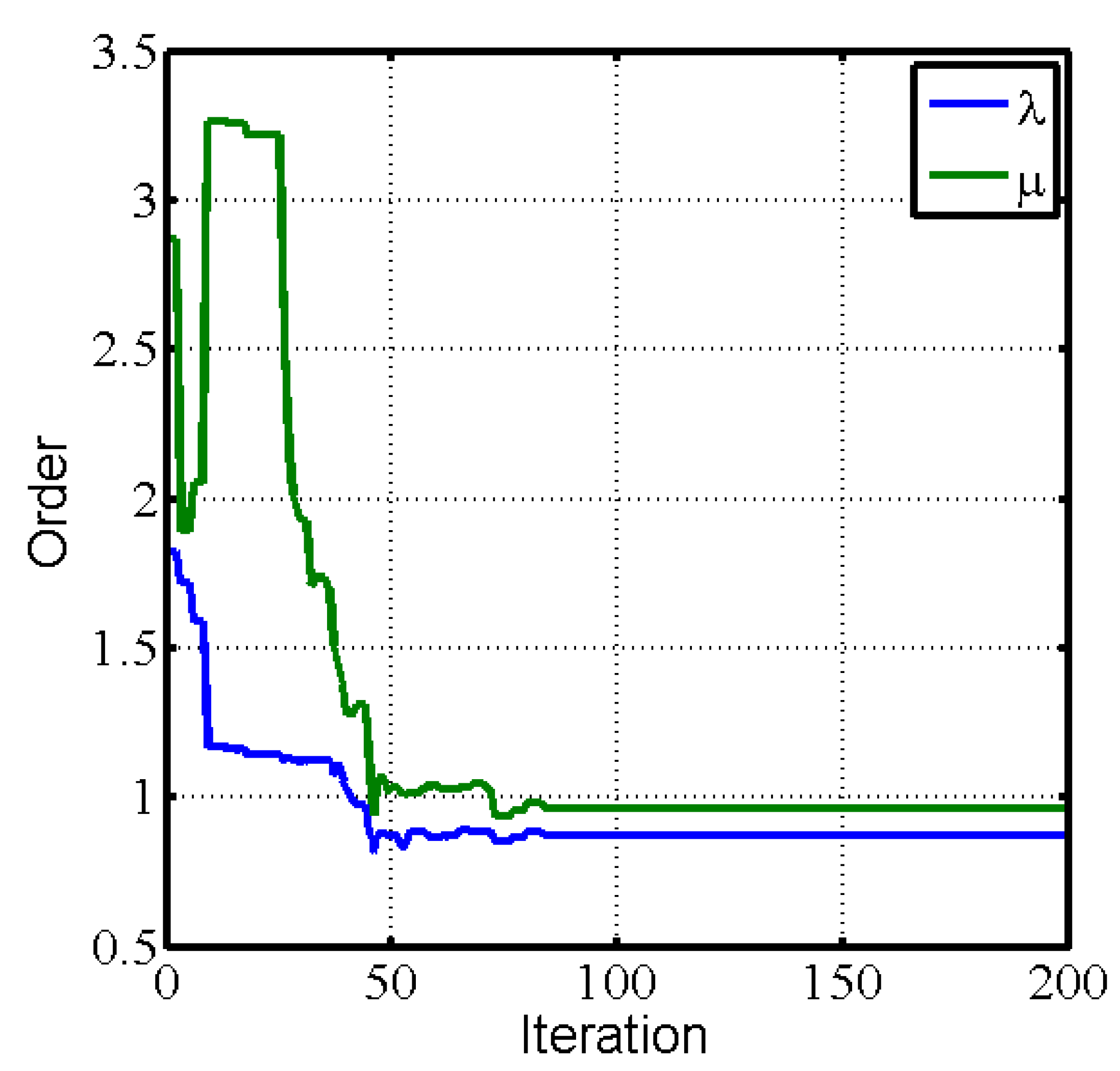
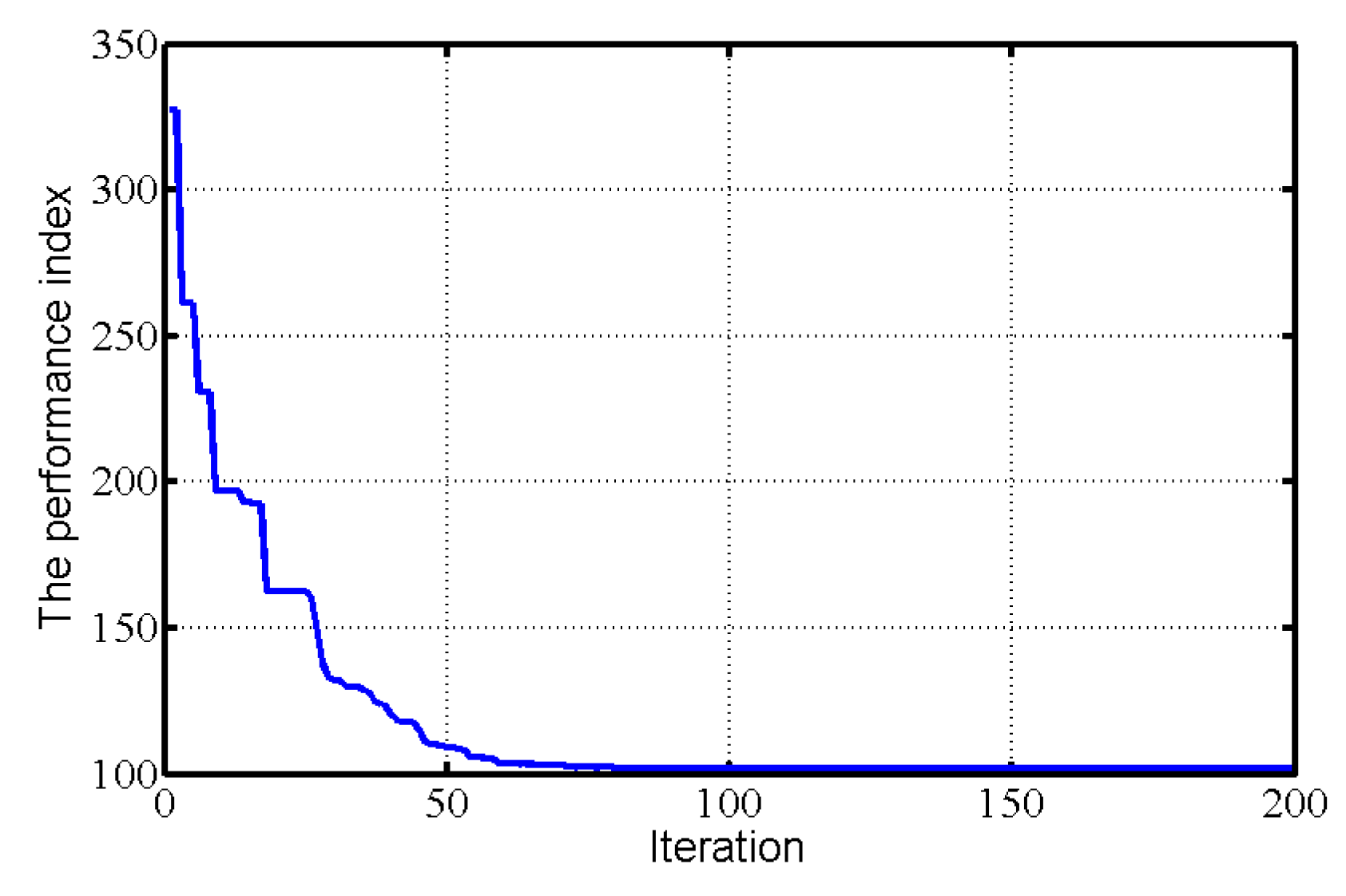
3.2. Parallel Fractional-Order Model Identification for the Spiral Heat Exchanger

The schematic block of the parameter estimation for a parallel fractional-order model of the spiral counter-flow heat exchanger is shown in Figure 3.

The specific parameter estimation steps are follows.

(1)

Determine the searched spaces for the estimated model.

Let $K_h$ = ${\displaystyle \frac{FkZA}{c_h{\rho }_h}}$, $K_c$ = ${\displaystyle \frac{FkZA}{c_c{\rho }_c}}$, then the parallel fractional-order model for the spiral counter-flow heat exchanger derived from (17) is the model’s identified object as follows.

$$\begin{array}{c}\hfill \left\{\begin{array}{c}\hfill T_{hk}={\left(\mathsf{\Delta }\theta \right)}^{q_1}{\displaystyle \frac{K_h}{Q{L}_1}}(H{T}_{cK-1}-T_{hK-1})+B_h{T}_{hk-1}\hfill \\ \hfill T_{ck}={\left(\mathsf{\Delta }\theta \right)}^{q_2}{\displaystyle \frac{K_c}{Q{L}_2}}(H{T}_{hK-1}-T_{cK-1})+B_c{T}_{ck-1}\hfill \\ \hfill T_{cout}=C{T}_{ck}\hfill \end{array}\right.\end{array}$$

(20)

The researched space vector is defined as

$$\begin{array}{c}\hfill x=\{q_1,q_2,K_h,K_c\}\end{array}$$

(21)

where $K_h$, $K_c$ are the model parameters and $q_1$, $q_2$ are the orders of the model. The range of the model parameter ($K_h$, $K_c$) was set to be [0 10], and the range of the model parameter ($q_1$, $q_2$) was set to be [0 2].

(2)

Determine the performance index (evaluation function).

In this paper, the input flow rate of the hot-fluid side $Q{L}_1$, the input flow rate of the cold-fluid side $Q{L}_2$, the input temperature of the cold-fluid side $T_{cin}$, and the input temperature of the hot-fluid side $T_{hin}$ were introduced as input signals of identified object. The sum of the squared errors between the output ${\widehat{T}}_{cout}$ and the true value ${\widehat{T}}_{cout}$,

$$\begin{array}{c}\hfill J={\int }_0^T{[T_{cout}\left(t\right)-{\widehat{T}}_{cout}\left(t\right)]}^{2}dt\end{array}$$

(22)

was used as the performance evaluation function.

(3)

The parameters of the algorithm are initialized to generate random search vectors.

(4)

According to the steps of the PSO algorithm, the parameters in the parallel fractional-order model are identified.

(5)

The iteration is repeated until the performance index is satisfactory or the sum of iterations go up to a maximum of 200.

The evolutionary curves of the estimated parameters are plotted in Figure 4, Figure 5, Figure 6 and Figure 7.

The evolutionary curve of the performance index is plotted in Figure 8.

The comparison of the output value from the identification system and the true value from the experiment is shown in Figure 9.

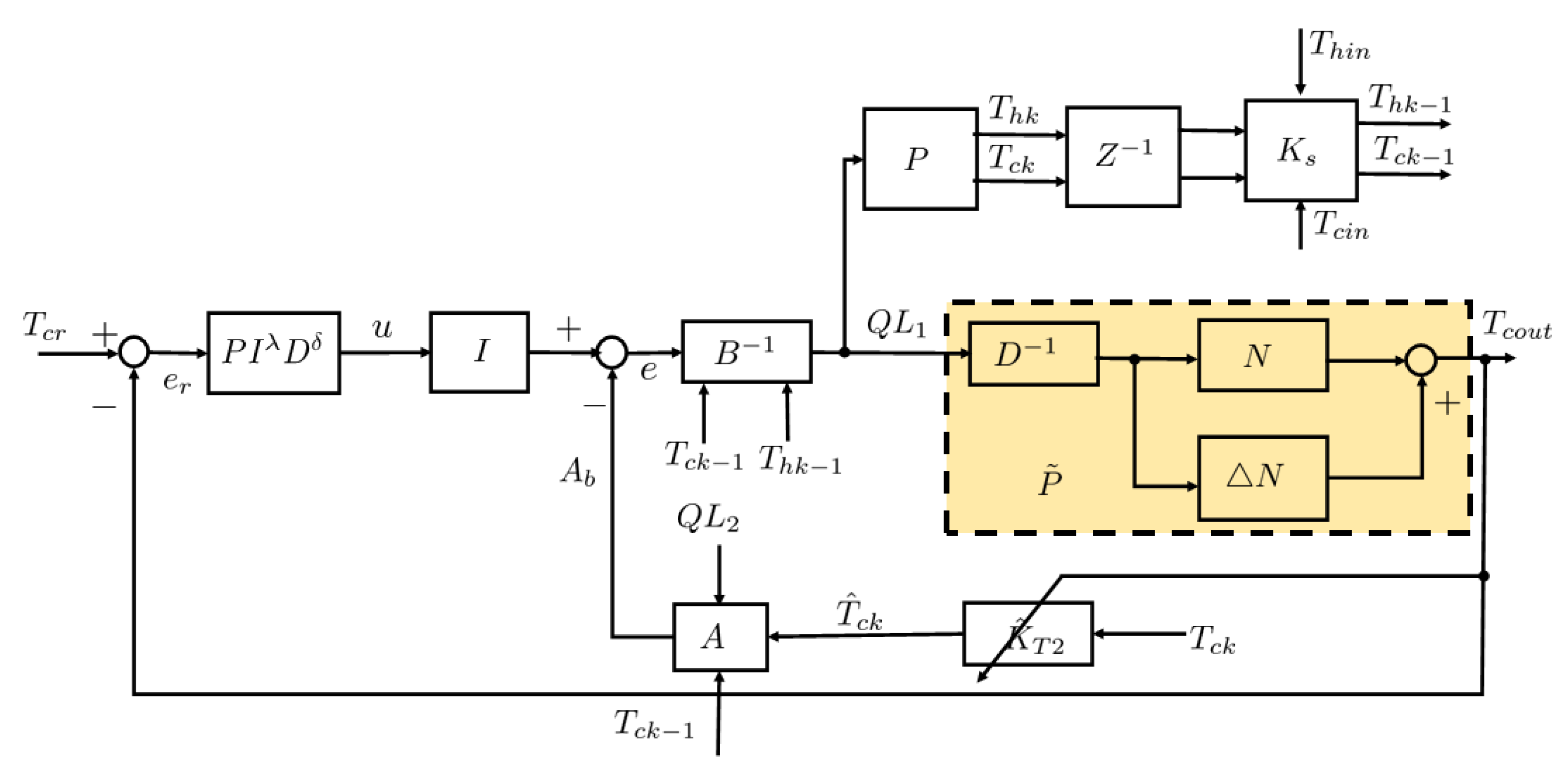
4. Operator-Based Fractional-Order PID Nonlinear Robust Control for the Spiral Heat Exchanger

In this section, two control schemes were designed in order to verify the tracking and antidisturbance performance of the operator-based fractional-order PID nonlinear robust control of the spiral heat exchanger, as shown Figure 10 and Figure 11.

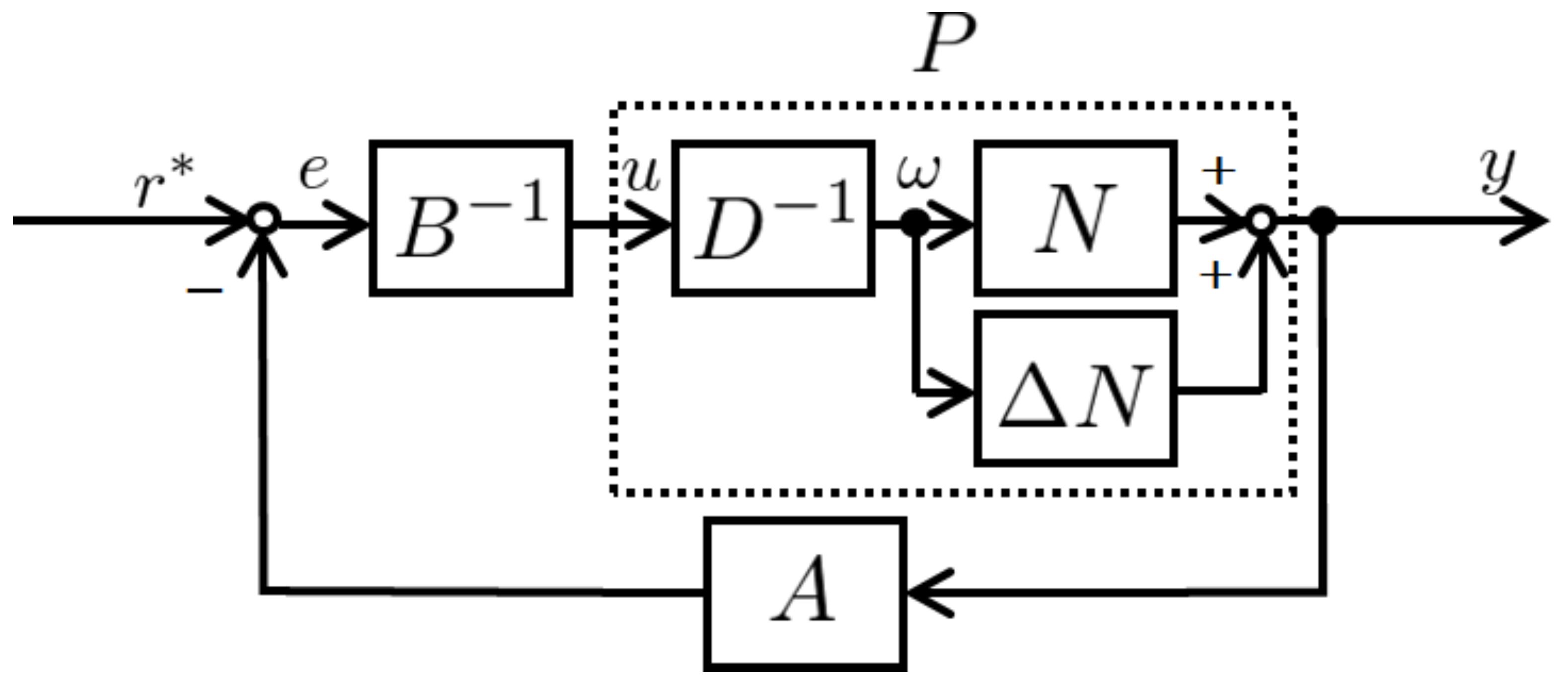
4.1. Parallel Fractional-Order Model for the Spiral Heat Exchanger with Uncertainties

The heat transfer coefficient of the spiral heat exchanger plant $\tilde{k}$ is a relationship between not only the flow velocity of water, but also others. We know that the spiral heat exchanger model is nonlinear. Therefore, the spiral heat exchanger model is nonlinear system with uncertainties. The nonlinear fractional-order equations for the spiral heat exchanger model are described as follows.

$$\begin{array}{c}\hfill \left\{\begin{array}{c}\hfill T_{hk}={\left(\mathsf{\Delta }\theta \right)}^{q_1}{\displaystyle \frac{K_h}{Q{L}_1}}(H{T}_{cK-1}-T_{hK-1})+B_h{T}_{hk-1}\hfill \\ \hfill T_{ck}={\left(\mathsf{\Delta }\theta \right)}^{q_2}{\displaystyle \frac{K_c}{Q{L}_2}}(H{T}_{hK-1}-T_{cK-1})+B_c{T}_{ck-1}\hfill \\ \hfill T_{cout}=C{T}_{ck}\hfill \end{array}\right.\end{array}$$

(23)

$$\begin{array}{c}\hfill \left\{\begin{array}{c}\hfill T_{hk}={\left(\mathsf{\Delta }\theta \right)}^{q_1}{\displaystyle \frac{(k+\mathsf{\Delta }k)Z{A}_1}{Q{L}_1{c}_h{\rho }_h}}(H{T}_{cK-1}-T_{hK-1})+B_h{T}_{hk-1}\hfill \\ \hfill T_{ck}={\left(\mathsf{\Delta }\theta \right)}^{q_2}{\displaystyle \frac{(k+\mathsf{\Delta }k)Z{A}_2}{Q{L}_2{c}_c{\rho }_c}}(H{T}_{hK-1}-T_{cK-1})+B_c{T}_{ck-1}\hfill \\ \hfill T_{cout}=C{T}_{ck}\hfill \end{array}\right.\end{array}$$

(24)

$$\begin{array}{c}\hfill k={\displaystyle \frac{{\delta }_s}{\lambda }}\end{array}$$

(25)

where $\mathsf{\Delta }k$ are the uncertainties for the spiral heat exchanger.

4.2. Operator-Based Controller Design

In Figure 2, we show an operator nonlinear control system, where operators V and Y are the input and output spaces of this plant. Let (N, D) be the right factorization of P. The feedback nonlinear control system shown in Figure 2 is BIBO stable if there exist two stable operators A: Y → V, B: V → V (B is also invertible) satisfying Bezout’s identity equation.

$$\begin{array}{c}\hfill AN+BD=M\end{array}$$

(26)

where M is a unimodular operator. Considering the uncertain term in the nonlinear system, with the designed operators A and B in Figure 2, the following equation could be satisfied.

$$\begin{array}{c}\hfill \parallel A((N+\mathsf{\Delta }N)-AN)M^{-1}{\parallel }_{Lip}<1\end{array}$$

(27)

where ${\parallel •\parallel }_{Lip}$ is a Lipschitz norm. Then, the nonlinear feedback control system has robust stability and M is a unimodular operator. The mathematical modeling $\tilde{P}$ considering the uncertain term $\mathsf{\Delta }P$ can be given for the the spiral heat exchanger system as follows.

$P:$

$$\begin{array}{c}\hfill \left\{\begin{array}{c}\hfill T_{hk}={\left(\mathsf{\Delta }\theta \right)}^{q_1}{\displaystyle \frac{kZ{A}_1}{Q{L}_1{c}_h{\rho }_h}}(H{T}_{cK-1}-T_{hK-1})+B_h{T}_{hk-1}\hfill \\ \hfill T_{ck}={\left(\mathsf{\Delta }\theta \right)}^{q_2}{\displaystyle \frac{kZ{A}_2}{Q{L}_2{c}_c{\rho }_c}}(H{T}_{hK-1}-T_{cK-1})+B_c{T}_{ck-1}\hfill \\ \hfill T_{cout}=C{T}_{ck}\hfill \end{array}\right.\end{array}$$

(28)

$K_s:$

$$\begin{array}{c}\hfill T_{hk-1}=\left(\begin{array}{ccccc}0& 0& \dots & 0& 0\\ 1& 0& \dots & 0& 0\\ 0& 1& \dots & 0& 0\\ ⋮& ⋮& ⋮& ⋮& ⋮\\ 0& 0& \dots & 1& 0\end{array}\right)T_{hk}+\left(\begin{array}{c}1\\ 0\\ 0\\ ⋮\\ 0\end{array}\right)T_{hin}\end{array}$$

(29)

$$\begin{array}{c}\hfill T_{ck-1}=\left(\begin{array}{ccccc}0& 0& \dots & 0& 0\\ 1& 0& \dots & 0& 0\\ 0& 1& \dots & 0& 0\\ ⋮& ⋮& ⋮& ⋮& ⋮\\ 0& 0& \dots & 1& 0\end{array}\right)T_{ck}+\left(\begin{array}{c}1\\ 0\\ 0\\ ⋮\\ 0\end{array}\right)T_{cin}\end{array}$$

(30)

where $Z^{-1}$ denotes a time delay of one sample period.

$P+\mathsf{\Delta }P:$

$$\begin{array}{c}\hfill \left\{\begin{array}{c}\hfill T_{hk}={\left(\mathsf{\Delta }\theta \right)}^{q_1}{\displaystyle \frac{(k+\mathsf{\Delta }k)Z{A}_1}{Q{L}_1{c}_h{\rho }_h}}(H{T}_{cK-1}-T_{hK-1})+B_h{T}_{hk-1}\hfill \\ \hfill T_{ck}={\left(\mathsf{\Delta }\theta \right)}^{q_2}{\displaystyle \frac{(k+\mathsf{\Delta }k)Z{A}_2}{Q{L}_2{c}_c{\rho }_c}}(H{T}_{hK-1}-I{T}_{cK-1})+B_c{T}_{ck-1}\hfill \\ \hfill T_{cout}=C{T}_{ck}\hfill \end{array}\right.\end{array}$$

(31)

The plant can be right-coprime-factorized as follows.

$N+\mathsf{\Delta }N:W\to Y$

$$\begin{array}{c}\hfill T_{ck}={\left(\mathsf{\Delta }\theta \right)}^{q_2}{\displaystyle \frac{(k+\mathsf{\Delta }k)Z{A}_2}{Q{L}_2{c}_c{\rho }_c}}(Hw-T_{cK-1})+B_c{T}_{ck-1}\end{array}$$

(32)

$D^{-1}:V\to W$

$$\begin{array}{c}\hfill w={\left(\mathsf{\Delta }\theta \right)}^{q_1}\left({\displaystyle \frac{k{A}_{1}Z}{Q{L}_1{c}_h{\rho }_h{\delta }_h}}(H{T}_{cK-1}-T_{hK-1})\right)+B_h{T}_{hk-1}\end{array}$$

(33)

The operator-based feedback control system is shown in Figure 10. The operators A and B were designed as follows.

$A:Y\to V$

$$\begin{array}{c}\hfill b=(1-K_p)H^{-1}[T_{ck-1}+\frac{Q{L}_2{c}_c{\rho }_c}{{\left(\mathsf{\Delta }\theta \right)}^{q_2}Zk{A}_2}({\widehat{T}}_{ck}-B_c{T}_{ck-1})]\end{array}$$

(34)

$$\begin{array}{c}\hfill {\widehat{T}}_{ck}={\displaystyle \frac{T_{cout}}{T_{ck}\left(end\right)}}{T}_{ck}\end{array}$$

(35)

$B^{-1}:V\to V$

$$\begin{array}{c}\hfill Q{L}_1={\left(\mathsf{\Delta }\theta \right)}^{q_1}{k}_p{\displaystyle \frac{Zk{A}_1(H{T}_{cK-1}-T_{hK-1})}{c_h{\rho }_h(e-B_h{T}_{hk-1})}}\end{array}$$

(36)

Operators A and $B^{-1}$ were designed to satisfy the Bezout identity and $k_p$ is the gain to be determined.

$$\begin{array}{c}\hfill \parallel A((N+\mathsf{\Delta }N)-AN)M^{-1}{\parallel }_{Lip}<1\end{array}$$

(37)

4.3. Fractional-Order Tracking Controller Design

The differential equation of fractional-order controller $P{I}^{\lambda }{D}^{\delta }$ is described as follows.

$$\begin{array}{c}\hfill q\left(t\right)=K_{p1}e\left(t\right)+K_i{D}_t^{-\lambda }e\left(t\right)+K_d{D}_t^{\delta }e\left(t\right)\end{array}$$

(38)

It is obvious that the fractional-order controller not only need three parameters $K_{p1},K_i$, and $K_d$, but also two orders $\lambda ,\delta$ of integral and derivative controller. The orders $\lambda ,\delta$ are not necessarily integers, but any real numbers. As shown in Figure 12, the FOPID (fractional-order PID) controller generalizes the conventional integer-order PID controller and expands it from a point to a plane. This expansion could provide much more flexibility in PID controller design.

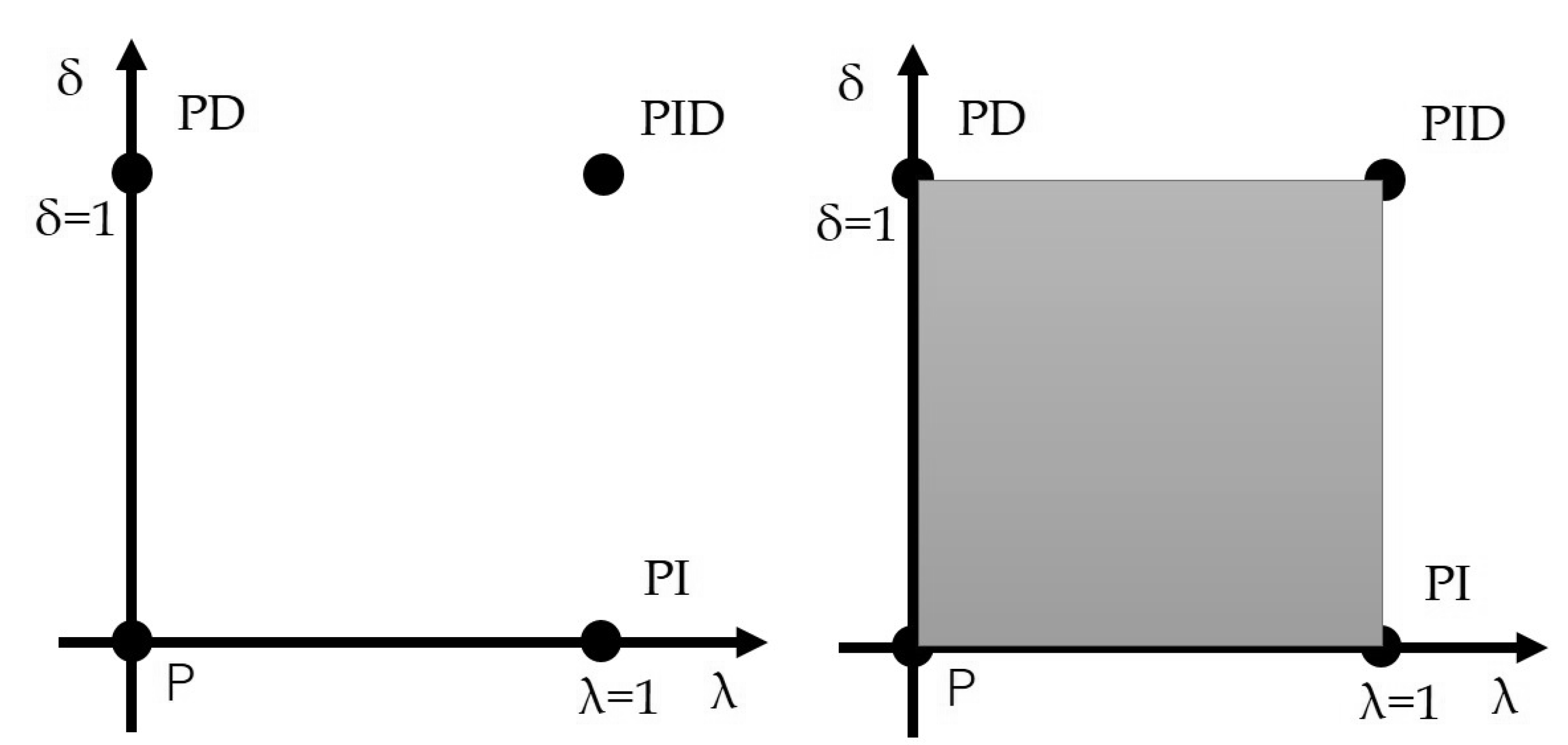
5. Parameter Optima for Fractional-Order PID Controller

The specific parameter estimation steps were follows.

(1)

Determine the searched spaces for the fractional-order PID controller.

The researched space vector was defined as

$$\begin{array}{c}\hfill x=\{K_p,K_i,K_d,\lambda ,\mathsf{\mu }\}\end{array}$$

(39)

where $K_p$, $K_i$, $K_d$ are the gains and $\lambda$, $\mathsf{\mu }$ are the orders. The range of the model parameter ($K_p$, $K_i$, $K_d$) was set to be [0 100], and the range of the model parameter ($\lambda$, $\mathsf{\mu }$) was set to be [0 1].

(2)

Determine the performance index (evaluation function).

For determining the optimum values of the gains of the controller, the integral of time multiplied by the absolute value of the error (ITAE), the integral of the absolute value of the error (IAE), the integral of time multiplied by the squared error (ITSE), and the integral of the squared error (ISE) were taken as objective functions. The expression for these error functions are given below:

$$\begin{array}{c}\hfill ITAE={\int }_0^{T}t|T_{cr}\left(t\right)-T_{cout}\left(t\right)|dt\end{array}$$

(40)

$$\begin{array}{c}\hfill IAE={\int }_0^T|T_{cr}\left(t\right)-T_{cout}\left(t\right)|dt\end{array}$$

(41)

$$\begin{array}{c}\hfill ITSE={\int }_0^{T}t{(T_{cr}\left(t\right)-T_{cout}\left(t\right))}^{2}dt\end{array}$$

(42)

$$\begin{array}{c}\hfill ISE={\int }_0^T{(T_{cr}\left(t\right)-T_{cout}\left(t\right))}^{2}dt\end{array}$$

(43)

where $T_{cout}$ is the output temperature, $T_{cr}$ is the reference input temperature, and T is the time range of the simulation. In this paper, The ITSE was used as the performance evaluation function.

(3)

The parameters of the algorithm are initialized to generate random search vectors.

(4)

According to the steps of the PSO algorithm, the parameters of fractional-order PID controller are identified.

(5)

The iteration is repeated until the performance index is satisfactory or the sum of iterations is maximal.

The evolutionary curves of the estimated parameters are plotted in Figure 13, Figure 14, Figure 15 and Figure 16. The evolutionary curves of the performance index are plotted in Figure 17 and Figure 18.

6. Analysis of Performance on Tracking and Antidisturbances for the Spiral Heat Exchanger with Disturbances

In this section, the performance of the temperature control system for the spiral heat exchanger is analyzed. Two control schemes were simulated as shown in Figure 10 and Figure 11.

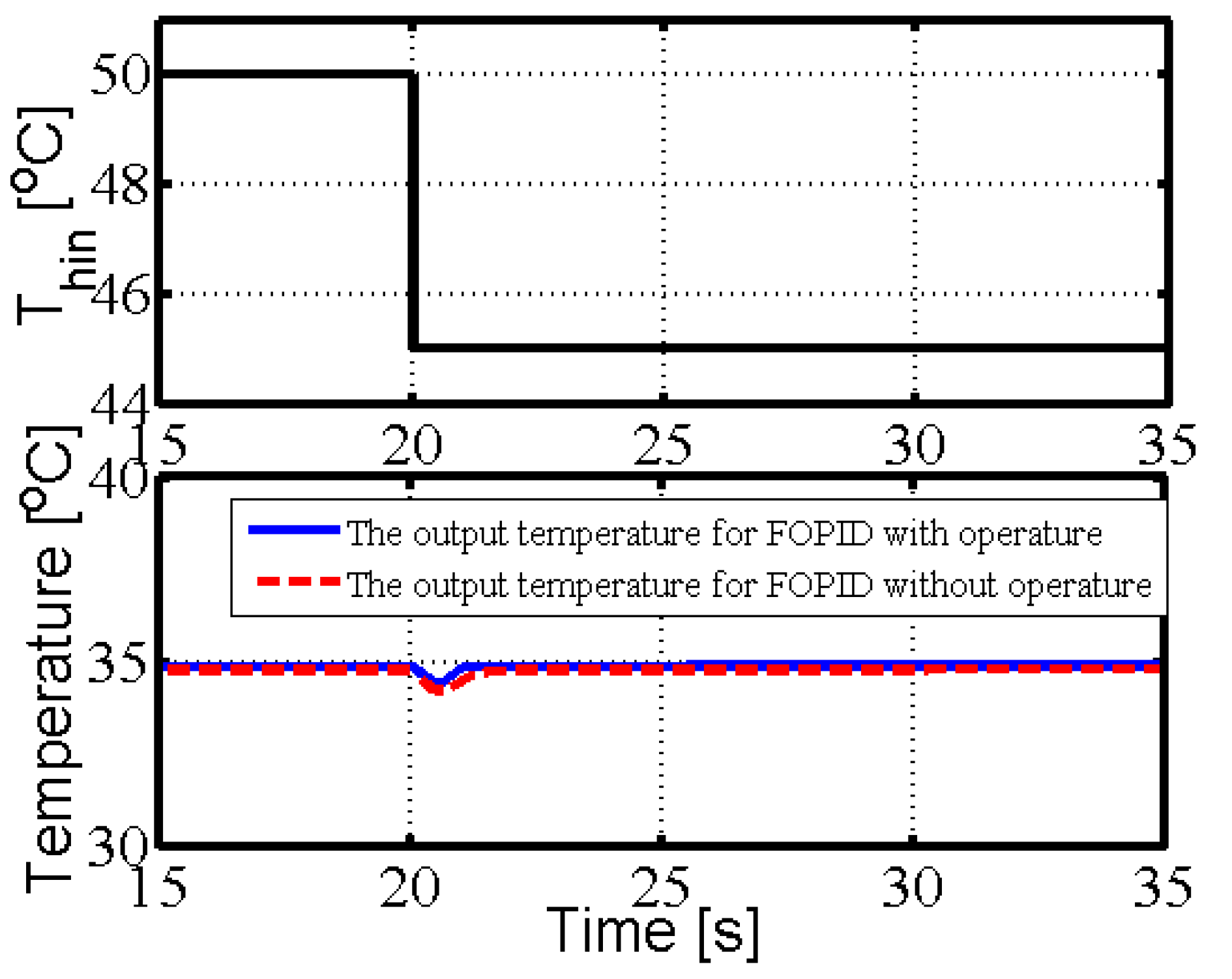
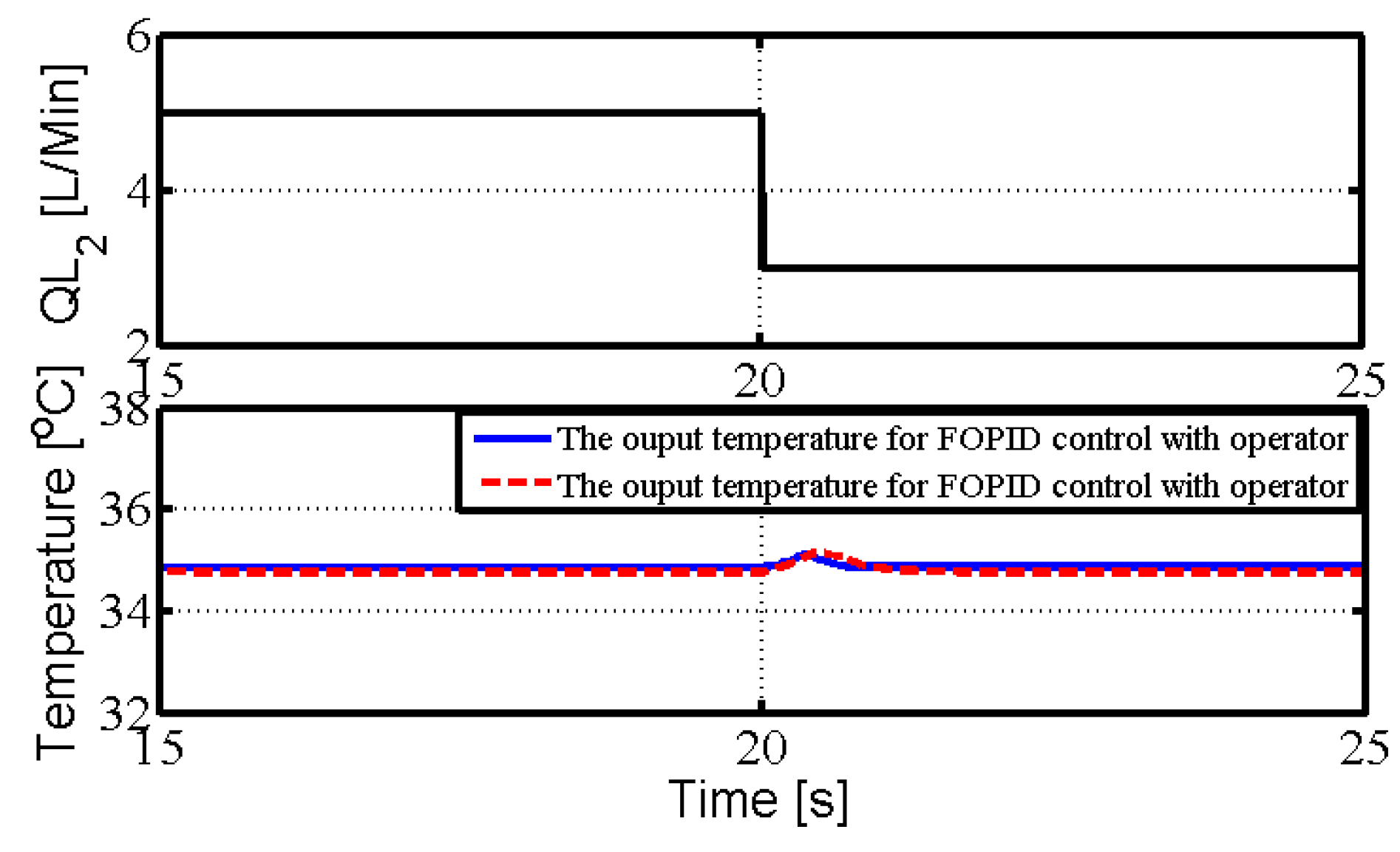
6.1. Tracking Performance for the Spiral Heat Exchanger with Disturbances

In the simulation, a reference input signal $T_r$ was decreased from 35 °C to 30 °C in 20 s; then, the reference input signal $T_r$ was up to 35 °C. Figure 19 shows the operator-based fractional-order PID control scheme has better tracking performance than the fractional-order PID control scheme without operator. There is a small overshoot and a short settling time.

6.2. Antidisturbance Performance for the Spiral Heat Exchanger

In real application, if the input flow rate of the hot-fluid side $Q{L}_1$ is as control input, then the input temperature of the cold-fluid side $T_{cin}$, the input temperature of the hot-fluid side $T_{hin}$, and the input flow rate of the cold-fluid side $Q{L}_2$ are three disturbances of the heat exchanger.

In the simulation, the disturbance signal $T_{cin}$ was decreased from 20 °C to 15 °C in 20 s, $T_{hin}$ was decreased from 50 °C to 45 °C in 20 s, and $Q{L}_1$ was decreased from 5 L/Min to 3 L/Min in 20 s. Figure 20, Figure 21 and Figure 22 show the operator-based fractional-order PID control scheme has better antidisturbance performance than the fractional-order PID control scheme without operator.

7. Conclusions

This paper proposed operator- and fractional-order-based nonlinear robust control for a spiral counter-flow heat exchanger described by a parallel fractional-order model in order to improve the tracking performance and antidisturbance ability. The parallel fractional-order model for the spiral counter-flow heat exchanger was identified by PSO and the parameters of the fractional-order PID controller were optimized by PSO. First, the parallel fractional-order mathematical model for the spiral heat exchanger plant was identified by PSO. Second, the operator controller for the spiral heat exchanger was designed under the identified parallel fractional-order model and a compensator was designed to reduce disturbance. Third, the parameters of the operator- and fractional-order-based PID controller were optimized by PSO. The tracking and antidisturbance performances of the control system were analyzed. Finally, comparisons of two control schemes were performed, and their effectiveness was illustrated.