A Wheeler–DeWitt Quantum Approach to the Branch-Cut Gravitation with Ordering Parameters
Abstract
1. Introduction
- The action is defined, using the Horaa–Lifshitz theory of gravity, which is the General Relativity augmented by counter-terms to render the theory regularized. For more information, please consult Ref. [24]. The basic ingredients are now expressed in terms of , which substitutes the standard scale factor . In Section 2.1, the classical impulse variable is defined and the classical Hamiltonian constructed.
- A quantization procedure is applied, elevating the momentum operator and Hamiltonian to operators. As a result we obtain the Wheeler–DeWitt equation.
- Following this path, a parameter appears which defines the ordering of the operators, as applied in the past to the Wheeler–DeWitt equation. This leaves us with three possible equations.
- These equations are solved using the Range–Kutta numerical analysis iterative method. Unlike the approaches usually found in the literature, in our calculations we do not use approximations. We then obtain new analytic solutions, depending on the boundary conditions based on the Bekenstein’s theorem, which provides an upper limit for the entropy. For more information, please consult [10,11,12,13,36].
2. Extended Class of the Branched Quantum Cosmological Solutions
2.1. Branch-Cut Formulation of the Weeler-DeWitt Equation
3. Spacetime Topological Canonical Quantization
3.1. Complex Conjugation of the Friedmann’s-Type Wave Equations
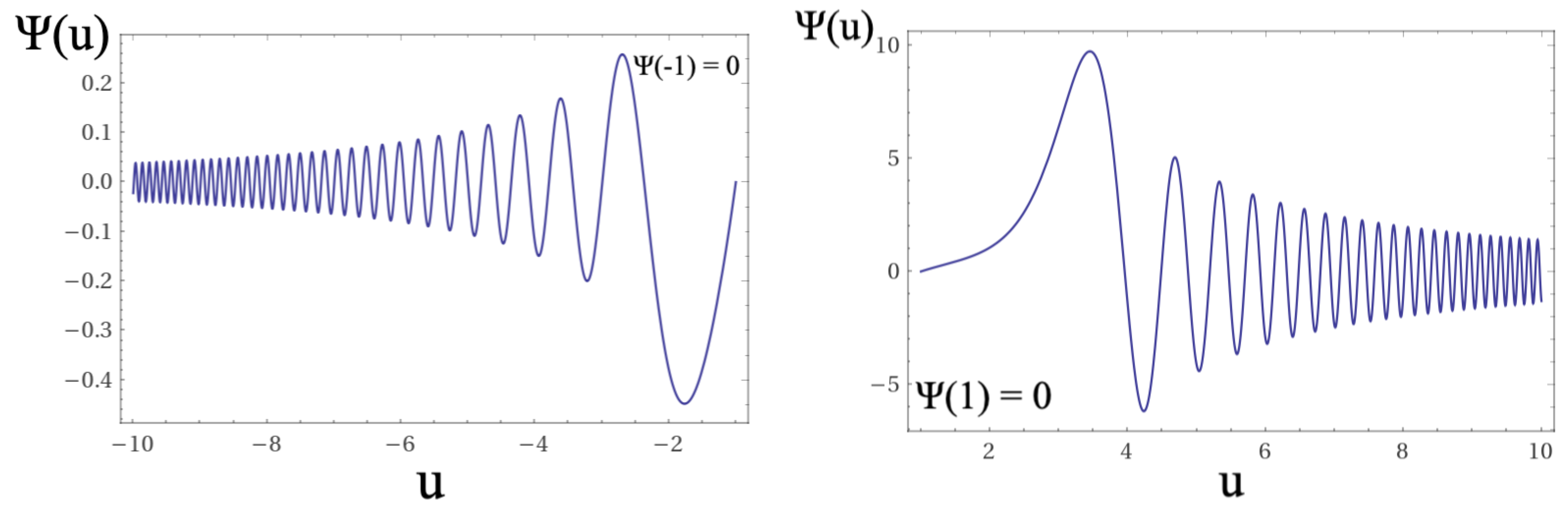
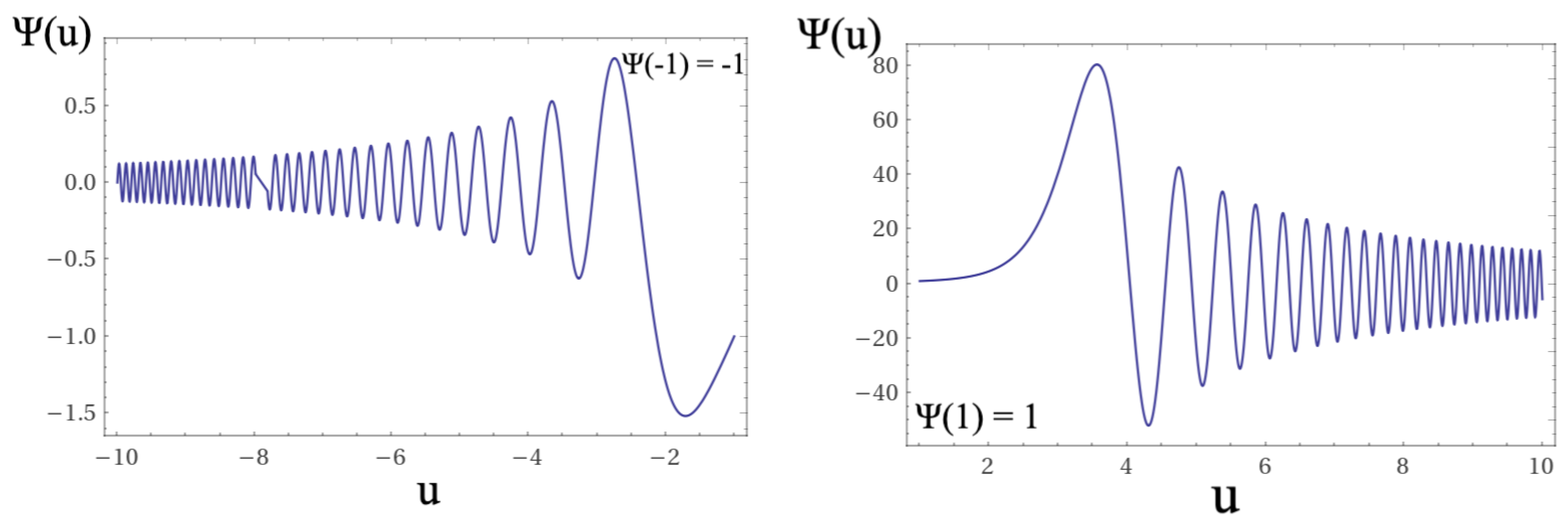
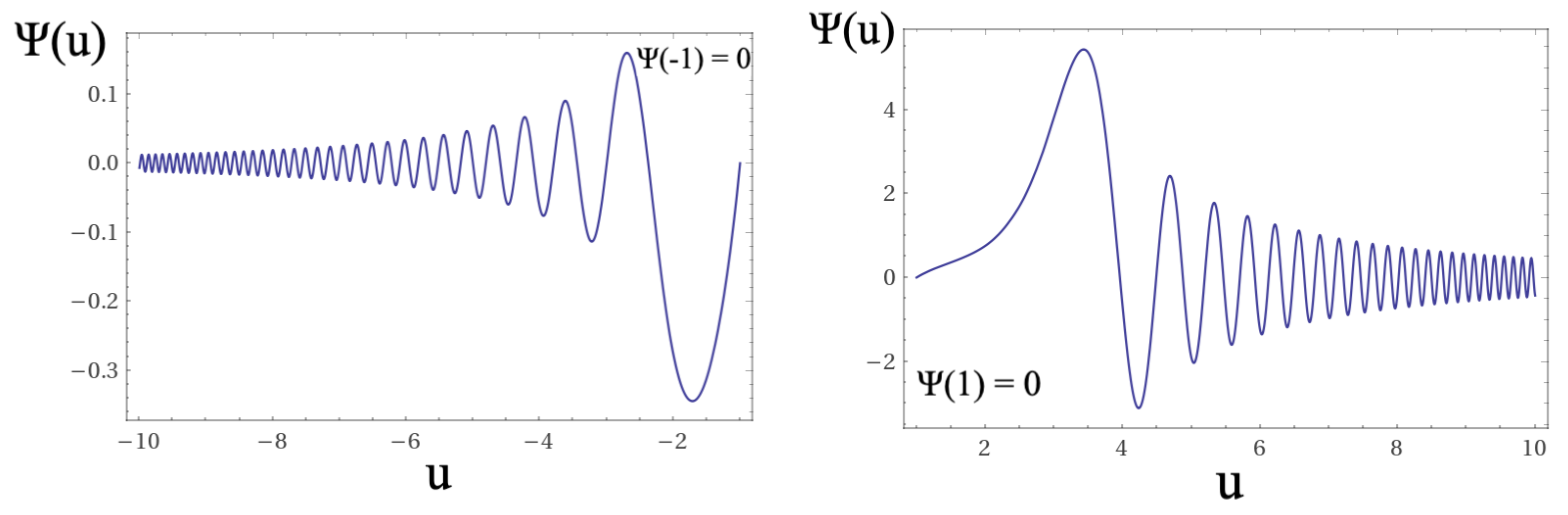
3.2. Solutions and Boundary Conditions
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
| 1 | Hawking and Hertog, in 2018, revisited the multiverse concept, conjecturing that the output of eternal inflation does not produce an infinite fractal-type multiverse, but is finite and reasonably smooth. |
| 2 | For simplicity the cosmological constant term has been suppressed. |
| 3 | We emphasize that these equations do not represent a direct parameterization or generalization of the conventional Friedmann equations described in a single-pole metric and likewise the new cosmic scale factor does not represent a simple parameterization of the standard theory scale factor. Due to the non-linearity of Einstein’s equations, such a direct generalization or parametrization would be inconsistent. For the details, see [7,8,9,12]. |
| 4 | The impossibility of packing energy and entropy according to the Bekenstein Criterion into a finite size makes the transition phase between contraction and expansion very peculiar, imposing a topology where space-time shapes itself topologically around a branch point. |
| 5 | The Hořava–Lifshitz (HL) formulation main goal is to get a renormalizable theory by means of higher spatial-derivative terms of the curvature which are added to the Einstein–Hilbert action [20]. A recurring problem addressed in the analysis of the Hořava–Lifshitz theory of gravity is related to the preservation of general diffeomorphism, a fundamental constraint of general relativity [22]. Although this is not the main topic of discussion, we would like to address that, in the case of restricted foliation preserving diffeomorphism invariance of the Hořava–Lifshitz theory, a well behaved Hamiltonian for gravity may be found [23]. |
| 6 | For an interesting discussion of this topic see Ref. [31]. |
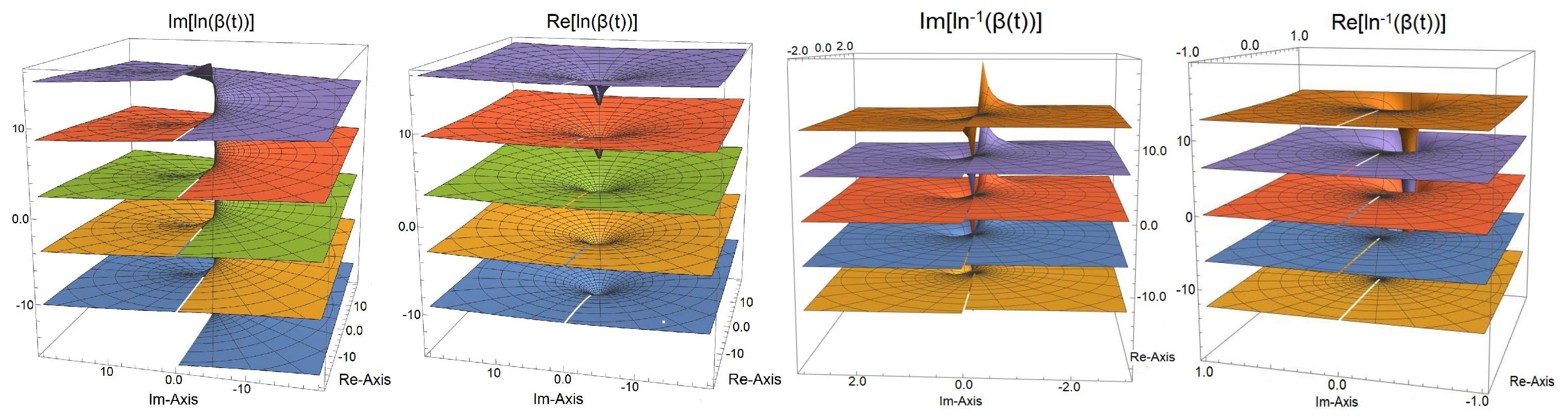
| 7 | We emphasize once more that represents the reciprocal of and identifies the range and cuts of the helix-like cosmological factor in branched gravitation. characterizes complex topological leafs of singular foliations by means of Riemann surfaces. |
| 8 | does note represent a dynamical quantity; in turn it denotes a pure gauge variable. |
| 9 | As is well know, there are several quantization methods, as for instance, the canonical quantization and the related Dirac scheme, Segal and Borel quantizations, geometric quantization, various ramifications of deformation quantization, Berezin and Berezin–Toeplitz quantizations, prime quantization and coherent state quantization. For a broad overview see [45]. The advantage of the canonical procedure to quantize a classical theory resides in the preservation of the original formal structure, symmetries and conservation laws. The denomination ‘spacetime topological canonical quantization’ is due to the combination of the conventional canonical quantization procedure applied to a variable, the helix-like complex cosmic scale factor of the branched gravitation, , raised to the category of quantum operator, which presents an intricate topology. |
| 10 | The condition excludes the multiplicative term in Equation (8). |
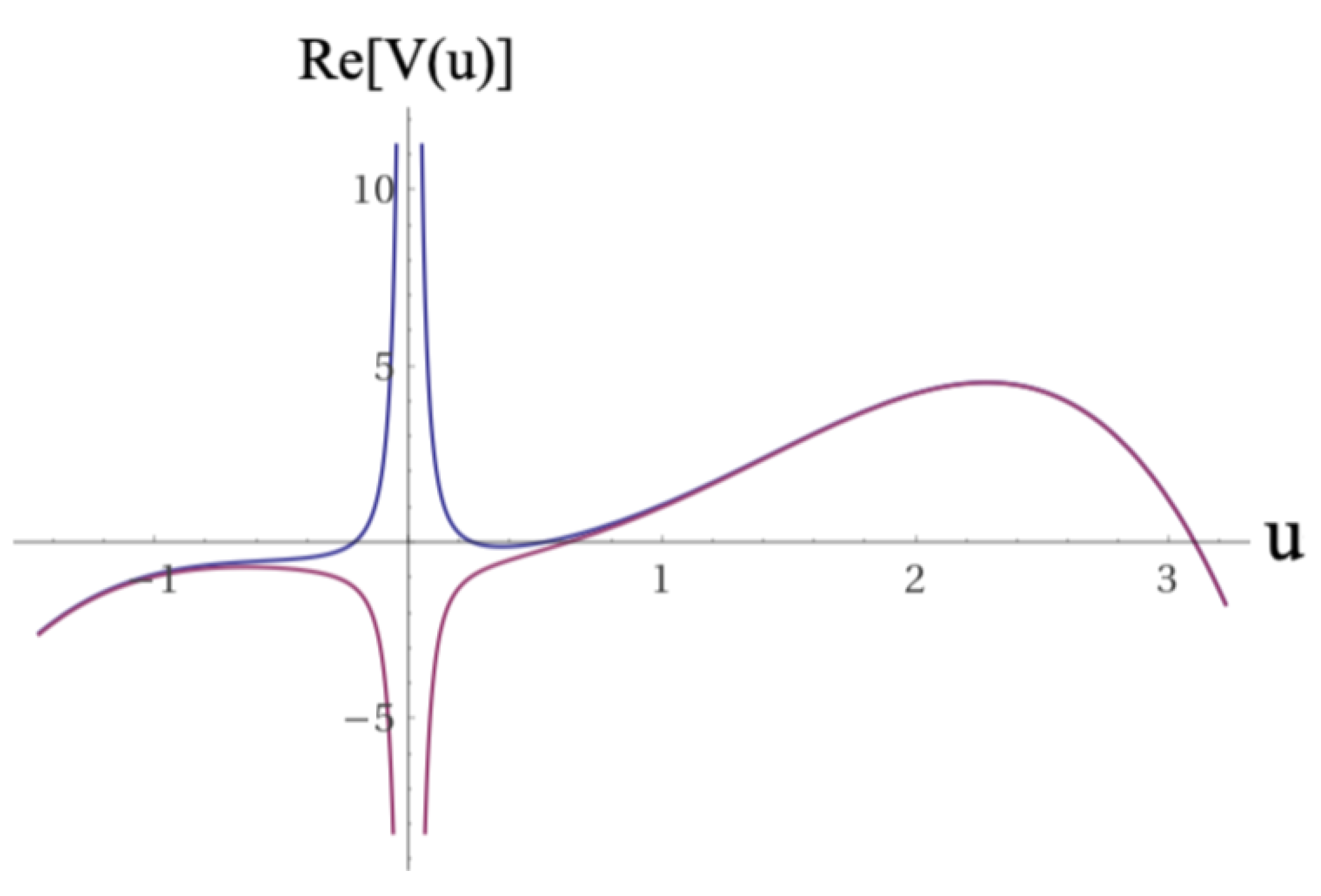
| 11 | Despite that we consider only the real part of the effective potential, the variable u is complex, and the solutions still have a broader scope, describing the behavior of the wave function of the Universe both for the contraction region, prior to the primordial singularity, and for the later expansion cosmological region. |
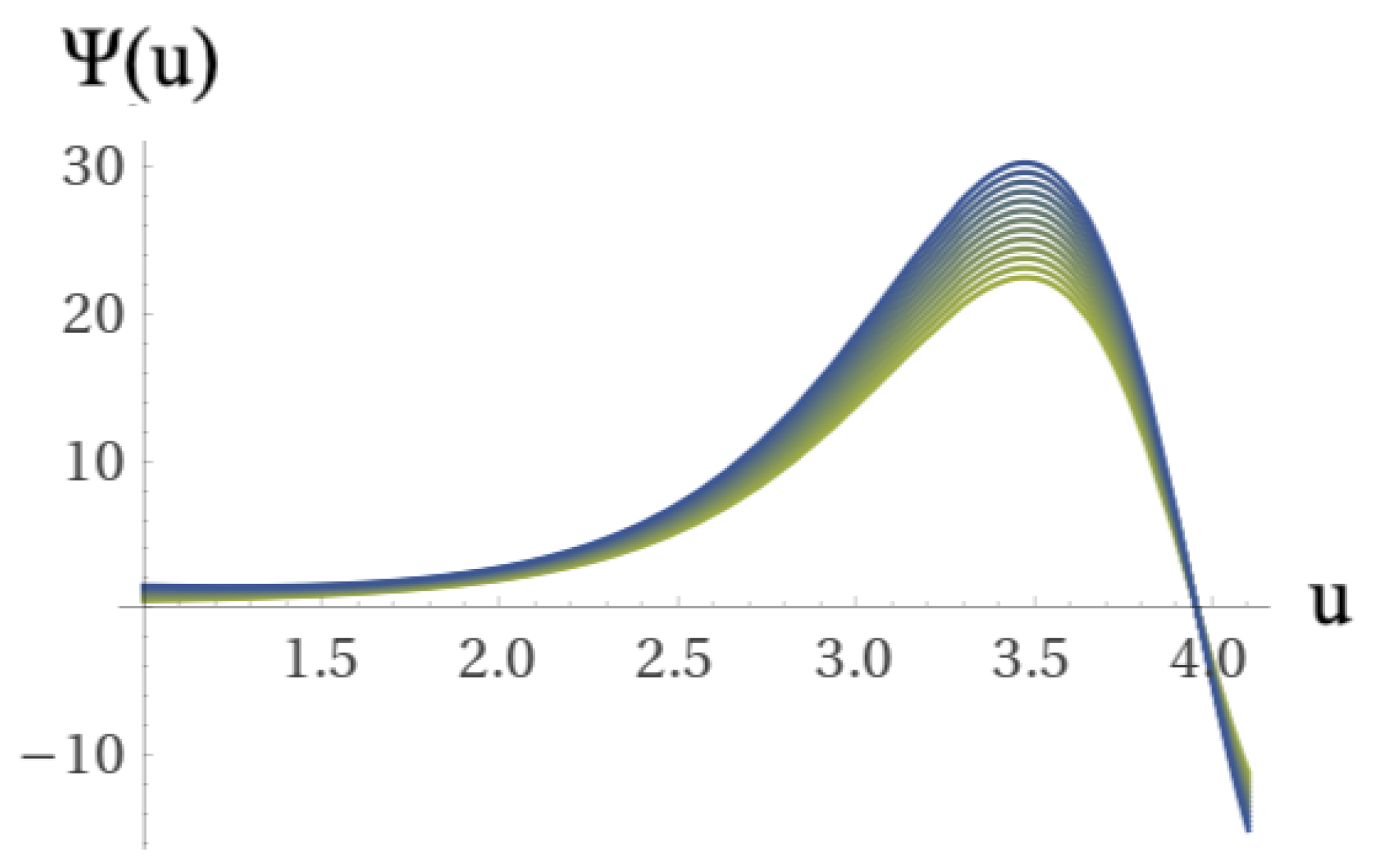
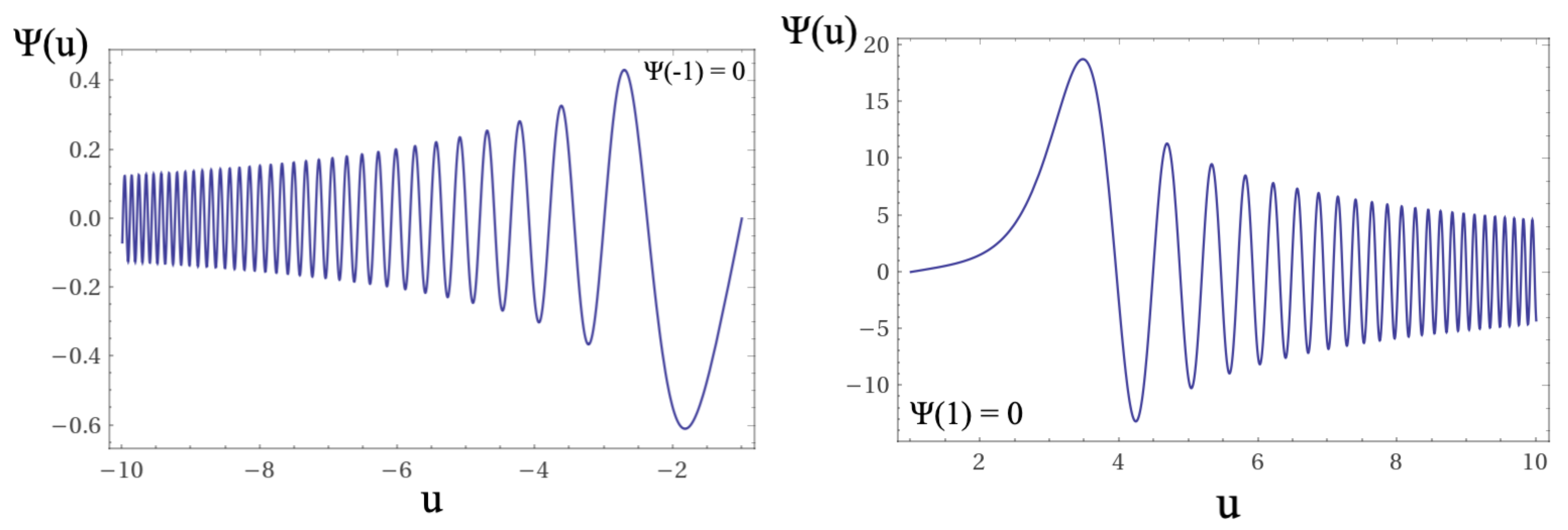
| 12 | The tunneling boundary condition of Vilenkin [51] in particular has two degrees of freedom: the scale factor and a homogeneous scalar field. A tunneling wave function then describes an ensemble of universes tunneling from ‘‘nothing’’ to a de Sitter space, and then evolving along the lines of an inflationary scenario and eventually collapsing to a singularity [51]. |
References
- Manders, K. Domain Extension and the Philosophy of Mathematics. J. Philos. 1989, 86, 553–562. [Google Scholar] [CrossRef]
- Dirac, P.A.M. Complex Variables in Quantum Mechanics. Proc. R. Soc. A 1937, 160, 48–59. [Google Scholar]
- Hess, P.O.; Greiner, W. Pseudo-complex General Relativity. Int. J. Mod. Phys. E 2009, 18, 51–77. [Google Scholar] [CrossRef]
- Hess, P.O.; Schäfer, M.; Greiner, W. Pseudo-Complex General Relativity; Springer International Publishing: Cham, Switzerland, 2016. [Google Scholar]
- Hess, P.O.; Greiner, W. Pseudo-Complex General Relativity: Theory and Observational Consequences. In Centennial of General Relativity: A Celebration; Zen Vasconcellos, C.A., Ed.; World Scientific Publishing Co.: Singapore, 2017; p. 97. [Google Scholar]
- Einstein, A. Die Grundlage der Allgemeinen Relativitätstheorie. Ann. Phys. 1916, 49, 769–822. [Google Scholar] [CrossRef]
- Zen Vasconcellos, C.A.; Hadjimichef, D.; Razeira, M.; Volkmer, G.; Bodmann, B. Pushing the Limits of General Relativity Beyond the Big Bang Singularity. Astron. Nachr. 2019, 340, 857–865. [Google Scholar] [CrossRef]
- Zen Vasconcellos, C.A.; Hess, P.O.; Hadjimichef, D.; Bodmann, B.; Razeira, M.; Volkmer, G.L. Pushing the limits of time beyond the Big Bang singularity: The branch cut universe. Astron. Nachr. 2021, 342, 765–775. [Google Scholar] [CrossRef]
- Zen Vasconcellos, C.A.; Hess, P.O.; Hadjimichef, D.; Bodmann, B.; Razeira, M.; Volkmer, G.L. Pushing the limits of time beyond the Big Bang singularity: Scenarios for the branch cut universe. Astron. Nachr. 2021, 342, 776–787. [Google Scholar] [CrossRef]
- Bodmann, B.; Zen Vasconcellos, C.A.; de Freitas Pacheco, J.; Hess, P.O.; Hadjimichef, D. Causality and the arrow of time in the branch-cut cosmology. Astron. Nachr. 2022, 344, e220086. [Google Scholar] [CrossRef]
- de Freitas Pacheco, J.; Zen Vasconcellos, C.A.; Hess, P.O.; Hadjimichef, D.; Bodmann, B. Branch-cut cosmology and the Bekenstein Criterion. Astron. Nachr. 2022, 344, e220070. [Google Scholar] [CrossRef]
- Zen Vasconcellos, C.A.; Hess, P.O.; de Freitas Pacheco, J.; Hadjimichef, D.; Bodmann, B. The branch-cut cosmology: Evidences and open questions. Astron. Nachr. 2022, e20220079. [Google Scholar] [CrossRef]
- Hess, P.O.; Zen Vasconcellos, C.A.; de Freitas Pacheco, J.; Hadjimichef, D.; Bodmann, B. The branch-cut cosmology: A topological canonical quantum-mechanics approach. Astron. Nachr. 2022, e20220101. [Google Scholar] [CrossRef]
- Aharonov, Y.; Bohm, D. Significance of electromagnetic potentials in the quantum theory. Phys. Rev. 1959, 115, 485–491. [Google Scholar] [CrossRef]
- Wu, K.-D.; Kondra, T.V.; Rana, S.; Scandolo, C.M.; Xiang, G.-Y.; Li, C.-F.; Guo, G.-C.; Streltsov, A. Operational resource theory of imaginarity. Phys. Rev. Lett. 2021, 126, 090401. [Google Scholar] [CrossRef]
- Hawking, S.W.; Hertog, T. A smooth exit from eternal inflation? J. High Energ. Phys. 2018, 4, 1–17. [Google Scholar] [CrossRef]
- Ijjas, A.; Steinhardt, P.J.; Loeb, A. Scale-free primordial cosmology. Phys. Rev. 2014, D89, 023525. [Google Scholar] [CrossRef]
- Ijjas, A.; Steinhardt, P.J. Bouncing cosmology made simple. Class. Quantum Gravity 2018, 35, 135004. [Google Scholar] [CrossRef]
- Ijjas, A.; Steinhardt, P.J. A new kind of cyclic universe. Phys. Lett. B 2019, 795, 666–672. [Google Scholar] [CrossRef]
- Hořava, P. Quantum gravity at a Lifshitz point. Phys. Rev. D 2009, 79, 084008. [Google Scholar] [CrossRef]
- DeWitt, B.S. Quantum Theory of Gravity. I. The Canonical Theory. Phys. Rev. 1967, 160, 1113. [Google Scholar] [CrossRef]
- Kiefer, C. Quantum Gravity, 3rd ed.; Oxford University Press: Oxford, UK, 2012. [Google Scholar]
- Klusoň, J. Hamiltonian analysis of nonrelativistic covariant restricted-foliation-preserving diffeomorphism invariant Hořava-Lifshitz gravity. Phys. Rev. D 2011, 83, 044049. [Google Scholar] [CrossRef]
- García-Compeán, H.; Mata-Pacheco, D. Lorentzian Vacuum Transitions in Hořava–Lifshitz Gravity. Universe 2022, 8, 237. [Google Scholar] [CrossRef]
- Hartle, J.B.; Hawking, S.W. Wave function of the Universe. Phys. Rev. D 1983, 28, 2960. [Google Scholar] [CrossRef]
- Hawking, S.W. The boundary conditions of the Universe. Pontif. Acad. Sci. Scr. Varia 1982, 48, 563. [Google Scholar]
- Rovelli, C. The strange equation of quantum gravity. Class. Quantum Gravity 2015, 32, 124005. [Google Scholar] [CrossRef]
- Rovelli, C. The Order of Time; Riverhead Books: New York, NY, USA, 2019. [Google Scholar]
- Rovelli, C. Quantum Gravity; Cambridge University Press: Cambridge, UK, 2004. [Google Scholar]
- Rovelli, C.; Smerlak, M. Thermal time and Tolman–Ehrenfest effect: ‘temperature as the speed of time’. Class. Quantum Gravity 2011, 28, 075007. [Google Scholar] [CrossRef]
- Steigl, R.; Hinterleitner, F. Factor ordering in standard quantum cosmology. Class. Quantum Gravity 2006, 23, 3879–3894. [Google Scholar] [CrossRef]
- Hawking, S.W.; Page, D.N. Operator ordering and the flatness of the universe. Nucl. Phys. B 1986, 264, 185. [Google Scholar] [CrossRef]
- Vieira, H.S.; Bezerra, V.B.; Muniz, C.R.; Cunha, M.S.; Christiansen, H.R. Class of solutions of the Wheeler–DeWitt equation with ordering parameter. Phys. Lett. B 2020, 809, 135712. [Google Scholar] [CrossRef]
- Caldwell, R.R.; Dave, R.; Steinhardt, P.J. Cosmological Imprint of an Energy Component with General Equation of State. Phys. Rev. Lett. 1998, 80, 1582. [Google Scholar] [CrossRef]
- Zlatev, I.; Wang, L.; Steinhardt, P.J. Quintessence, Cosmic Coincidence, and the Cosmological Constant. Phys. Rev. Lett. 1999, 82, 896. [Google Scholar] [CrossRef]
- Bekenstein, J.D. Universal upper bound on the entropy-to-energy ratio for bounded systems. Phys. Rev. D 1981, 23, 287. [Google Scholar] [CrossRef]
- Gibbons, G.; Hawking, S.W. Cosmological event horizons, thermodynamics, and particle creation. Phys. Rev. D 1977, 15, 2752–2756. [Google Scholar] [CrossRef]
- York, J.W. Boundary terms in the action principles of general relativity. Found. Phys. 1986, 16, 249–257. [Google Scholar] [CrossRef]
- York, J.W. Role of Conformal Three-Geometry in the Dynamics of Gravitation. Phys. Rev. Lett. 1972, 28, 1082–1085. [Google Scholar] [CrossRef]
- Lukasz, A.G. Novel solution of Wheeler–DeWitt theory. Appl. Math. Phys. 2014, 2, 73–81. [Google Scholar]
- Bertolami, O.; Carlos, A.D.; Zarro, C.A.D. Hořava-Lifshitz quantum cosmology. Phys. Rev. D 2011, 84, 044042. [Google Scholar] [CrossRef]
- Maeda, K.-I.; Misonoh, Y.; Kobayashi, T. Oscillating Bianchi IX Universe in Hořava-Lifshitz Gravity. Phys. Rev. D 2010, 82, 064024. [Google Scholar] [CrossRef]
- Cordero, R.; Garcia-Compean, H.; Turrubiates, F.J. Lorentzian vacuum transitions in Hořava–Lifshitz Gravity. Gen. Relativ. Gravit. 2019, 51, 138. [Google Scholar] [CrossRef]
- Garattini, R.; Faizal, M. Cosmological constant from a deformation of the Wheeler–DeWitt equation. Nucl. Phys. B 2016, 905, 313–326. [Google Scholar] [CrossRef]
- Ali, S.T.; Engliš, M. Quantization Methods: A guide for physicists and analysts. Rev. Math. Phys. 2005, 17, 391–490. [Google Scholar] [CrossRef]
- França, U.; Rosenfeld, R. Quintessence models with exponential potentials. JHEP 2002, 0210, 15. [Google Scholar] [CrossRef]
- Hinshaw, G.; Larson, D.; Komatsu, E.; Spergel, D.N.; Bennett, C.L.; Dunkley, J.; Nolta, M.R.; Halpern, M.; Hill, R.S.; Odegard, N. Nine-year Wilkinson Microwave Anisotropy Probe (WMAP) observations: Cosmological parameter results. Astrophys. J. Suppl. Ser. 2013, 208, 19. [Google Scholar] [CrossRef]
- Kornmesser, M. History of the Universe (ESO). 2020. Available online: https://supernova.eso.org/exhibition/1101/ (accessed on 29 May 2023).
- Damour, T. and Vilenkin, A. Quantum instability of an oscillating universe. Phys. Rev. D 2019, 100, 083525. [Google Scholar] [CrossRef]
- Vilenkin, A. Creation of universes from nothing. Phys. Lett. B 1982, 117, 25. [Google Scholar] [CrossRef]
- Vilenkin, A. Boundary conditions in quantum cosmology. Phys. Rev. D 1986, 33, 3560. [Google Scholar] [CrossRef]
- Migkas, K.; Schellenberger, G.; Reiprich, T.H.; Pacaud, F.; Ramos-Ceja, M.E.; Lovisari, L. Probing cosmic isotropy with a new X-ray galaxy cluster sample through the LX–T scaling relation. Astron. Astroph. 2020, 636, A15. [Google Scholar] [CrossRef]
- Belinskii, V.A.; Khalatnikov, I.M.; Lifshitz, E.M. A general solution of the Einstein equations with a time singularity. Adv. Phys. 1982, 31, 639. [Google Scholar] [CrossRef]
- Mulryne, D.J.; Tavakol, R.; Lidsey, J.E.; Ellis, G.F.R. An Emergent Universe from a loop. Phys. Rev. D 2005, 71, 123512. [Google Scholar] [CrossRef]





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bodmann, B.A.L.; Vasconcellos, C.A.Z.; Bechstedt, P.O.H.; de Freitas Pacheco, J.A.; Hadjimichef, D.; Razeira, M.; Degrazia, G.A. A Wheeler–DeWitt Quantum Approach to the Branch-Cut Gravitation with Ordering Parameters. Universe 2023, 9, 278. https://doi.org/10.3390/universe9060278
Bodmann BAL, Vasconcellos CAZ, Bechstedt POH, de Freitas Pacheco JA, Hadjimichef D, Razeira M, Degrazia GA. A Wheeler–DeWitt Quantum Approach to the Branch-Cut Gravitation with Ordering Parameters. Universe. 2023; 9(6):278. https://doi.org/10.3390/universe9060278
Chicago/Turabian StyleBodmann, Benno August Ludwig, César Augusto Zen Vasconcellos, Peter Otto Hess Bechstedt, José Antonio de Freitas Pacheco, Dimiter Hadjimichef, Moisés Razeira, and Gervásio Annes Degrazia. 2023. "A Wheeler–DeWitt Quantum Approach to the Branch-Cut Gravitation with Ordering Parameters" Universe 9, no. 6: 278. https://doi.org/10.3390/universe9060278
APA StyleBodmann, B. A. L., Vasconcellos, C. A. Z., Bechstedt, P. O. H., de Freitas Pacheco, J. A., Hadjimichef, D., Razeira, M., & Degrazia, G. A. (2023). A Wheeler–DeWitt Quantum Approach to the Branch-Cut Gravitation with Ordering Parameters. Universe, 9(6), 278. https://doi.org/10.3390/universe9060278








