Collapse Dynamics of Vector Vortex Beams in Kerr Medium with Parity–Time-Symmetric Lattice Modulation
Abstract
1. Introduction
2. Theoretical Model
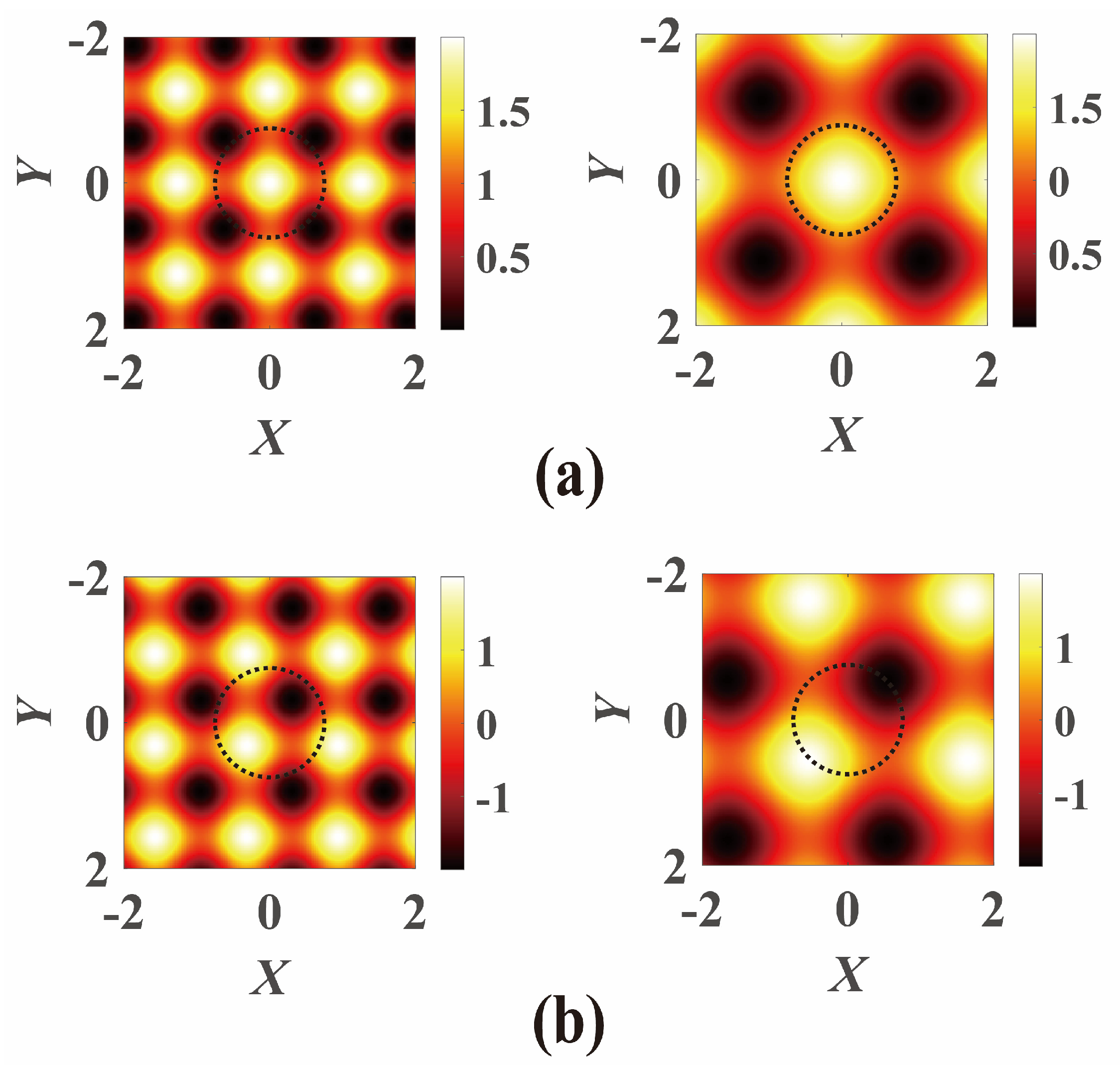
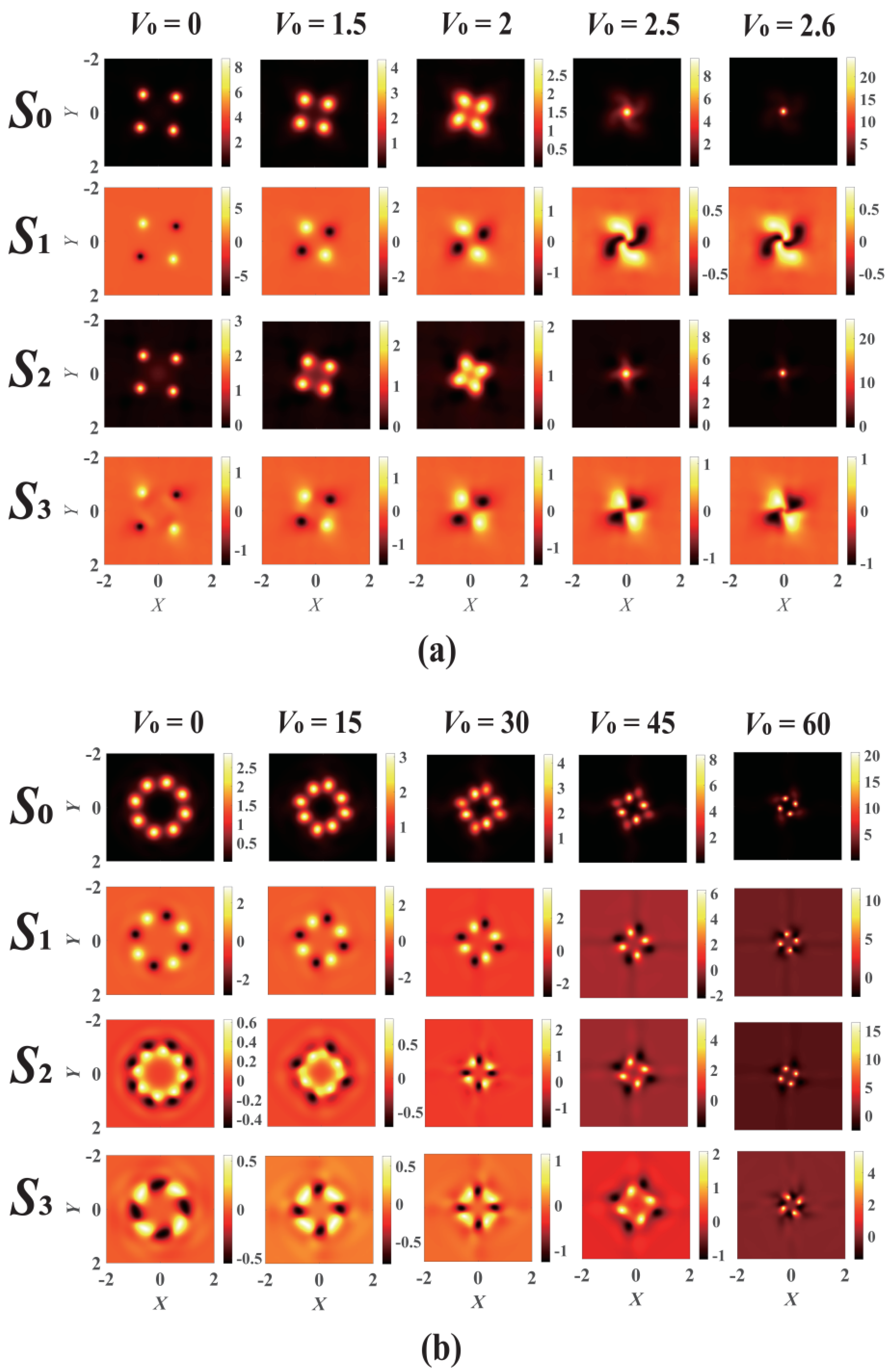
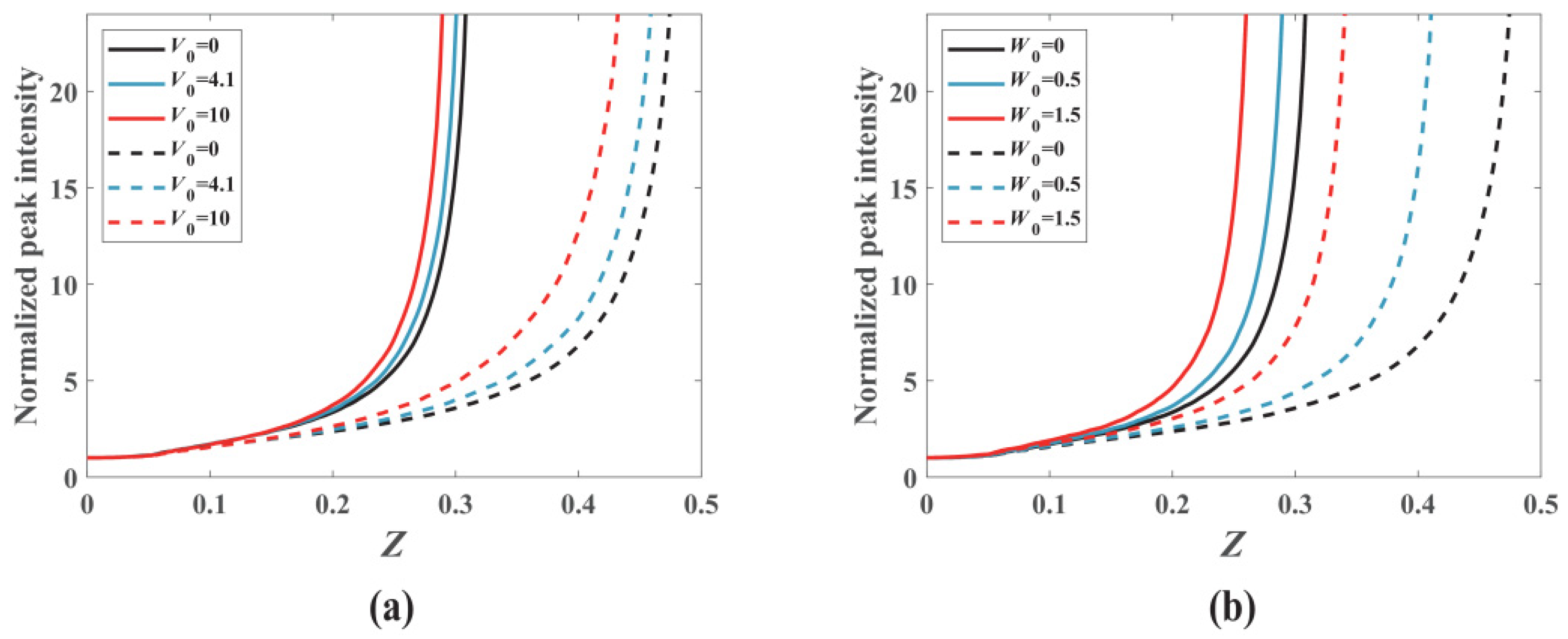
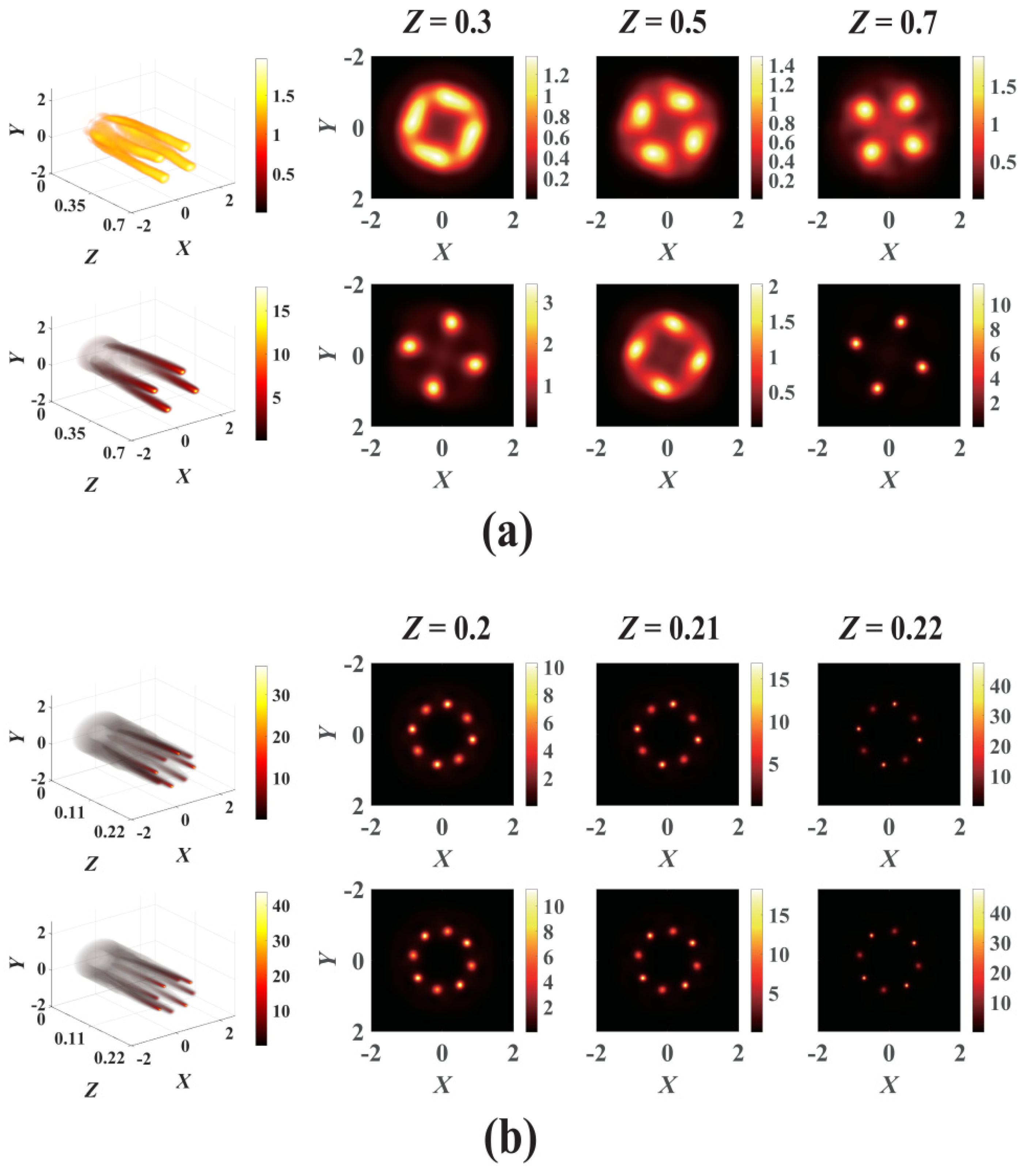
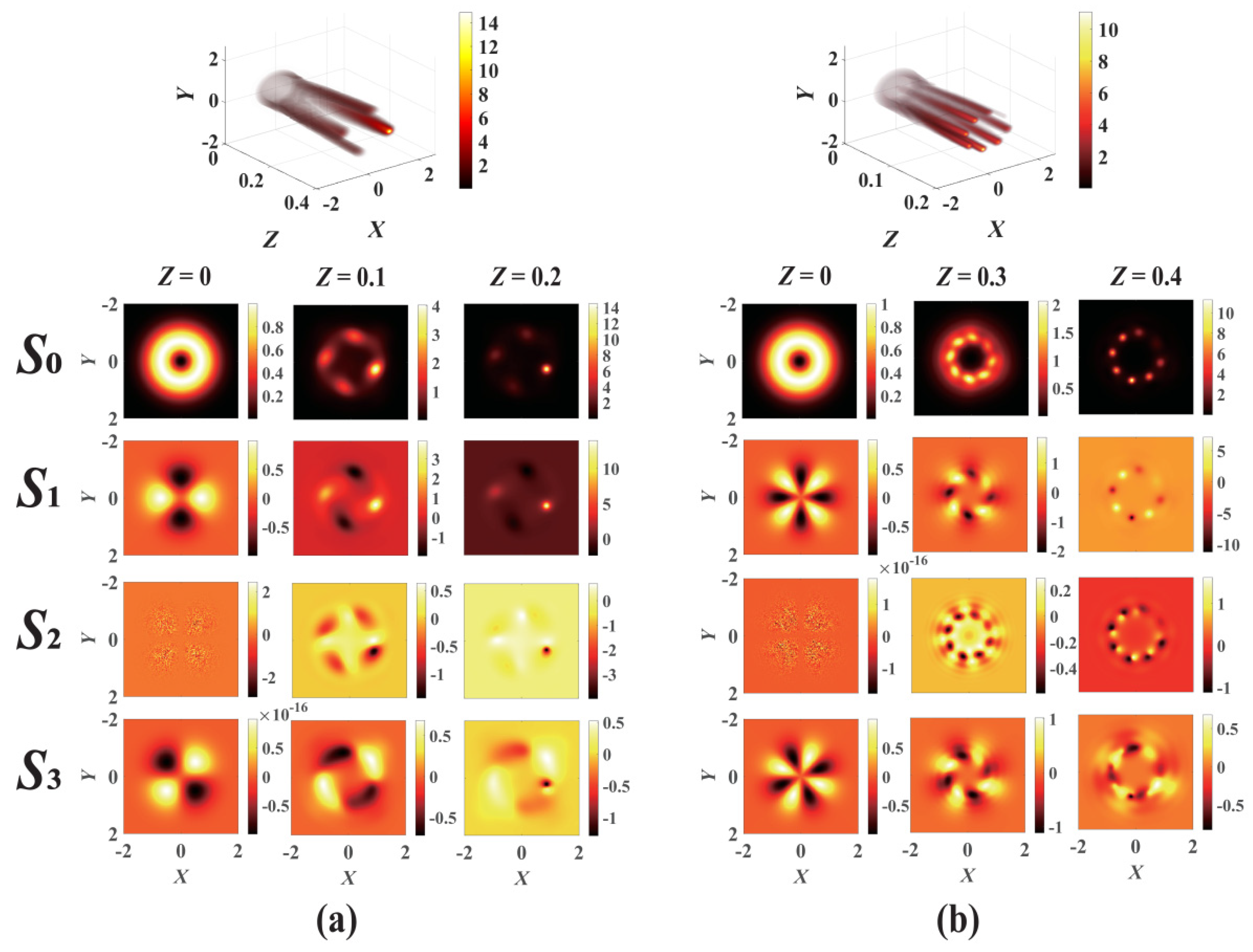
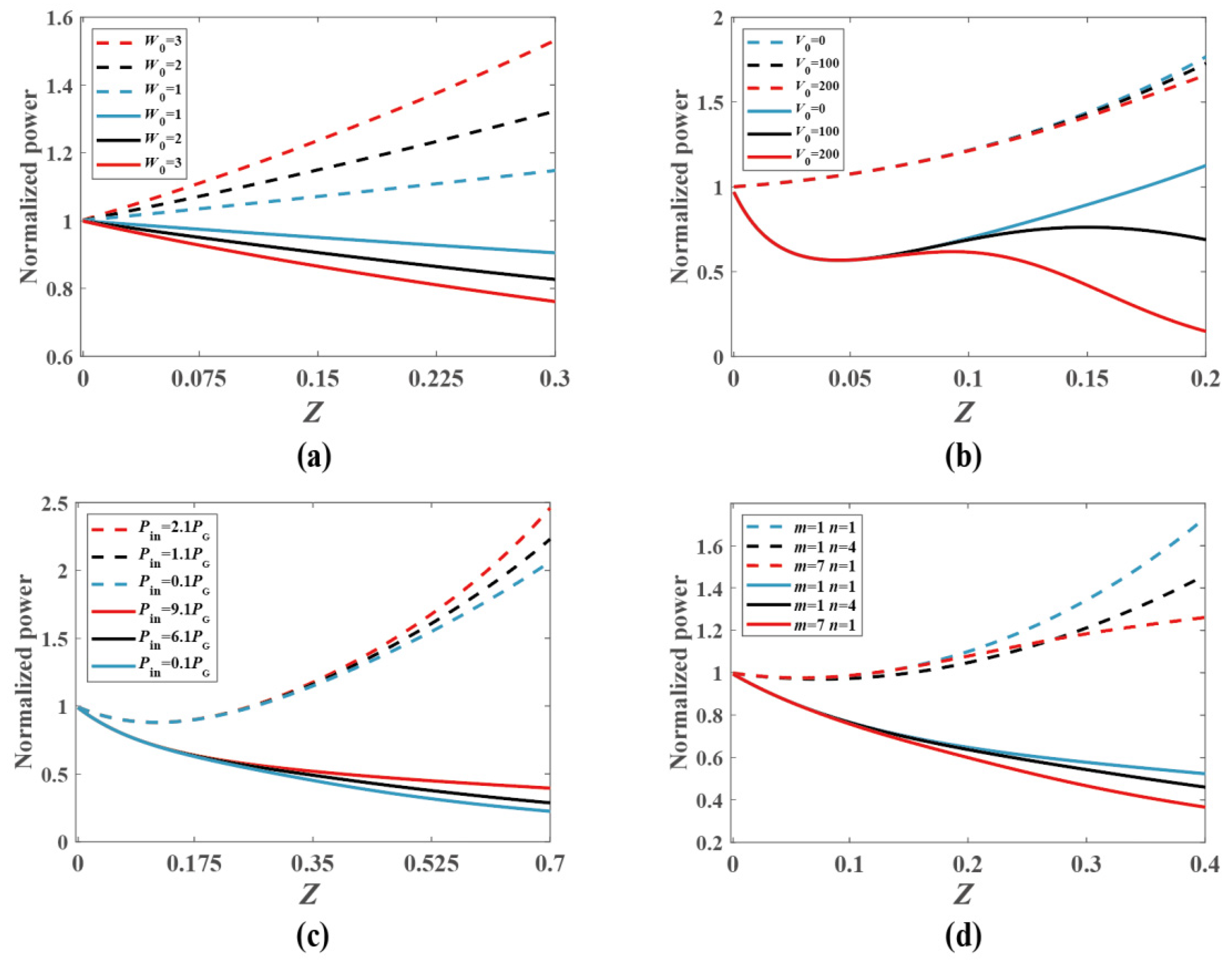
3. Numerical Results and Analysis
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Litchinitser, N.M. Structured Light Meets Structured Matter. Science 2012, 337, 1054–1055. [Google Scholar] [CrossRef] [PubMed]
- Chen, R.P.; Dai, C.Q. Vortex solitons of the (3+1)-dimensional spatially modulated cubic-quintic nonlinear Schrodinger equation with the transverse modulation. Nonlinear Dyn. 2017, 90, 1563–1570. [Google Scholar] [CrossRef]
- Zhang, H.; Tzortzakis, S. Robust authentication through stochastic femtosecond laser filament induced scattering surfaces. Appl. Phys. Lett. 2016, 108, 211107. [Google Scholar] [CrossRef]
- Tan, C.; Li, N.; Xu, D.; Chen, Z. Spatial focusing of surface polaritons based on cross-phase modulation. Results Phys. 2021, 27, 104531. [Google Scholar] [CrossRef]
- Tian, X.; Duan, Y.; Liu, S.; Liu, Q.; Tan, C. Propagation and excitation properties of nonlinear surface plasmon polaritons in a rectangular barrier. Phys. E 2022, 144, 115417. [Google Scholar] [CrossRef]
- Liu, S.; Han, Z.; Li, D.; Tan, C. Analysis of Interference Effect in Double Optomechanically Induced Transparency System. Photonics 2024, 11, 289. [Google Scholar] [CrossRef]
- Chen, R.P.; Chew, K.H.; He, S.L. Dynamic Control of Collapse in a Vortex Airy Beam. Sci. Rep. 2013, 3, 1406. [Google Scholar] [CrossRef]
- Chen, R.P.; Chew, K.H.; Zhou, G.Q.; Dai, C.Q.; He, S.L. Vectorial effect of hybrid polarization states on the collapse dynamics of a structured optical field. Opt. Express 2016, 24, 28143–28153. [Google Scholar] [CrossRef]
- Chen, R.P.; Zhong, L.X.; Chew, K.H.; Zhao, T.Y.; Zhang, X.B. Collapse dynamics of a vector vortex optical field with inhomogeneous states of polarization. Laser Phys. 2015, 25, 075401. [Google Scholar] [CrossRef]
- Yao, G.; Li, Y.H.; Chen, R.P. Collapse Dynamics of Vortex Beams in a Kerr Medium with Refractive Index Modulation and PT-Symmetric Lattices. Photonics 2022, 9, 249. [Google Scholar] [CrossRef]
- Kolesik, M.; Wright, E.M.; Moloney, J.V. Femtosecond filamentation in air and higher-order nonlinearities. Opt. Lett. 2010, 35, 2550–2552. [Google Scholar] [CrossRef]
- Trisorio, A.; Hauri, C.P. Control and characterization of multiple circularly polarized femtosecond filaments in argon. Opt. Lett. 2007, 32, 1650–1652. [Google Scholar] [CrossRef]
- Dubietis, A.; Tamosauskas, G.; Fibich, G.; Ilan, B. Multiple filamentation induced by input-beam ellipticity. Opt. Lett. 2004, 29, 1126–1128. [Google Scholar] [CrossRef]
- Bergé, L.; Gouédard, C.; Schjødt-Eriksen, J.; Ward, H. Filamentation patterns in Kerr media vs. beam shape robustness, nonlinear saturation and polarization states. Phys. D 2003, 176, 181–211. [Google Scholar] [CrossRef]
- Bergé, L. Wave collapse in physics: Principles and applications to light and plasma waves. Phys. Rep. 1998, 303, 259–370. [Google Scholar] [CrossRef]
- Robinson, P. Nonlinear wave collapse and strong turbulence. Rev. Mod. Phys. 1997, 69, 507. [Google Scholar] [CrossRef]
- Kasparian, J.; Wolf, J.-P. Physics and applications of atmospheric nonlinear optics and filamentation. Opt. Express 2008, 16, 466–493. [Google Scholar] [CrossRef]
- Tao, H.; Lin, J.; Hao, Z.; Gao, X.; Song, X.; Sun, C.; Tan, X. Formation of strong light-trapping nano-and microscale structures on a spherical metal surface by femtosecond laser filament. Appl. Phys. Lett. 2012, 100, 201111. [Google Scholar] [CrossRef]
- Zhao, J.; Guo, L.; Chu, W.; Zeng, B.; Gao, H.; Cheng, Y.; Liu, W. Simple method to enhance terahertz radiation from femtosecond laser filament array with a step phase plate. Opt. Lett. 2015, 40, 3838–3841. [Google Scholar] [CrossRef]
- Bender, C.M.; Boettcher, S. Real spectra in non-Hermitian Hamiltonians having PT symmetry. Phys. Rev. Lett. 1998, 80, 5243. [Google Scholar] [CrossRef]
- Bender, C.M.; Brody, D.C.; Jones, H.F. Complex extension of quantum mechanics. Phys. Rev. Lett. 2002, 89, 270401. [Google Scholar] [CrossRef] [PubMed]
- Klaiman, S.; Guenther, U.; Moiseyev, N. Visualization of branch points in PT-symmetric waveguides. Phys. Rev. Lett. 2008, 101, 080402. [Google Scholar] [CrossRef] [PubMed]
- Makris, K.G.; El-Ganainy, R.; Christodoulides, D.N.; Musslimani, Z.H. Beam dynamics in PT symmetric optical lattices. Phys. Rev. Lett. 2008, 100, 103904. [Google Scholar] [CrossRef] [PubMed]
- Ruter, C.E.; Makris, K.G.; El-Ganainy, R.; Christodoulides, D.N.; Segev, M.; Kip, D. Observation of parity-time symmetry in optics. Nat. Phys. 2010, 6, 192–195. [Google Scholar] [CrossRef]
- Hu, S.; Ma, X.; Lu, D.; Yang, Z.; Zheng, Y.; Hu, W. Solitons supported by complex PT-symmetric Gaussian potentials. Phys. Rev. A 2011, 84, 043818. [Google Scholar] [CrossRef]
- Zhu, X.; Yang, F.; Cao, S.; Xie, J.; He, Y. Multipole gap solitons in fractional Schrodinger equation with parity-time-symmetric optical lattices. Opt. Express 2020, 28, 1631–1639. [Google Scholar] [CrossRef] [PubMed]
- Konotop, V.V.; Yang, J.K.; Zezyulin, D.A. Nonlinear waves in PT-symmetric systems. Rev. Mod. Phys. 2016, 88, 035002. [Google Scholar] [CrossRef]
- Zeng, L.W.; Zeng, J.H. Preventing critical collapse of higher-order solitons by tailoring unconventional optical diffraction and nonlinearities. Commun. Phys. 2020, 3, 26. [Google Scholar] [CrossRef]
- Tamilselvan, K.; Govindarajan, A.; Inbavalli, I.; Alagesan, T.; Lakshmanan, M. Modulational instability in PT-symmetric Bragg grating structures with saturable nonlinearity. Phys. Rev. A 2023, 107, 053510. [Google Scholar] [CrossRef]
- Thasneem, A.R.; Subha, P.A. One-dimensional PT-symmetric eigenmodes in k-wave number Scarf II potential with defocusing nonlinearity. Phys. Scr. 2023, 98, 035208. [Google Scholar] [CrossRef]
- Li, L.; Li, H.G.; Ruan, W.; Leng, F.C.; Luo, X.B. Gap solitons in parity-time-symmetric lattices with fractional-order diffraction. J. Opt. Soc. Am. B 2020, 37, 488–494. [Google Scholar] [CrossRef]
- Che, W.W.; Yang, F.W.; Cao, S.L.; Wu, Z.L.; Zhu, X.; He, Y.J. Gray solitons in parity-time-symmetric localized potentials with fractional-order diffraction. Phys. Lett. A 2021, 413, 127606. [Google Scholar] [CrossRef]
- Li, J.W.; Zhang, Y.P.; Zeng, J.H. Matter-wave gap solitons and vortices in three-dimensional parity-time-symmetric optical lattices. Iscience 2022, 25, 104026. [Google Scholar] [CrossRef] [PubMed]
- Jin, L.J.; Hang, C.; Huang, G.X. Multidimensional optical solitons and their manipulation in a cold atomic gas with a parity-time-symmetric optical Bessel potential. Phys. Rev. A 2023, 107, 053501. [Google Scholar] [CrossRef]
- Liu, X.Y.; Zeng, J.H. Gap solitons in parity-time symmetric moire optical lattices. Photonics Res. 2023, 11, 196–202. [Google Scholar] [CrossRef]
- Feng, L.; El-Ganainy, R.; Ge, L. Non-Hermitian photonics based on parity-time symmetry. Nat. Photonics 2017, 11, 752–762. [Google Scholar] [CrossRef]
- Musslimani, Z.H.; Makris, K.G.; El-Ganainy, R.; Christodoulides, D.N. Optical solitons in PT periodic potentials. Phys. Rev. Lett. 2008, 100, 030402. [Google Scholar] [CrossRef]
- Zyablovsky, A.A.; Vinogradov, A.P.; Pukhov, A.A.; Dorofeenko, A.V.; Lisyansky, A.A. PT-symmetry in optics. Phys.-Uspekhi 2014, 57, 1063–1082. [Google Scholar] [CrossRef]
- Hang, C.; Zezyulin, D.A.; Huang, G.X.; Konotop, V.V.; Malomed, B.A. Tunable nonlinear double-core PT-symmetric waveguides. Opt. Lett. 2014, 39, 5387–5390. [Google Scholar] [CrossRef]
- Ramezani, H.; Kottos, T.; El-Ganainy, R.; Christodoulides, D.N. Unidirectional nonlinear PT-symmetric optical structures. Phys. Rev. A 2010, 82, 043803. [Google Scholar] [CrossRef]
- Yao, G.; Chew, K.H.; Wu, Y.; Li, Y.H.; Chen, R.P. Propagation dynamics of vector vortex beams in a strongly nonlocal nonlinear medium with parity-time-symmetric potentials. J. Opt. 2022, 24, 035606. [Google Scholar] [CrossRef]
- Chen, Y.; Yan, Z.; Mihalache, D. Soliton formation and stability under the interplay between parity-time-symmetric generalized Scarf-II potentials and Kerr nonlinearity. Phys. Rev. E 2020, 102, 012216. [Google Scholar] [CrossRef] [PubMed]
- Fan, Z.; Malomed, B.A. Dynamical control of solitons in a parity-time-symmetric coupler by periodic management. Commun. Nonlinear Sci. Simul. 2019, 79, 104906. [Google Scholar] [CrossRef]
- El-Ganainy, R.; Makris, K.G.; Christodoulides, D.N.; Musslimani, Z.H. Theory of coupled optical PT-symmetric structures. Opt. Lett. 2007, 32, 2632–2634. [Google Scholar] [CrossRef]
- Garcia-Ripoll, J.J.; Perez-Garcia, V.M.; Torres, P. Extended parametric resonances in nonlinear Schrödinger systems. Phys. Rev. lett 1999, 83, 1715. [Google Scholar] [CrossRef]
- Pérez-García, V.M.; Torres, P.J.; Montesinos, G.D. The method of moments for nonlinear Schrodinger equations:: Theory and applications. SIAM J. Appl. Math. 2007, 67, 990–1015. [Google Scholar] [CrossRef]
- Chen, R.-P.; Yin, C.-F.; Chu, X.-X.; Wang, H. Effect of Kerr nonlinearity on an Airy beam. Phys. Rev. A 2010, 82, 043832. [Google Scholar] [CrossRef]
- Kruglov, V.; Logvin, Y.A.; Volkov, V. The theory of spiral laser beams in nonlinear media. J. Mod. Opt. 1992, 39, 2277–2291. [Google Scholar] [CrossRef]
- Fibich, G.; Gavish, N. Critical power of collapsing vortices. Phy. Rev. A 2008, 77, 045803. [Google Scholar] [CrossRef]
- Fibich, G.; Gaeta, A.L. Critical power for self-focusing in bulk media and in hollow waveguides. Opt. Lett. 2000, 25, 335–337. [Google Scholar] [CrossRef]
- Chiao, R.Y.; Garmire, E.; Townes, C.H. Self-Trapping of Optical Beams. Phys. Rev. Lett. 1964, 13, 479. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zan, X.; Yao, G.; Wu, Y.; Guan, Y.; Chew, K.-H.; Chen, R.-P. Collapse Dynamics of Vector Vortex Beams in Kerr Medium with Parity–Time-Symmetric Lattice Modulation. Photonics 2024, 11, 345. https://doi.org/10.3390/photonics11040345
Zan X, Yao G, Wu Y, Guan Y, Chew K-H, Chen R-P. Collapse Dynamics of Vector Vortex Beams in Kerr Medium with Parity–Time-Symmetric Lattice Modulation. Photonics. 2024; 11(4):345. https://doi.org/10.3390/photonics11040345
Chicago/Turabian StyleZan, Xiaoxu, Gang Yao, Yan Wu, Ying Guan, Khian-Hooi Chew, and Rui-Pin Chen. 2024. "Collapse Dynamics of Vector Vortex Beams in Kerr Medium with Parity–Time-Symmetric Lattice Modulation" Photonics 11, no. 4: 345. https://doi.org/10.3390/photonics11040345
APA StyleZan, X., Yao, G., Wu, Y., Guan, Y., Chew, K.-H., & Chen, R.-P. (2024). Collapse Dynamics of Vector Vortex Beams in Kerr Medium with Parity–Time-Symmetric Lattice Modulation. Photonics, 11(4), 345. https://doi.org/10.3390/photonics11040345





