Relationship Between Pore-Size Distribution and 1D Compressibility of Different Reconstituted Clays Based on Fractal Theory
Abstract
1. Introduction
2. Testing Materials
3. Testing Procedures and Methods
3.1. One-Dimensional Compression Tests
3.2. Sample Preparations for Pore Structure Analysis
3.3. Measurement of Pore-Size Distribution (PSD)
3.4. PSD-Based Fractal Analysis
4. Testing Results
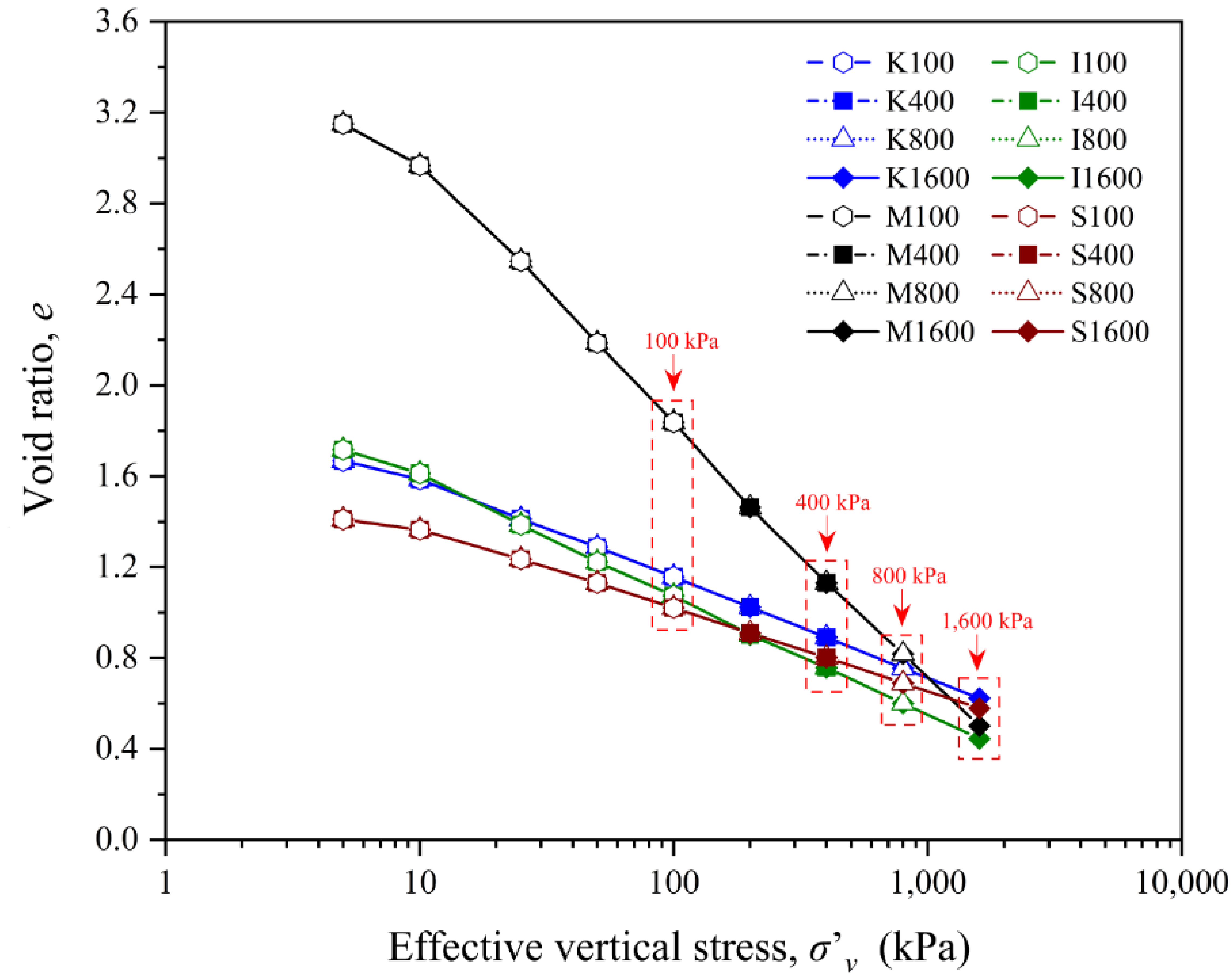
4.1. One-Dimensional Compressibility
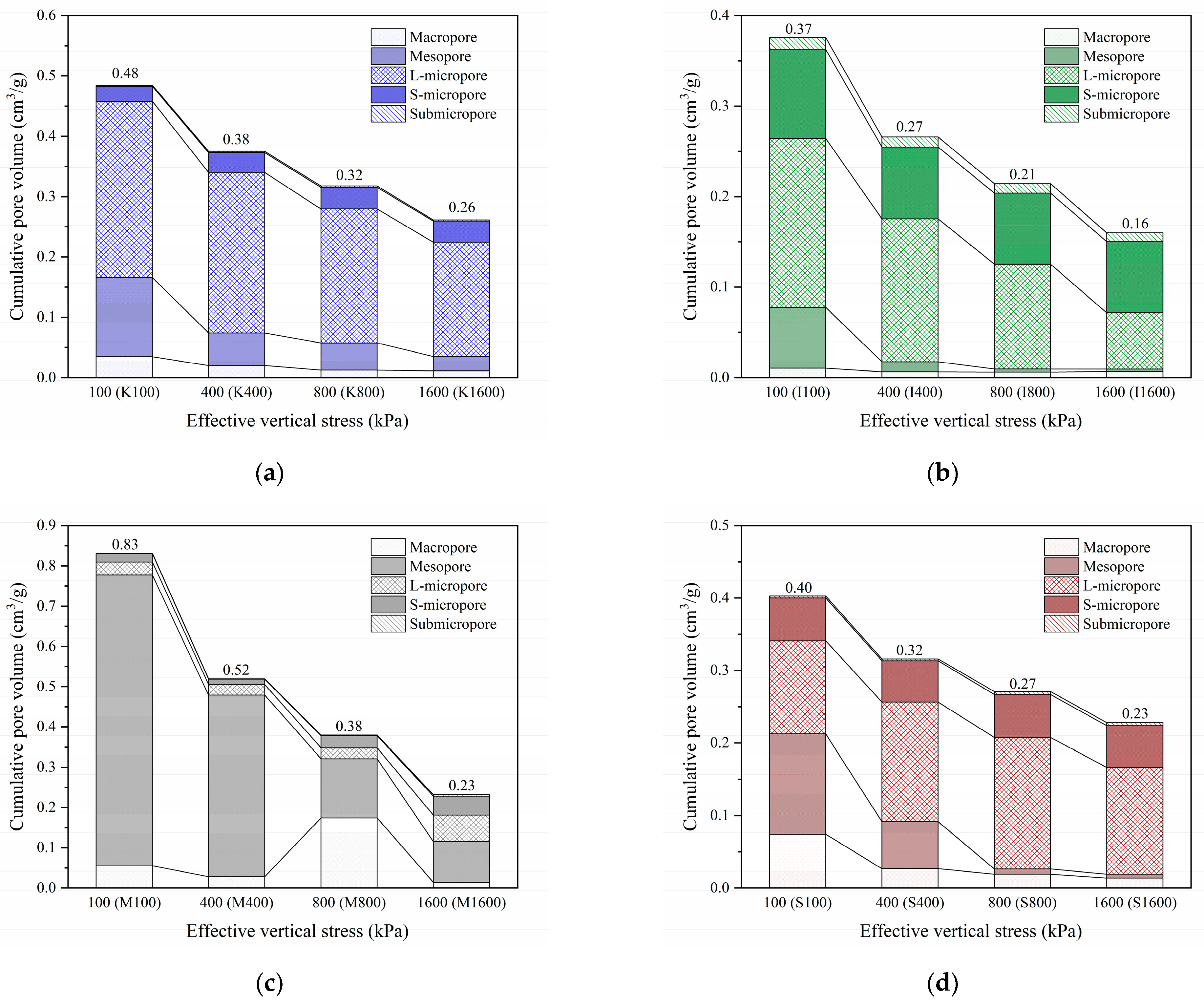
4.2. Evolution of the PSD
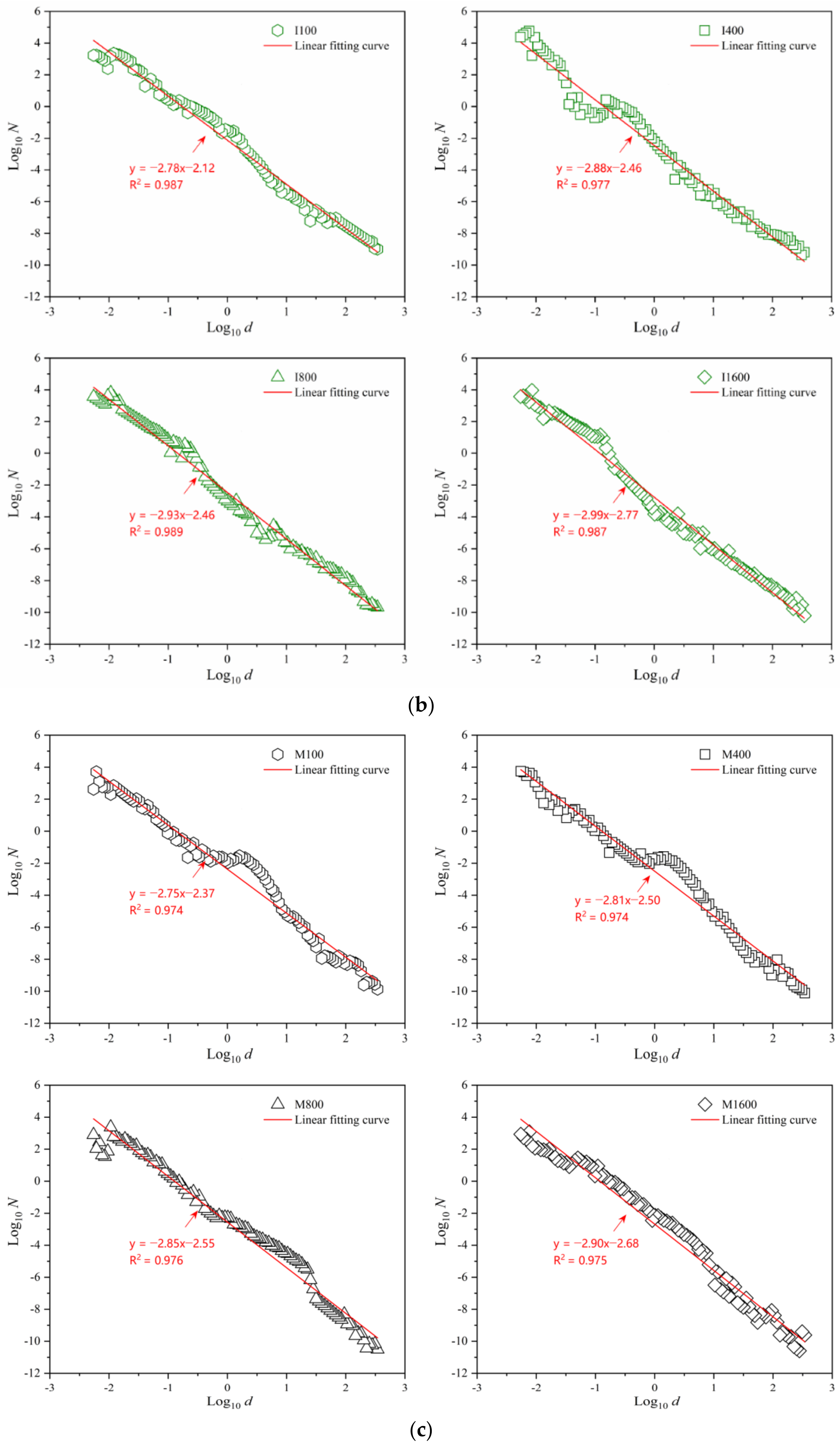
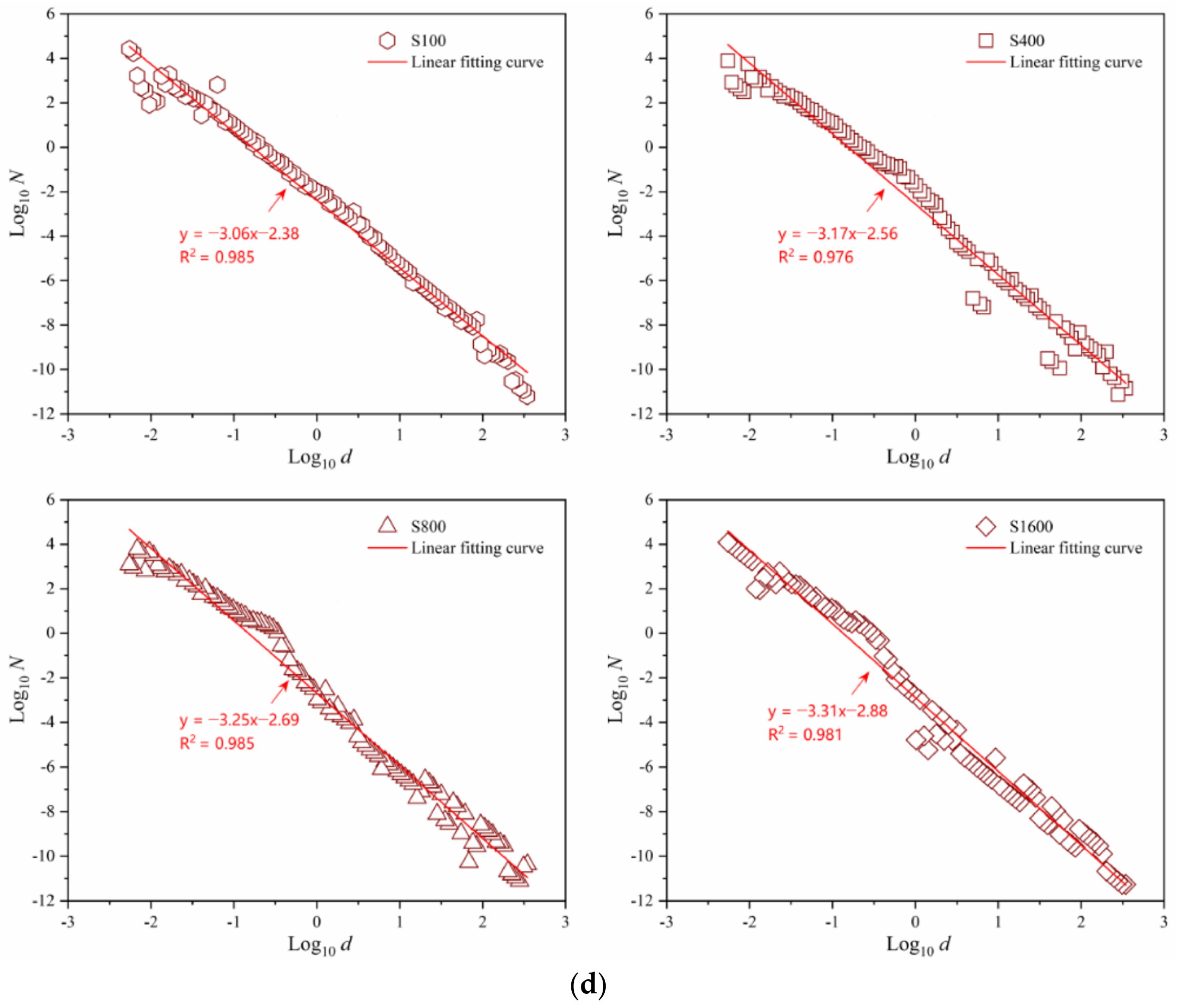
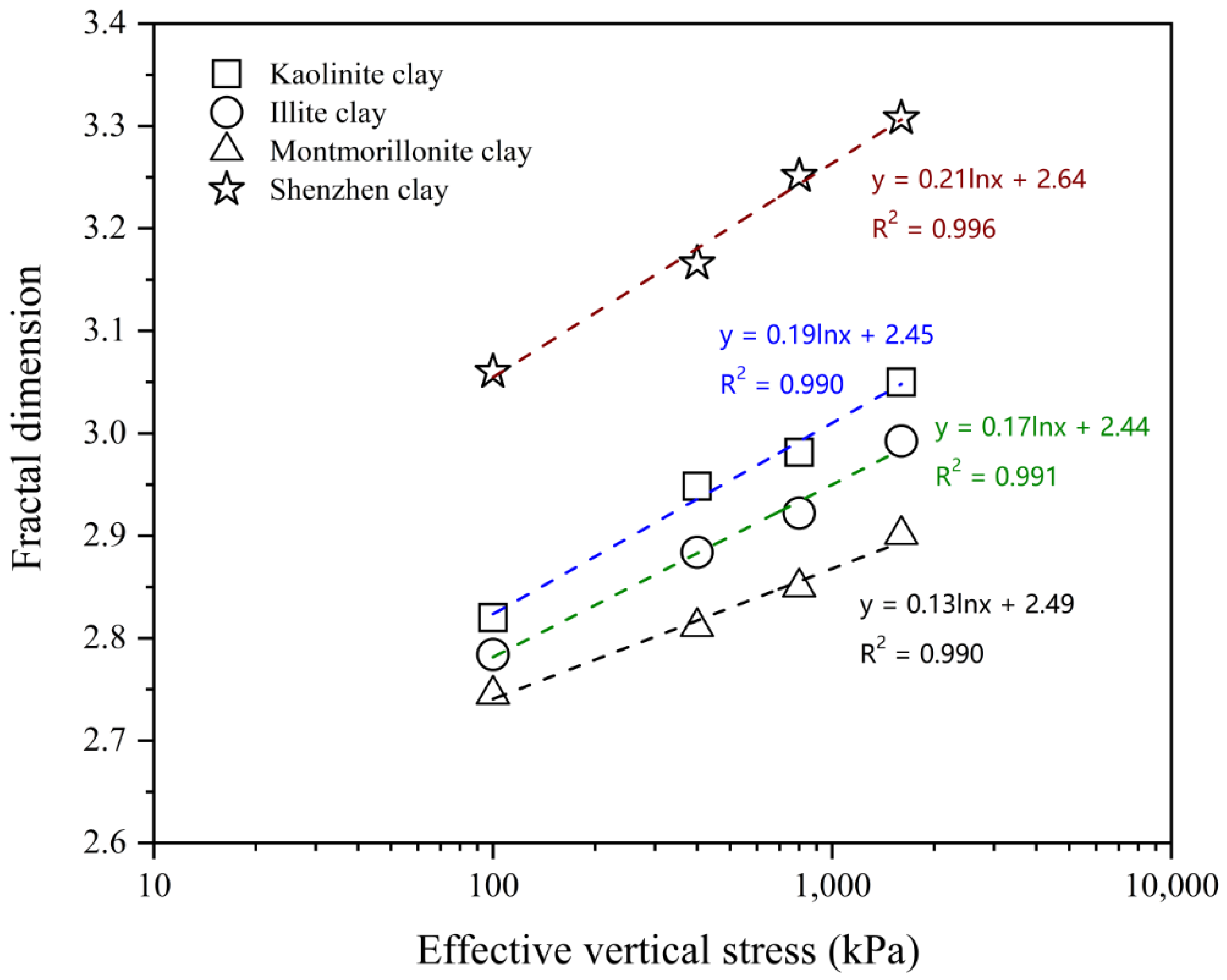
4.3. Evolution of the Fractal Dimension
5. Discussion
5.1. Response of Pore Size to 1D Loading
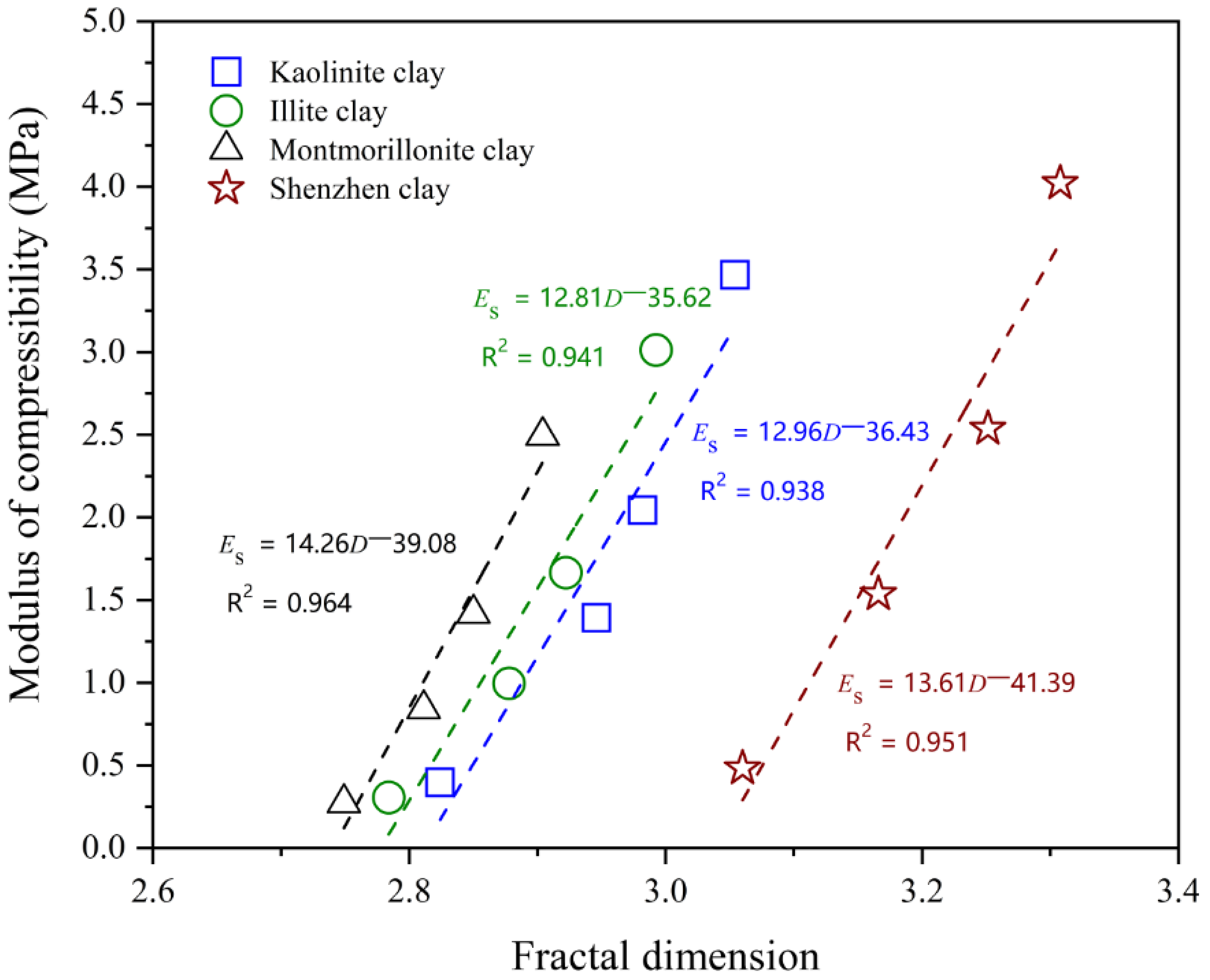
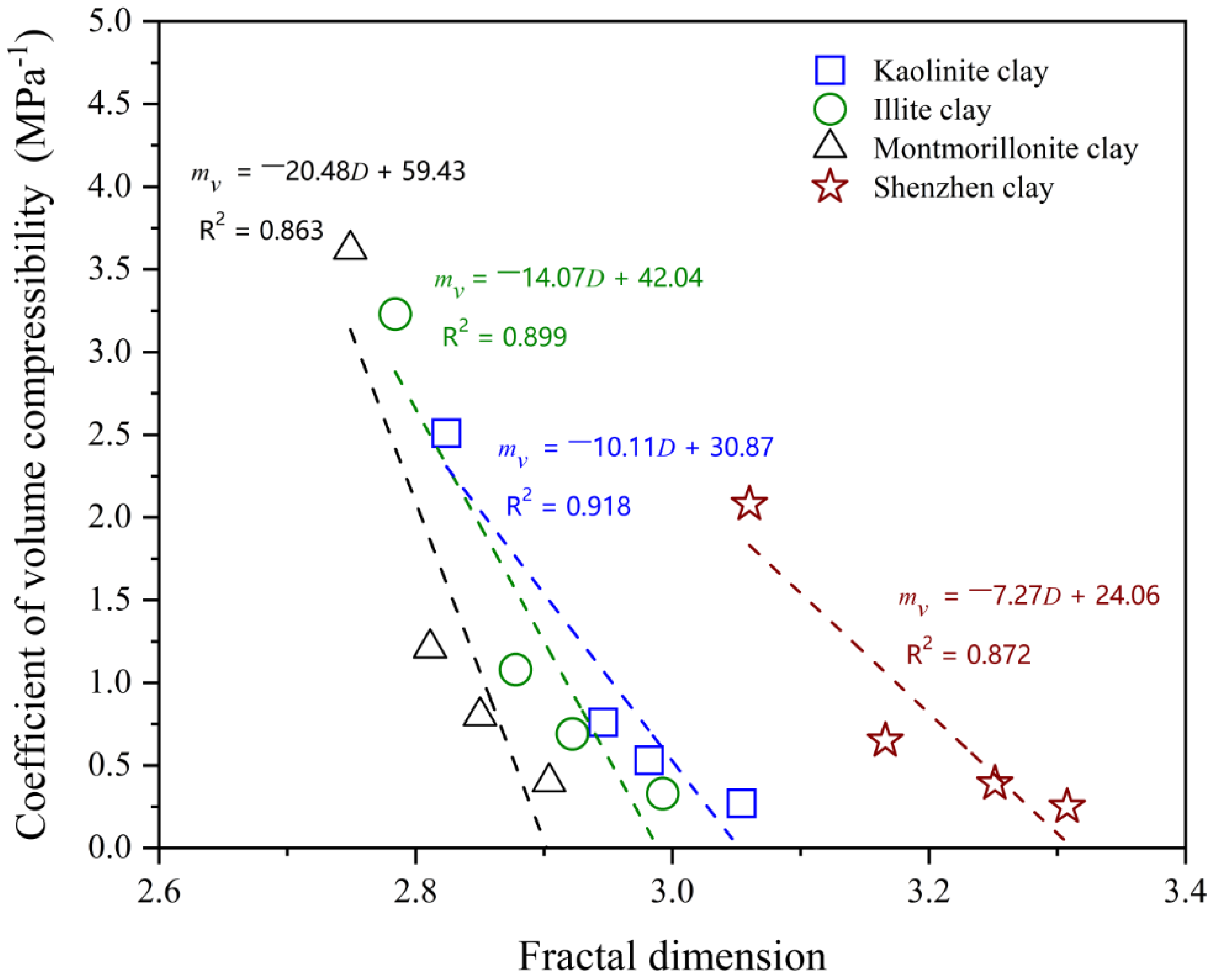
5.2. Relationship Between Fractal Dimension and 1D Compressibility
6. Conclusions
- (1)
- The 1D compressibility of four different reconstituted clays, including kaolinite clay, illite clay, montmorillonite clay, and Shenzhen clay, was systematically characterised, where montmorillonite clay exhibits the highest compressibility, while Shenzhen clay with chlorite as the dominant mineral demonstrates the lowest, revealing distinct compression behaviours linked to their mineralogical compositions. Correspondingly, the distinct pore-size evolution of each type of clay under 1D loading was revealed. This innovative observation highlights the importance of PSD in predicting 1D compressibility and emphasises the need to consider mineral composition when evaluating clay compression behaviour. It is found that mesopores (1–10 μm), typically corresponding to inter-aggregate pores, play a dominant role in the macroscopic deformation of clays, with their compression contributing significantly to the overall volume reduction during 1D compression. This insight highlights the critical influence of pore-size distribution and mineralogy on compressibility behaviour.
- (2)
- The PSD-based fractal dimension, D, was demonstrated to be an effective proxy for quantifying pore structure complexity. As 1D loading increased, the fractal dimension gradually rose, reflecting the increasing complexity and refinement of the pore structure in reconstituted clays during 1D compression. This change was found to follow a logarithmic trend, with the relationship being most pronounced in Shenzhen clay compared to other commercial soils, indicating its more intricate initial microstructure. The varying fractal dimension values of these four types of clay at the same stress level can be attributed to differences in the initial pore structures induced by the distinct mineral compositions, as evidenced by the SEM images.
- (3)
- The core and most innovative contribution of this study is the establishment of some robust mathematical relationships between the fractal dimension, D, and key compressibility parameters, namely Es and mv, for four different types of clays, each containing one of the four common clay minerals—kaolinite, illite, montmorillonite, and chlorite. These relationships were quantitatively described by the derived equations for each clay type, offering a predictive tool for estimating 1D compressibility based on fractal dimension. These findings not only provide a novel theoretical basis for future soil mechanics models, particularly valuable for pore-based constitutive models that rely on PSD data, but also serve as a significant advancement in understanding how microstructural characteristics, specifically pore structure complexity, influence macroscopic 1D compressibility of reconstituted clays.
- (4)
- The experimental protocols adopted in this study, ranging from precise control of the initial void ratio to MIP-based pore structure characterisation, were carefully designed to ensure the reliability and representativeness of the PSD data. These procedures provide valuable methodological references for future studies on the microstructural behaviour of clays under various loading conditions, including triaxial and cyclic shear tests.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Delage, P. A Microstructure Approach to the Sensitivity and Compressibility of Some Eastern Canada Sensitive Clays. Géotechnique 2010, 60, 353–368. [Google Scholar]
- Jia, R.; Lei, H.; Li, K. Compressibility and Microstructure Evolution of Different Reconstituted Clays during 1D Compression. Int. J. Geomech. 2020, 20, 04020181. [Google Scholar]
- Zheng, Y.; Baudet, B.A.; Delage, P.; Pereira, J.-M.; Sammonds, P. Pore Changes in an Illitic Clay during One-Dimensional Compression. Géotechnique 2023, 73, 917–932. [Google Scholar] [CrossRef]
- Zhong, B.; Zhu, Y.; Feng, G.; Xiang, J.; Wang, Y. Matrix Compression and Pore Heterogeneity in the Coal-Measure Shale Reservoirs of the Qinshui Basin: A Multifractal Analysis. Fractal Fract. 2024, 8, 580. [Google Scholar] [CrossRef]
- Sridharan, A.; Altschaeffl, A.G.; Diamond, S. Pore Size Distribution Studies. J. Soil Mech. Found. Div. 1971, 97, 771–787. [Google Scholar] [CrossRef]
- Delage, P.; Lefebvre, G. Study of the Structure of a Sensitive Champlain Clay and of Its Evolution during Consolidation. Can. Geotech. J. 1984, 21, 21–35. [Google Scholar] [CrossRef]
- Hicher, P.Y.; Wahyudi, H.; Tessier, D. Microstructural Analysis of Inherent and Induced Anisotropy in Clay. Mech. Cohesive-Frict. Mater. 2000, 5, 341–371. [Google Scholar] [CrossRef]
- Jadda, K.; Bag, R. The Fabric Evaluation of Two Indian Bentonites Subjected to Different Suctions and Consolidation Pressures. Eng. Geol. 2022, 298, 106535. [Google Scholar] [CrossRef]
- Mandelbrot, B. How Long Is the Coast of Britain? Statistical Self-Similarity and Fractional Dimension. Science 1967, 156, 636–638. [Google Scholar] [CrossRef]
- Song, C.; Havlin, S.; Makse, H.A. Origins of fractality in the growth of complex networks. Nat. Phys. 2006, 2, 275–281. [Google Scholar] [CrossRef]
- Carpinteri, A.; Mainardi, F. Fractals and Fractional Calculus in Continuum Mechanics; International Centre for Mechanical Sciences, Courses and Lectures; Springer Vienna: Vienna, Austria, 1997. [Google Scholar]
- Rivero, J.J.B. The fractal geometry of Luhmann’s sociological theory or debugging systems theory. Technol. Forecast. Soc. Change 2019, 146, 31–40. [Google Scholar]
- Hattaf, K. A New Class of Generalized Fractal and Fractal-Fractional Derivatives with Non-Singular Kernels. Fractal Fract. 2023, 7, 395. [Google Scholar] [CrossRef]
- Rieu, M.; Sposito, G. Fractal Fragmentation, Soil Porosity, and Soil Water Properties: I. Theory. Soil Sci. Soc. Am. J. 1991, 55, 1231–1238. [Google Scholar] [CrossRef]
- Pyun, S.I.; Rhee, C.K. An investigation of fractal characteristics of mesoporous carbon electrodes with various pore structures. Electrochim. Acta 2004, 49, 4171–4180. [Google Scholar] [CrossRef]
- Kang, X.; Li, H.; Zhang, G.; Li, S.; Shan, L.; Zhao, J.; Zhang, Z. A Binary Medium Constitutive Model for Frozen Solidified Saline Soil in Cold Regions and Its Fractal Characteristics Analysis. Fractal Fract. 2024, 8, 33. [Google Scholar] [CrossRef]
- De Oliveira, J.A.; Pires, L.F.; Cássaro, F.A.; Gaspareto, J.V.; Posadas, A.N.; Mooney, S.J. Soil pore system complexity and heterogeneity as affected by contrasting management practices. Soil Tillage Res. 2022, 224, 105497. [Google Scholar]
- Wang, L.; Zeng, X.; Yang, H.; Lv, X.; Guo, F.; Shi, Y.; Hanif, A. Investigation and application of fractal theory in cement-based materials: A review. Fractal Fract. 2021, 5, 247. [Google Scholar] [CrossRef]
- Tao, G.; Wu, X.; Xiao, H.; Chen, Q.; Cai, J. A Unified Fractal Model for Permeability Coefficient of Unsaturated Soil. Fractals 2019, 27, 1940012. [Google Scholar]
- Zhang, Y.; Zhong, X.; Lin, J.; Zhao, D.; Jiang, F.; Wang, M.-K.; Ge, H.; Huang, Y. Effects of Fractal Dimension and Water Content on the Shear Strength of Red Soil in the Hilly Granitic Region of Southern China. Geomorphology 2020, 351, 106956. [Google Scholar]
- Li, J.; Chen, H.; Gao, X.; Ding, Q.; Shan, W.; Guo, H.; Zhuo, J. Cracks Evolution and Micro Mechanism of Compacted Clay under Wet-Dry Cycles and Wet-Dry-Freeze-Thaw Cycles. Cold Reg. Sci. Technol. 2023, 214, 103944. [Google Scholar] [CrossRef]
- ASTM D854-23; Standard Test Methods for Specific Gravity of Soil Solids by the Water Displacement Method. ASTM International: West Conshohocken, PA, USA, 2023.
- ASTM D4318-17e1; Standard Test Methods for Liquid Limit, Plastic Limit, and Plasticity Index of Soils. ASTM International: West Conshohocken, PA, USA, 2017.
- Burland, J.B. On the Compressibility and Shear Strength of Natural Clays. Géotechnique 1990, 40, 329–378. [Google Scholar]
- Rocchi, I.; Coop, M.R. Experimental Accuracy of the Initial Specific Volume. Geotech. Test. J. 2014, 37, 169–175. [Google Scholar]
- Zheng, Y.; Baudet, B.A. Evolution of Nano-Pores in Illite-Dominant Clay during Consolidation. Acta Geotech. 2024, 19, 71–83. [Google Scholar]
- Washburn, E.W. The Dynamics of Capillary Flow. Phys. Rev. 1921, 17, 273–283. [Google Scholar]
- Diamond, S. Pore Size Distributions in Clays. Clays Clay Miner. 1970, 18, 7–23. [Google Scholar]
- Pachepsky, Y.A.; Korsunskaia, L.P.; Hajnos, M. Fractal parameters of soil pore surface area under a developing crop. Fractals 1996, 4, 97–104. [Google Scholar]
- Ju, X.; Jia, Y.; Li, T.; Gao, L.; Gan, M. Morphology and multifractal characteristics of soil pores and their functional implication. Catena 2021, 196, 104822. [Google Scholar]
- Liu, Y.; Deng, H.; Wang, P.; Yu, S. Analysis of pore structure characteristics and strength prediction model of coarse-grained soil based on fractal theory. Environ. Earth Sci. 2023, 82, 592. [Google Scholar]
- Tricot, C. Curves and Fractal Dimension; Springer: New York, NY, USA, 1995. [Google Scholar]
- Griffiths, F.J.; Joshi, R.C. Change in Pore Size Distribution Due to Consolidation of Clays. Géotechnique 1989, 39, 159–167. [Google Scholar]
- Jalal, F.E.; Xu, Y.; Li, X.; Jamhiri, B.; Iqbal, M. Fractal approach in expansive clay-based materials with special focus on compacted GMZ bentonite in nuclear waste disposal: A systematic review. Environ. Sci. Pollut. Res. 2021, 28, 43287–43314. [Google Scholar] [CrossRef]
- Mitchell, J.K. Fundamentals of Soil Behavior, 2nd ed.; Wiley: New York, NY, USA, 1993. [Google Scholar]
- Schofield, A.N.; Wroth, P. Critical State Soil Mechanics; McGraw-Hill: London, UK, 1968; Volume 310. [Google Scholar]
- Callisto, L.; Rampello, S. An interpretation of structural degradation for three natural clays. Can. Geotech. J. 2004, 41, 392–407. [Google Scholar] [CrossRef]
- Fathalikhani, M.; Graham, J.; Kurz, D.; Maghoul, P. Investigation and modification of a CSSM-based elastic–thermoviscoplastic model for clay. Int. J. Geomech. 2022, 22, 04022161. [Google Scholar] [CrossRef]




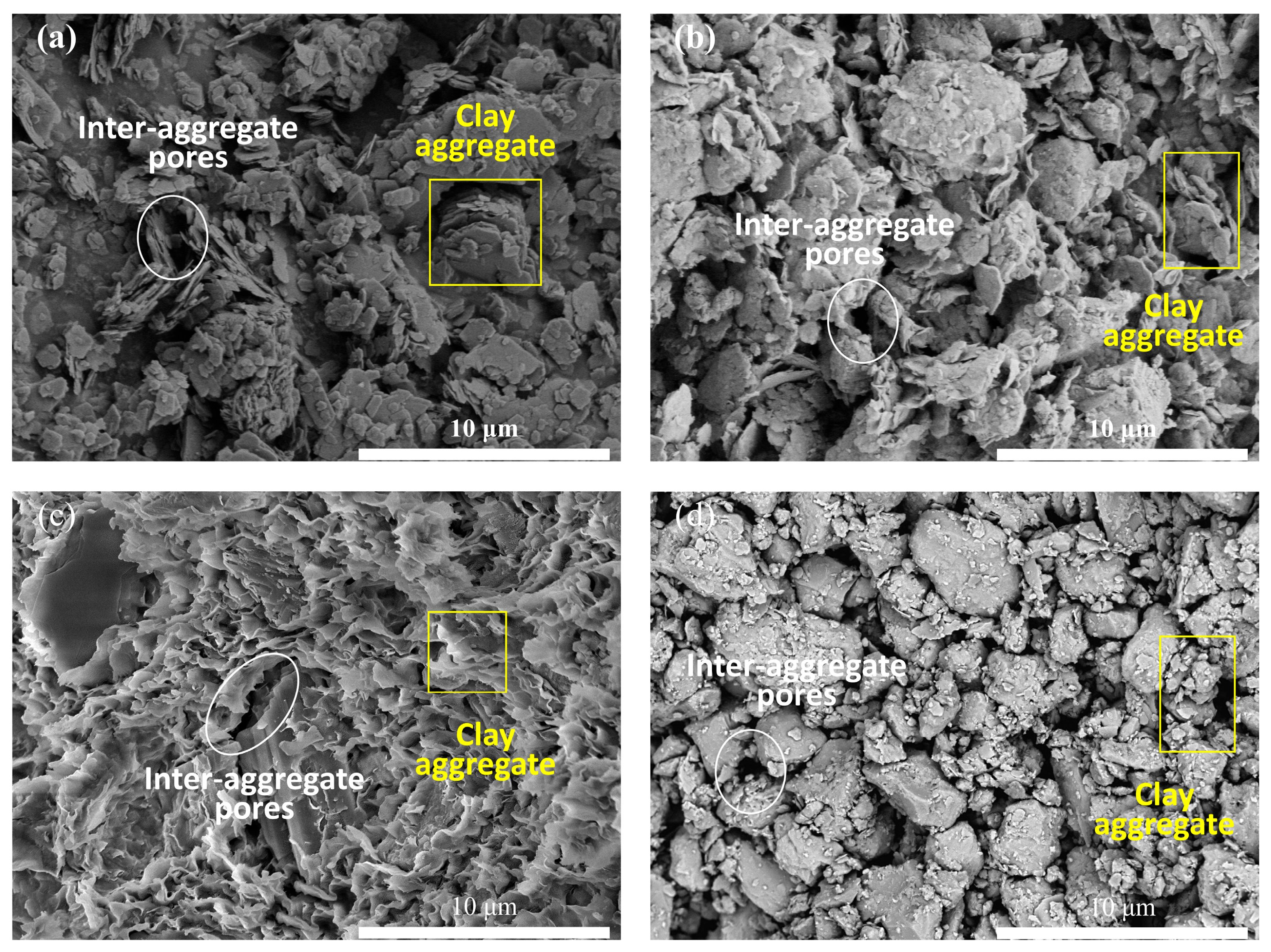
| Kaolinite Clay | Illite Clay | Montmorillonite Clay | Shenzhen Clay | |
|---|---|---|---|---|
| Kaolinite (%) | 92.5 | 0 | 0 | 0 |
| Illite (%) | 1.2 | 86.2 | 21.5 | 19.5 |
| Montmorillonite (%) | 0 | 0 | 51.7 | 1.5 |
| Chlorite (%) | 0 | 0 | 0 | 48.4 |
| Quartz (%) | 5.6 | 12.5 | 19.6 | 17.3 |
| Calcite (%) | 0 | 0 | 7.2 | 9.6 |
| Micaceous minerals (%) | 1.9 | 0 | 0 | 3.7 |
| Feldspar minerals (%) | 0 | 1.3 | 6.5 | 0 |
| Kaolinite Clay | Illite Clay | Montmorillonite Clay | Shenzhen Clay | |
|---|---|---|---|---|
| Specific gravity, G_s | 2.59 | 2.64 | 2.35 | 2.78 |
| Nature water content, w_n (%) | - | - | - | 52.6 |
| Liquid limit, w_L (%) | 60.2 | 65.8 | 106.5 | 48.4 |
| Plastic limit, w_P (%) | 27.3 | 27.5 | 28.4 | 22.8 |
| Plasticity index (PI) (%) | 32.9 | 38.3 | 78.1 | 25.6 |
| USCS classification * | CH | CH | CE | CI |
| Kaolinite clay | Illite clay | Montmorillonite clay | Shenzhen clay | |
| Specific gravity, G_s | 2.59 | 2.64 | 2.35 | 2.78 |
| Test No. | Maximum Stress Level (kPa) | Water Content, w (%) * | Saturation, Sr (%) | Initial Void Ratio, ei | Final Void Ratio | |||
|---|---|---|---|---|---|---|---|---|
| Equation (1) | Equation (2) | Equation (3) | Chosen Value ** | |||||
| K0 | — | 71.38 | 99.2 | 1.876 | 1.885 | 1.882 | 1.88 | — |
| K100 | 100 | 45.83 | 100 | 1.877 | 1.884 | 1.883 | 1.88 | 1.16 |
| K400 | 400 | 35.25 | 100 | 1.878 | 1.883 | 1.881 | 1.88 | 0.89 |
| K800 | 800 | 30.13 | 100 | 1.875 | 1.887 | 1.880 | 1.88 | 0.75 |
| K1600 | 1600 | 24.85 | 100 | 1.879 | 1.882 | 1.883 | 1.88 | 0.62 |
| I0 | — | 78.15 | 98.7 | 2.079 | 2.081 | 2.080 | 2.08 | — |
| I100 | 100 | 42.83 | 100 | 2.081 | 2.083 | 2.081 | 2.08 | 1.08 |
| I400 | 400 | 30.08 | 100 | 2.078 | 2.082 | 2.081 | 2.08 | 0.76 |
| I800 | 800 | 23.72 | 100 | 2.077 | 2.080 | 2.079 | 2.08 | 0.61 |
| I1600 | 1600 | 17.95 | 100 | 2.080 | 2.082 | 2.080 | 2.08 | 0.45 |
| M0 | — | 147.35 | 100 | 3.163 | 3.169 | 3.171 | 3.17 | — |
| M100 | 100 | 85.24 | 100 | 3.17 | 3.171 | 3.164 | 3.17 | 1.84 |
| M400 | 400 | 52.28 | 100 | 3.159 | 3.175 | 3.169 | 3.17 | 1.13 |
| M800 | 800 | 34.86 | 100 | 3.169 | 3.169 | 3.166 | 3.17 | 0.82 |
| M1600 | 1600 | 25.32 | 100 | 3.161 | 3.172 | 3.171 | 3.17 | 0.49 |
| S0 | — | 58.16 | 97.5 | 1.54 | 1.556 | 1.552 | 1.55 | — |
| S100 | 100 | 37.68 | 100 | 1.542 | 1.557 | 1.548 | 1.55 | 1.02 |
| S400 | 400 | 31.42 | 100 | 1.548 | 1.551 | 1.549 | 1.55 | 0.80 |
| S800 | 800 | 25.96 | 100 | 1.544 | 1.554 | 1.55 | 1.55 | 0.69 |
| S1600 | 1600 | 21.37 | 100 | 1.545 | 1.552 | 1.551 | 1.55 | 0.58 |
| Test No. | Vertical Loading (kPa) | D | Es (MPa) | mv (MPa−1) |
|---|---|---|---|---|
| K100 | 0→100 | 2.82 | 0.40 | 2.51 |
| K400 | 0→400 | 2.95 | 1.39 | 0.72 |
| K800 | 0→800 | 2.98 | 2.04 | 0.49 |
| K1600 | 0→1600 | 3.05 | 3.47 | 0.29 |
| I100 | 0→100 | 2.78 | 0.31 | 3.23 |
| I400 | 0→400 | 2.88 | 1.00 | 1.00 |
| I800 | 0→800 | 2.92 | 1.67 | 0.60 |
| I1600 | 0→1600 | 2.99 | 3.01 | 0.33 |
| M100 | 0→100 | 2.75 | 0.27 | 3.62 |
| M400 | 0→400 | 2.81 | 0.84 | 1.19 |
| M800 | 0→800 | 2.85 | 1.42 | 0.70 |
| M1600 | 0→1600 | 2.90 | 2.49 | 0.40 |
| S100 | 0→100 | 3.06 | 0.48 | 2.08 |
| S400 | 0→400 | 3.17 | 1.54 | 0.65 |
| S800 | 0→800 | 3.25 | 2.54 | 0.39 |
| S1600 | 0→1600 | 3.31 | 4.02 | 0.25 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zheng, Y.; Zhu, T.; Chen, J.; Shan, K.; Li, J. Relationship Between Pore-Size Distribution and 1D Compressibility of Different Reconstituted Clays Based on Fractal Theory. Fractal Fract. 2025, 9, 235. https://doi.org/10.3390/fractalfract9040235
Zheng Y, Zhu T, Chen J, Shan K, Li J. Relationship Between Pore-Size Distribution and 1D Compressibility of Different Reconstituted Clays Based on Fractal Theory. Fractal and Fractional. 2025; 9(4):235. https://doi.org/10.3390/fractalfract9040235
Chicago/Turabian StyleZheng, Yanhao, Tanfang Zhu, Junqi Chen, Kun Shan, and Junru Li. 2025. "Relationship Between Pore-Size Distribution and 1D Compressibility of Different Reconstituted Clays Based on Fractal Theory" Fractal and Fractional 9, no. 4: 235. https://doi.org/10.3390/fractalfract9040235
APA StyleZheng, Y., Zhu, T., Chen, J., Shan, K., & Li, J. (2025). Relationship Between Pore-Size Distribution and 1D Compressibility of Different Reconstituted Clays Based on Fractal Theory. Fractal and Fractional, 9(4), 235. https://doi.org/10.3390/fractalfract9040235





