Entanglement Witness for the Weak Equivalence Principle
Abstract
:1. Introduction
2. QGEM Scheme
3. Entanglement Entropy
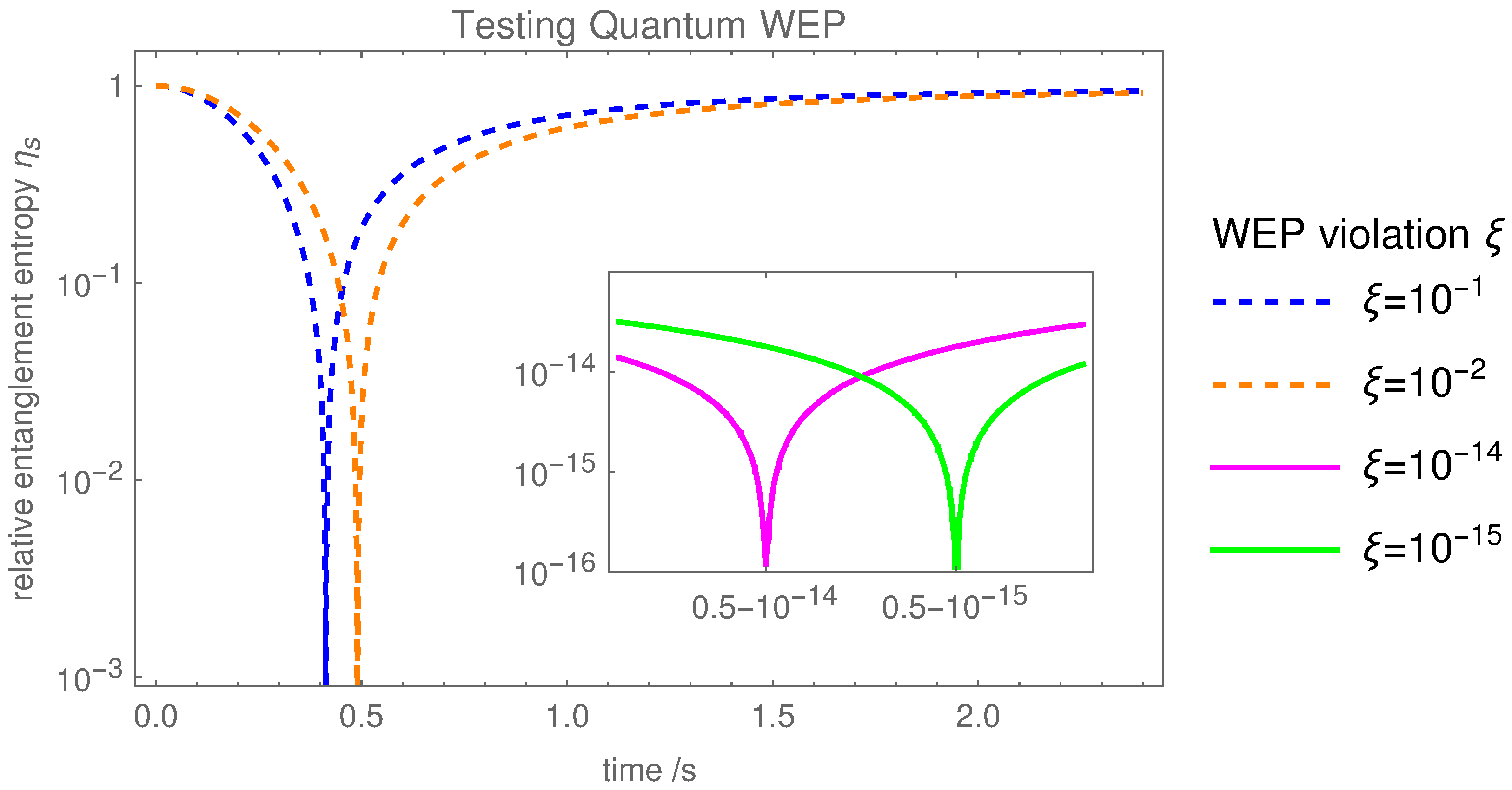
4. EEWEP
5. Discussion
Author Contributions
Funding
Conflicts of Interest
Abbreviations
| WEP | Weak equivalence principle |
| EEWEP | Entanglement entropy weak equivalence principle |
| QGEM | Quantum-gravity-induced entanglement of masses |
| LLI | Local Lorenz invariance |
| LPI | Local Position invariance |
| EEP | Einstein equivalence principle |
| COW | Collela-Overhauser-Werner |
References
- Will, C.M. The confrontation between general relativity and experiment. Living Rev. Relativ. 2014, 17, 1–117. [Google Scholar] [CrossRef] [Green Version]
- Dicke, R.H. Experimental relativity. Relativ. Groups Topol. Relativ. Topol. 1964, 165–313. [Google Scholar]
- Touboul, P.; Métris, G.; Rodrigues, M.; André, Y.; Baghi, Q.; Bergé, J.; Boulanger, D.; Bremer, S.; Chhun, R.; Visser, P.; et al. Space test of the Equivalence Principle: First results of the MICROSCOPE mission. Class. Quant. Grav. 2019, 36, 225006. [Google Scholar] [CrossRef] [Green Version]
- Prestage, J.D.; Bollinger, J.J.; Itano, W.M.; Wineland, D.J. Limits for spatial anisotropy by use of nuclear-spin-polarized Be+ 9 ions. Phys. Rev. Lett. 1985, 54, 2387. [Google Scholar] [CrossRef] [PubMed]
- Lamoreaux, S.K.; Jacobs, J.P.; Heckel, B.R.; Raab, F.J.; Fortson, E.N. New Limits on Spatial Anisotropy from Optically Pumped Hg 201 and Hg 199. Phys. Rev. Lett. 1987, 58, 746. [Google Scholar] [CrossRef]
- Chupp, T.E.; Hoare, R.J.; Loveman, R.A.; Oteiza, E.R.; Richardson, J.M.; Wagshul, M.E.; Thompson, A.K. Results of a new test of local Lorentz invariance: A search for mass anisotropy in Ne 21. Phys. Rev. Lett. 1989, 631541. [Google Scholar]
- Steven, P.; Crane, S.; Hanssen, J.L.; Swanson, T.B.; Ekstrom, C.R. Tests of local position invariance using continuously running atomic clocks. Phys. Rev. A 2013, 87, 010102. [Google Scholar]
- Giulini, D. Equivalence principle, quantum mechanics, and atom-interferometric tests. In Quantum Field Theory and Gravity; Springer: Basel, Switzerland, 2012; pp. 345–370. [Google Scholar]
- Colella, H.; Overhauser, A.W.; Werner, S.A. Observation of Gravitationally Induced Quantum Interference. Phys. Rev. Lett. 1975, 34, 23. [Google Scholar] [CrossRef]
- Aharonov, Y.; Carmi, G. Quantum aspects of the equivalence principle. Found. Phys. 1973, 3, 493–498. [Google Scholar] [CrossRef]
- Hohensee, M.A.; Estey, B.; Hamilton, P.; Zeilinger, A.; Müller, H. Force-free gravitational redshift: Proposed gravitational Aharonov-Bohm experiment. Phys. Rev. Lett. 2012, 108, 230404. [Google Scholar] [CrossRef] [Green Version]
- Overstreet, C.; Asenbaum, P.; Curti, J.; Kim, M.; Kasevich, M.A. Observation of a gravitational Aharonov-Bohm effect. Science 2022, 375, 226–229. [Google Scholar] [CrossRef]
- Lämmerzahl, C. On the equivalence principle in quantum theory. Gen. Relativ. Gravit. 1996, 28, 1043–1070. [Google Scholar] [CrossRef] [Green Version]
- Viola, L.; Onofrio, R. Testing the equivalence principle through freely falling quantum objects. Phys. Rev. D 1997, 55, 455. [Google Scholar] [CrossRef] [Green Version]
- Seveso, L.; Peri, V.; Paris, M.G.A. Does universality of free-fall apply to the motion of quantum probes? J. Phys. 2017, 880, 012067. [Google Scholar] [CrossRef] [Green Version]
- Seveso, L.; Peri, V.; Paris, M.G.A. Can quantum probes satisfy the weak equivalence principle? Ann. Phys. 2017, 380, 213. [Google Scholar] [CrossRef] [Green Version]
- Seveso, L.; Peri, V.; Paris, M.G.A. Quantum limits to mass sensing in a gravitational field. J. Phys. A 2017, 50, 235301. [Google Scholar] [CrossRef]
- Rosi, G.; D’Amico, G.; Cacciapuoti, L.; Sorrentino, F.; Prevedelli, M.; Zych, M.; Brukner, C.; Tino, G. Quantum test of the equivalence principle for atoms in coherent superposition of internal energy states. Nat. Commun. 2017, 8, 116. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Anastopoulos, C.; Hu, B.L. Equivalence principle for quantum systems: Dephasing and phase shift of free-falling particles. Class. Quant. Grav. 2018, 35, 035011. [Google Scholar] [CrossRef] [Green Version]
- Dimopoulos, S.; Graham, P.W.; Hogan, J.M.; Kasevich, M.A. Testing general relativity with atom interferometry. Phys. Rev. Lett. 2007, 98, 111102. [Google Scholar] [CrossRef] [Green Version]
- Roura, A. Circumventing Heisenberg’s Uncertainty Principle in Atom Interferometry Tests of the Equivalence Principle. Phys. Rev. Lett. 2017, 118, 160401. [Google Scholar] [CrossRef] [Green Version]
- Asenbaum, P.; Overstreet, C.; Kim, M.; Curti, J.; Kasevich, M.A. Atom-Interferometric Test of the Equivalence Principle at the 10-12 Level. Phys. Rev. Lett. 2020, 125, 191101. [Google Scholar] [CrossRef] [PubMed]
- Overstreet, C.; Asenbaum, P.; Kovachy, T.; Notermans, R.; Hogan, J.M.; Kasevich, M.A. Effective inertial frame in an atom interferometric test of the equivalence principle. Phys. Rev. Lett. 2018, 120, 183604. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Penrose, R. On gravity’s role in quantum state reduction. Gen. Rel. Grav. 1996, 28, 581. [Google Scholar] [CrossRef] [Green Version]
- Penrose, R. Quantum computation, entanglement and state reduction. Philos. Trans. R. Soc. Lond. A 1998, 356, 1927. [Google Scholar] [CrossRef]
- Hardy, L. Implementation of the Quantum Equivalence Principle. In Progress and Visions in Quantum Theory in view of Gravity: Bridging Foundations of Physics and Mathematics; Springer International Publishing: Cham, Switzerland, 2020; pp. 189–220. [Google Scholar]
- Giacomini, F.; Brukner, Č. Einstein’s Equivalence principle for superpositions of gravitational fields. arXiv 2012, arXiv:2012.13754. [Google Scholar]
- Giacomini, F.; Castro-Ruiz, E.; Brukner, Č. Quantum mechanics and the covariance of physical laws in quantum reference frames. Nat. Commun. 2019, 10, 494. [Google Scholar] [CrossRef] [Green Version]
- Zych, M.; Brukner, Č. Quantum formulation of the Einstein Equivalence Principle. Nat. Phys. 2018, 14, 10271031. [Google Scholar] [CrossRef] [Green Version]
- Marletto, C.; Vedral, V. On the Testability of the Equivalence Principle as a Gauge Principle Detecting the Gravitational t3 Phase. Front. Phys. 2020, 8, 176. [Google Scholar] [CrossRef]
- Pipa, F.; Paunković, N.; Vojinović, M. Entanglement-induced deviation from the geodesic motion in quantum gravity. JCAP 2019, 9, 57. [Google Scholar] [CrossRef] [Green Version]
- Paunkovic, N.; Vojinovic, M. Equivalence Principle in Classical and Quantum Gravity. Universe 2022, 8, 598. [Google Scholar] [CrossRef]
- Bose, S.; Mazumdar, A.; Morley, G.W.; Ulbricht, H.; Toroš, M.; Paternostro, M.; Geraci, A.; Barker, P.; Kim, M.S.; Milburn, G. Spin Entanglement Witness for Quantum Gravity. Phys. Rev. Lett. 2017, 119, 240401. [Google Scholar] [CrossRef] [Green Version]
- Marshman, R.J.; Mazumdar, A.; Bose, S. Locality and entanglement in table-top testing of the quantum nature of linearized gravity. Phys. Rev. A 2020, 101, 052110. [Google Scholar] [CrossRef]
- Bose, S.; Mazumdar, A.; Schut, M.; Toroš, M. Mechanism for the quantum natured gravitons to entangle masses. Phys. Rev. D 2022, 105, 106028. [Google Scholar] [CrossRef]
- Marletto, C.; Vedral, V. Gravitationally induced entanglement between two massive particles is sufficient evidence of quantum effects in gravity. Phys. Rev. Lett. 2017, 119, 240402. [Google Scholar] [CrossRef] [Green Version]
- Kiefer, C. Quantum Gravity; Oxford University Press: Oxford, UK, 2007. [Google Scholar]
- Gupta, S.N. Quantization of Einstein’s Gravitational Field: Linear Approximation. Proc. Phys. Soc. A 1952, 65, 161. [Google Scholar] [CrossRef]
- Gupta, S.N. Gravitation and electromagnetism. Phys. Rev. 1954, 96, 1683. [Google Scholar] [CrossRef]
- Donoghue, J.F. General relativity as an effective field theory: The leading quantum corrections. Phys. Rev. D 1994, 50, 3874–3888. [Google Scholar] [CrossRef] [Green Version]
- Danielson, D.L.; Satishchandran, G.; Wald, R.M. Left Gravitationally mediated entanglement: Newtonian field versus gravitons. Phys. Rev. D 2022, 105, 086001. [Google Scholar] [CrossRef]
- Christodoulou, M.; Di Biagio, A.; Aspelmeyer, M.; Brukner, Č.; Rovelli, C.; Howl, R. Locally mediated entanglement through gravity from first principles. arXiv 2022, arXiv:2202.03368. [Google Scholar]
- Bennett, C.H.; DiVincenzo, D.P.; Smolin, J.A.; Wootters, W.K. Mixed-state entanglement and quantum error correction. Phys. Rev. A 1996, 54, 3824. [Google Scholar] [CrossRef] [Green Version]
- Marshman, R.J.; Mazumdar, A.; Folman, R.; Bose, S. Constructing nano-object quantum superpositions with a Stern-Gerlach interferometer. Phys. Rev. Res. 2022, 4, 023087. [Google Scholar] [CrossRef]
- Zhou, R.; Marshman, R.J.; Bose, S.; Mazumdar, A. Catapulting towards massive and large spatial quantum superposition. Phys. Rev. Res. 2022, 4, 043157. [Google Scholar] [CrossRef]
- Sakurai, J.J.; Commins, E.D. Modern Quantum Mechanics; Cambridge University Press: Cambridge, UK, 1995. [Google Scholar]
- Rauch, H.; Werner, S.A. Neutron Interferometry: Lessons in Experimental Quantum Mechanics, Wave-Particle Duality, and Entanglement; Oxford University Press: New York, NY, USA, 2015; Volume 12. [Google Scholar]
- Boulder Atomic Clock Optical Network (BACON) Collaboration. Frequency ratio measurements at 18-digit accuracy using an optical clock network. Nature 2021, 591, 564–569. [Google Scholar] [CrossRef]
- Mourou, G.; Stancampiano, C.V.; Blumenthal, D. Picosecond microwave pulse generation. Appl. Phys. Lett. 1981, 38, 2. [Google Scholar] [CrossRef] [Green Version]
- Kim, J.; Cox, J.A.; Chen, J.; Kärtner, F.X. Drift-free femtosecond timing synchronization of remote optical and microwave sources. Nat. Photon. 2008, 2, 733. [Google Scholar] [CrossRef]
- Tarallo, M.G.; Mazzoni, T.; Poli, N.; Sutyrin, D.V.; Zhang, X.; Tino, G.M. Test of Einstein Equivalence Principle for 0-spin and half-integer-spin atoms: Search for spin-gravity coupling effects. Phys. Rev. Lett. 2014, 113, 023005. [Google Scholar] [CrossRef] [Green Version]
- Schlippert, D.; Hartwig, J.; Albers, H.; Richardson, L.L.; Schubert, C.; Roura, A.; Schleich, W.P.; Ertmer, W.; Rasel, E.M. Quantum Test of the Universality of Free Fall. Phys. Rev. Lett. 2014, 112, 203002. [Google Scholar] [CrossRef] [Green Version]
- Fray, S.; Alvarez Diez, C.; Hansch, T.W.; Weitz, M. Atomic interferometer with amplitude gratings of light and its applications to atom based tests of the equivalence principl. Phys. Rev. Lett. 2004, 93, 240404. [Google Scholar] [CrossRef] [Green Version]
- Van de Kamp, T.W.; Marshman, R.J.; Bose, S.; Mazumdar, A. Quantum Gravity Witness via Entanglement of Masses: Casimir Screening. Phys. Rev. A 2020, 102, 062807. [Google Scholar] [CrossRef]
- Bose, S.; Mazumdar, A.; Toroš, M. Gravitons in a box. Phys. Rev. D 2021, 104, 066019. [Google Scholar] [CrossRef]
- Bose, S.; Mazumdar, A.; Toroš, M. Infrared scaling for a graviton condensate. Nucl. Phys. B 2022, 977, 115730. [Google Scholar] [CrossRef]
- Toroš, M.; Mazumdar, A.; Bose, S. Loss of coherence of matter-wave interferometer from fluctuating graviton bath. arXiv 2020, arXiv:2008.08609. [Google Scholar]
- De Sabbata, V.; Gasperini, M. Introduction to Gravitation; World Scientific Publishing Company: Singapore, 1986. [Google Scholar]
- Delic, U.; Reisenbauer, M.; Dare, K.; Grass, D.; Vuletić, V.; Kiesel, N.; Aspelmeyer, M. Cooling of a levitated nanoparticle to the motional quantum ground state. Science 2020, 367, 892. [Google Scholar] [CrossRef] [Green Version]
- Magrini, L.; Rosenzweig, P.; Bach, C.; Deutschmann-Olek, A.; Hofer, S.G.; Hong, S.; Kiesel, N.; Kugi, A.; Aspelmeyer, M. Real-time optimal quantum control of mechanical motion at room temperature. Nature 2021, 595, 373–377. [Google Scholar] [CrossRef]
- Tebbenjohanns, F.; Mattana, M.L.; Rossi, M.; Frimmer, M.; Novotny, L. Quantum control of a nanoparticle optically levitated in cryogenic free space. Nature 2021, 595, 378–382. [Google Scholar] [CrossRef]
- Scala, M.; Kim, M.S.; Morley, G.W.; Barker, P.F.; Bose, S. Matter-Wave Interferometry of a Levitated Thermal Nano-Oscillator Induced and Probed by a Spin. Phys. Rev. Lett. 2013, 111, 180403. [Google Scholar] [CrossRef] [Green Version]
- Wan, C.; Scala, M.; Morley, G.W.; Rahman, A.A.; Ulbricht, H.; Bateman, J.; Barker, P.F.; Bose, S.; Kim, M.S. Free Nano-Object Ramsey Interferometry for Large Quantum Superpositions. Phys. Rev. Lett. 2016, 117, 143003. [Google Scholar] [CrossRef] [Green Version]
- Margalit, Y.; Dobkowski, O.; Zhou, Z.; Amit, O.; Japha, Y.; Moukouri, S.; Rohrlich, D.; Mazumdar, A.; Bose, S.; Henkel, C.; et al. Realization of a complete Stern-Gerlach interferometer: Towards a test of quantum gravity. Sci. Adv. 2021, 7, 22. [Google Scholar] [CrossRef]
- Machluf, S.; Japha, Y.; Folman, R. Coherent Stern–Gerlach momentum splitting on an atom chip. Nat. Commun. 2013, 4, 2424. [Google Scholar] [CrossRef] [Green Version]
- Zhou, Z.; Margalit, Y.; Moukouri, S.; Meir, Y.; Folman, R. An experimental test of the geodesic rule proposition for the noncyclic geometric phase. Sci. Adv. 2020, 6, eaay8345. [Google Scholar] [CrossRef] [Green Version]
- Margalit, Y.; Zhou, Z.; Machluf, S.; Japha, Y.; Moukouri, S.; Folman, R. Analysis of a high-stability Stern–Gerlach spatial fringe interferometer. New J. Phys. 2019, 21, 073040. [Google Scholar] [CrossRef]
- Pedernales, J.S.; Morley, G.W.; Plenio, M.B. Motional Dynamical Decoupling for Interferometry with Macroscopic Particles. Phys. Rev. Lett. 2020, 125, 023602. [Google Scholar] [CrossRef] [PubMed]
- Romero-Isart, O. Quantum superposition of massive objects and collapse models. Phys. Rev. A 2011, 84, 052121. [Google Scholar] [CrossRef] [Green Version]
- Tilly, J.; Marshman, R.J.; Mazumdar, A.; Bose, S. Qudits for witnessing quantum-gravity-induced entanglement of masses under decoherence. Phys. Rev. A 2021, 104, 052416. [Google Scholar] [CrossRef]
- Schut, M.; Tilly, J.; Marshman, R.J.; Bose, S.; Mazumdar, A. Improving resilience of the Quantum Gravity Induced Entanglement of Masses (QGEM) to decoherence using 3 superpositions. Phys. Rev. A 2022, 105, 032411. [Google Scholar] [CrossRef]
- Gunnink, F.; Mazumdar, A.; Schut, M.; Toroš, M. Gravitational decoherence by the apparatus in the quantum-gravity induced entanglement of masses. arXiv 2022, arXiv:2210.16919. [Google Scholar]
- Torrieri, G. The equivalence principle and inertial-gravitational decoherence. arXiv 2023, arXiv:2210.08586. [Google Scholar]
- Toroš, M.; Van De Kamp, T.W.; Marshman, R.J.; Kim, M.S.; Mazumdar, A.; Bose, S. Relative acceleration noise mitigation for nanocrystal matter-wave interferometry: Applications to entangling masses via quantum gravity. Phys. Rev. Res. 2021, 3, 023178. [Google Scholar] [CrossRef]
- Damour, T. Theoretical Aspects of the Equivalence Principle. Class. Quant. Grav. 2012, 29, 184001. [Google Scholar] [CrossRef]
- Brans, C.H.; Dicke, R.H. Mach’s Principle and a Relativistic Theory of Gravitation. Phys. Rev. 1961, 124, 925. [Google Scholar] [CrossRef]
- Mende, P.F. String theory at short distance and the principle of equivalence. arXiv 1992, arXiv:hep-th/9210001. [Google Scholar]
- Damour, T.; Piazza, F.; Veneziano, G. Runaway dilaton and equivalence principle violations. Phys. Rev. Lett. 2002, 89, 081601. [Google Scholar] [CrossRef] [Green Version]
- Van Nieuwenhuizen, P. On ghost-free tensor lagrangians and linearized gravitation. Nucl. Phys. 1973, B60, 478. [Google Scholar] [CrossRef]
- Biswas, T.; Koivisto, T.; Mazumdar, A. Nonlocal theories of gravity: The flat space propagator. arXiv 2013, arXiv:1302.0532. [Google Scholar]
- Biswas, T.; Gerwick, E.; Koivisto, T.; Mazumdar, A. Towards singularity and ghost free theories of gravity. Phys. Rev. Lett. 2012, 108, 031101. [Google Scholar] [CrossRef] [Green Version]
- Biswas, T.; Mazumdar, A.; Siegel, W. Bouncing universes in string-inspired gravity. JCAP 2006, 3, 9. [Google Scholar] [CrossRef]
- Tomboulis, E.T. Superrenormalizable gauge and gravitational theories. arXiv 1997, arXiv:hep-th/9702146. [Google Scholar]
- Modesto, L. Super-renormalizable Quantum Gravity. Phys. Rev. D 2012, 86, 044005. [Google Scholar] [CrossRef] [Green Version]
- Edholm, J.; Koshelev, A.S.; Mazumdar, A. Behavior of the Newtonian potential for ghost-free gravity and singularity-free gravity. Phys. Rev. D 2016, 94, 104033. [Google Scholar] [CrossRef] [Green Version]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bose, S.; Mazumdar, A.; Schut, M.; Toroš, M. Entanglement Witness for the Weak Equivalence Principle. Entropy 2023, 25, 448. https://doi.org/10.3390/e25030448
Bose S, Mazumdar A, Schut M, Toroš M. Entanglement Witness for the Weak Equivalence Principle. Entropy. 2023; 25(3):448. https://doi.org/10.3390/e25030448
Chicago/Turabian StyleBose, Sougato, Anupam Mazumdar, Martine Schut, and Marko Toroš. 2023. "Entanglement Witness for the Weak Equivalence Principle" Entropy 25, no. 3: 448. https://doi.org/10.3390/e25030448
APA StyleBose, S., Mazumdar, A., Schut, M., & Toroš, M. (2023). Entanglement Witness for the Weak Equivalence Principle. Entropy, 25(3), 448. https://doi.org/10.3390/e25030448






