Design and Analysis of a Novel Flexure-Based XY Micropositioning Stage
Abstract
1. Introduction
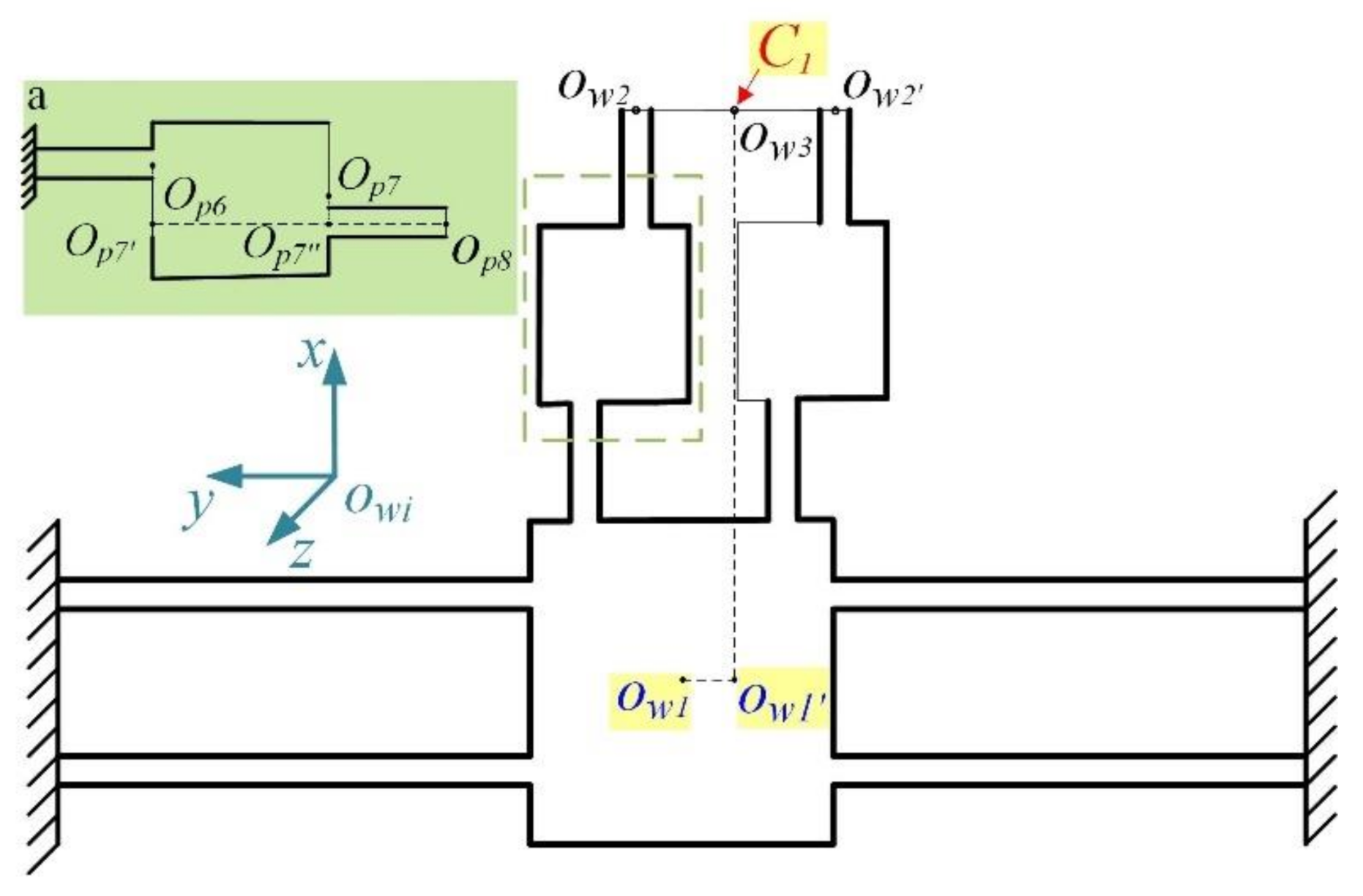
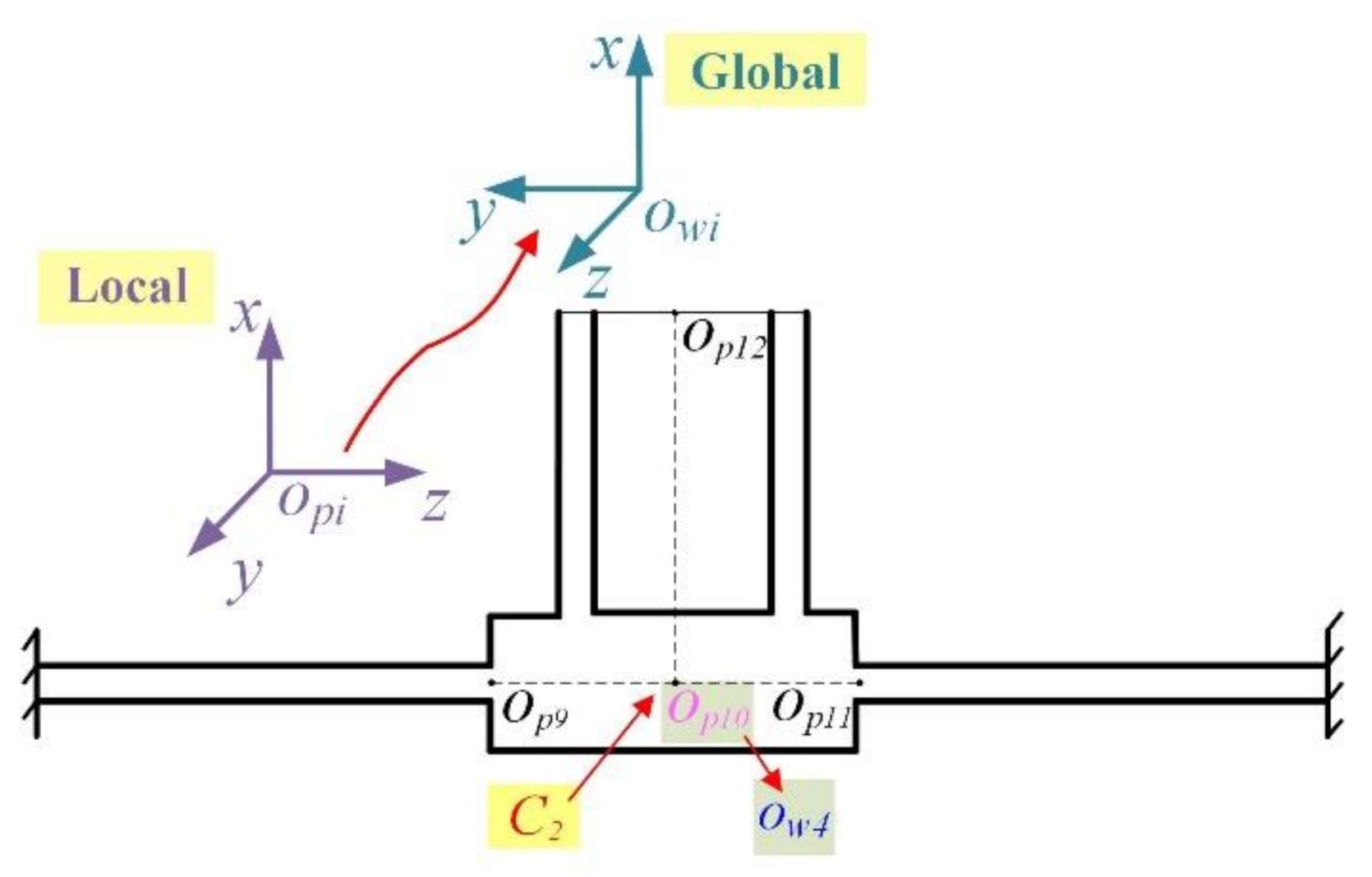
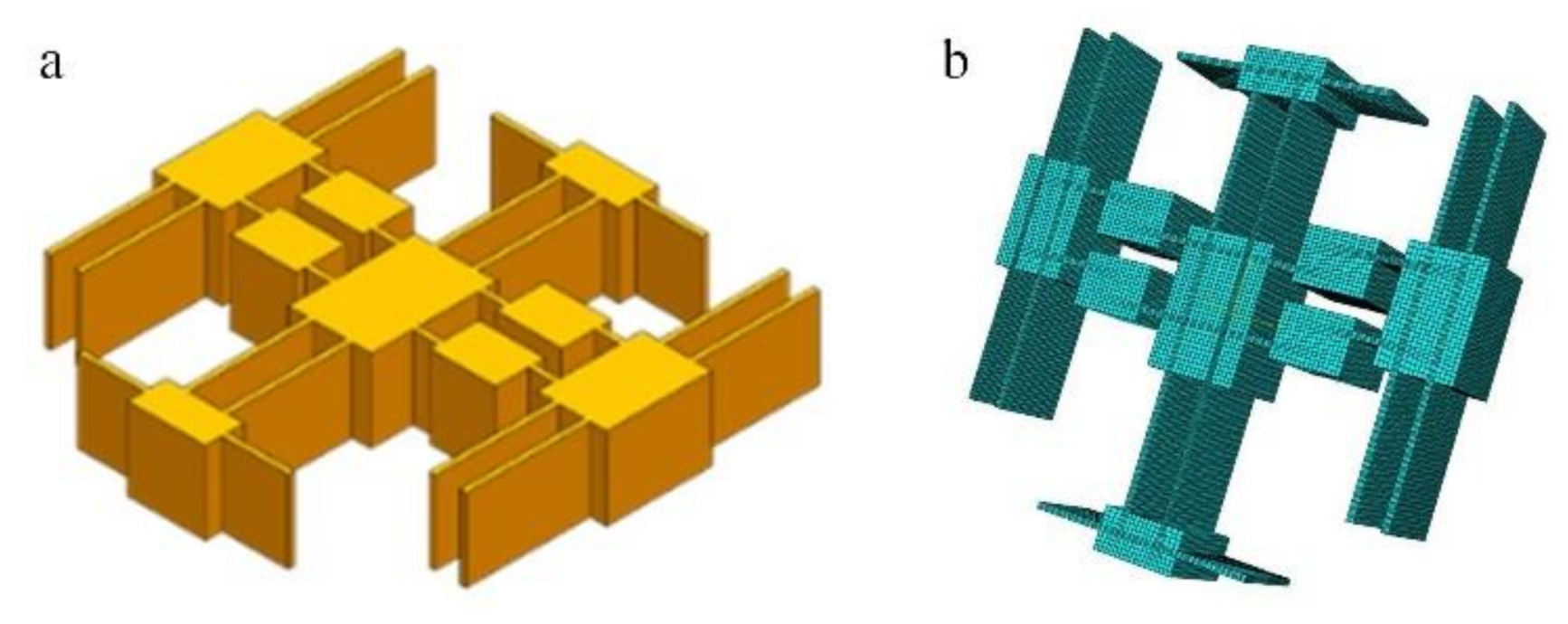
2. Mechanical Design
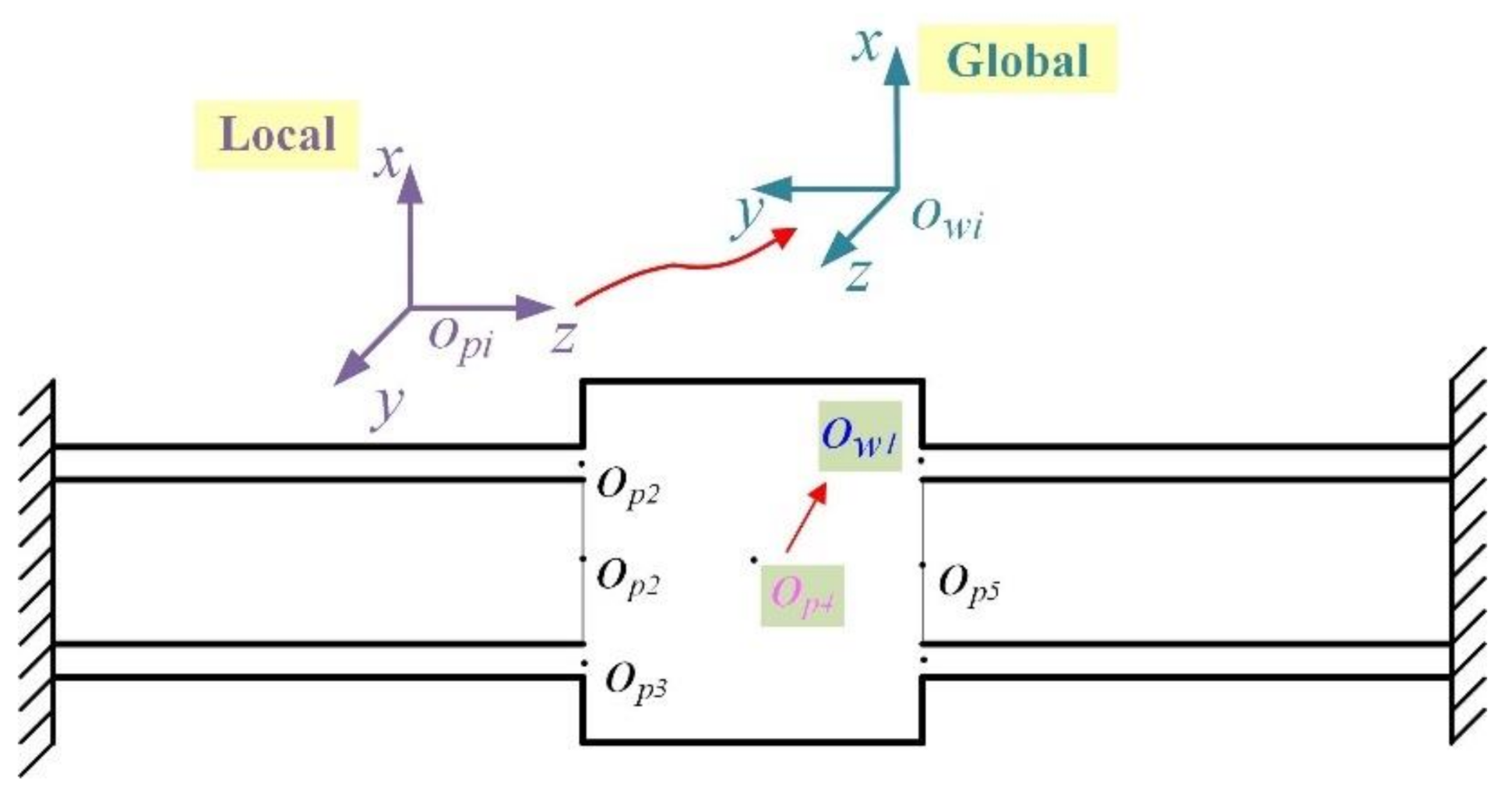
3. Mathematical Model
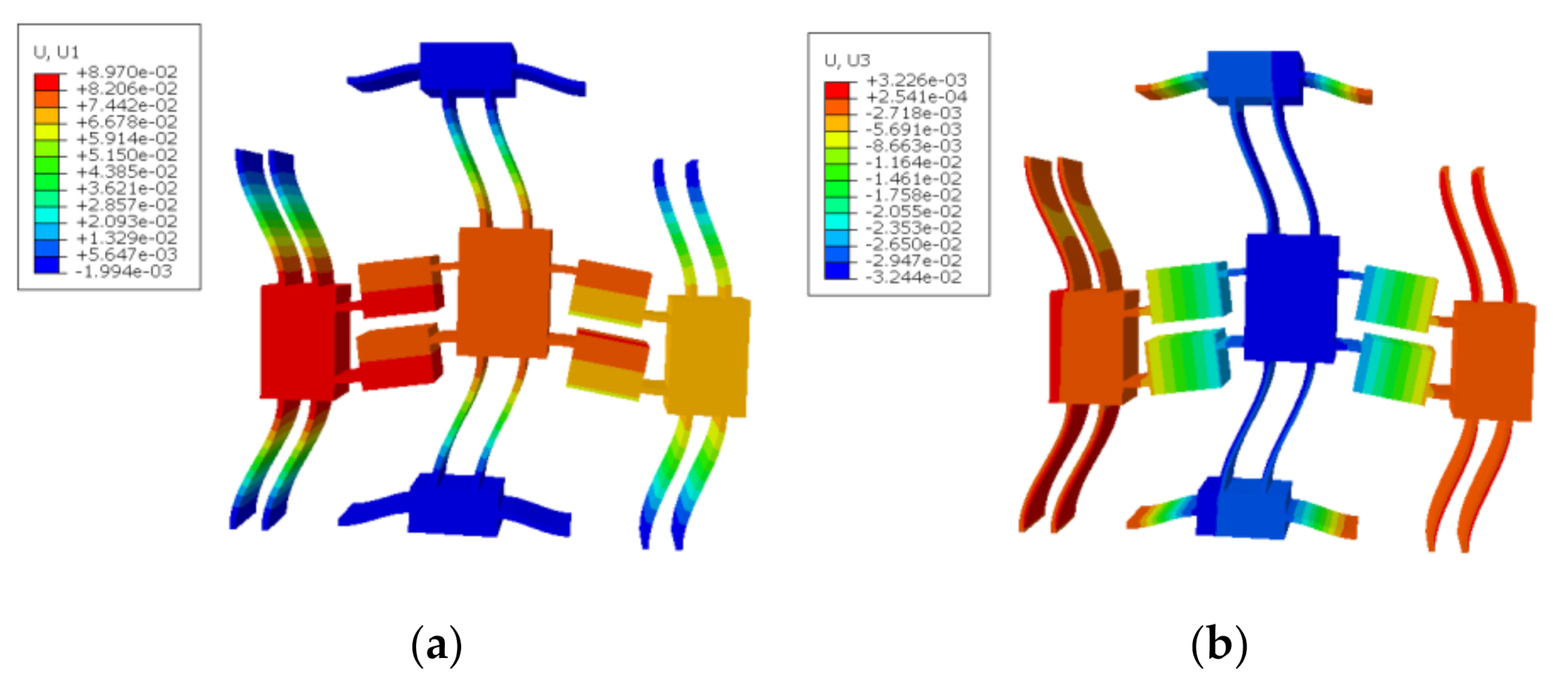
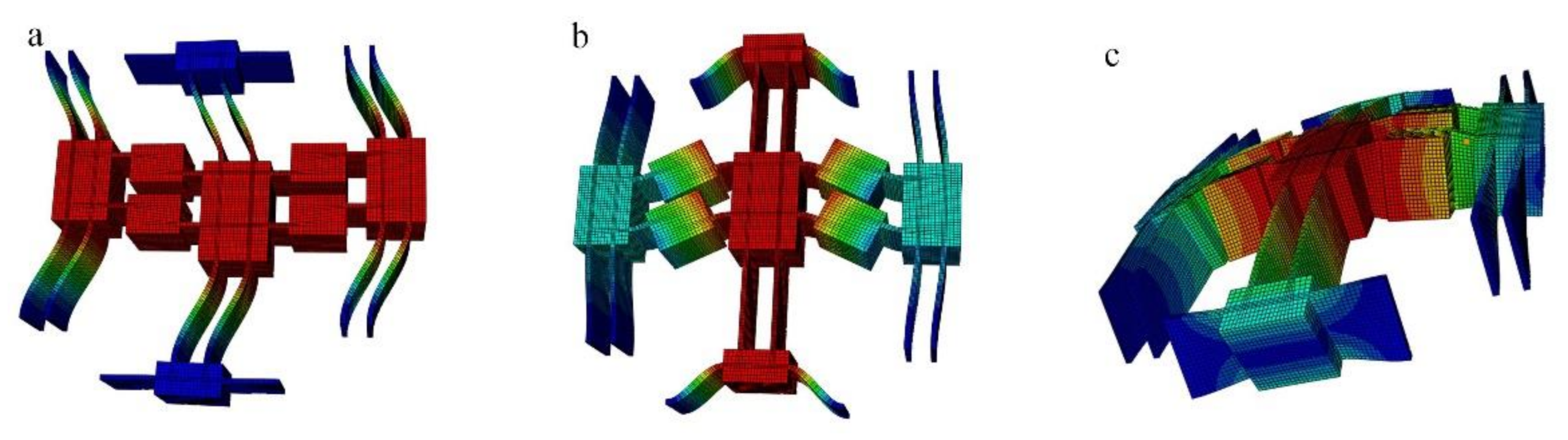
4. Numerical Simulations
5. Experiments
5.1. Method
5.2. Discussions
6. Conclusions
- (1)
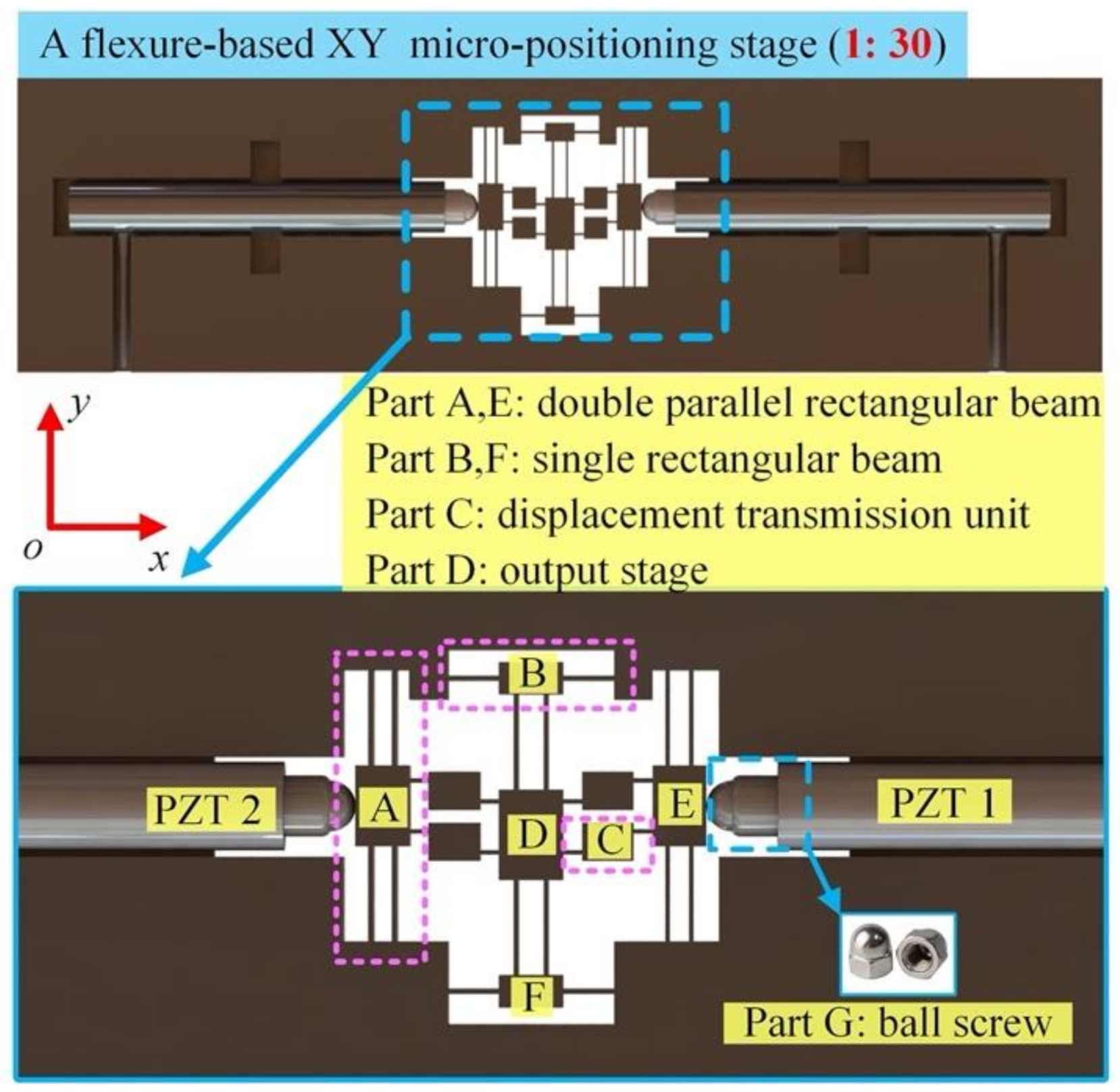
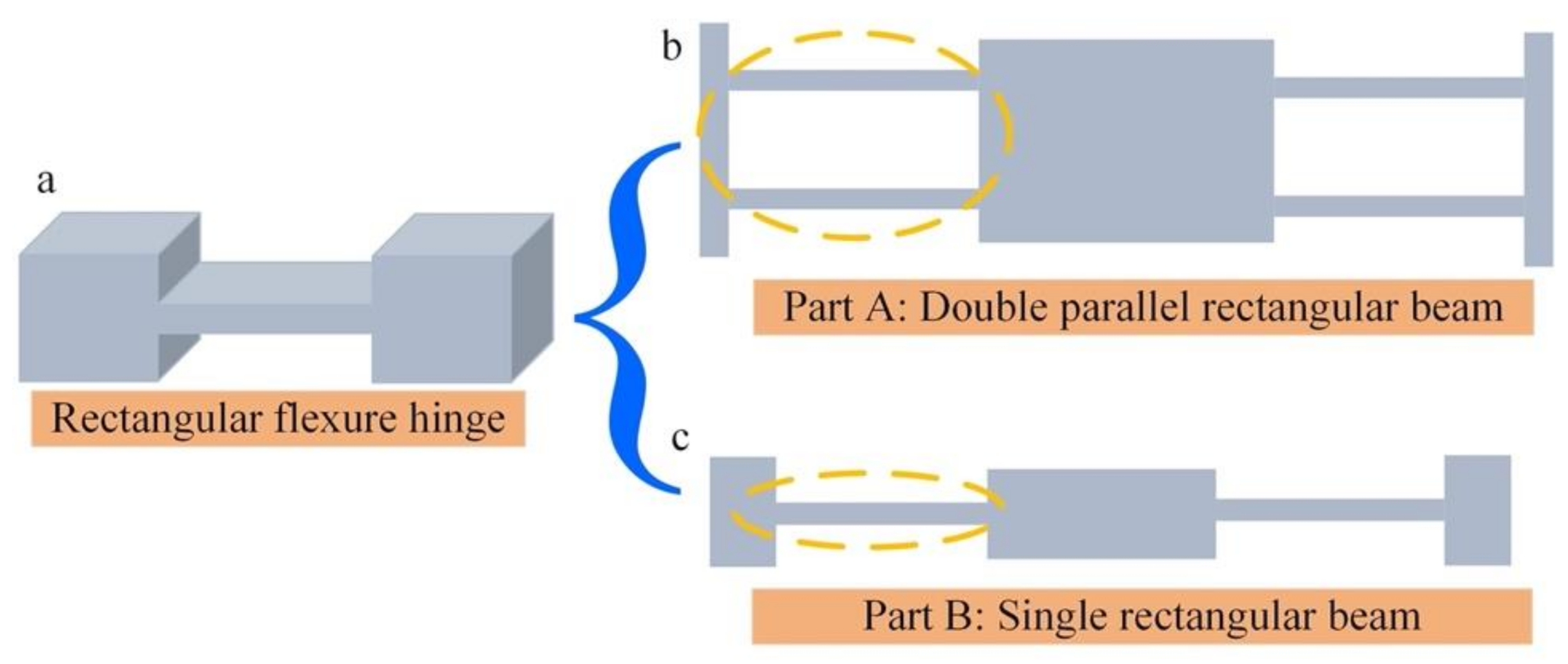
- The stage was symmetrical along the Y-axis, and consisted of an output stage, double parallel rectangular beams, single rectangular beams, and displacement transmission units, while they were all on a rectangular beam hinge base. The two piezoelectric actuators were arranged symmetrically along the X-axis to realize the displacement input. The input displacement was transferred by displacement transmission units. Different working ranges were achieved via the cooperation of two piezoelectric actuators. Along the X-axis, double parallel rectangular beams and displacement transmission units added individual stiffness to improve the transmission stability of displacement input.
- (2)
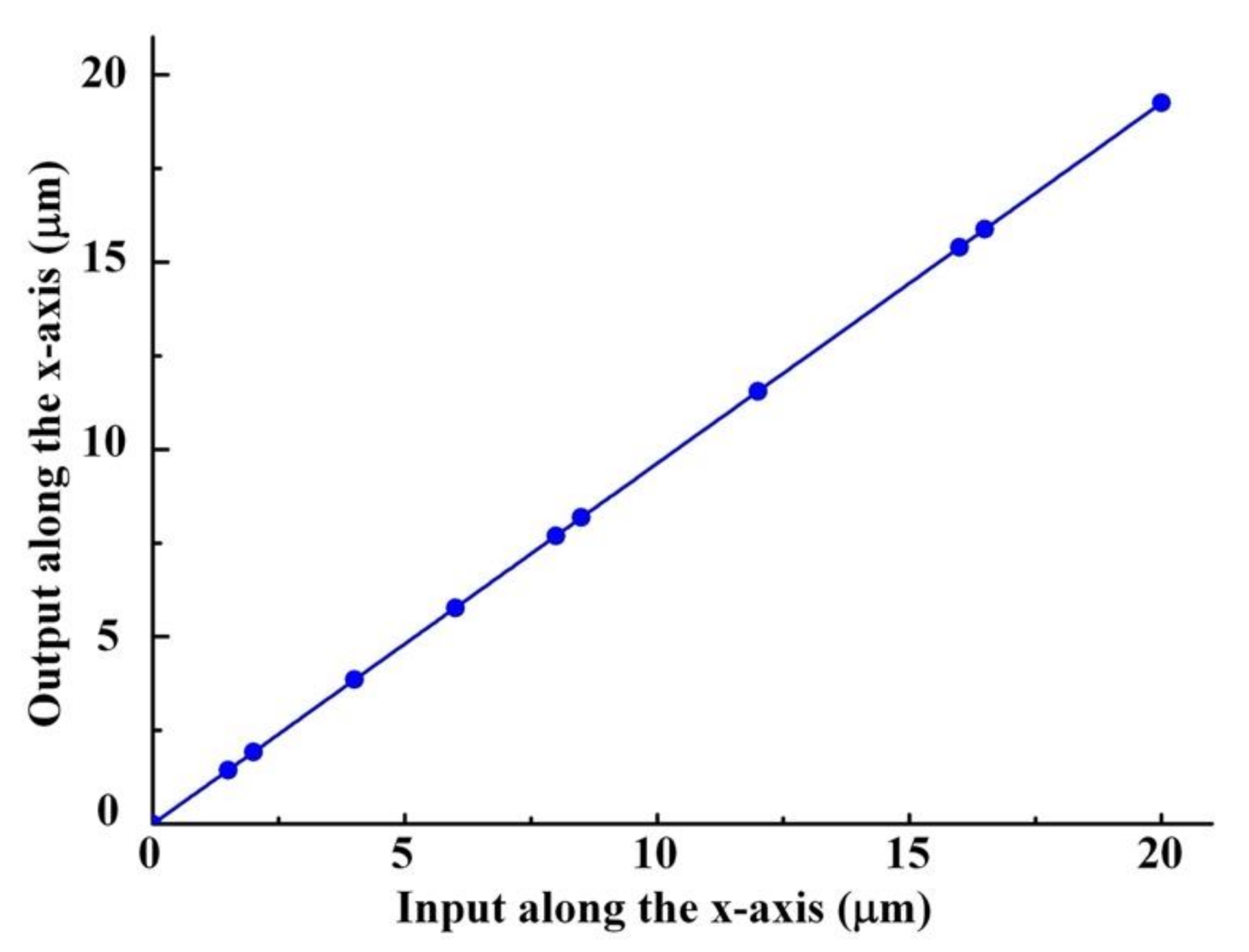
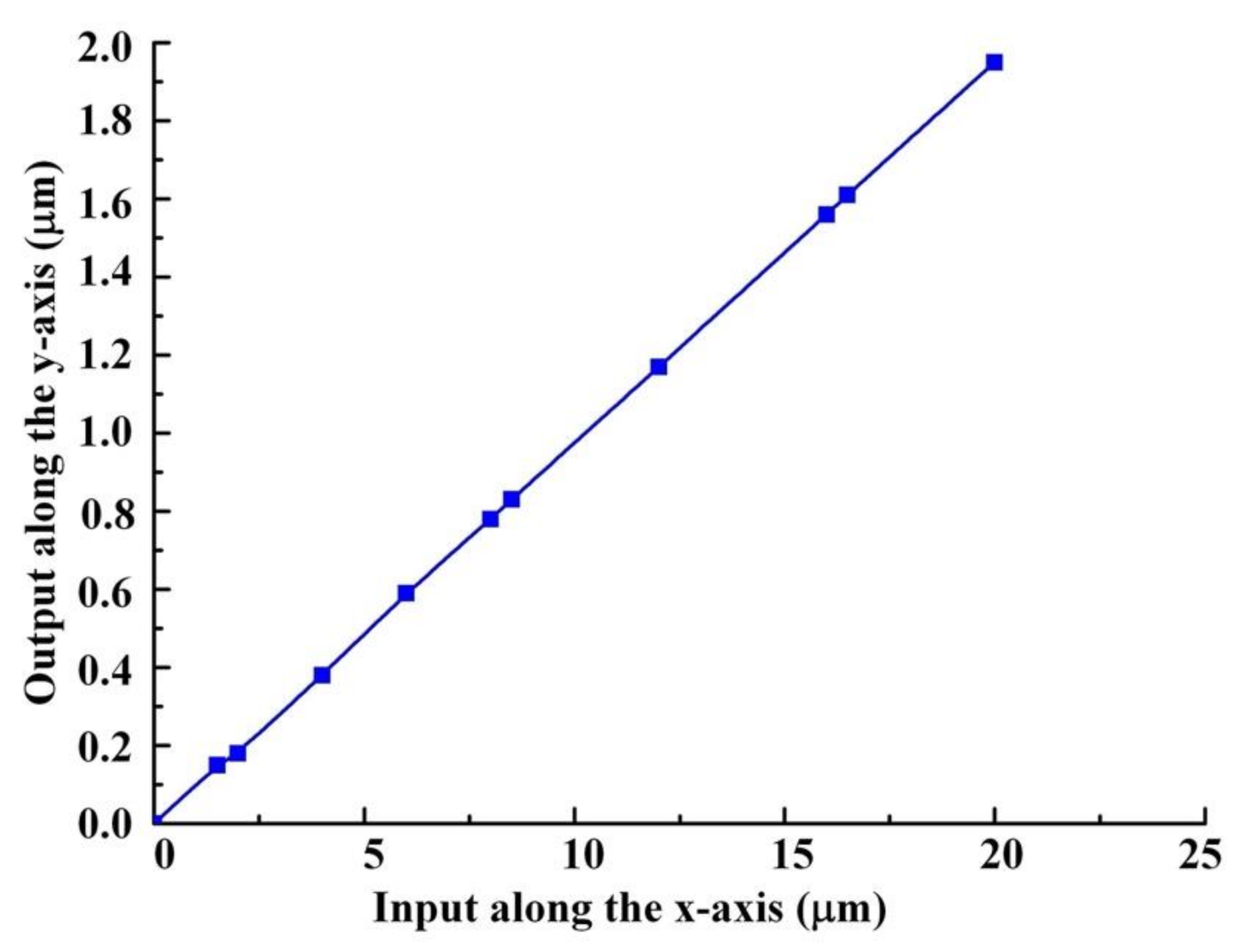
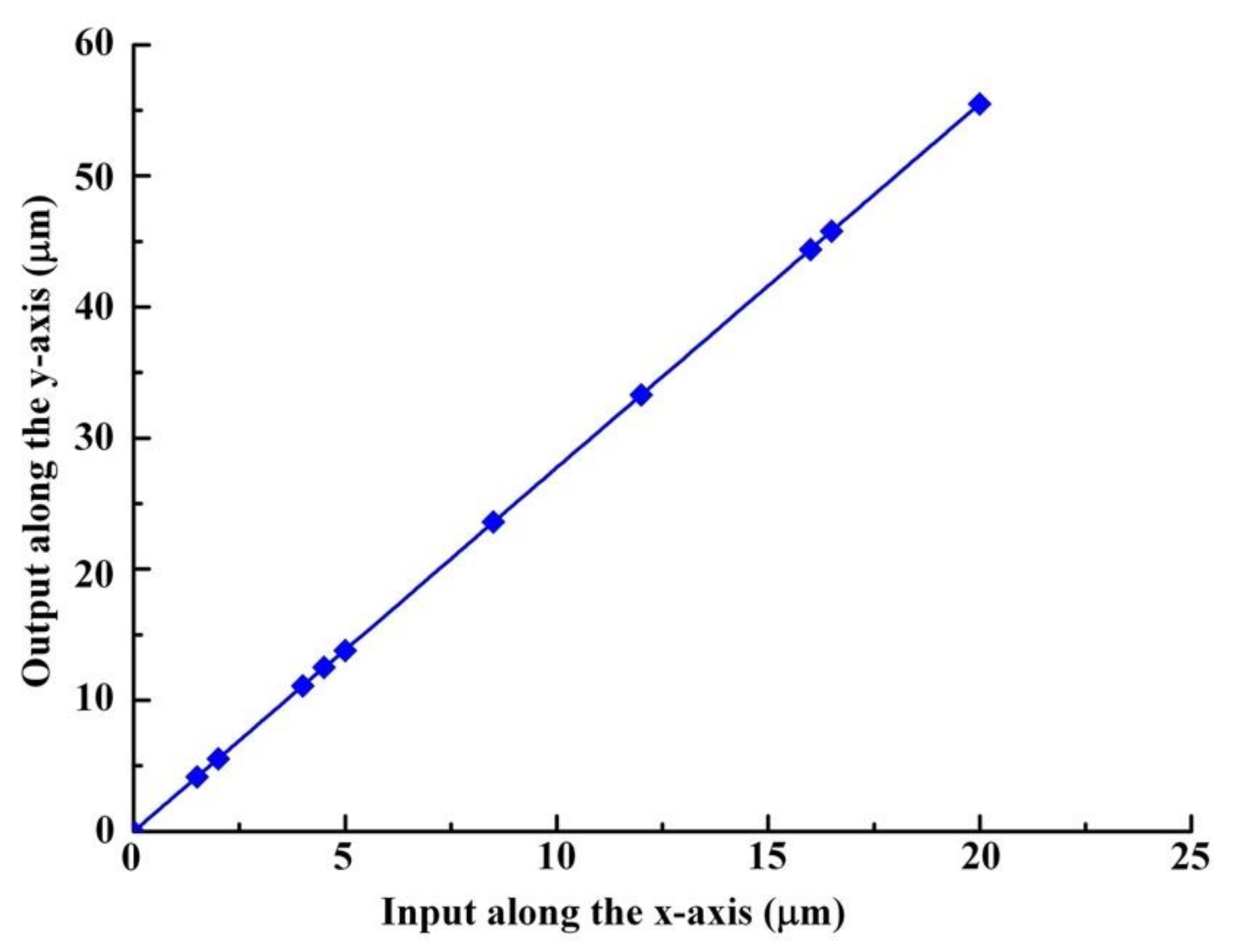
- The compliance matrix method was used to establish an analytical model of the proposed positioning stage. The output stiffness in the X- and Y-directions were 1.34 μm/N and 0.438 μm/N. Finite element analysis was used to validate the theoretical equation. The error between the theoretical calculation and simulation analysis was within 2.2 and 5.3% in the X- and Y-directions, respectively. The frequency of the X- and Y-directions were 1088.9 and 2240.1 Hz, respectively. When given only a single direction input, the displacement average error was 3.83% and the displacement ratio of the X- to Y-directions was 10.07%. When given double direction inputs, in the Y-axis, the stage could realize a better displacement magnification, where the magnification ratio was 2.77 and the coupling error was 1 × 10−5%. Using this input method, the positioning stage could be used as a great direction amplifier, while also increasing the positioning range.
- (3)
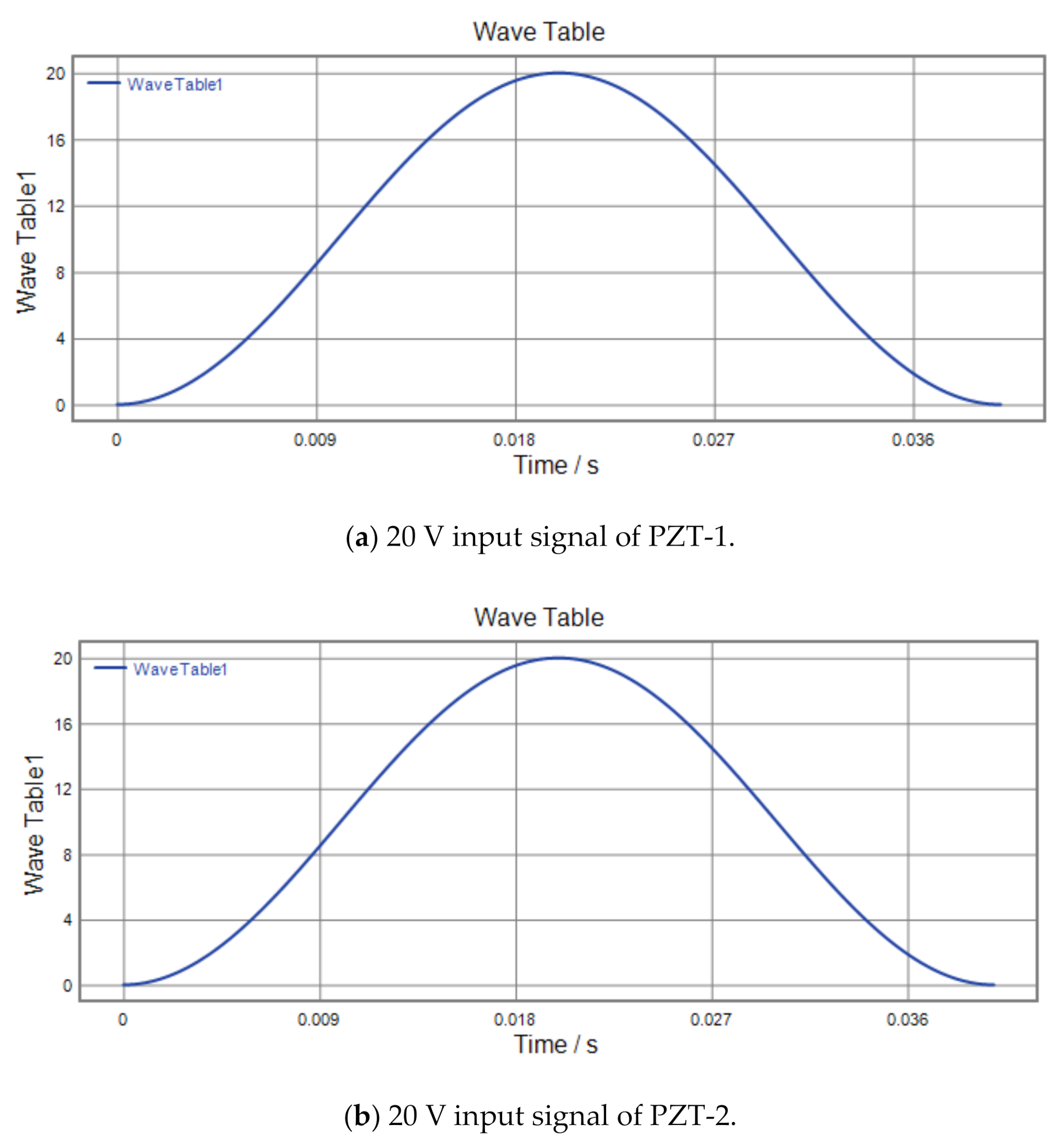
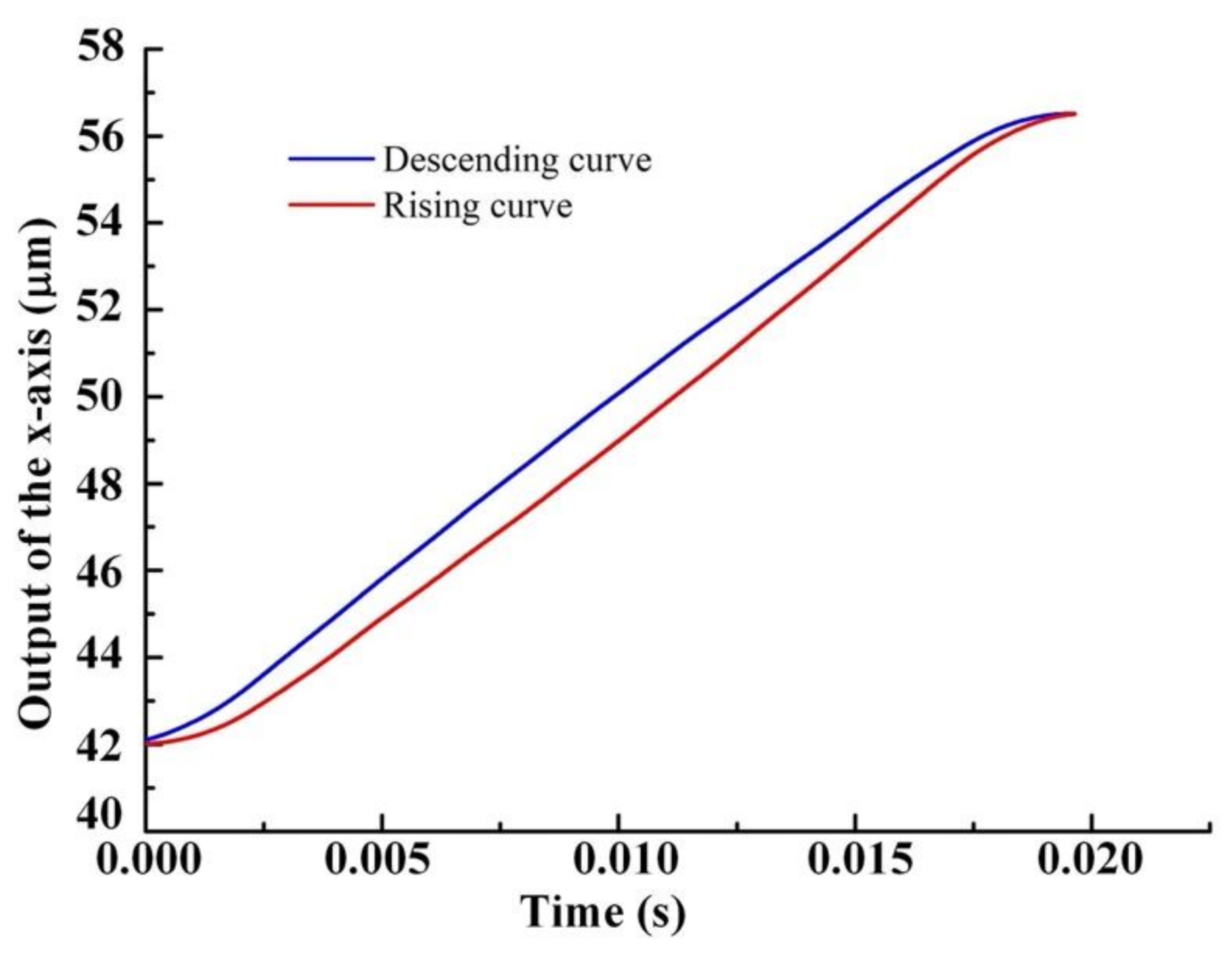
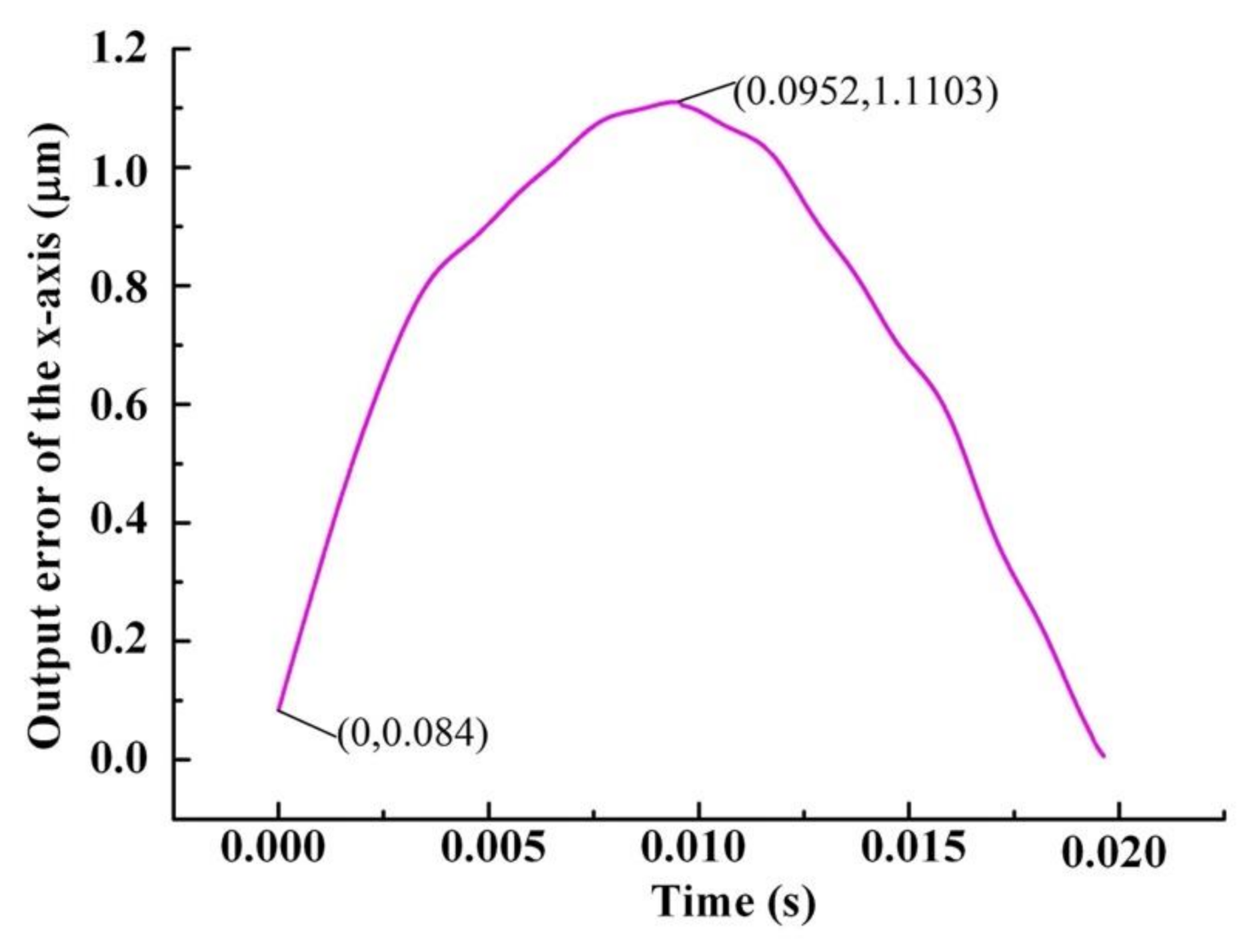
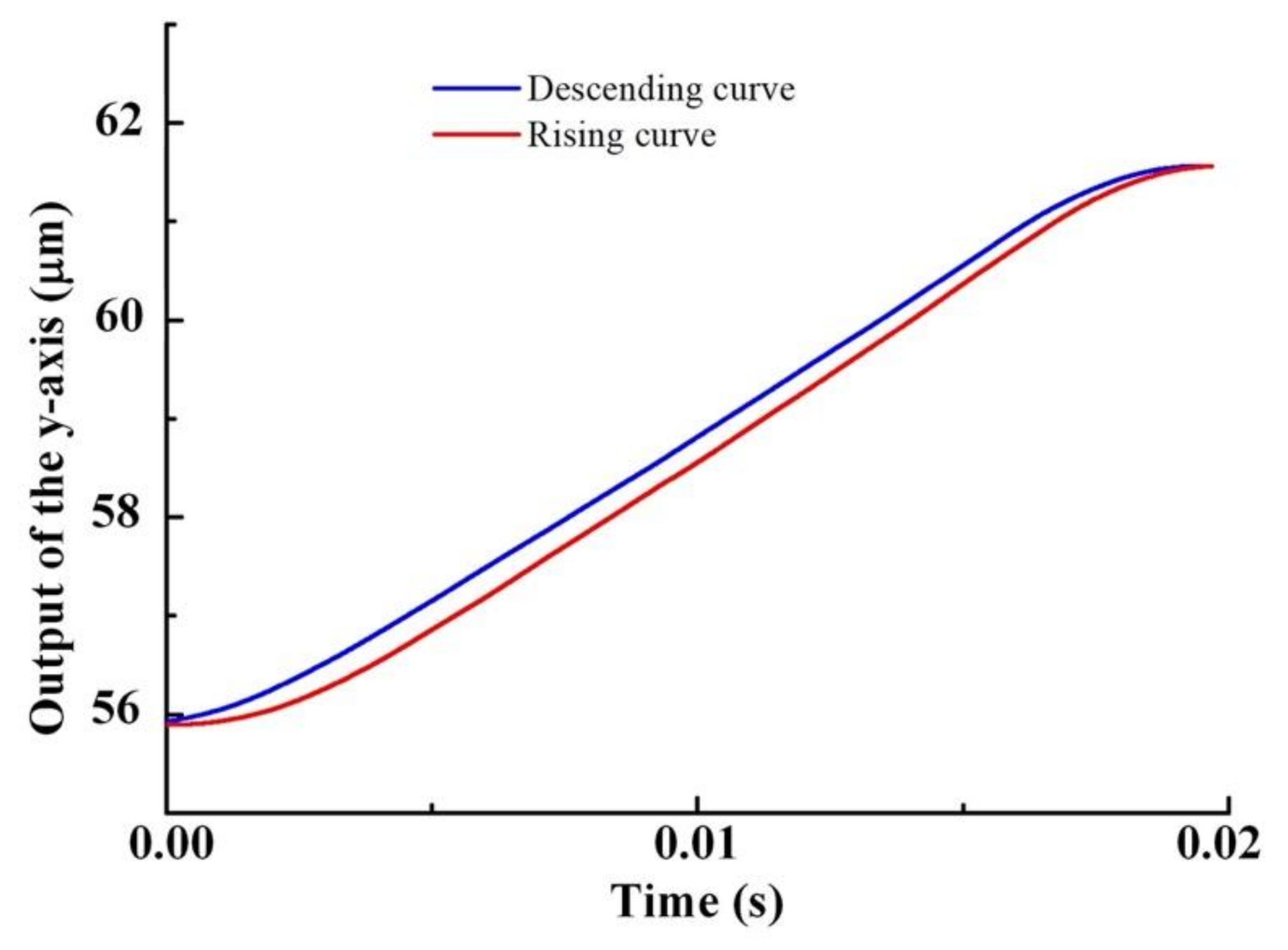
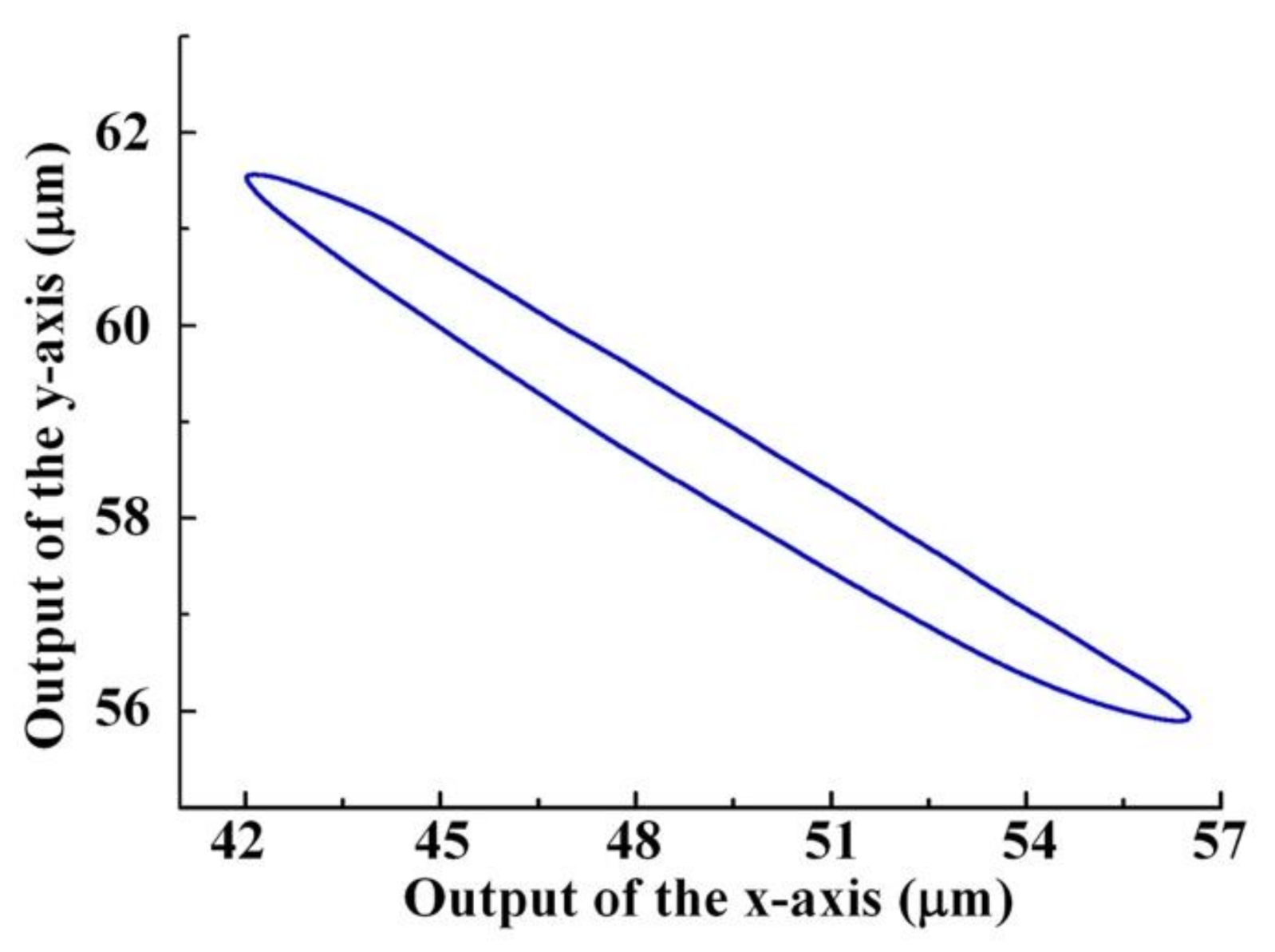
- The performance evaluation of the mechanism was tested via experiments. The actual output signal tended to be flat after approximately 0.02 s. Along the X-axis, the initial position offset was approximately 0.084 μm and the maximum displacement difference caused by hysteresis was 1.11 μm. Along the Y-axis, the initial position offset was about 0.03 μm and the maximum displacement error was 0.3 μm. Under a certain safety factor and the stroke limit of the piezoelectric actuator, the maximum displacement in the X-direction was about 14.5 μm; the maximum displacement in the Y-direction was 6.97 μm. The experimental tests showed that the working range had an elliptical trajectory.
Author Contributions
Funding
Conflicts of Interest
Appendix A
| Parameter | ||||||||
|---|---|---|---|---|---|---|---|---|
| Formula |

Appendix B
Appendix C
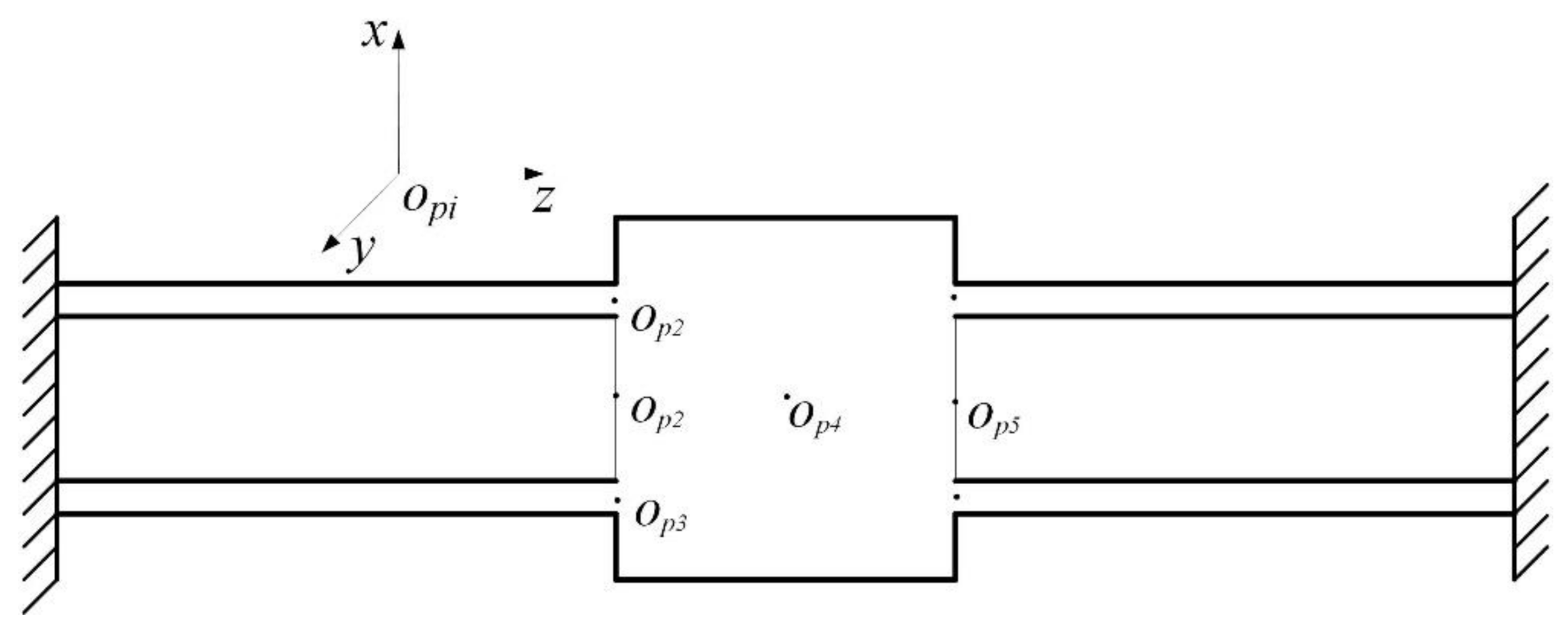
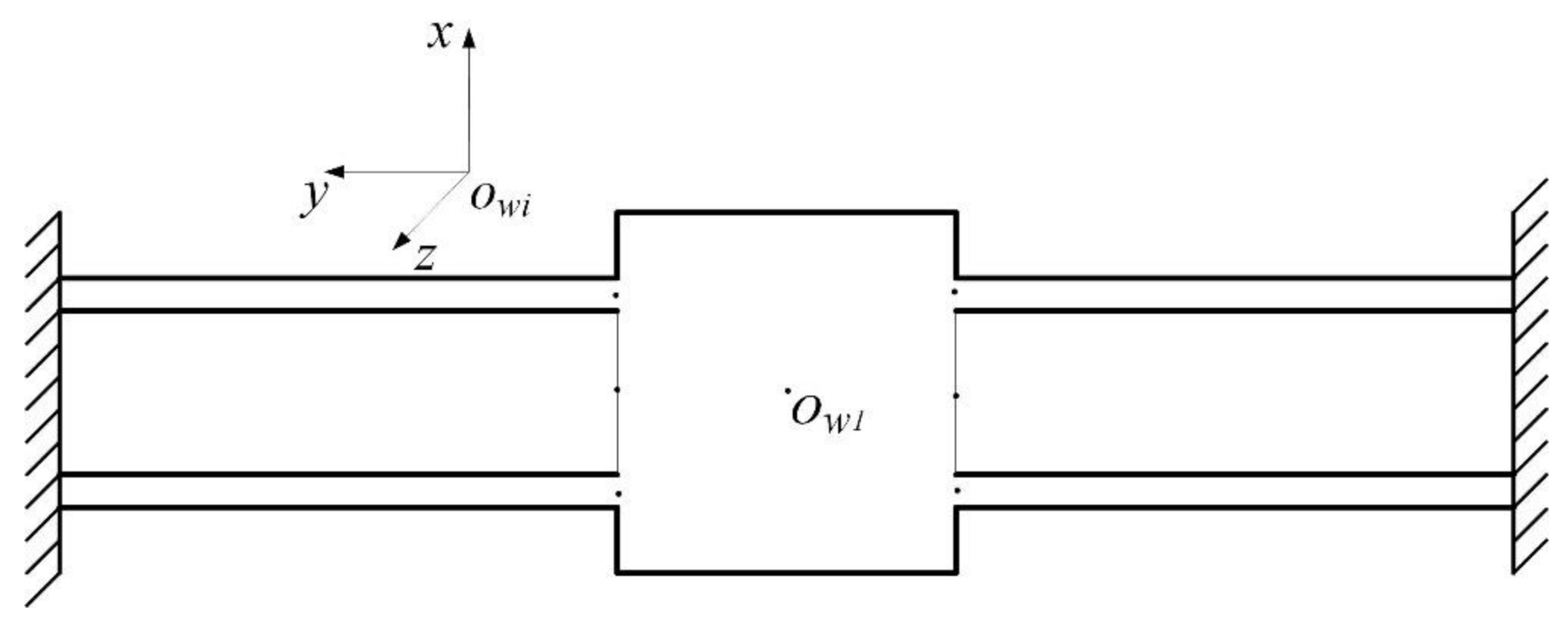
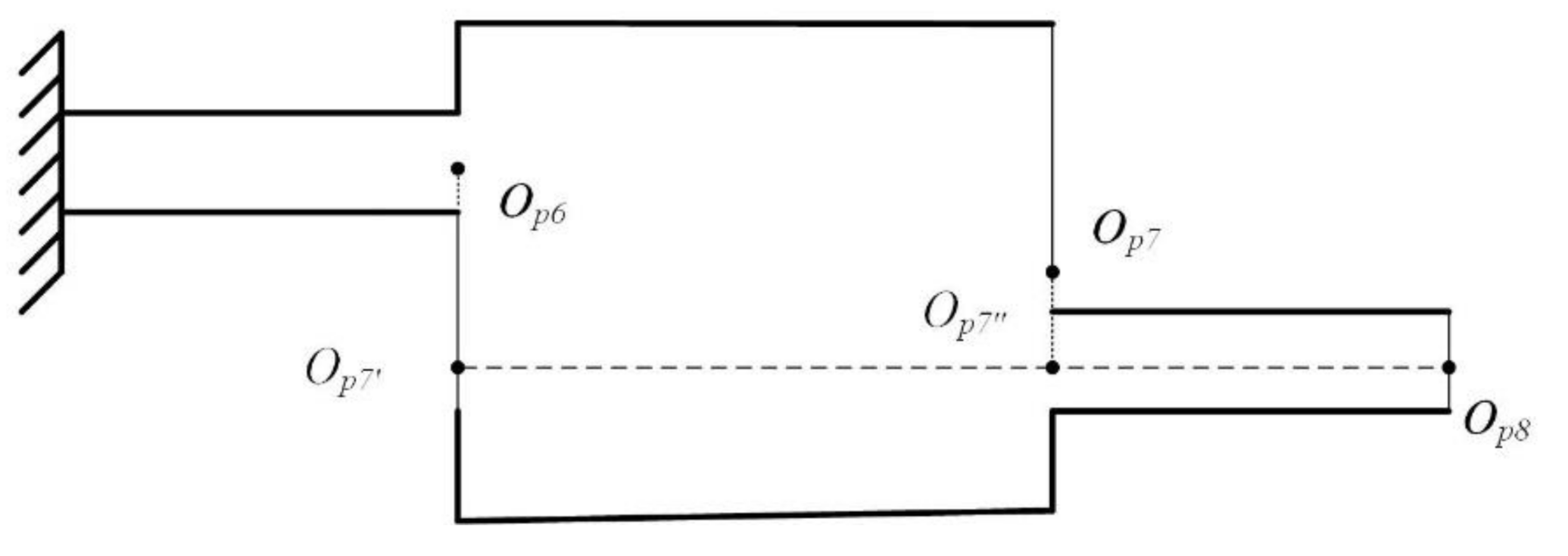

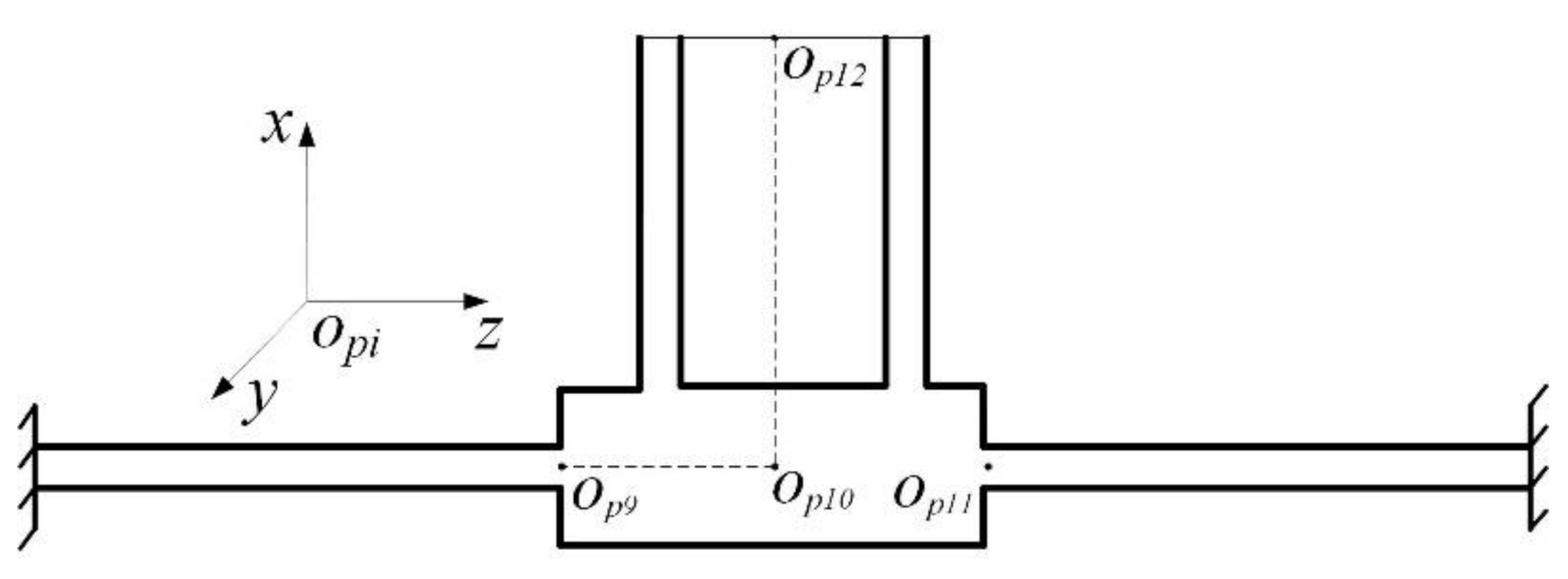
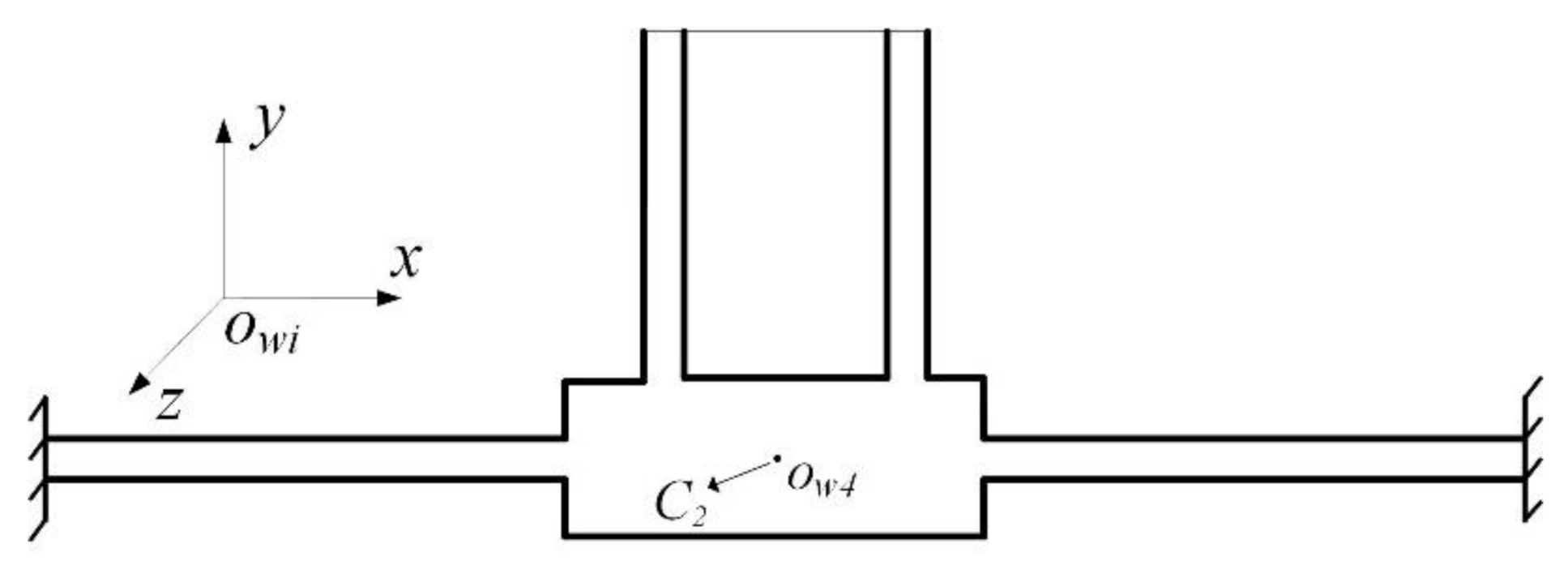

Appendix D

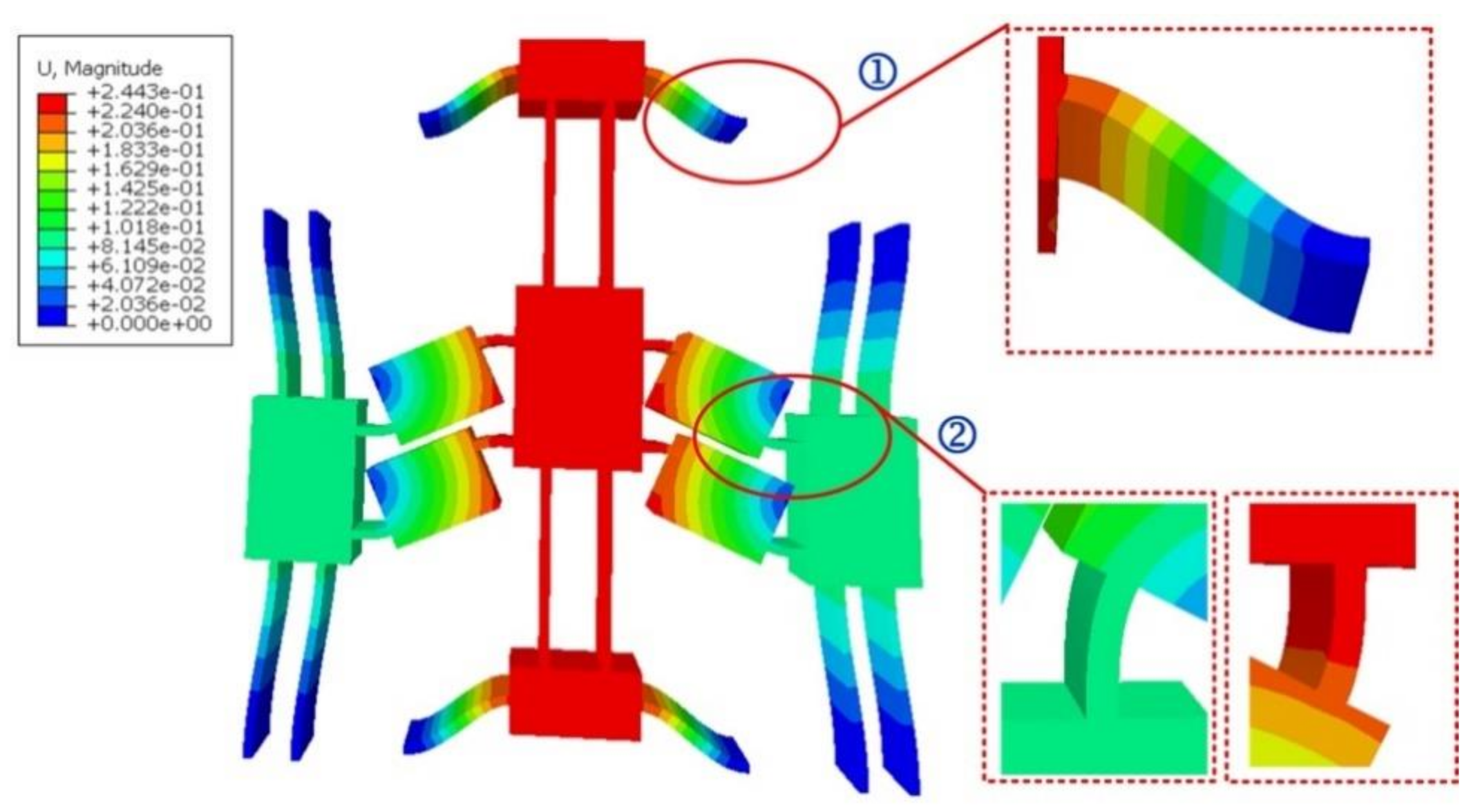

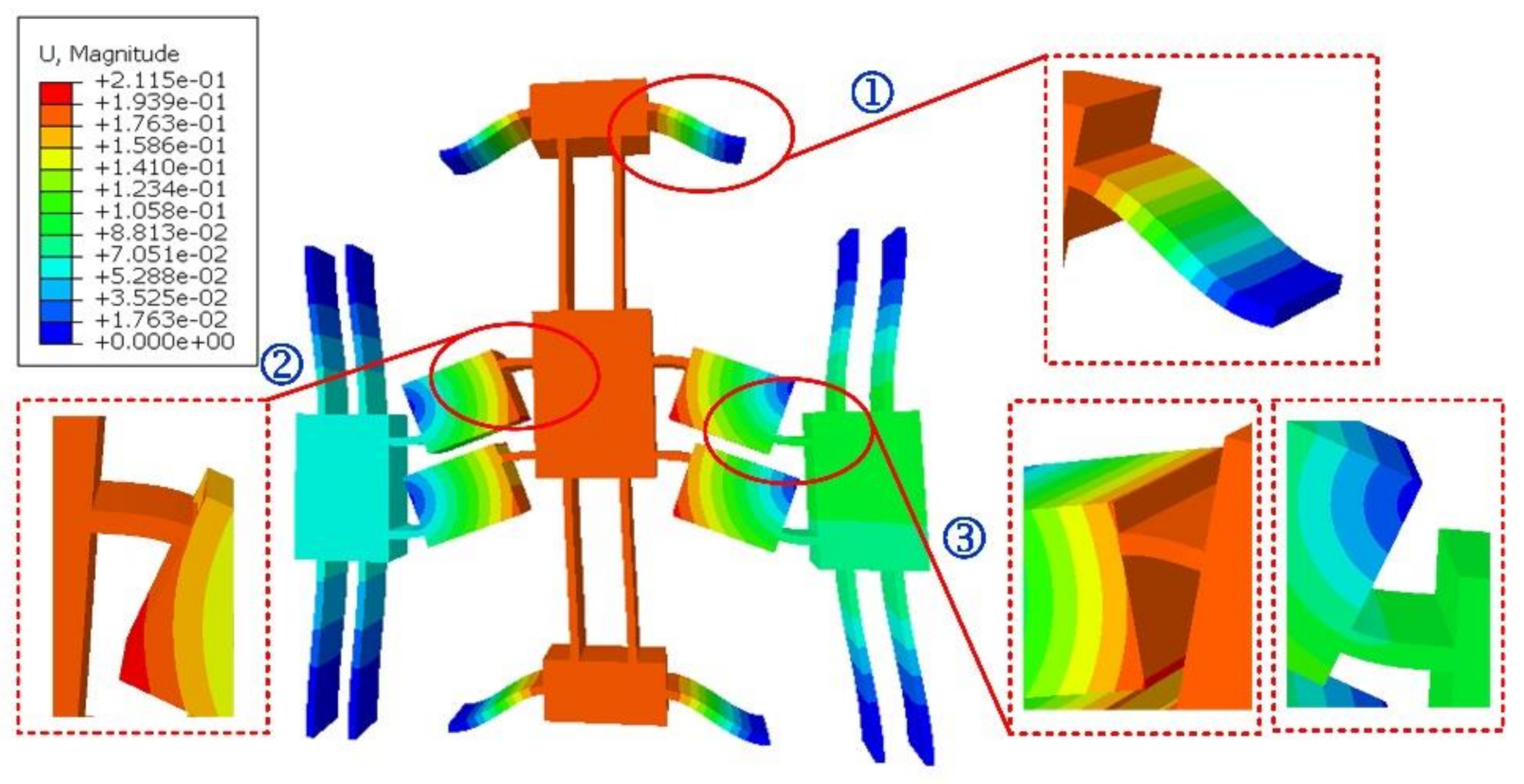
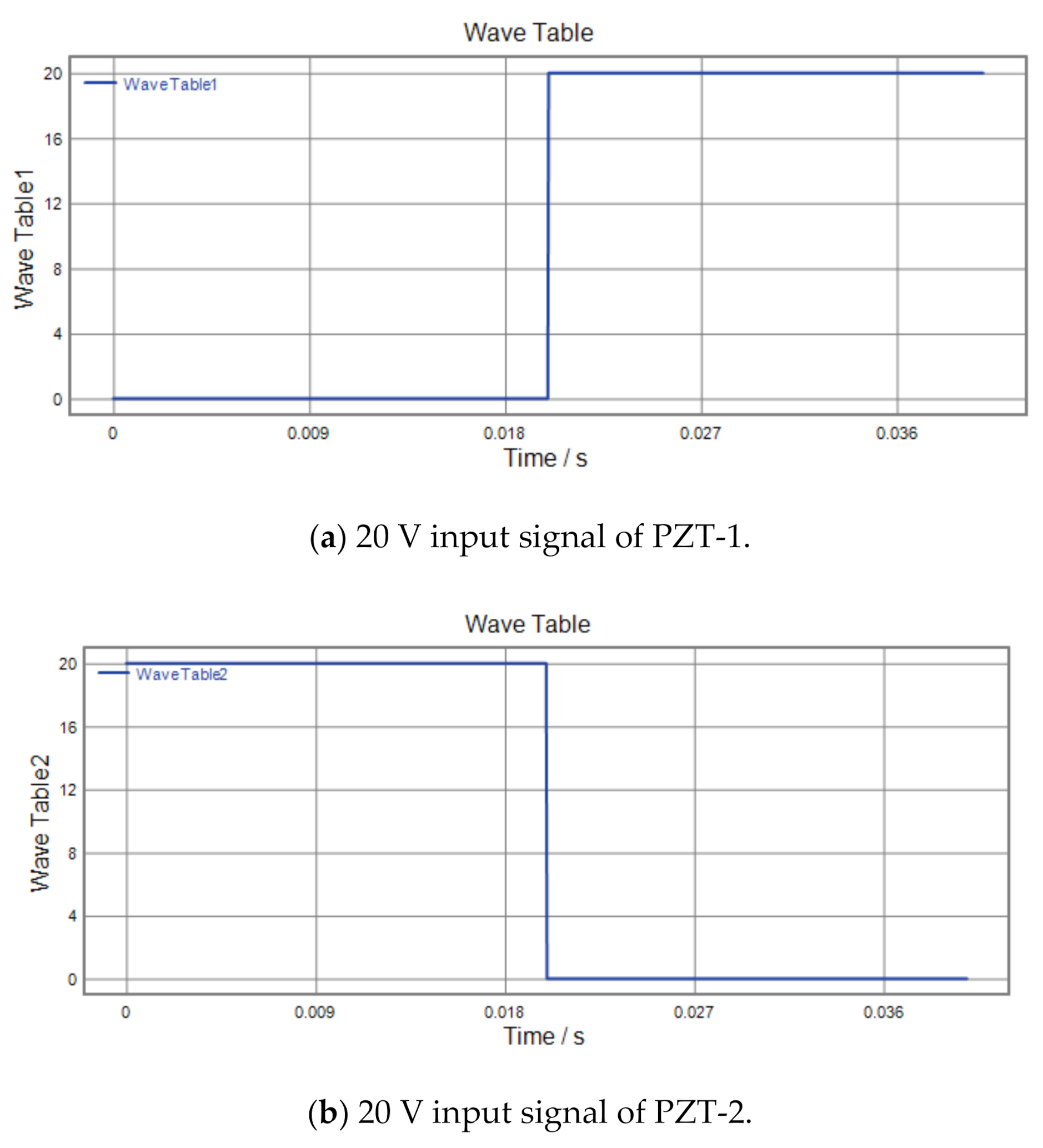

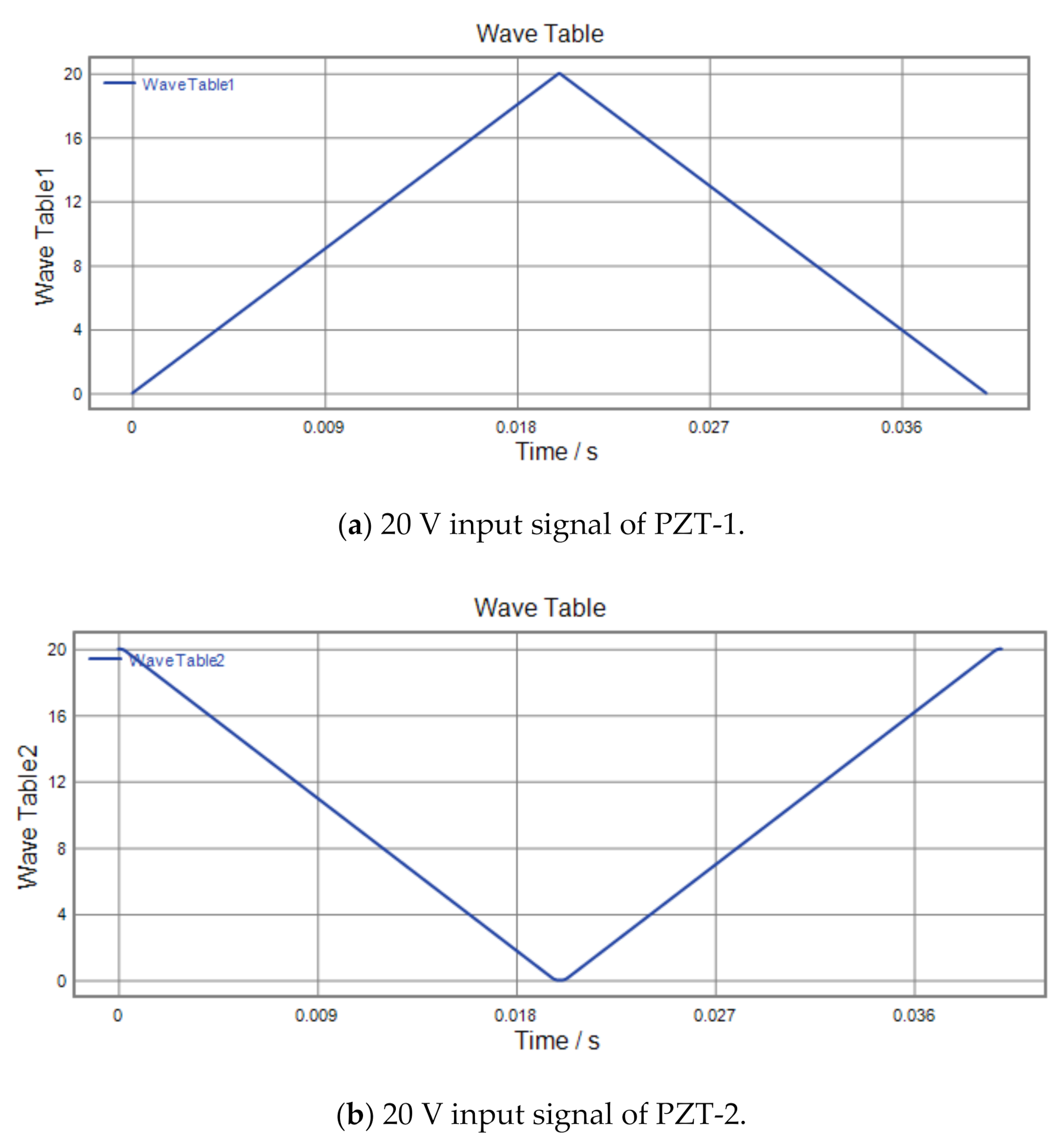
References
- Sun, X.; Chen, W.; Fatikow, S.; Tian, Y.; Zhou, R.; Zhang, J.; Mikczinski, M. A novel piezo-driven microgripper with a large jaw displacement. Microsyst. Technol. 2015, 21, 931–942. [Google Scholar] [CrossRef]
- Qu, J.; Chen, W.; Zhang, J.; Chen, W. A large-range compliant micropositioning stage with remote-center-of-motion characteristic for parallel alignment. Microsyst. Technol. 2016, 22, 777–789. [Google Scholar] [CrossRef]
- Li, J.; Zhao, H.; Qu, X.; Qu, H.; Zhou, X.; Fan, Z.; Fu, H. Development of a compact 2-DOF precision piezoelectric positioning platform based on inchworm principle. Sens. Actuators A Phys. 2015, 222, 87–95. [Google Scholar] [CrossRef]
- Lee, H.J.; Woo, S.; Park, J.; Jeong, J.H.; Kim, M.; Ryu, J.; Gweon, D.G.; Choi, Y.M. Compact compliant parallel XY nano-positioning stage with high dynamic performance, small crosstalk, and small yaw motion. Microsyst. Technol. 2018, 24, 2653–2662. [Google Scholar] [CrossRef]
- Chen, W.; Qu, J.; Chen, W.; Zhang, J. A compliant dual-axis gripper with integrated position and force sensing. Mechatronics 2017, 47, 105–115. [Google Scholar] [CrossRef]
- Wang, N.; Zhang, Z.; Zhang, X.; Cui, C. Optimization of a 2-DOF micro-positioning stage using corrugated flexure units. Mech. Mach. Theory 2018, 121, 683–696. [Google Scholar] [CrossRef]
- Zhang, X.; Xu, Q. Design and testing of a new 3-DOF spatial flexure parallel micropositioning stage. Int. J. Precis. Eng. Manuf. 2018, 19, 109–118. [Google Scholar] [CrossRef]
- Noveanu, S.; Ivan, I.A.; Noveanu, D.C.; Rusu, C.; Lates, D. SiMFlex Micromanipulation Cell with Modular Structure. Appl. Sci. 2020, 10, 2861. [Google Scholar] [CrossRef]
- Cai, K.; He, X.; Tian, Y.; Liu, X.; Zhang, D.; Shirinzadeh, B. Design of a XYZ scanner for home-made high-speed atomic force microscopy. Microsyst. Technol. 2018, 24, 3123–3132. [Google Scholar] [CrossRef]
- Li, Y.; Xu, Q. A novel design and analysis of a 2-DOF compliant parallel micromanipulator for nanomanipulation. IEEE Trans. Autom. Sci. Eng. 2006, 3, 247–254. [Google Scholar]
- Jensen, K.A.; Lusk, C.P.; Howell, L.L. An XYZ Micromanipulator with Three Translational Degrees of Freedom. Robotica 2006, 24.3, 305–314. [Google Scholar] [CrossRef]
- Awtar, S.; Slocum, A.H. Constraint-Based Design of Parallel Kinematic XY Flexure Mechanisms. ASME J. Mech. Des. 2007, 129, 816–830. [Google Scholar] [CrossRef]
- Teo, T.J.; Yang, G.; Chen, I.M. A large deflection and high payload flexure-based parallel manipulator for UV nanoimprint lithography: Part I. Modeling and analyses. Precis. Eng. 2014, 38, 861–871. [Google Scholar] [CrossRef]
- Li, Y.; Xu, Q. A novel piezoactuated XY stage with parallel, decoupled, and stacked flexure structure for micro-/nanopositioning. IEEE Trans. Ind. Electron. 2010, 58, 3601–3615. [Google Scholar] [CrossRef]
- Xiao, S.; Li, Y.; Zhao, X. Design and analysis of a novel flexure-based XY micro-positioning stage driven by electromagnetic actuators. In Proceedings of the 2011 International Conference on Fluid Power and Mechatronics, Beijing, China, 17–20 August 2011; pp. 953–958. [Google Scholar]
- Kang, B.H.; Wen, J.Y.; Dagalakis, N.G.; Gorman, J.J. Analysis and design of parallel mechanisms with flexure joints. IEEE Trans. Robot. 2005, 21, 1179–1185. [Google Scholar] [CrossRef]
- Choi, K.B.; Kim, D.H. Monolithic parallel linear compliant mechanism for two axes ultraprecision linear motion. Rev. Sci. Instrum. 2006, 77, 065106. [Google Scholar] [CrossRef]
- DiBiasio, C.M.; Culpepper, M.L.; Panas, R.; Howell, L.L.; Magleby, S.P. Comparison of molecular simulation and pseudo-rigid-body model predictions for a carbon nanotube–based compliant parallel-guiding mechanism. J. Mech. Des. 2008, 130, 1–7. [Google Scholar] [CrossRef]
- Yong, Y.K.; Lu, T.F. Kinetostatic modeling of 3-RRR compliant micro-motion stages with flexure hinges. Mech. Mach. Theory 2009, 44, 1156–1175. [Google Scholar] [CrossRef]
- Liaw, H.C.; Shirinzadeh, B. Constrained motion tracking control of piezo-actuated flexure-based four-bar mechanisms for micro/nano manipulation. IEEE Trans. Automat. Sci. Eng. 2010, 7, 699–705. [Google Scholar] [CrossRef]
- Xue, G.; Toda, M.; Ono, T. Comb-drive XYZ-microstage based on assembling technology for low temperature measurement systems. In Proceedings of the 2015 International Conference on Electronics Packaging and iMAPS All Asia Conference (ICEP-IAAC), Kyoto, Japan, 14–17 April 2015; pp. 83–88. [Google Scholar]
- Khan, M.U.; Prelle, C.; Lamarque, F.; Büttgenbach, S. Design and assessment of a micropositioning system driven by electromagnetic actuators. IEEE/ASME Trans. Mechatron. 2016, 22, 551–560. [Google Scholar] [CrossRef]
- Lai, L.J.; Zhu, Z.N. Design, modeling and testing of a novel flexure-based displacement amplification mechanism. Sens. Actuators A: Phys. 2017, 266, 122–129. [Google Scholar] [CrossRef]
- Lin, C.; Shen, Z.; Wu, Z.; Yu, J. Kinematic characteristic analysis of a micro-/nano positioning stage based on bridge-type amplifier. Sens. Actuators A Phys. 2018, 271, 230–242. [Google Scholar] [CrossRef]
- Jiang, Y.; Li, T.; Wang, L.; Chen, F. Systematic Design Method and Experimental Validation of a 2-DOF Compliant Parallel Mechanism with Excellent Input and Output Decoupling Performances. Appl. Sci. 2017, 7, 591. [Google Scholar] [CrossRef]
- Jieqiong, L.; Jinguo, H.; Mingming, L.; Yan, G.; Wenhui, Z. Development of nonresonant elliptical vibration cutting device based on parallel piezoelectric actuator. Aip Adv. 2017, 7, 035304. [Google Scholar] [CrossRef]
- Wang, P.; Xu, Q. Design of a flexure-based constant-force XY precision positioning stage. Mech. Mach. Theory 2017, 108, 1–13. [Google Scholar] [CrossRef]
- Arata, J.; Kogiso, S.; Sakaguchi, M.; Nakadate, R.; Oguri, S.; Uemura, M.; Byunghyun, C.; Akahoshi, T.; Ikeda, T.; Hashizume, M. Articulated minimally invasive surgical instrument based on compliant mechanism. Int. J. Comput. Assist. Radiol. Surg. 2015, 10, 1837–1843. [Google Scholar] [CrossRef]
- Wang, P.; Xu, Q. Design of a flexure-based XY precision positioning stage with constant force output. In Proceedings of the IECON 2016-42nd Annual Conference of the IEEE Industrial Electronics Society, Florence, Italy, 23–26 October 2016; pp. 524–529. [Google Scholar]
- Wu, Y.; Zhou, Z. Design calculations for flexure hinges. Rev. Sci. Instrum. 2002, 73, 3101–3106. [Google Scholar] [CrossRef]
- Xu, Q. A novel compliant micropositioning stage with dual ranges and resolutions. Sens. Actuators A Phys. 2014, 205, 6–14. [Google Scholar] [CrossRef]
- Trease, B.P.; Moon, Y.-M.; Kota, S. Design of Large-Displacement Compliant Joints. J. Mech. Design. 2005, 127, 788–798. [Google Scholar] [CrossRef]
- Schotborgh, W.O.; Kokkeler, F.G.; Tragter, H.; Van Houten, F.J. Dimensionless design graphs for flexure elements and a comparison between three flexure elements. Precis. Eng. 2005, 29, 41–47. [Google Scholar] [CrossRef]
- Paros, J.M.; Weisboro, L. How to design flexure hinges. Mach. Des. 1965, 37, 151–157. [Google Scholar]
- Lobontiu, N. Compliant Mechanisms: Design of Flexure Hinges; CRC Press: Boca Raton, FL, USA, 2002. [Google Scholar]
- Xie, Y.; Li, Y.; Cheung, C.F.; Zhu, Z.; Chen, X. Design and analysis of a novel compact XYZ parallel precision positioning stage. Microsyst. Technol. 2020, 26, 1–8. [Google Scholar] [CrossRef]
- Lobontiu, N. Modeling and design of planar parallel-connection flexible hinges for in- and out-of-plane mechanism applications. Precis. Eng. 2015, 42, 113–132. [Google Scholar] [CrossRef]
- Lin, C.Y.; Po-Ying, C. Precision motion control of a nano stage using repetitive control and double-feedforward compensation. In Proceedings of the SICE Annual Conference, Taipei, Taiwan, 18–21 August 2010; pp. 22–29. [Google Scholar]
- Čeponis, A.; Mazeika, D. An inertial piezoelectric plate type rotary motor. Sens. Actuators A Phys. 2017, 263, 131–139. [Google Scholar] [CrossRef]
- Gu, G.-Y.; Zhu, L.-M.; Su, C.-Y.; Ding, H. Motion Control of Piezoelectric Positioning Stages: Modeling, Controller Design, and Experimental Evaluation. IEEE/ASME Trans. Mechatron. 2012, 18, 1459–1471. [Google Scholar] [CrossRef]
- Yuan, S.; Zhao, Y.; Chu, X.; Zhu, C.; Zhong, Z. Analysis and Experimental Research of a Multilayer Linear Piezoelectric Actuator. Appl. Sci. 2016, 6, 225. [Google Scholar] [CrossRef]
- Jia, S.; Jiang, Y.; Li, T.; Du, Y. Learning-Based Optimal Desired Compensation Adaptive Robust Control for a Flexure-Based Micro-Motion Manipulator. Appl. Sci. 2017, 7, 406. [Google Scholar] [CrossRef]
- Choi, K.-B.; Lee, J.J.; Hata, S. A piezo-driven compliant stage with double mechanical amplification mechanisms arranged in parallel. Sens. Actuators A Phys. 2010, 161, 173–181. [Google Scholar] [CrossRef]





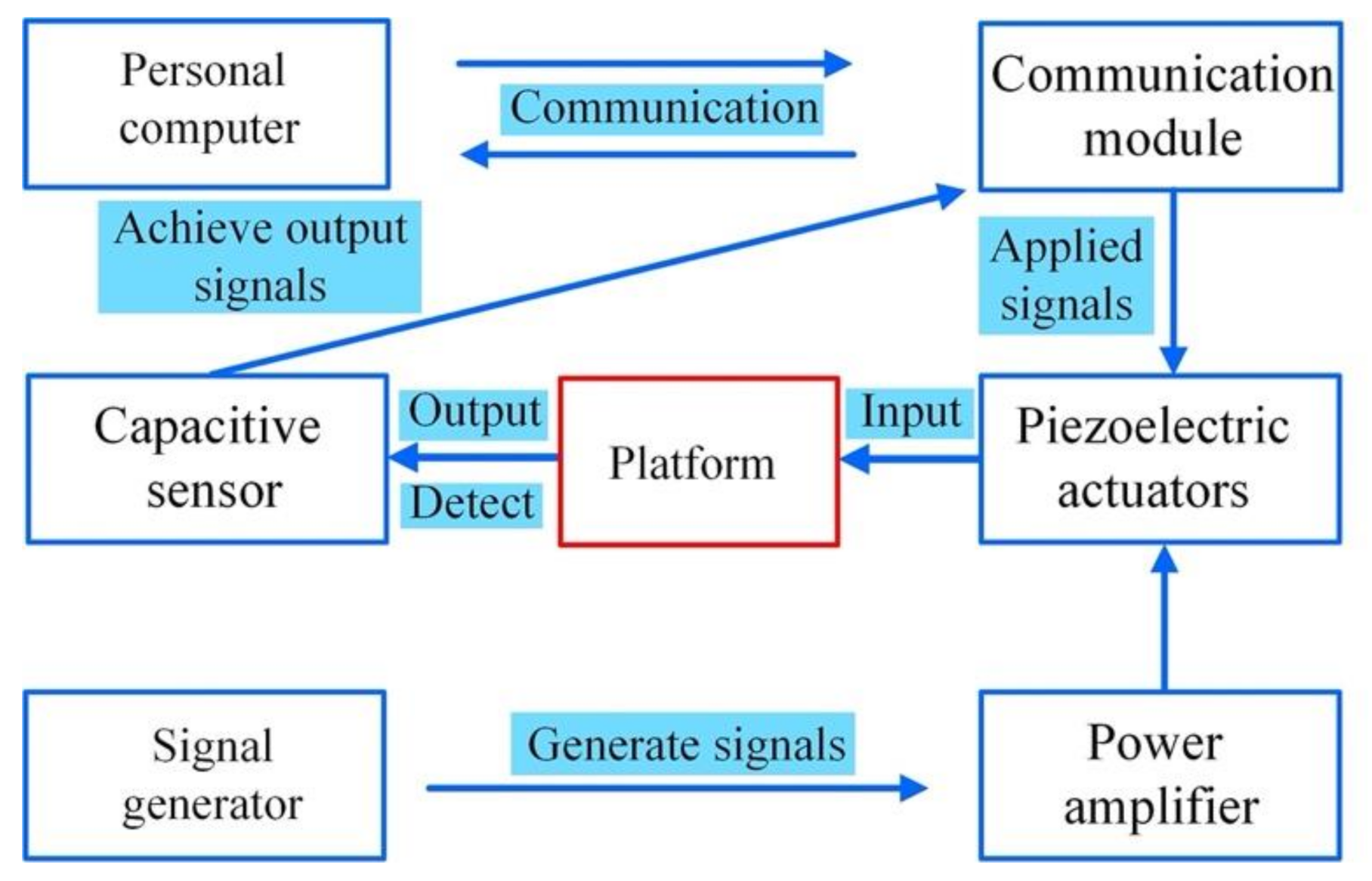
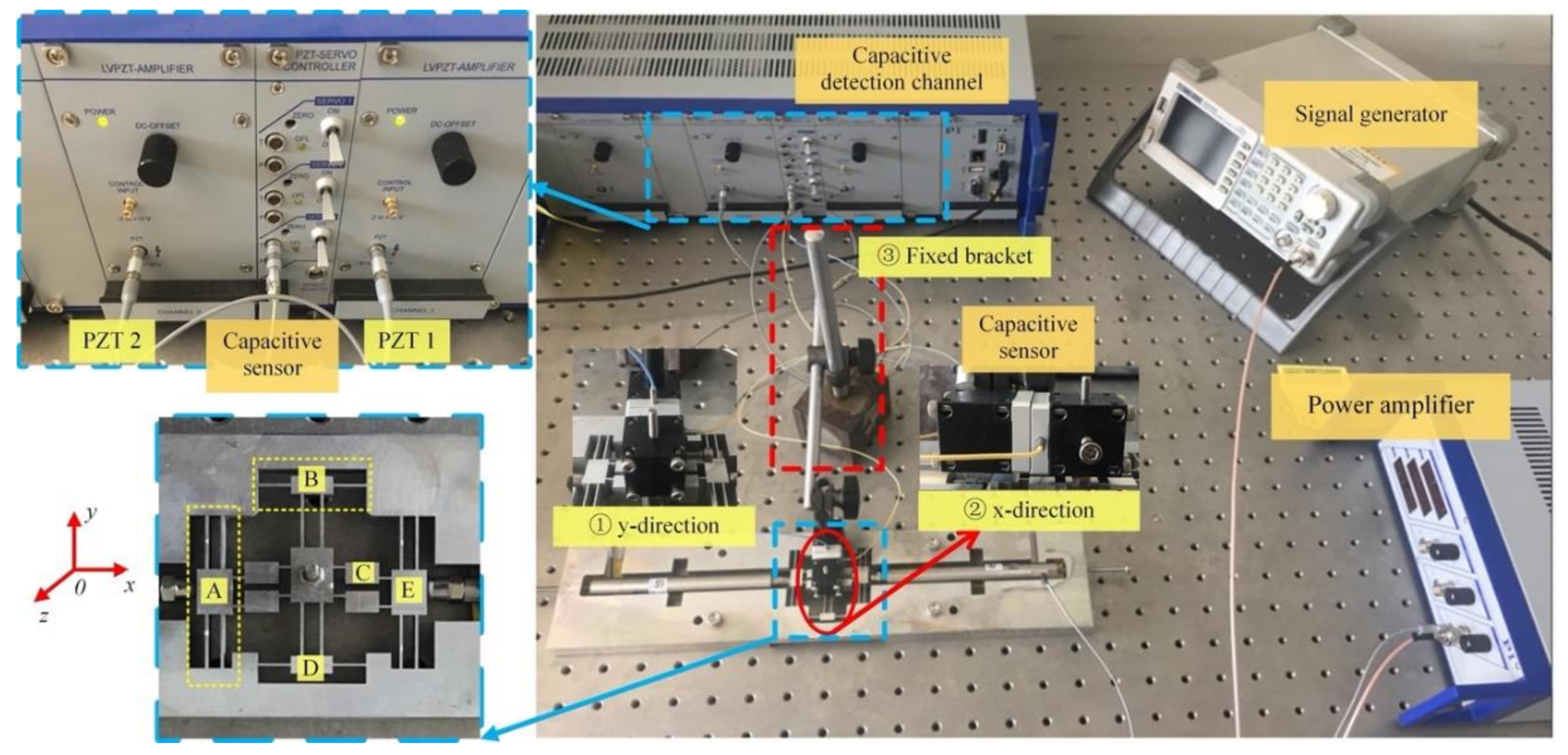





| Part | Symbol | Length (mm) |
|---|---|---|
| Part 1 | 0.7 | |
| 10 | ||
| 15 | ||
| Part 2 | 0.7 | |
| 3 | ||
| Part 3 | 0.7 | |
| 8 | ||
| Part 4 | 6 | |
| 8 | ||
| Part 5 | 0.7 | |
| 15 |
| Material | Density ρ (kg/m3) | Young’s Modulus E (GPa) | Poisson Ratio ν | Shear Modulus G (GPa) |
|---|---|---|---|---|
| Aluminum | 2.7 × 103 | 70 | 0.36 | 25.6 |
| Axis | Theory (μm) | Simulation (μm) | Error (%) |
|---|---|---|---|
| 78 | 76.3 | 2.2 | |
| 26.3 | 24.9 | 5.3 |
| Modal Analysis | First | Second | Third | Fourth | Fifth |
|---|---|---|---|---|---|
| Frequency (Hz) | 1088.9 | 2240.1 | 5989.9 | 7866.9 | 10,739 |
| Parameters | P-842.60 | Unit | Tolerance |
|---|---|---|---|
| Travel range at 0 to 100 V | 90 | μm | ±20% |
| Resolution | 0.9 | nm | |
| Static large-signal stiffness | 10 | N/μm | ±20% |
| Push force capacity | 800 | N | max. |
| Pull force capacity | 300 | N | max. |
| Torque on tip | 0.35 | Nm | max. |
| Electrical capacitance | 9 | μF | ±20% |
| Resonant frequency f0 (no load) | 6 | kHz | ±20% |
| Operating temperature range | −40 to 80 | °C | |
| Mass without cable | 86 | g | ±5% |
| Length L | 127 | mm | ±0.2 mm |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jiao, C.; Wang, Z.; Lv, B.; Wang, G.; Yue, W. Design and Analysis of a Novel Flexure-Based XY Micropositioning Stage. Appl. Sci. 2020, 10, 8336. https://doi.org/10.3390/app10238336
Jiao C, Wang Z, Lv B, Wang G, Yue W. Design and Analysis of a Novel Flexure-Based XY Micropositioning Stage. Applied Sciences. 2020; 10(23):8336. https://doi.org/10.3390/app10238336
Chicago/Turabian StyleJiao, Chenlei, Zhe Wang, Bingrui Lv, Guilian Wang, and Weiliang Yue. 2020. "Design and Analysis of a Novel Flexure-Based XY Micropositioning Stage" Applied Sciences 10, no. 23: 8336. https://doi.org/10.3390/app10238336
APA StyleJiao, C., Wang, Z., Lv, B., Wang, G., & Yue, W. (2020). Design and Analysis of a Novel Flexure-Based XY Micropositioning Stage. Applied Sciences, 10(23), 8336. https://doi.org/10.3390/app10238336





