Investigation on Distributed Vibration Damping of Bridge Based on Energy Harvesting Technique and Finite Element Analysis
Abstract
:1. Introduction
2. Theoretical Analysis of Distributed Vibration Reduction and Electromagnetic Energy Collection for Bridges
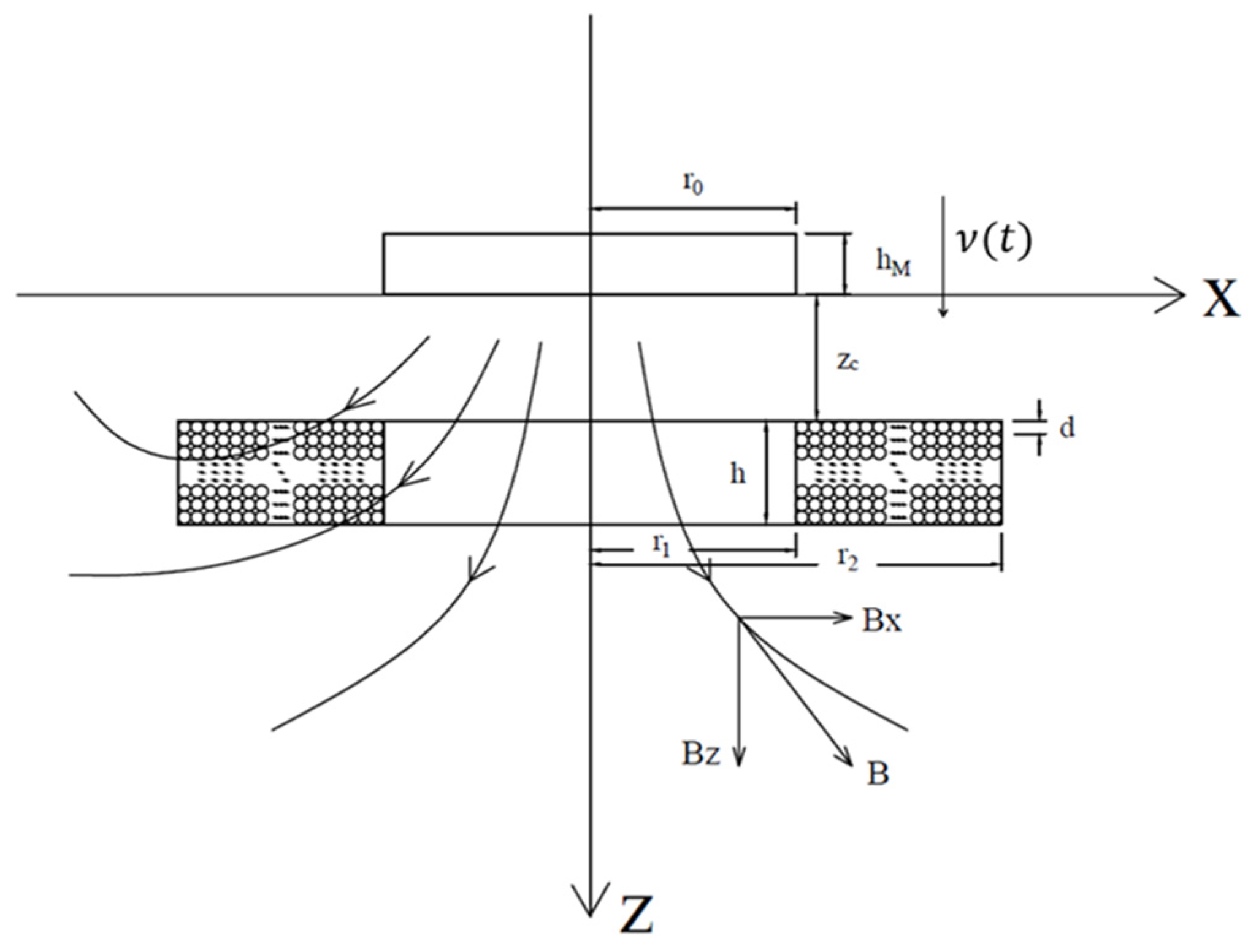
2.1. Electromotive Force of Magnetic Induction Coil
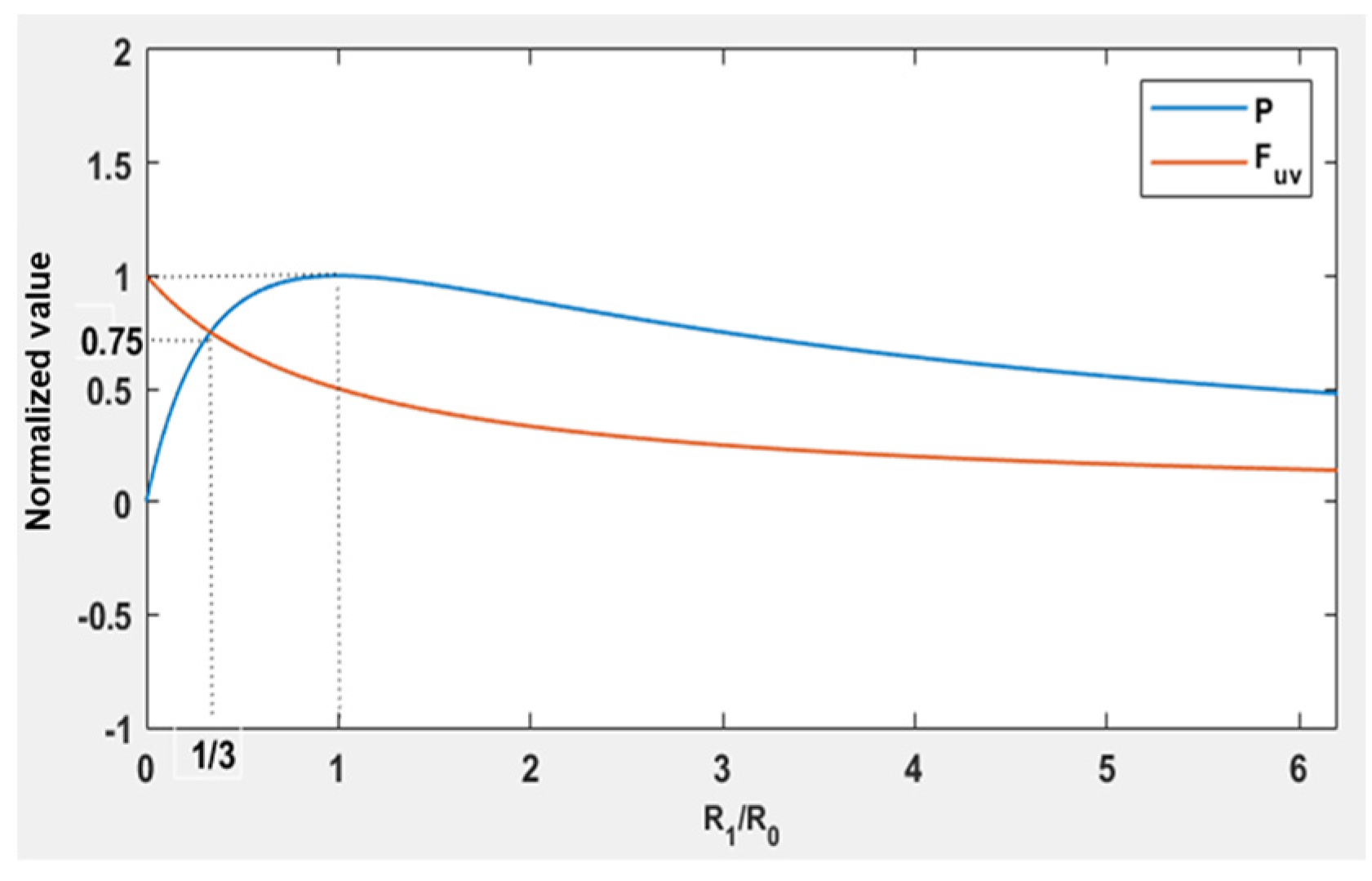
2.2. Coil Output Power and Electromagnetic Damping Force
2.3. Calculation of Structural Vibration
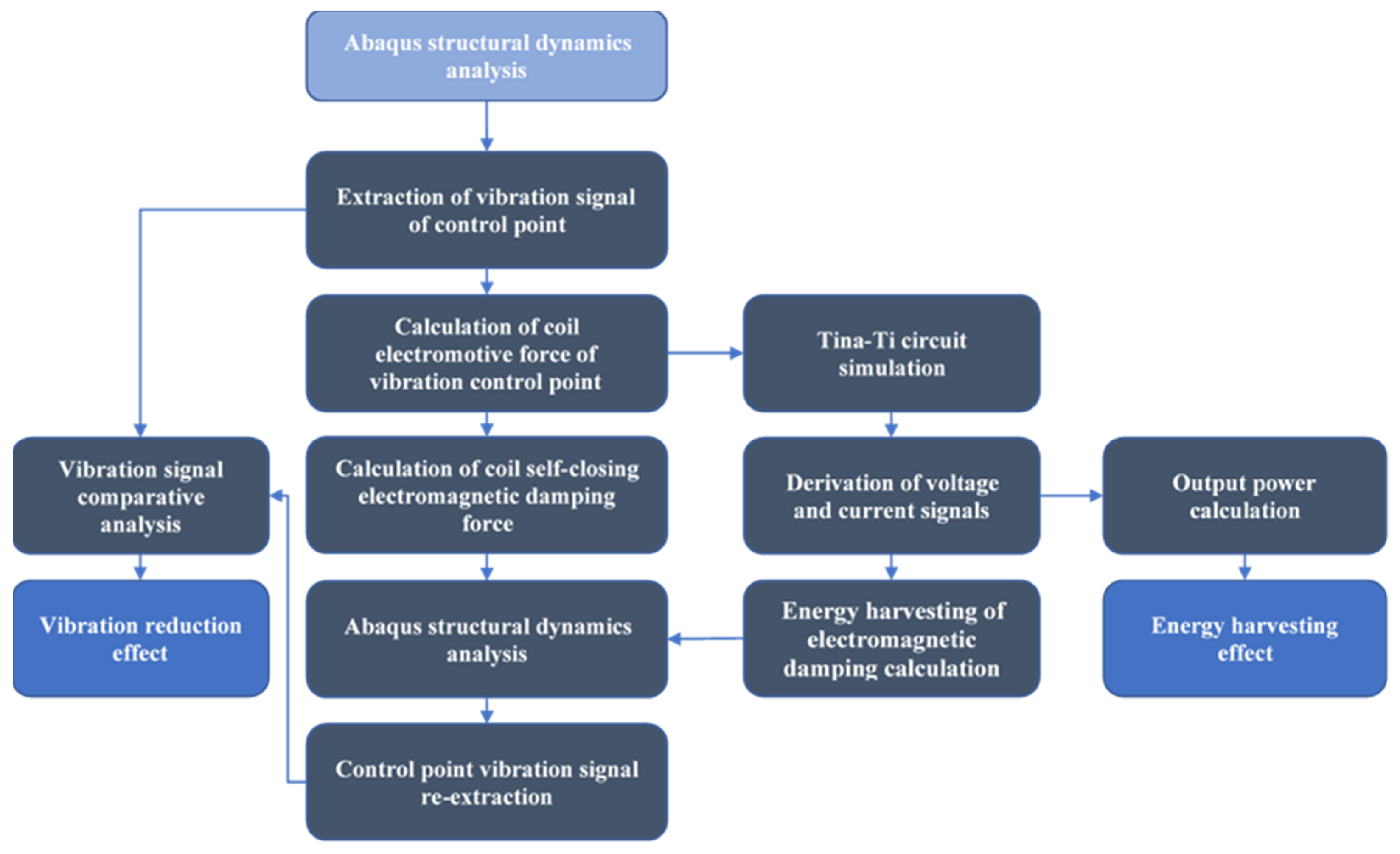
3. Joint Simulation Process and Modelling of Vibration and Circuit
3.1. Simulation Process
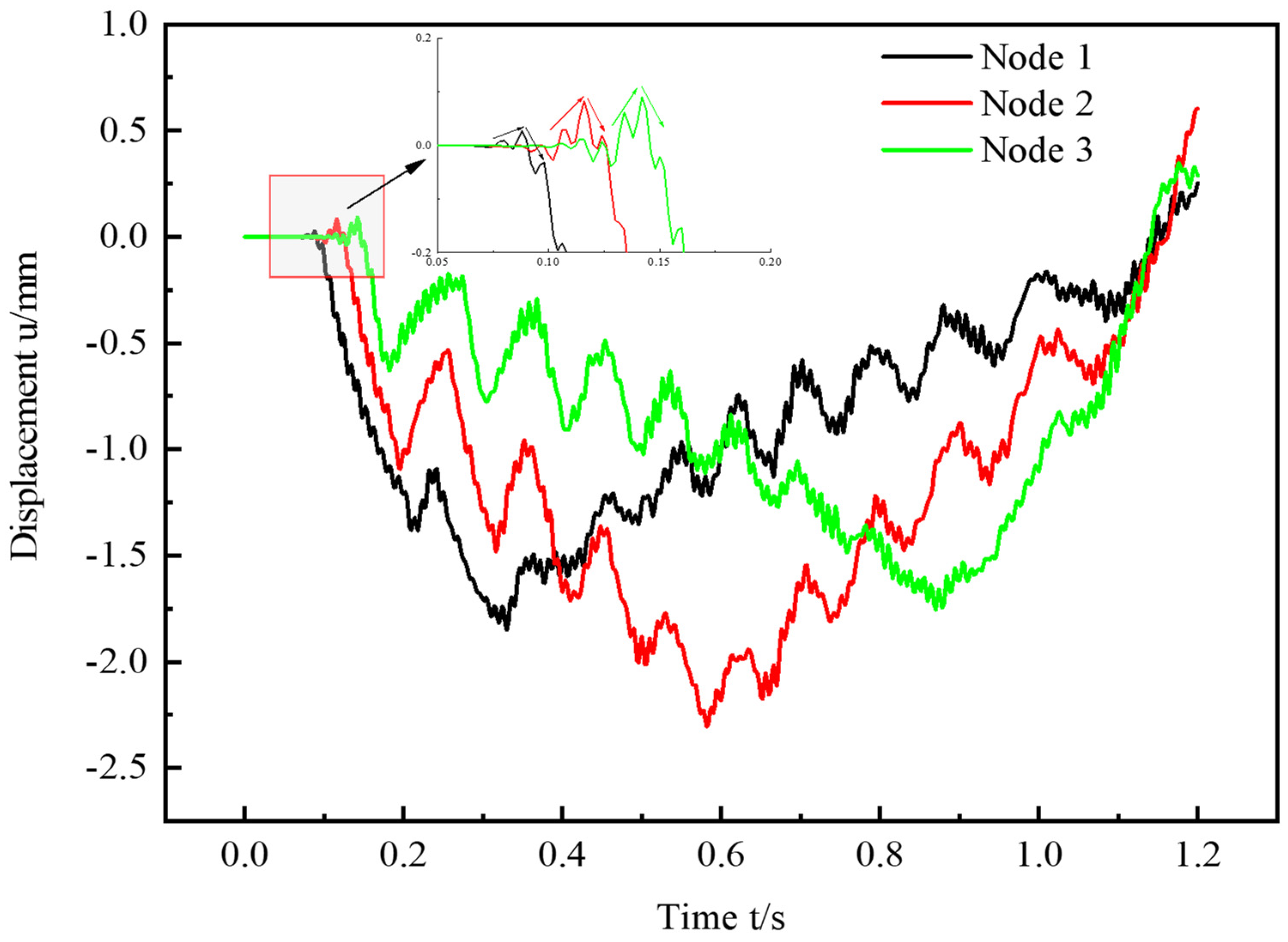
3.2. Finite Element Simulation of Vibration Signal
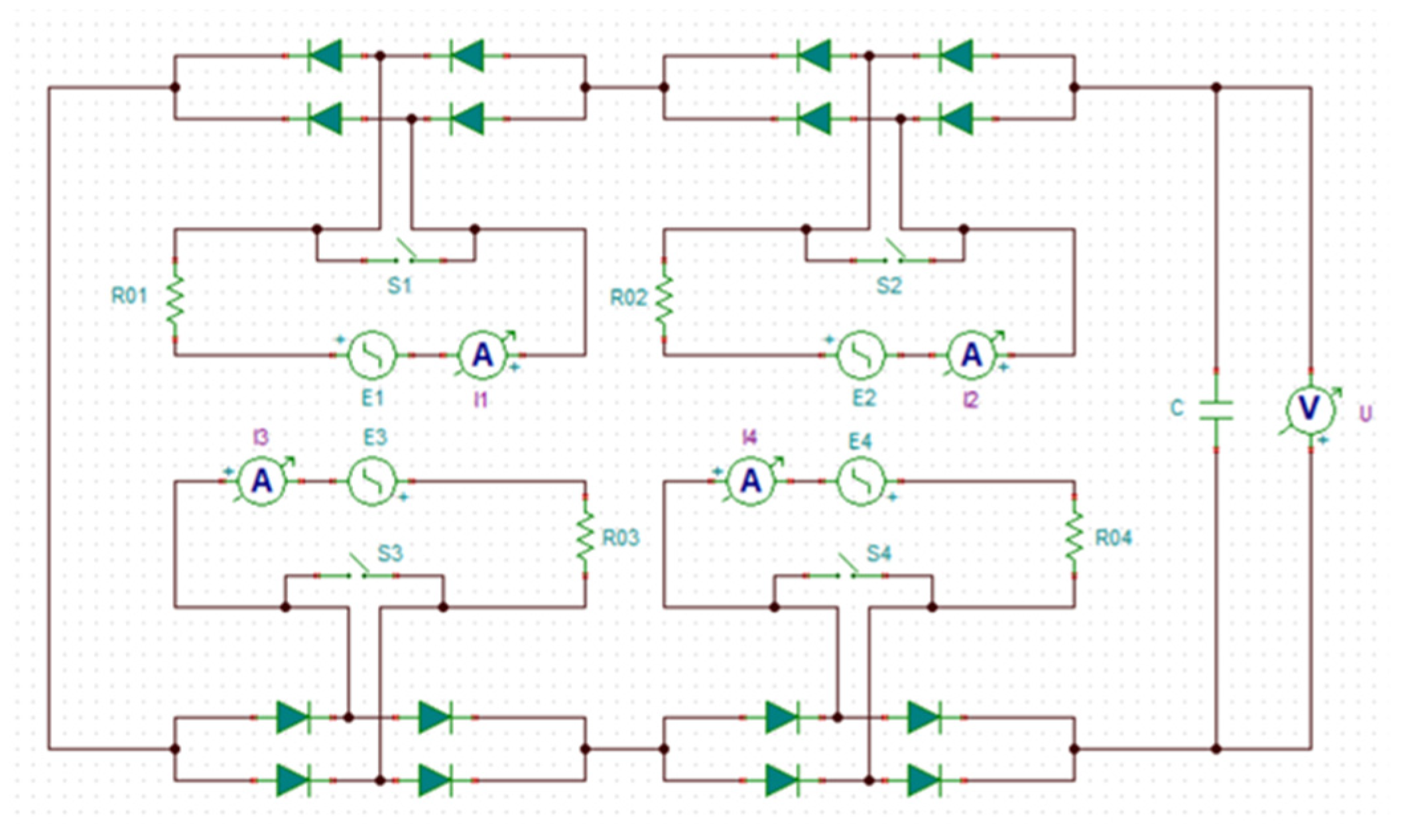
3.3. Design of Array Energy Acquisition Circuit
4. Results and Discussion
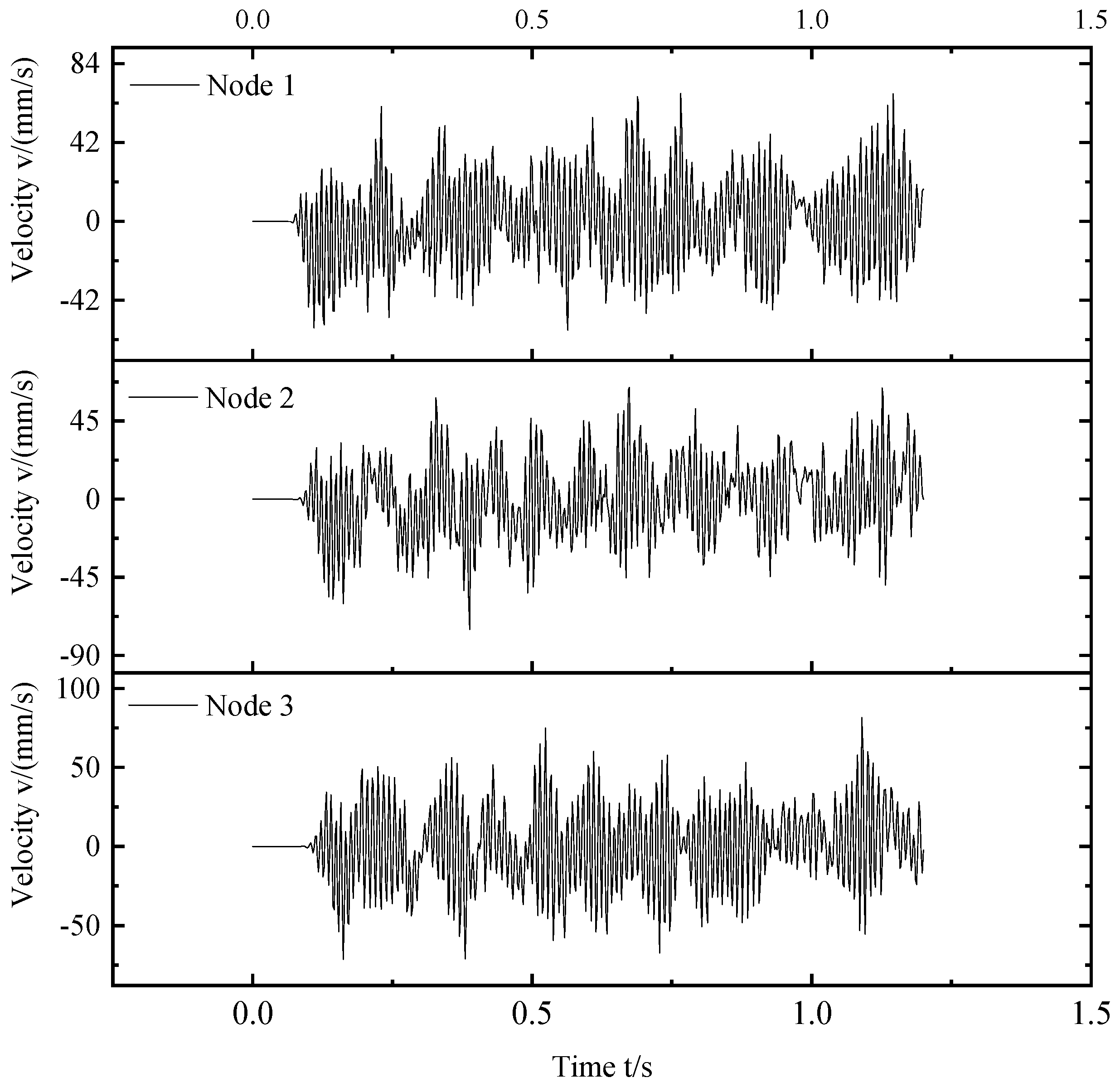
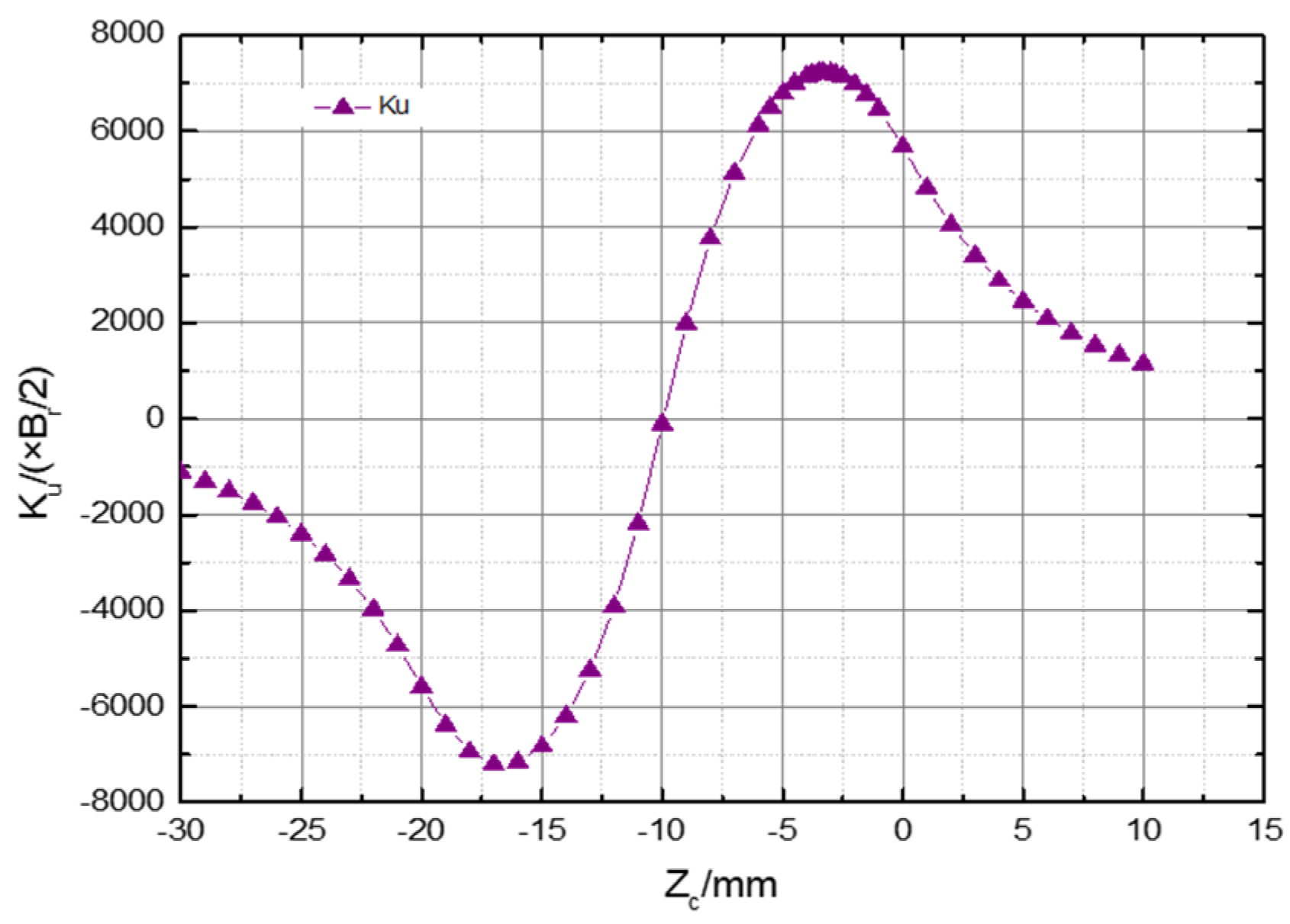
4.1. Vibration Signal Extraction and Electromotive Force Calculation
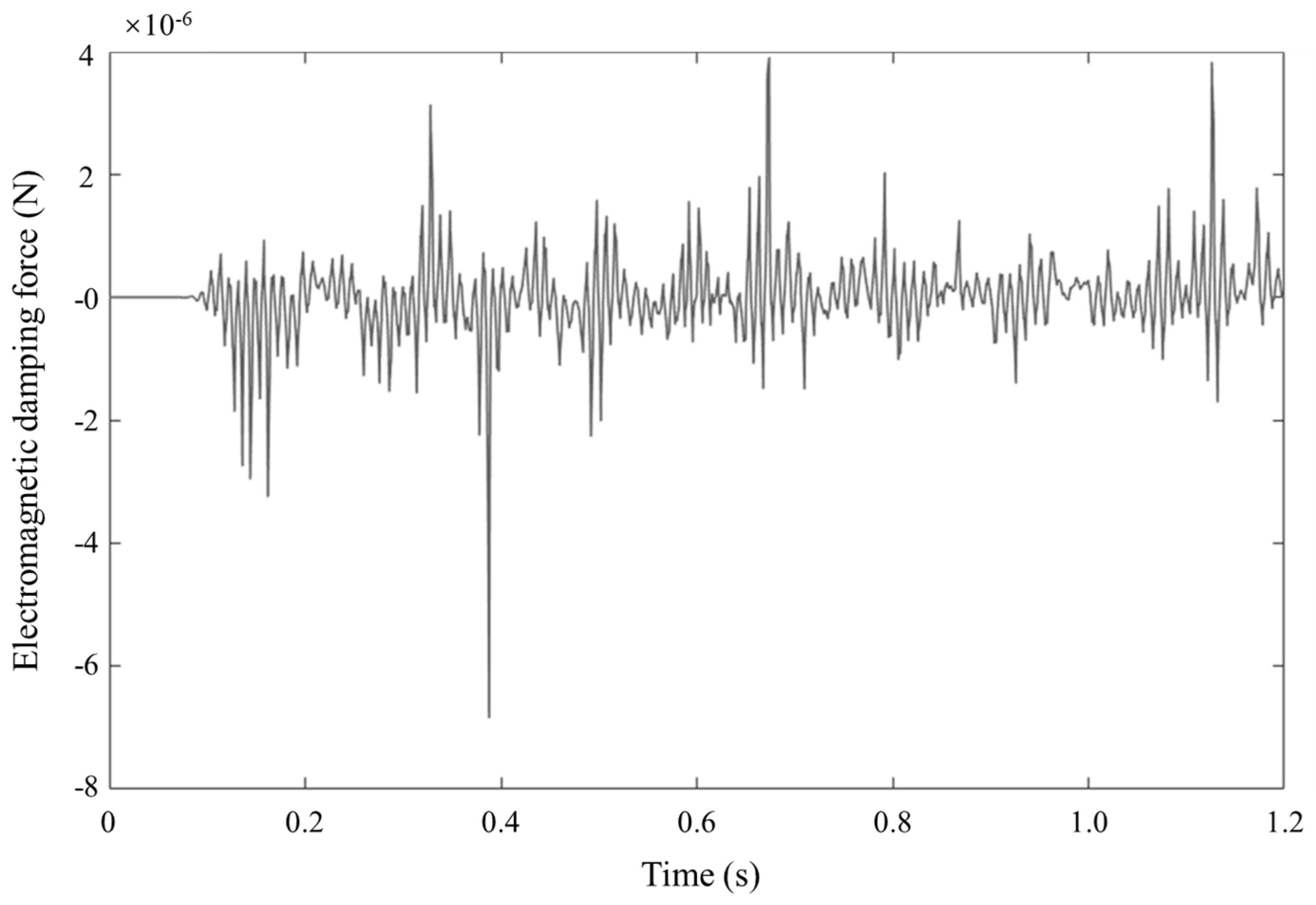
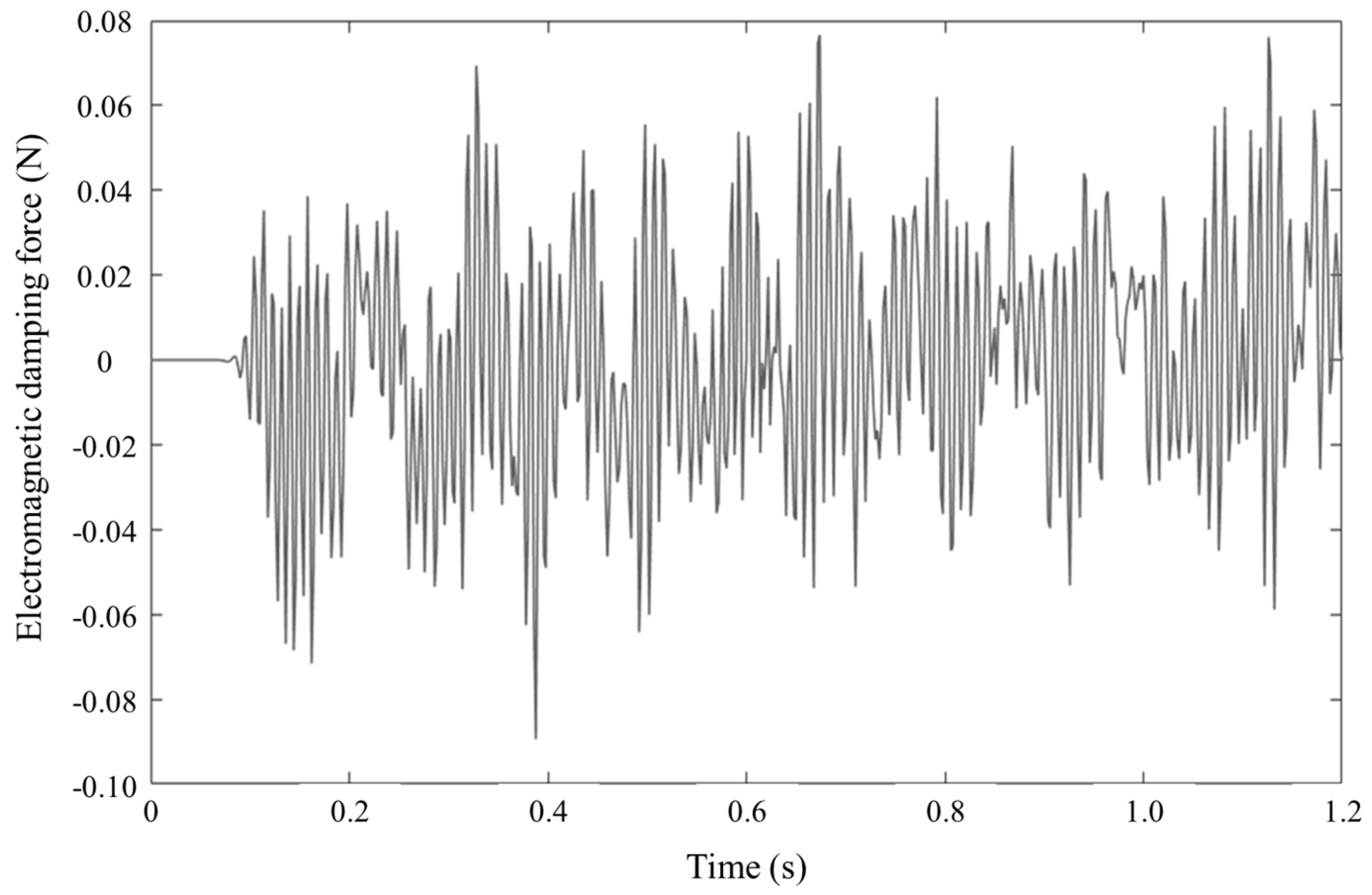
4.2. Simulation Analysis of Energy Harvesting Circuit
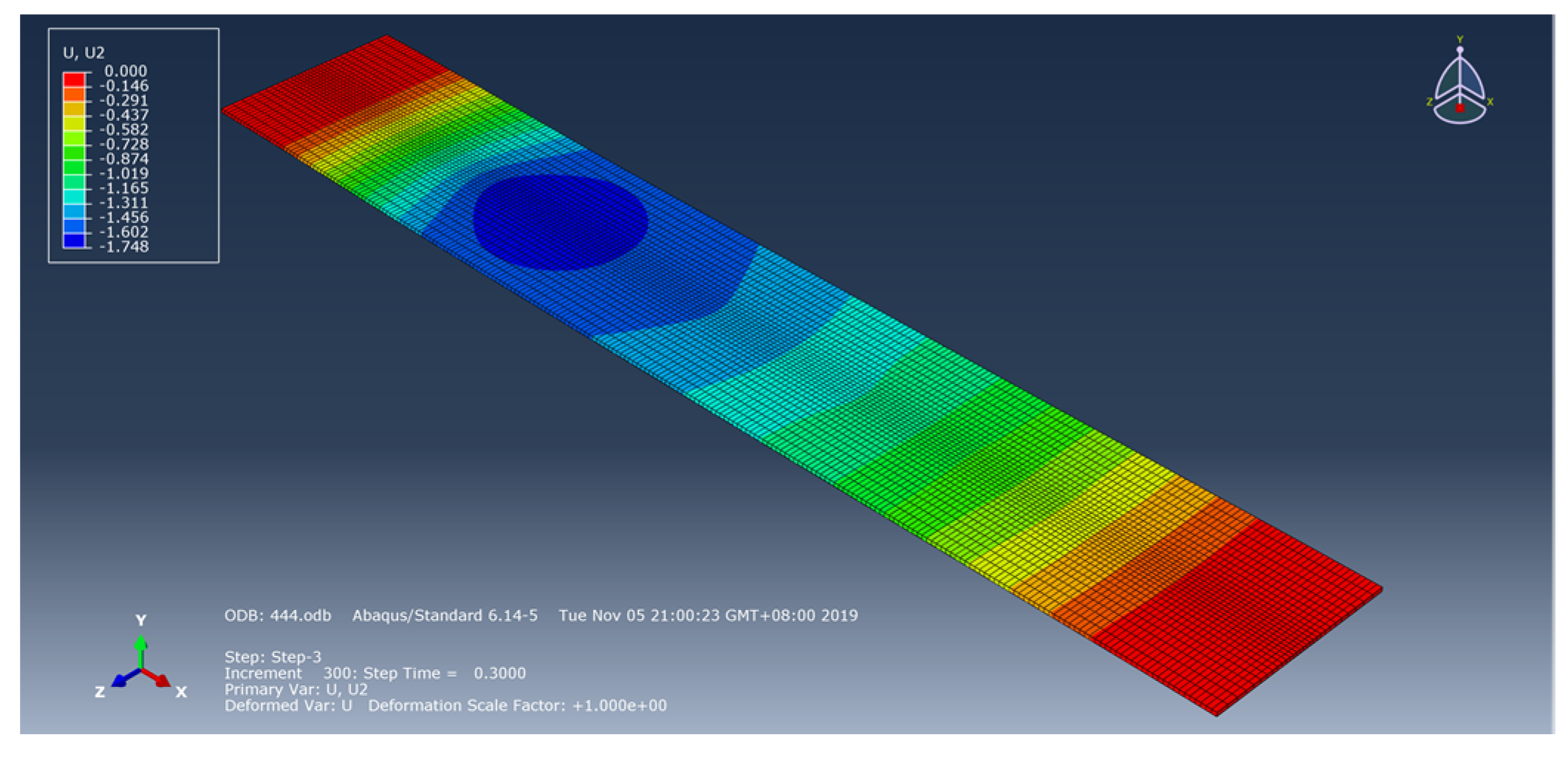
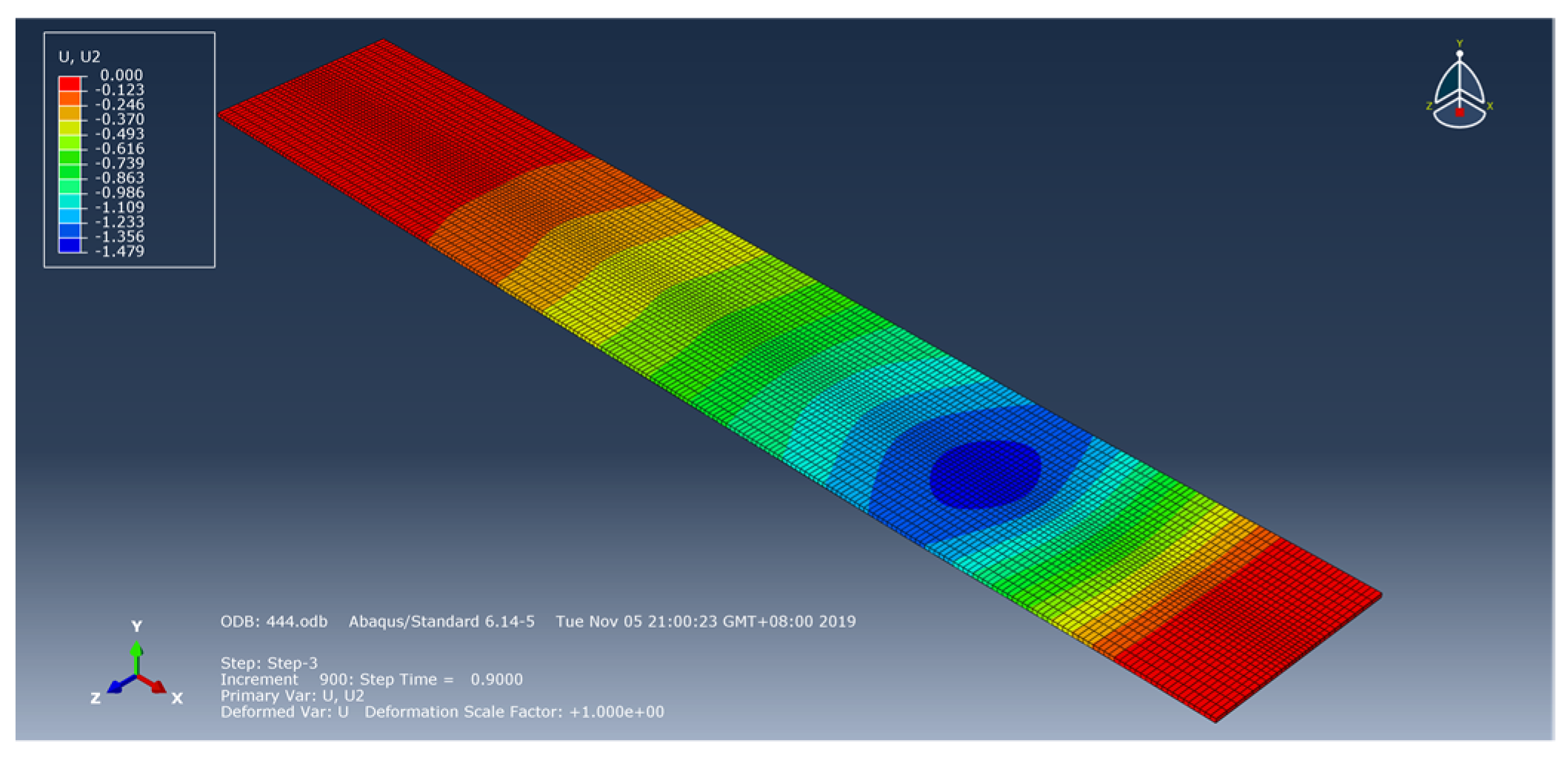
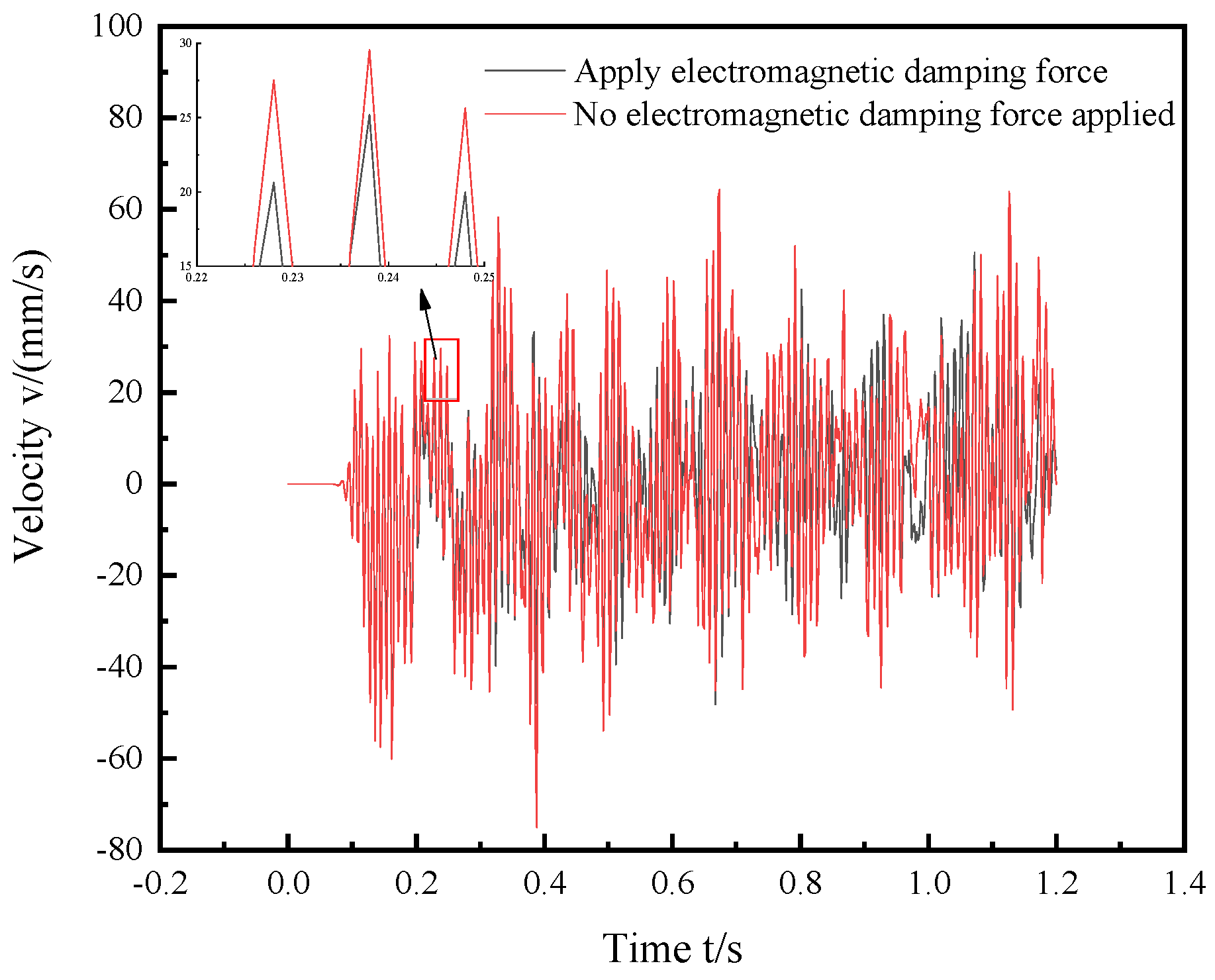
4.3. Finite Element Simulation Analysis of System Vibration Reduction
5. Conclusions
- Through the theoretical analysis, the calculation formulas of the electromotive force, output power and maximum electromagnetic damping force of the energy acquisition circuit were obtained.
- By programming MATLAB, based on the study of the composition of the energy acquisition circuit, the corresponding array energy acquisition circuit was designed according to the structure of the array coil permanent magnet. By calculating the instantaneous power of the energy collection of the design circuit, the average instantaneous power collected by the design method was 1.093 × 10−9 W.
- The initial vibration signal of the target node was obtained through analysis. The vibration signals of the node before and after the electromagnetic damping were obtained through a joint simulation. The acceleration signal energies before and after energy harvesting were calculated to be 3.1048 × 108 and 3.1044 × 108, and the reduction rate of the vibration energy before and after the node was 0.01%. The velocity signal energies before and after energy harvesting were 5.9724 × 102 and 5.9750 × 102, and the reduction rate of the vibration energy before and after the node was 0.02%.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Tong, X.; Hou, Y.; Dong, Y.; Zhang, Y.; Yang, H.; Qian, Z. Research and Development of a Wireless Self-Powered Sensing Device Based on Bridge Vibration Energy Collection. Sensors 2021, 21, 8319. [Google Scholar] [CrossRef] [PubMed]
- Peigney, M.; Siegert, D. Piezoelectric energy harvesting from traffic-induced bridge vibrations. Smart Mater. Struct. 2013, 22, 095019. [Google Scholar] [CrossRef] [Green Version]
- McEvoy, T.; Dierks, E.; Weaver, J.; Inamdar, S.; Zimowski, K.; Wood, K.L.; Crawford, R.H.; Jensen, D. Developing innovative energy harvesting approaches for infrastructure health monitoring systems. In Proceedings of the 37th Design Automation Conference, Parts A and B, Washington, DC, USA, 28–31 August 2011. [Google Scholar]
- Rhimi, M.; Lajnef, N. Tunable energy harvesting from ambient vibrations in civil structures. J. Energy Eng. 2012, 138, 185–193. [Google Scholar] [CrossRef]
- Wang, H.; Tang, L. Modeling and experiment of bistable two-degree-of-freedom energy harvester with magnetic coupling. Mech. Syst. Signal Process. 2017, 86, 29–39. [Google Scholar] [CrossRef]
- Zhang, Y.; Cai, C.S.; Zhang, W. Experimental study of a multi-impact energy harvester under low frequency excitations. Smart Mater. Struct. 2014, 23, 055002. [Google Scholar] [CrossRef]
- Williams, C.B.; Yates, R.B. Analysis of a micro-electric generator for microsystems. Sens. Actuators A Phys. 1996, 52, 8–11. [Google Scholar] [CrossRef]
- Wang, L.; Yuan, F.G. Vibration energy harvesting by magnetostrictive material. Smart Mater. Struct. 2008, 17, 45009–45014. [Google Scholar] [CrossRef]
- Adly, A.; Davino, D.; Giustiniani, A.; Visone, C. Experimental tests of a magnetostrictive energy harvesting device and its modeling. J. Appl. Phys. 2010, 107, 09A935. [Google Scholar] [CrossRef]
- Marin, A.; Tadesse, Y.; Priya, S. Multi-mechanism non-linear vibration harvester combining inductive and magnetostrictive mechanisms. Integr. Ferroelectr. 2013, 148, 27–52. [Google Scholar] [CrossRef]
- Miao, S. Energy Harvesting from Wind-Induced Vibration of Suspension Bridges. Master’s Thesis, Nanyang Technological University, Singapore, 2012. [Google Scholar]
- Jo, B.W.; Lee, Y.S.; Yun, G.W.; Park, C.; Kim, J.W. Vibration-induced energy harvesting for green technology. In Proceedings of the 4th International Conference on Chemical, Environment and Civil Engineering (ICCECE), Manila, Philippines, 17–18 November 2012. [Google Scholar]
- Kwon, S.D.; Park, J.; Law, K. Electromagnetic energy harvester with repulsively stacked multilayer magnets for low frequency vibrations. Smart Mater. Struct. 2013, 22, 055007. [Google Scholar] [CrossRef]
- Ahmad, I.; Khan, F.U. Vibration-based electromagnetic type energy harvester for bridge monitoring sensor application. In Proceedings of the 10th International Conference on Emerging Technologies (ICET), Islamabad, Pakistan, 8–9 December 2014. [Google Scholar]
- Yang, B.; Lee, C.; Xiang, W.; Xie, J.; He, J.H.; Kotlanka, R.K.; Low, S.P.; Feng, H. Electromagnetic energy harvesting from vibrations of multiple frequencies. J. Micromech. Microeng. 2009, 19, 035001. [Google Scholar] [CrossRef]
- Mohammadi, S.; Esfandiari, A. Magnetostrictive vibration energy harvesting using strain energy method. Energy 2015, 81, 519–525. [Google Scholar] [CrossRef]
- Mori, K.; Horibe, T.; Ishikawa, S.; Shindo, Y.; Narita, F. Characteristics of vibration energy harvesting using giant magnetostrictive cantilevers with resonant tuning. Smart Mater. Struct. 2015, 24, 125032. [Google Scholar] [CrossRef]
- Ueno, T.; Yamada, S. Performance of energy harvester using Iron-Gallium alloy in Free Vibration. IEEE Trans. Magn. 2011, 47, 2407–2409. [Google Scholar] [CrossRef]
- Zuo, L. Effective and robust vibration control using series multiple tuned-mass dampers. J. Vib. Acoust. 2009, 131, 031003. [Google Scholar] [CrossRef]
- Ni, T.; Zuo, L.; Kareem, A. Assessment of energy potential and vibration mitigation of regenerative tuned mass dampers on wind excited tall buildings. In Proceedings of the ASME 2011 International Design Engineering Technical Conferences and Computers and Information in Engineering Conference, Washington, DC, USA, 28–31 August 2011. [Google Scholar]
- Harne, R.L. Modeling and analysis of distributed electromagnetic oscillators for broadband vibration attenuation and concurrent energy harvesting. Appl. Math. Model. 2013, 37, 4360–4370. [Google Scholar] [CrossRef]
- Liu, P.; Zhao, Q.; Yang, H.; Wang, D.; Oeser, M.; Wang, L.; Tan, Y. Numerical study on influence of piezoelectric energy harvester on asphalt pavement structural responses. J. Mater. Civ. Eng. 2019, 31, 04019008. [Google Scholar] [CrossRef]
- Liu, P.; Wang, D.; Otto, F.; Oeser, M. Application of semi-analytical finite element method on analyzing bearing capacity of asphalt pavements under moving loads. Front. Struct. Civ. Eng. 2018, 12, 215–221. [Google Scholar] [CrossRef]
- Du, C.; Liu, P.; Jin, C.; Yang, H.; Li, C.; Jiang, G.; Oeser, M. Evaluation of the piezoelectric and mechanical behaviors of asphalt pavements embedded with a piezoelectric energy harvester based on multiscale finite element simulations. Constr. Build. Mater. 2022, 333, 127438. [Google Scholar] [CrossRef]
- Yang, H.; Zhao, Q.; Guo, X.; Zhang, W.; Liu, P.; Wang, L. Numerical analysis of signal response characteristic of piezoelectric energy harvesters embedded in pavement. Materials 2020, 13, 2770. [Google Scholar] [CrossRef] [PubMed]
- Oppenheim, A.V.; Willsky, A.S.; Nawab, S.H. Signals and Systems, 2nd ed.; Prentice-Hall: Upper Saddle River, NJ, USA, 1996; pp. 5–7. [Google Scholar]

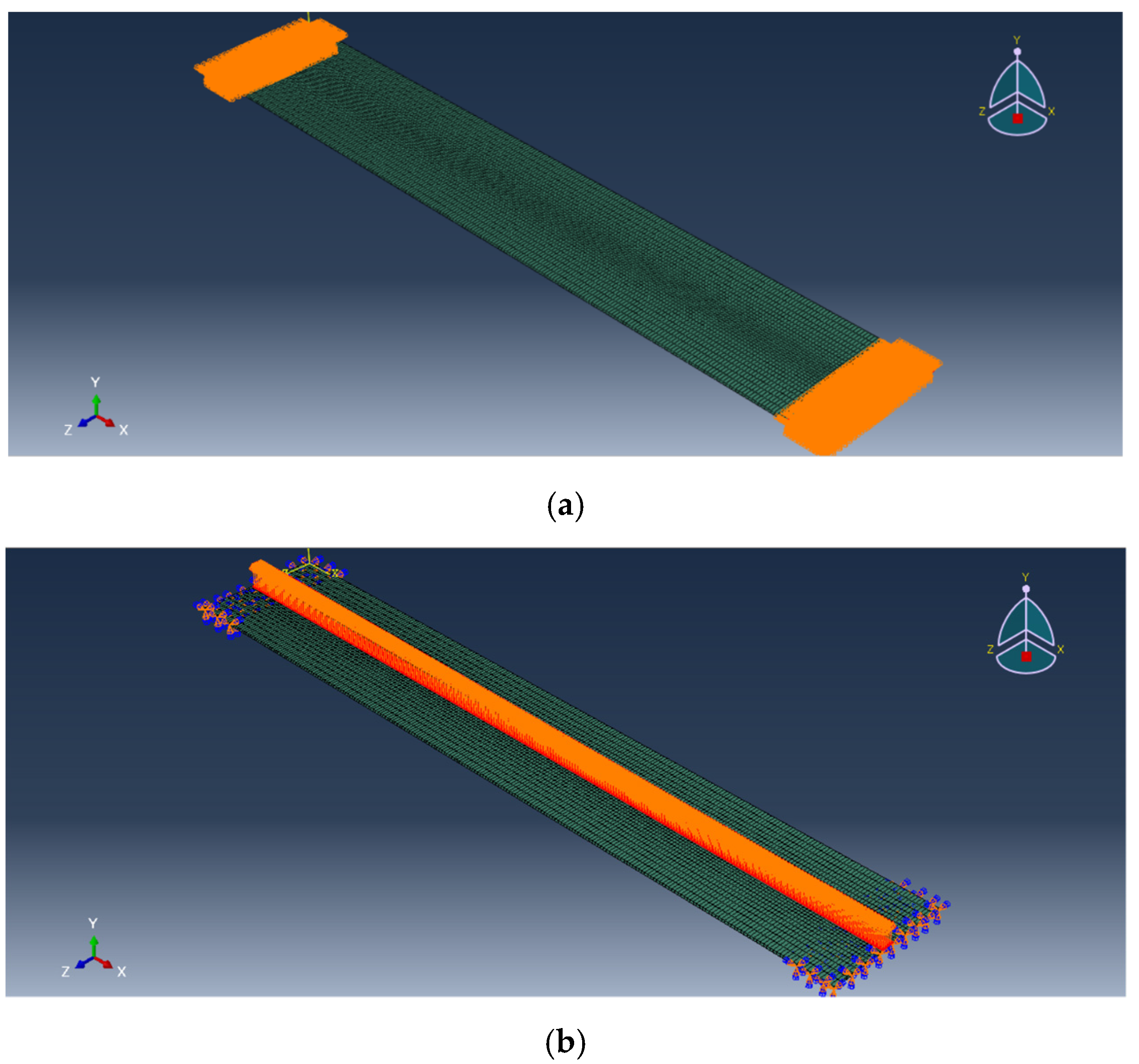





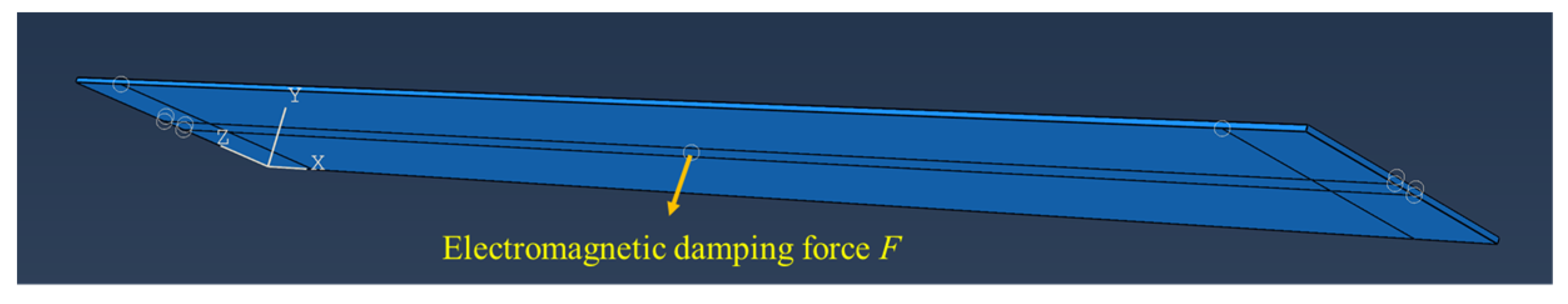

| Parameters | Symbol | Unit | Value |
|---|---|---|---|
| Young’s modulus | Y | MPa | 3000 |
| Poisson’s rate | ν | / | 0.4 |
| Density | ρ | g/cm3 | 1.18 |
| Rayleigh damping coefficients | α | / | 0.267 |
| β | / | 0.001 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, H.; Chen, Q.; Liu, H.; Chang, H.; Yang, S.-H.; Wang, L.; Liu, P. Investigation on Distributed Vibration Damping of Bridge Based on Energy Harvesting Technique and Finite Element Analysis. Appl. Sci. 2023, 13, 382. https://doi.org/10.3390/app13010382
Yang H, Chen Q, Liu H, Chang H, Yang S-H, Wang L, Liu P. Investigation on Distributed Vibration Damping of Bridge Based on Energy Harvesting Technique and Finite Element Analysis. Applied Sciences. 2023; 13(1):382. https://doi.org/10.3390/app13010382
Chicago/Turabian StyleYang, Hailu, Qun Chen, Huifang Liu, Haoran Chang, Shih-Hsien Yang, Linbing Wang, and Pengfei Liu. 2023. "Investigation on Distributed Vibration Damping of Bridge Based on Energy Harvesting Technique and Finite Element Analysis" Applied Sciences 13, no. 1: 382. https://doi.org/10.3390/app13010382
APA StyleYang, H., Chen, Q., Liu, H., Chang, H., Yang, S.-H., Wang, L., & Liu, P. (2023). Investigation on Distributed Vibration Damping of Bridge Based on Energy Harvesting Technique and Finite Element Analysis. Applied Sciences, 13(1), 382. https://doi.org/10.3390/app13010382








