Determination of Subgrade Reaction Modulus Considering the Relative Stiffnesses of Soil–Foundation Systems
Abstract
:1. Introduction
2. Materials and Methods
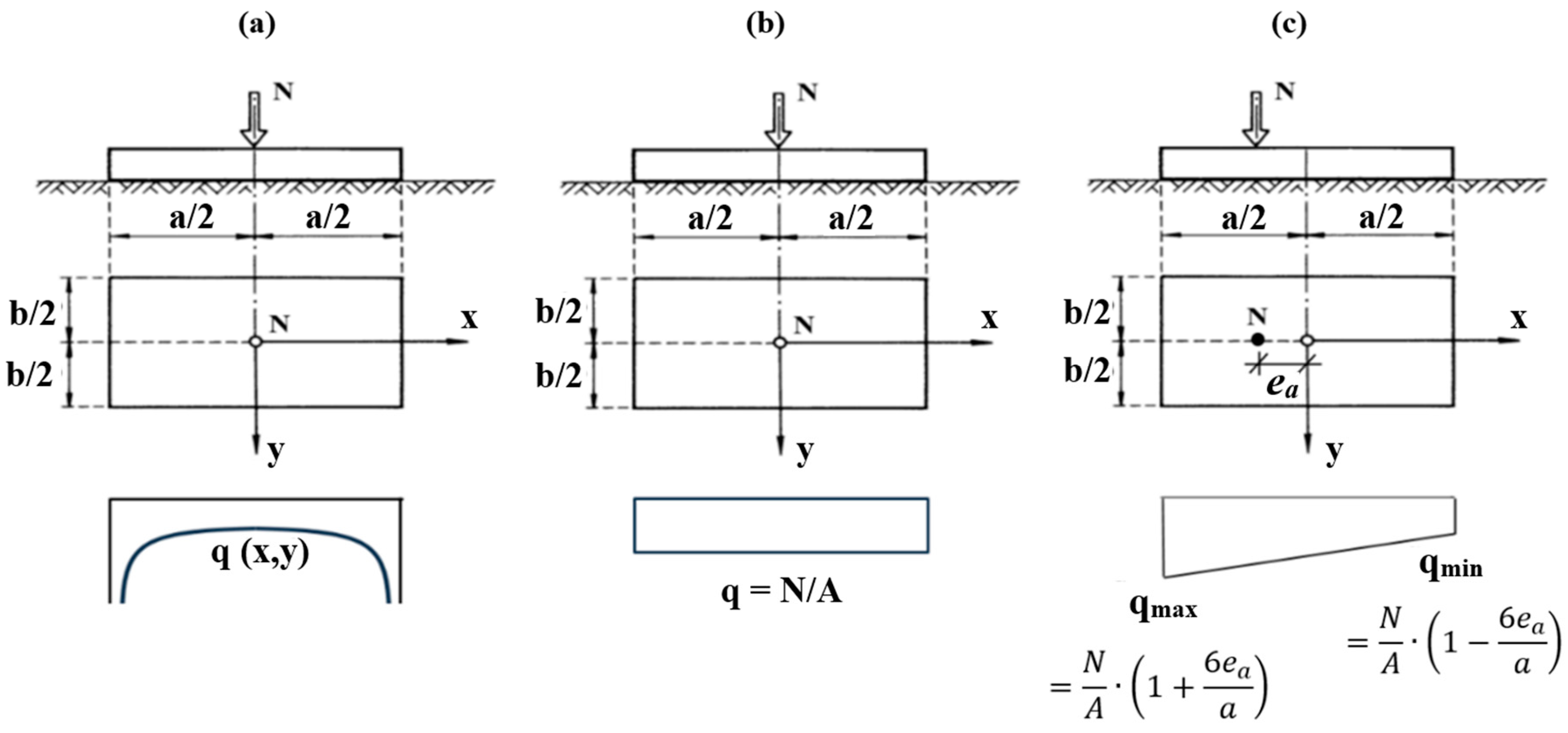
2.1. Determination of Subgrade Reaction Modulus Considering the Soil–Foundation Interaction
2.2. Soil Model
2.3. Properties of Foundations
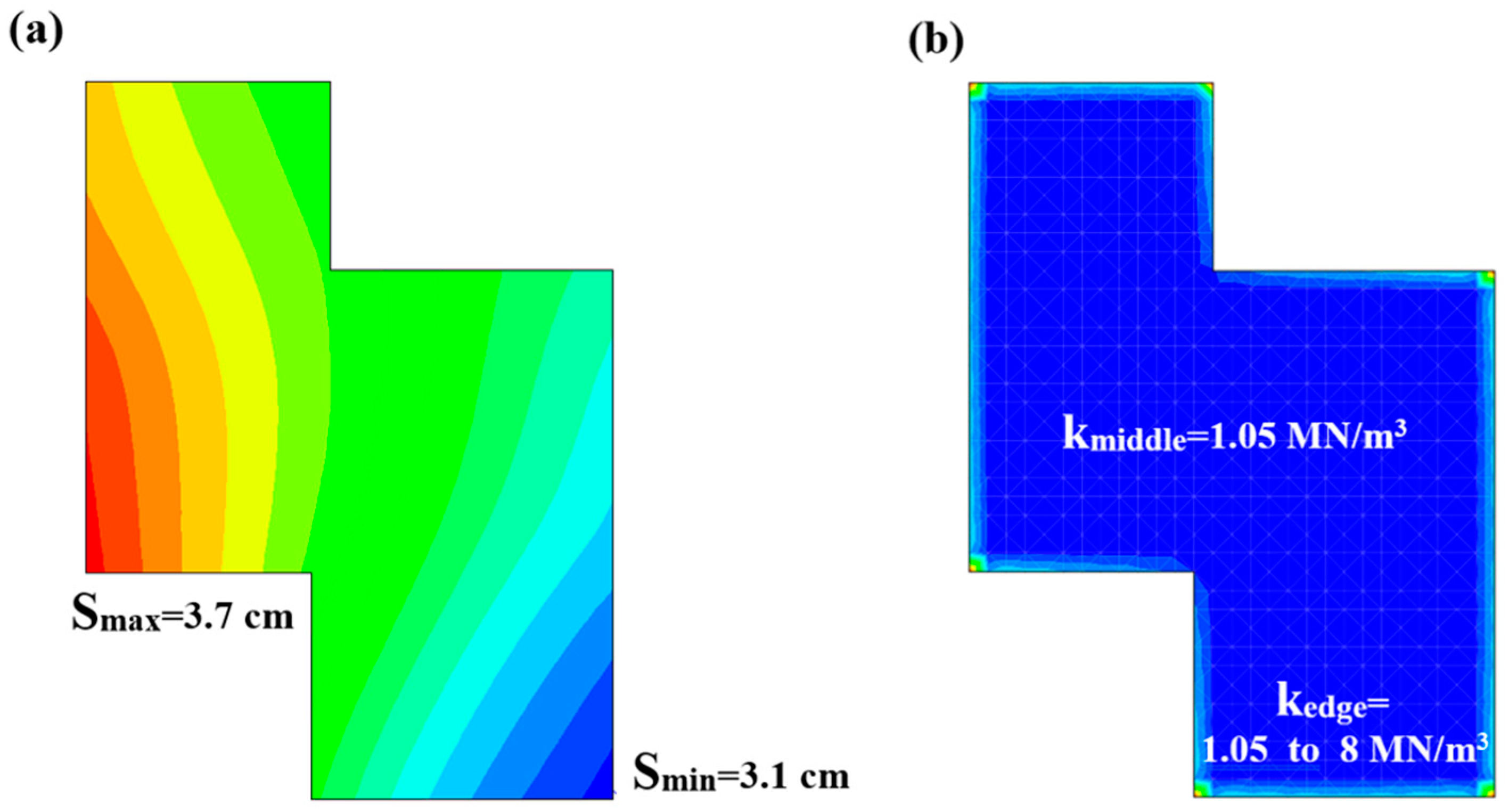
3. Results and Discussion
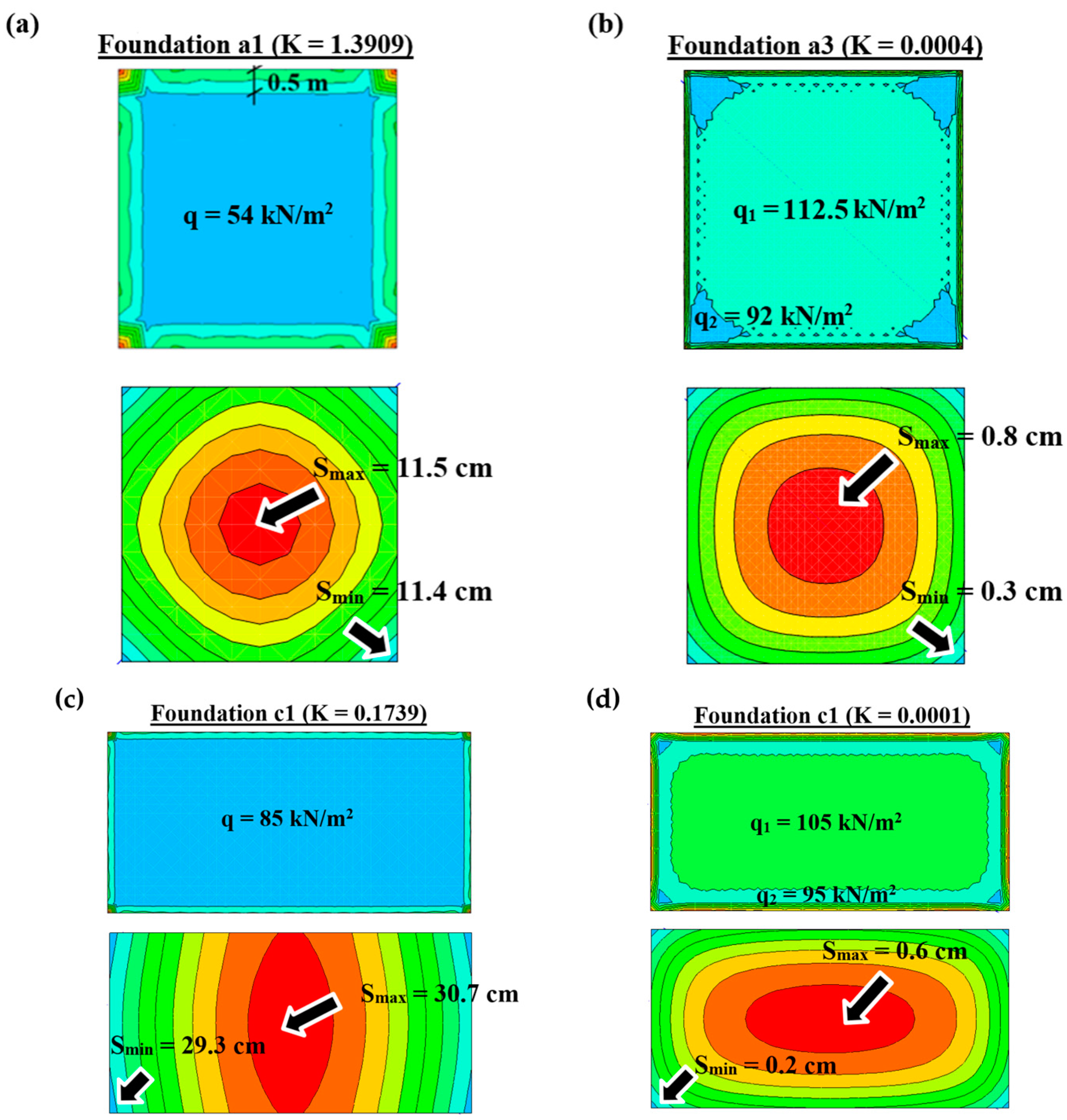
3.1. FE Analyses
3.2. Comparison of Subgrade Reaction Moduli Obtained from the Finite Element Analyses and Analytical Method Considering the Relative Stiffnesses of Soil–Foundation Systems
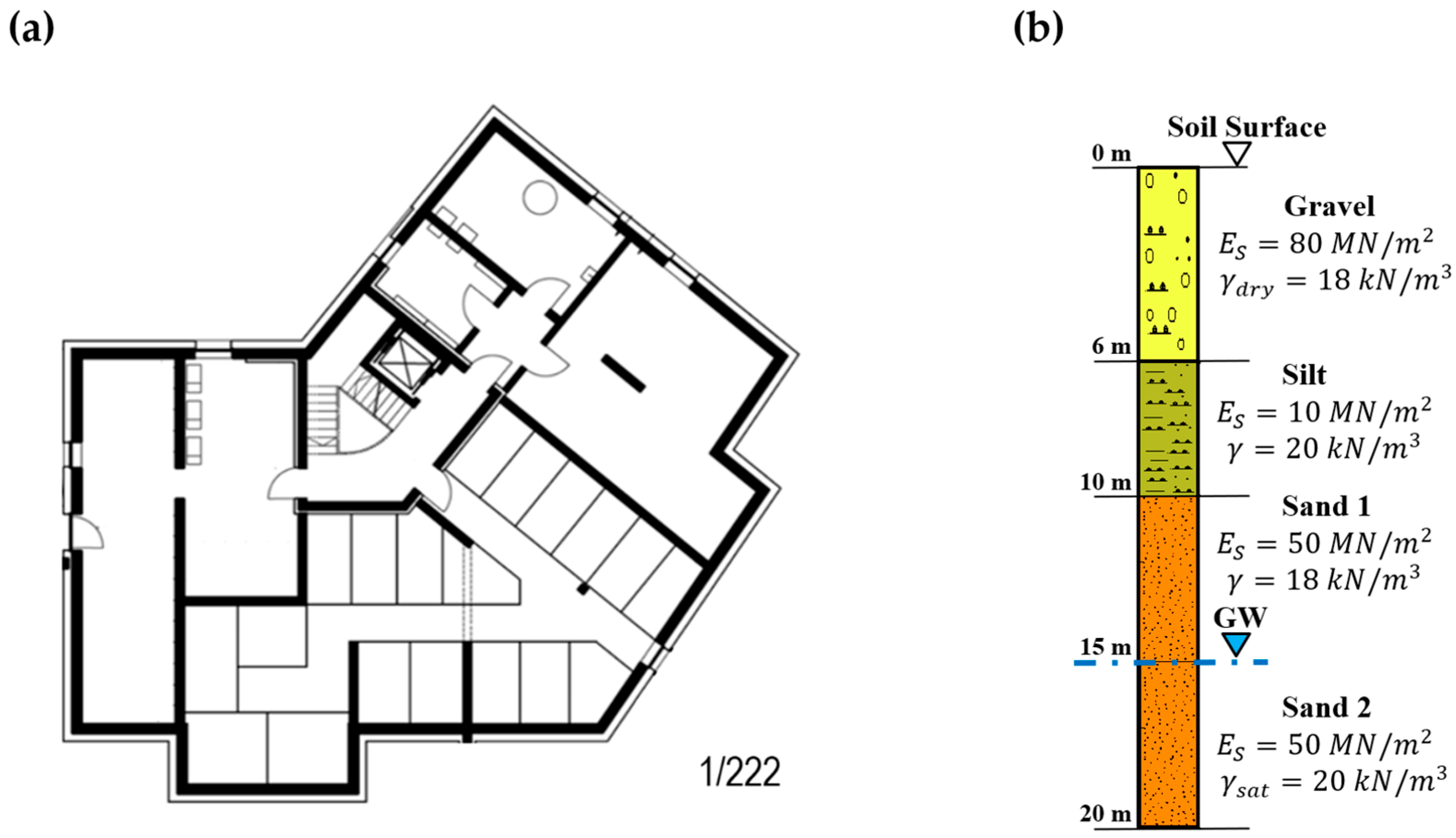
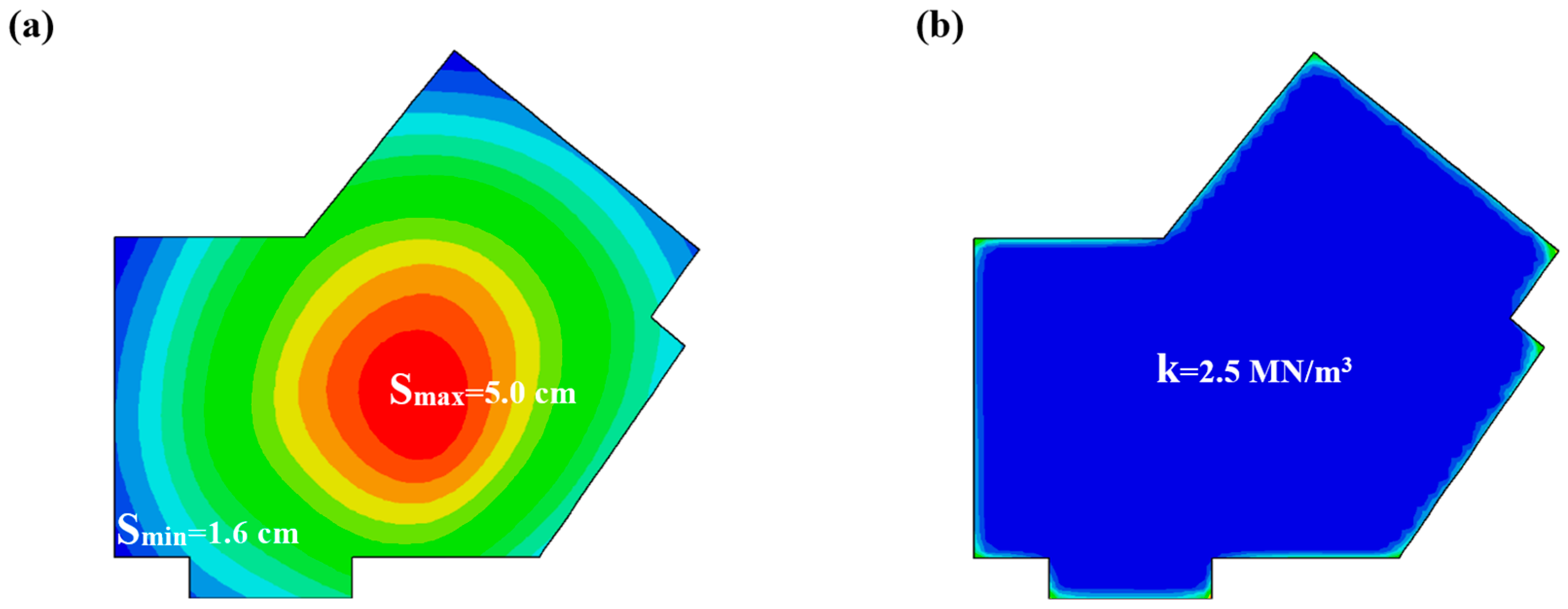
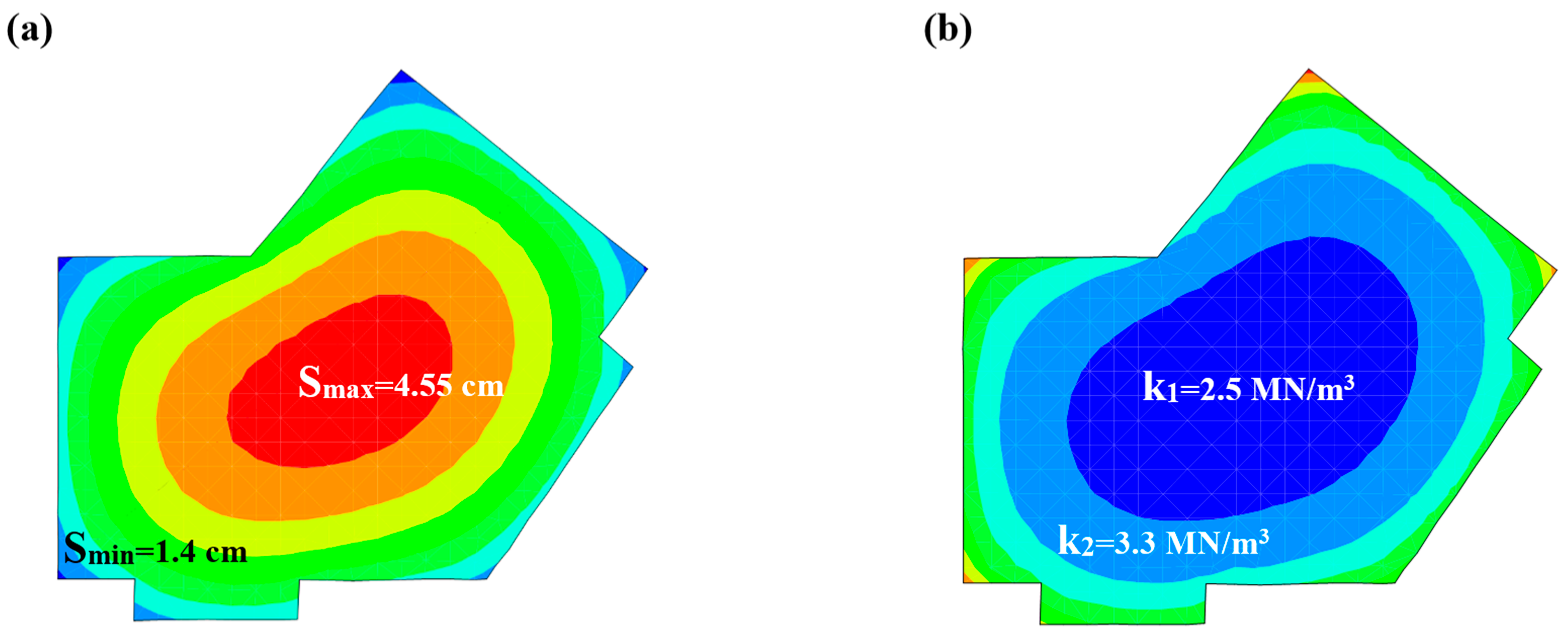
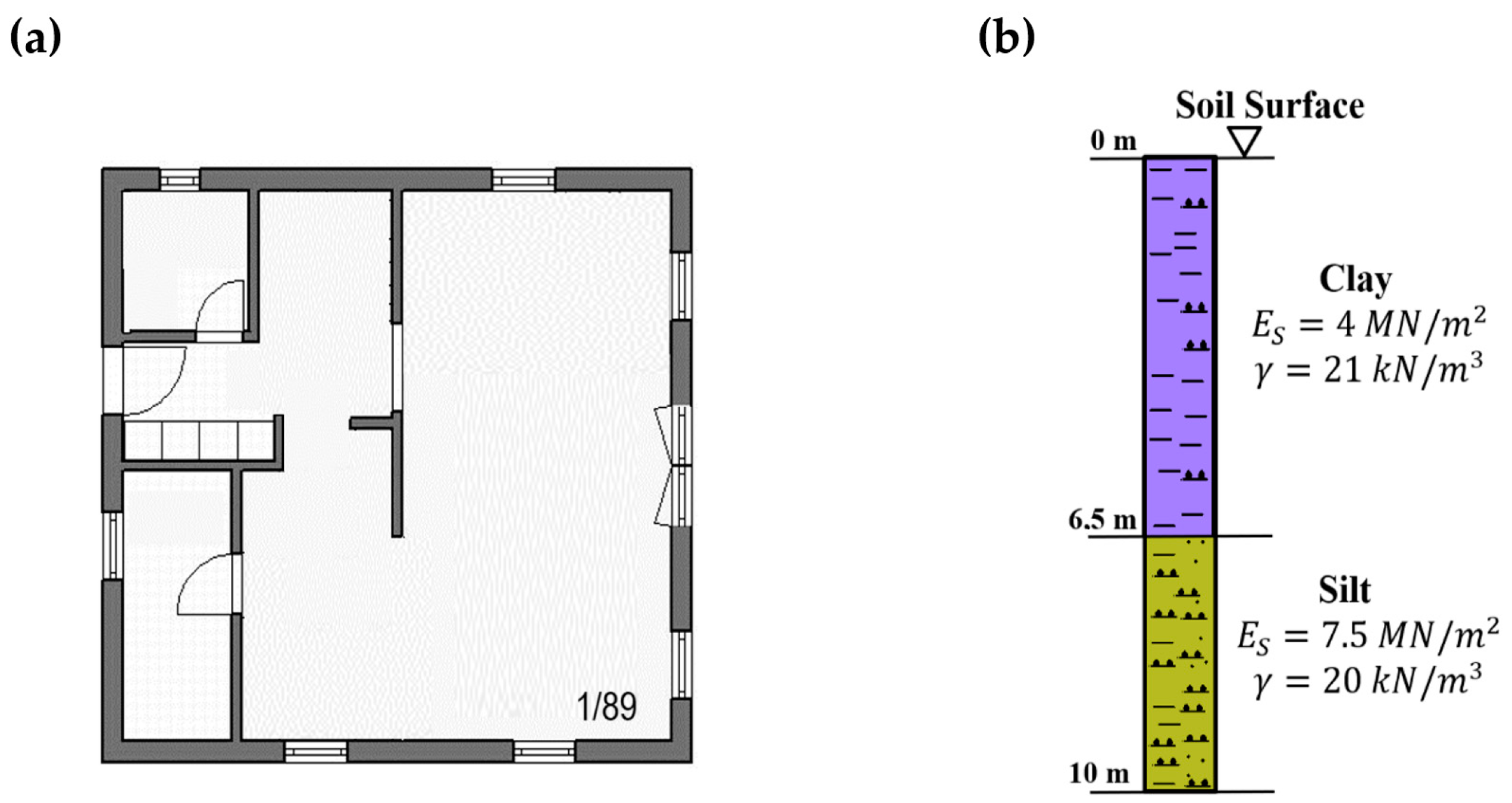
3.2.1. Case Study 1
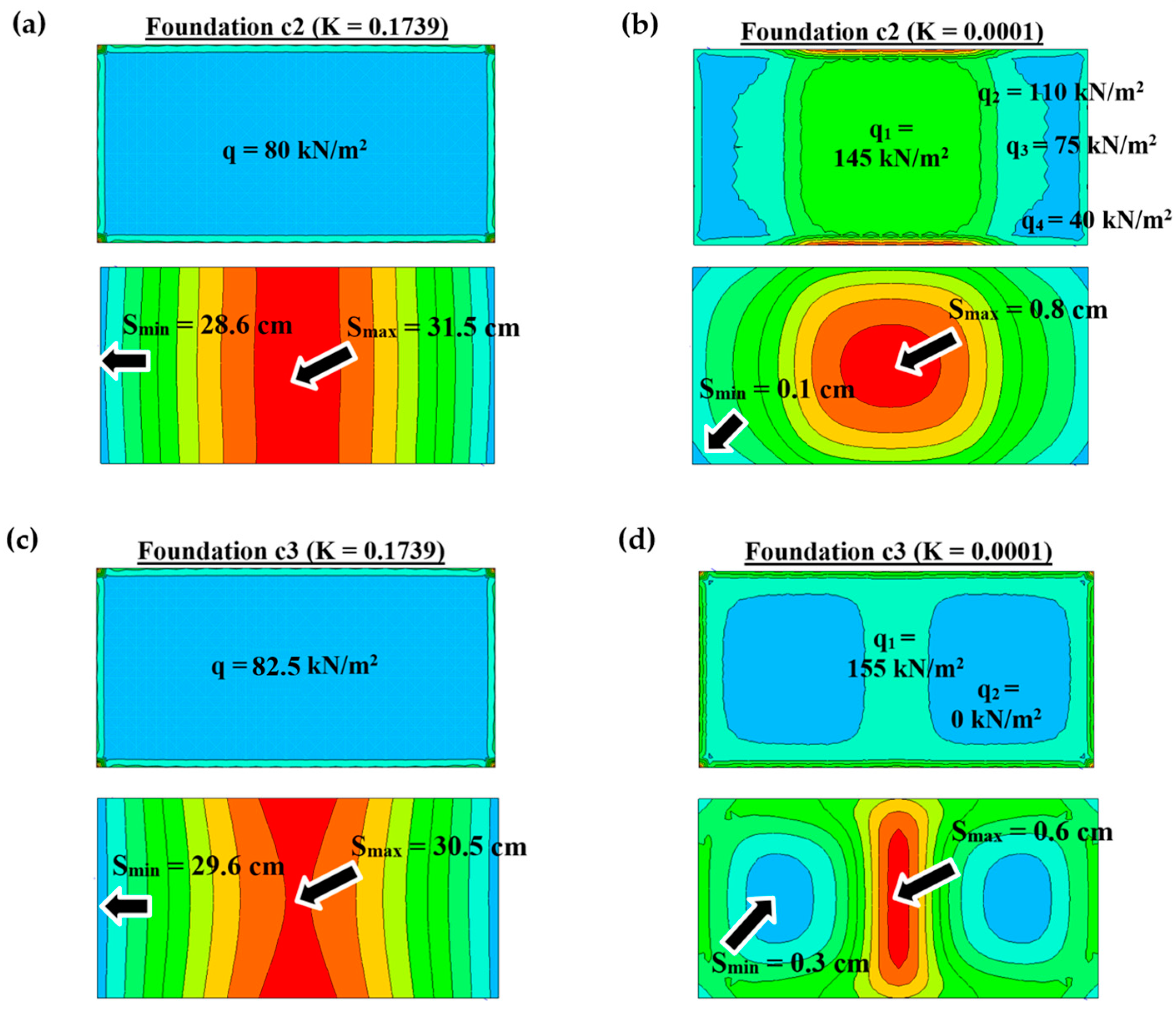
3.2.2. Case Study 2
3.2.3. Case Study 3
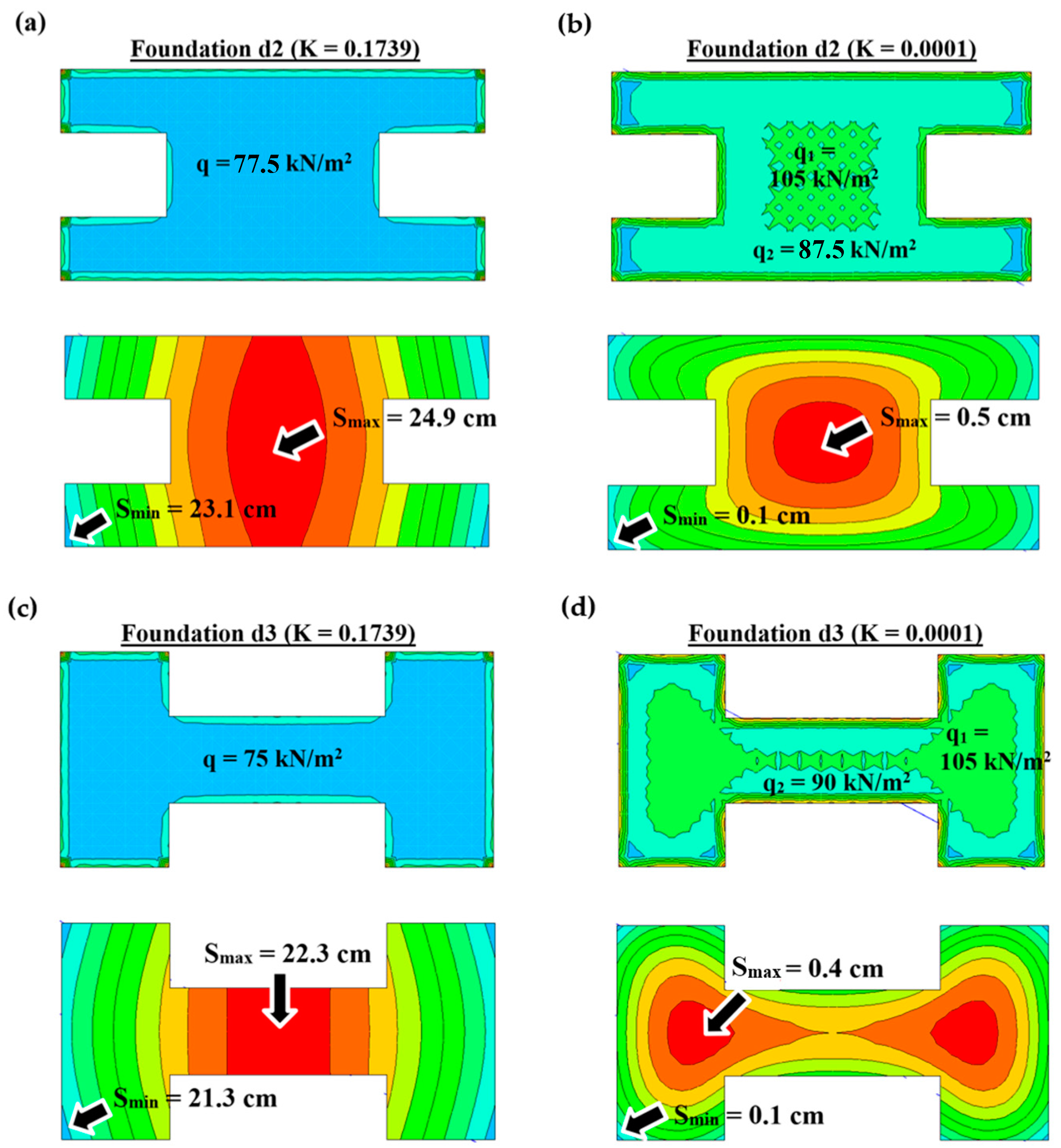
3.2.4. Case Study 4
4. Conclusions
- (1)
- Concerning the estimation of the behavior of soil–foundation systems:
- -
- Assuming the soil–foundation systems are rigid, a value of K ≥ 0.174 is sufficient for foundations with symmetrical shapes and symmetrical distributions of uniformly distributed loads. In this case, the ratio of Smax/Smin is lower than 1.1.
- -
- Assuming the soil–foundation systems are flexible, a value of K ≤ 0.001 is satisfactory only for foundations with regular shapes and a single uniform load. In this case, the ratio of qload/qcontact is lower than 1.1 and 1.15 for the square-shaped and rectangular-shaped foundations, respectively.
- (2)
- Concerning the determination of subgrade reaction modulus:
- -
- For soil–foundation systems with K ≥ 0.174, the subgrade reaction moduli obtained from the conventional analytical method are about 1.5 to 2.0 times higher than those in the FE analyses considering the soil–foundation interaction;
- -
- For soil–foundation systems with K ≤ 0.0004, both the analytical and the FE methods yield similar values of subgrade reaction moduli. The ratio of kanalytical to kfem varies between 1.05 and 1.15.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Winkler, E. Die Lehre von der Elastizität und Festigkeit; Dominicus: Prague, Czech Republic, 1867. [Google Scholar]
- Terzaghi, K. Evaluation of coefficient of subgrade reaction. Geotechnique 1955, 5, 297–326. [Google Scholar] [CrossRef]
- Daloglu, A.T.; Vallabhan, C.V.G. Values of k for slab on Winkler foundation. J. Geotech. Geoenvironmental Eng. 2000, 126, 463–471. [Google Scholar] [CrossRef]
- Alzoaby, H.; Saad, G.; Abou-Jaoude, G. Implementation of the discrete area method and its impact on the steel reinforcement of large mat foundations. Innov. Infrastruct. Solut. 2025, 10, 1–22. [Google Scholar] [CrossRef]
- Tabsh, S.W.; El-Emam, M. Influence of foundation rigidity on the structural response of mat foundation. Adv. Civ. Eng. 2021, 2021, 5586787. [Google Scholar] [CrossRef]
- Khosravifardshirazi, A.; Johari, A.; Javadi, A.A.; Khanjanpour, M.H.; Khosravifardshirazi, B.; Akrami, M. Role of Subgrade Reaction Modulus in Soil-Foundation-Structure Interaction in Concrete Buildings. Buildings 2022, 12, 540. [Google Scholar] [CrossRef]
- ACI, 336.2R-88; Suggested Analysis and Design Procedures for Combined Footings and Mats. ACI Committee: Farmington Hills, MI, USA, 2002.
- DIN-Fachbericht, 130. In Wechselwirkung Baugrund/Bauwerk Flachgründungen; Deutsches Institut für Normung e.V., Beuth-Verlag: Berlin, Deutschland, 2003.
- Bergmeister, K.; Wörner, J.-H. Beton-Kalender 2006: Schwerpunkt: Turmbauwerke, Industriebauten; Ernst&Sohn: Berlin, Germany, 2006. [Google Scholar]
- Brown, P.T. Numerical Analyses of Uniformly Loaded Circular Rafts on Deep Elastic Foundations. Geotechnique 1969, 19, 399–404. [Google Scholar] [CrossRef]
- El Gendy, M. An analysis for determination of foundation rigidity. In Proceedings of the 8th International Colloquium on Structural and Geotechnical Engineering, Cairo, Egypt, 15–17 December 1998. [Google Scholar]
- Lang, H.J.; Huder, J.; Amann, P.; Puzrin, A.M. Bodenmechanik und Grundbau (in German); Springer Verlag: Berlin, Germany, 2010. [Google Scholar]
- Biot, M.A. General theory of three-dimensional consolidation. J. Appl. Phys. 1941, 12, 155–164. [Google Scholar] [CrossRef]
- Vesic, A.B. Beams on elastic subgrade and the Winkler’s hypothesis. In Proceedings of the 5th International Conference on Soil Mechanics and Foundation Engineering, Paris, France, 17–22 July 1961. [Google Scholar]
- Bowles, J.E. Foundation Analysis and Design, 5th ed.; McGraw-Hill: New York, NY, USA, 1996. [Google Scholar]
- Poulos, H. Rational assessment of modulus of subgrade reaction. Geotech. Eng. J. SEAGS AGSSEA 2018, 49, 1–7. [Google Scholar] [CrossRef]
- Lee, J.; Jeong, S.; Lee, J.K. 3D analytical method for mat foundations considering coupled soil springs. Geomech. Eng. 2015, 8, 845–857. [Google Scholar] [CrossRef]
- Jeong, S.; Park, J.; Hong, M.; Lee, J. Variability of subgrade reaction modulus on flexible mat foundation. Geomech. Eng. 2017, 13, 757–774. [Google Scholar] [CrossRef]
- Loukidis, D.; Tamiolakis, G.P. Spatial distribution of Winkler spring stiffness for rectangular mat foundation analysis. Eng. Struct. 2017, 153, 443–459. [Google Scholar] [CrossRef]
- Son, M.; Jung, H.S.; Yoon, H.H.; Sung, D.; Kim, J.S. Numerical Study on Scale Effect of Repetitive Plate-Loading Test. Appl. Sci. 2019, 9, 4442. [Google Scholar] [CrossRef]
- Roy, S.S.; Deb, K. Modulus of subgrade reaction of unreinforced and georid-reinforced granular fill over soft clay. Int. J. Geomech. 2021, 21, 04021156. [Google Scholar] [CrossRef]
- Hamza, O.; Kourdey, A.; Hussain, Y.; Mawas, A. Subgrade reaction for closely spaced raft and isolated foundations on sand: Case study. Proc. Inst. Civ. Eng.-Geotech. Eng. 2024, 177, 135–146. [Google Scholar] [CrossRef]
- Rahgooy, K.; Bahmanpour, A.; Derakhshandi, M.; Bagherzadeh-Khalkhali, A. Distribution of elastoplastic modulus of subgrade reaction for analysis of raft foundations. Geomech. Eng. 2022, 28, 89–105. [Google Scholar] [CrossRef]
- Dehghanbanadaki, A.; Rashid, A.S.F.; Ahmad, K.; Yunus, N.Z.M.; Said, K.N.M. A computational estimation model for the subgrade reaction modulus of soil improved with DCM columns. Geomech. Eng. 2022, 28, 385–396. [Google Scholar] [CrossRef]
- Chang, D.-W.; Lu, C.-W.; Tu, Y.-J.; Cheng, S.-H. Settlements and Subgrade Reactions of Surface Raft Foundations Subjected to Vertically Uniform Load. Appl. Sci. 2022, 12, 5484. [Google Scholar] [CrossRef]
- Li, W.; Tao, Q.; Gu, R.; Li, C.; Dai, G.; Gong, W. Plate Size Effects in Gravelly Soil Based on In Situ Plate Load Tests and Finite Element Analysis. Appl. Sci. 2025, 15, 760. [Google Scholar] [CrossRef]
- Buß, J. GGU-Slab User Manual, version 12.04; Civilserve GmbH: Steinfeld, Germany, 2023. [Google Scholar]
- Boussinesq, J. Application des Potentiels à L’étude de L’équilibre et du Mouvement des Solides Élastiques; Gauthier-Villars: Paris, France, 1883. [Google Scholar]
- Buß, J. GGU-Settle User Manual, version 7.00; Civilserve GmbH: Steinfeld, Germany, 2023. [Google Scholar]
- DIN 4019:2015-05; Baugrund-Setzungsberechnungen. Deutsches Institut für Normung e.V., Beuth-Verlag: Berlin, Deutschland, 2015.


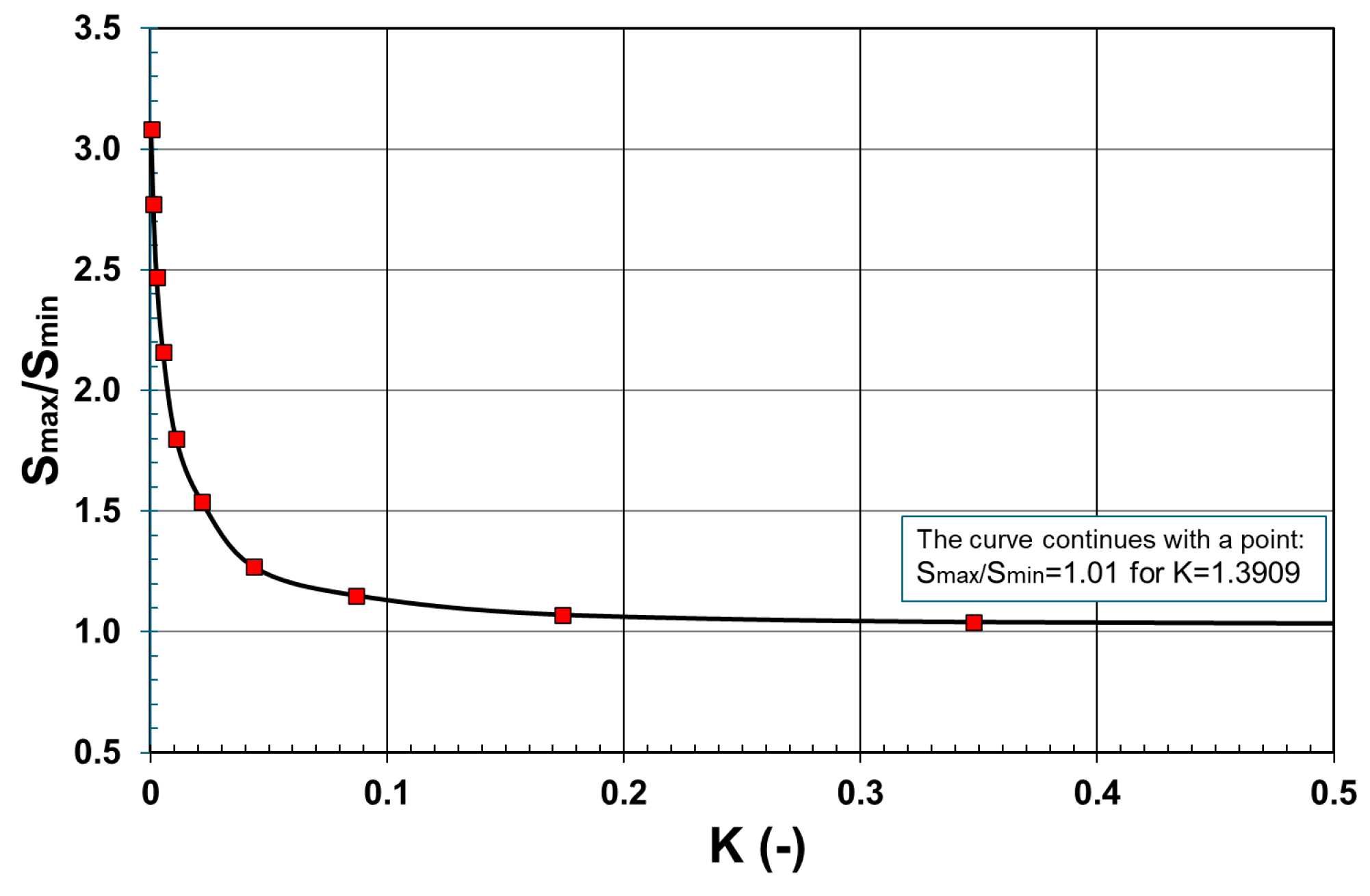
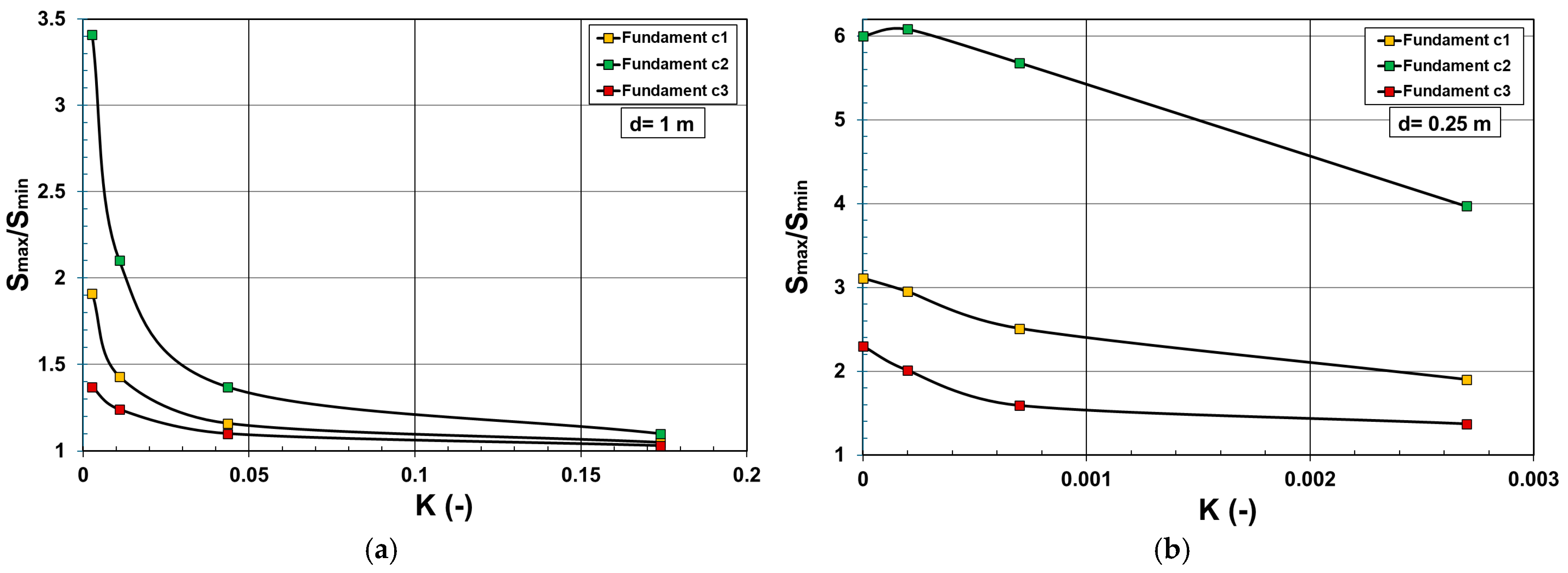
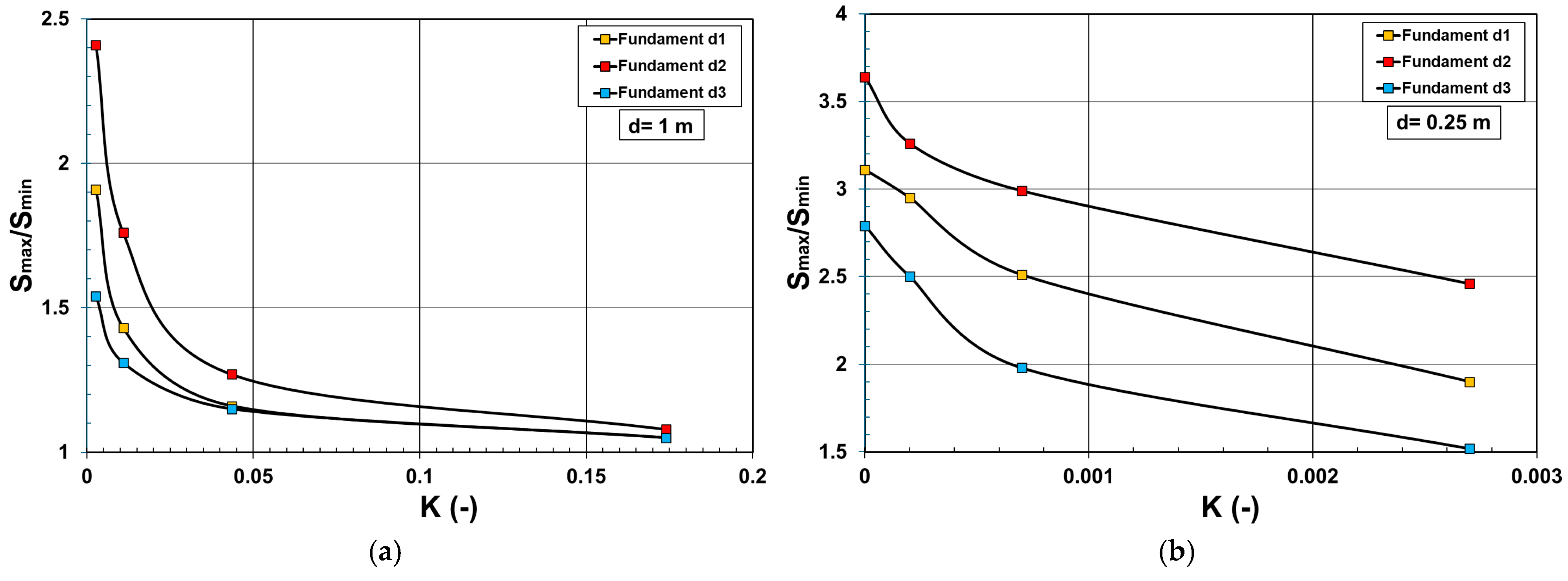




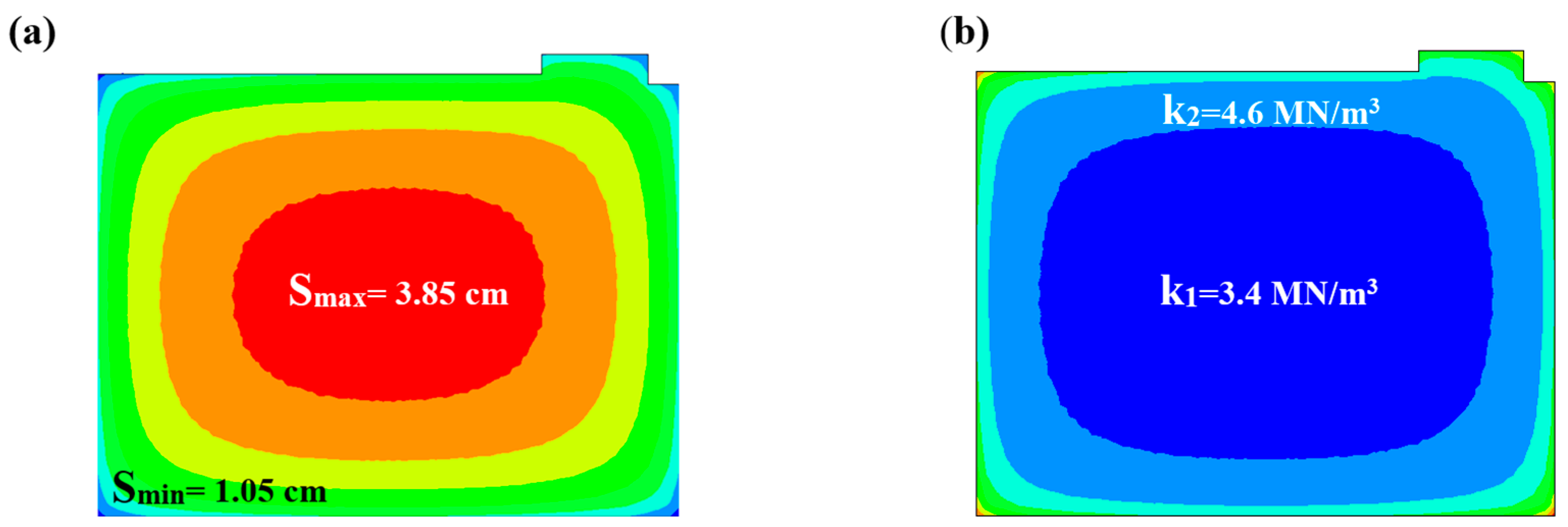


| Stiffness Modulus of Soil Es (MPa) | Relative Stiffness of the Soil–Foundation System K (-) | ||
|---|---|---|---|
| Square Foundation (5 m × 5 m × 0.5 m) | Square Foundation (10 m × 10 m × 0.5 m) | Square Foundation (20 m × 20 m × 0.5 m) | |
| 2.5 | 1.3909 a | 0.1739 a | 0.0217 b |
| 10 | 0.3477 a | 0.0435 b | 0.0054 c |
| 40 | 0.0870 b | 0.0109 c | 0.0013 c |
| 160 | 0.0217 b | 0.0027 c | 0.0004 d |
| Stiffness Modulus of Soil Es (MPa) | Relative Stiffness of the Soil–Foundation System K (-) | ||
|---|---|---|---|
| Square Foundation (10 m × 10 m × 0.25 m) | Square Foundation (10 m × 10 m × 0.5 m) | Square Foundation (10 m × 10 m × 1 m) | |
| 2.5 | 0.0217 b | 0.1739 a | 1.3909 a |
| 10 | 0.0054 c | 0.0435 b | 0.3477 a |
| 40 | 0.0013 c | 0.0109 c | 0.0870 b |
| 160 | 0.0004 d | 0.0027 c | 0.0217 b |
| Stiffness Modulus of Soil Es (MPa) | Relative Stiffness of the Soil–Foundation System K (-) Foundations in Figure 3c,d | |
|---|---|---|
| d = 1.0 m | d = 0.25 m | |
| 2.5 | 0.1739 a | 0.0027 c |
| 10 | 0.0435 b | 0.0007 d |
| 40 | 0.0109 c | 0.0002 d |
| 160 | 0.0027 c | 0.0001 d |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Koltuk, S.; Topçu, S. Determination of Subgrade Reaction Modulus Considering the Relative Stiffnesses of Soil–Foundation Systems. Appl. Sci. 2025, 15, 4714. https://doi.org/10.3390/app15094714
Koltuk S, Topçu S. Determination of Subgrade Reaction Modulus Considering the Relative Stiffnesses of Soil–Foundation Systems. Applied Sciences. 2025; 15(9):4714. https://doi.org/10.3390/app15094714
Chicago/Turabian StyleKoltuk, Serdar, and Sadettin Topçu. 2025. "Determination of Subgrade Reaction Modulus Considering the Relative Stiffnesses of Soil–Foundation Systems" Applied Sciences 15, no. 9: 4714. https://doi.org/10.3390/app15094714
APA StyleKoltuk, S., & Topçu, S. (2025). Determination of Subgrade Reaction Modulus Considering the Relative Stiffnesses of Soil–Foundation Systems. Applied Sciences, 15(9), 4714. https://doi.org/10.3390/app15094714






