Kink, Dark, Bright, and Singular Optical Solitons to the Space–Time Nonlinear Fractional (41)-Dimensional Davey–Stewartson–Kadomtsev–Petviashvili Model+
Abstract
:1. Introduction
2. Techniques
2.1. Unified Technique
- (i)
- if , thenififwhere and q and d are any real constants.
2.2. The METhEF Technique
- (i)
- if , thenor
- (ii)
- if , then
- (iii)
- if , thenor
3. New Exact Solitons
3.1. Unified Technique
3.2. By METhEF Technique
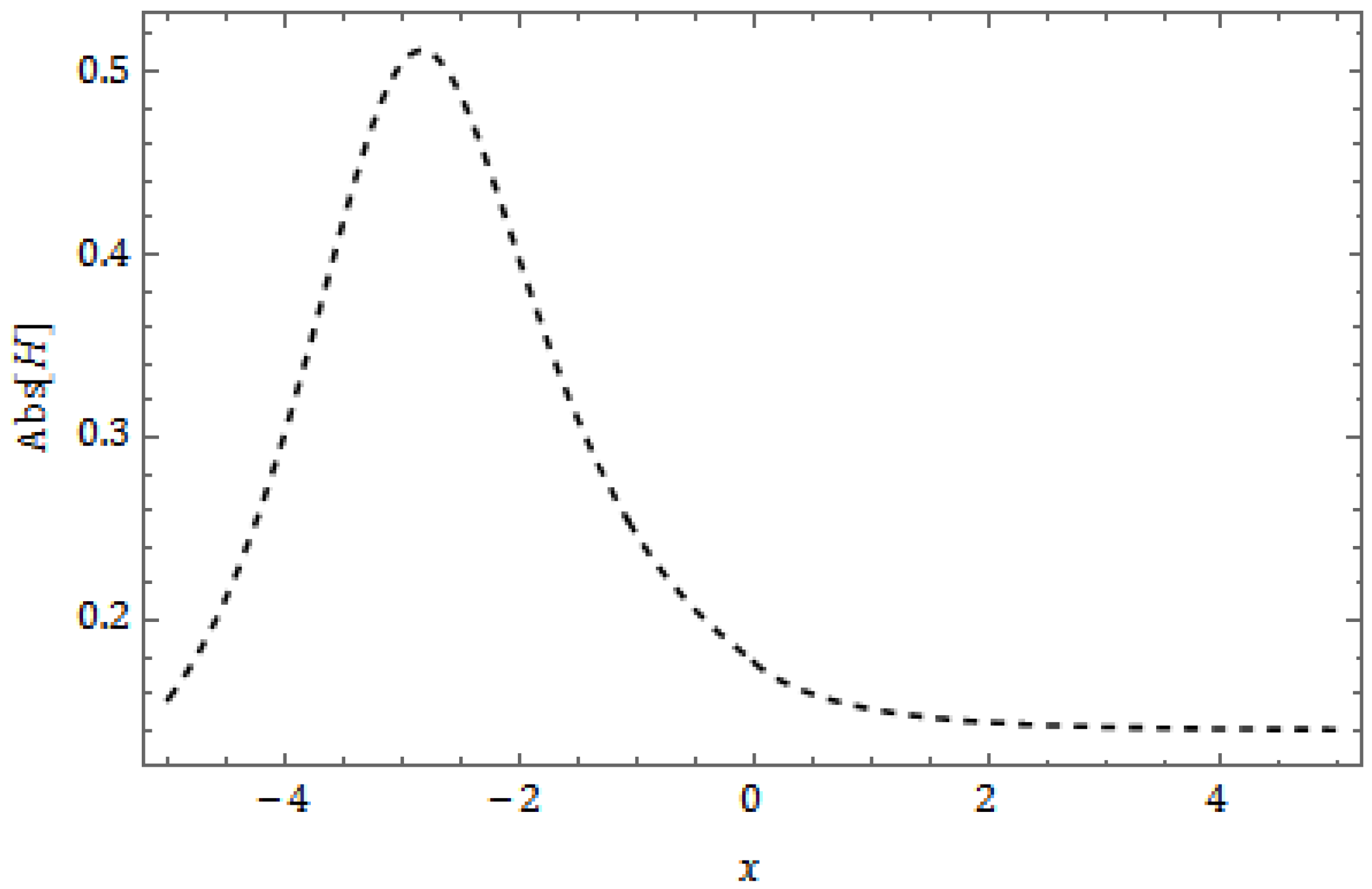
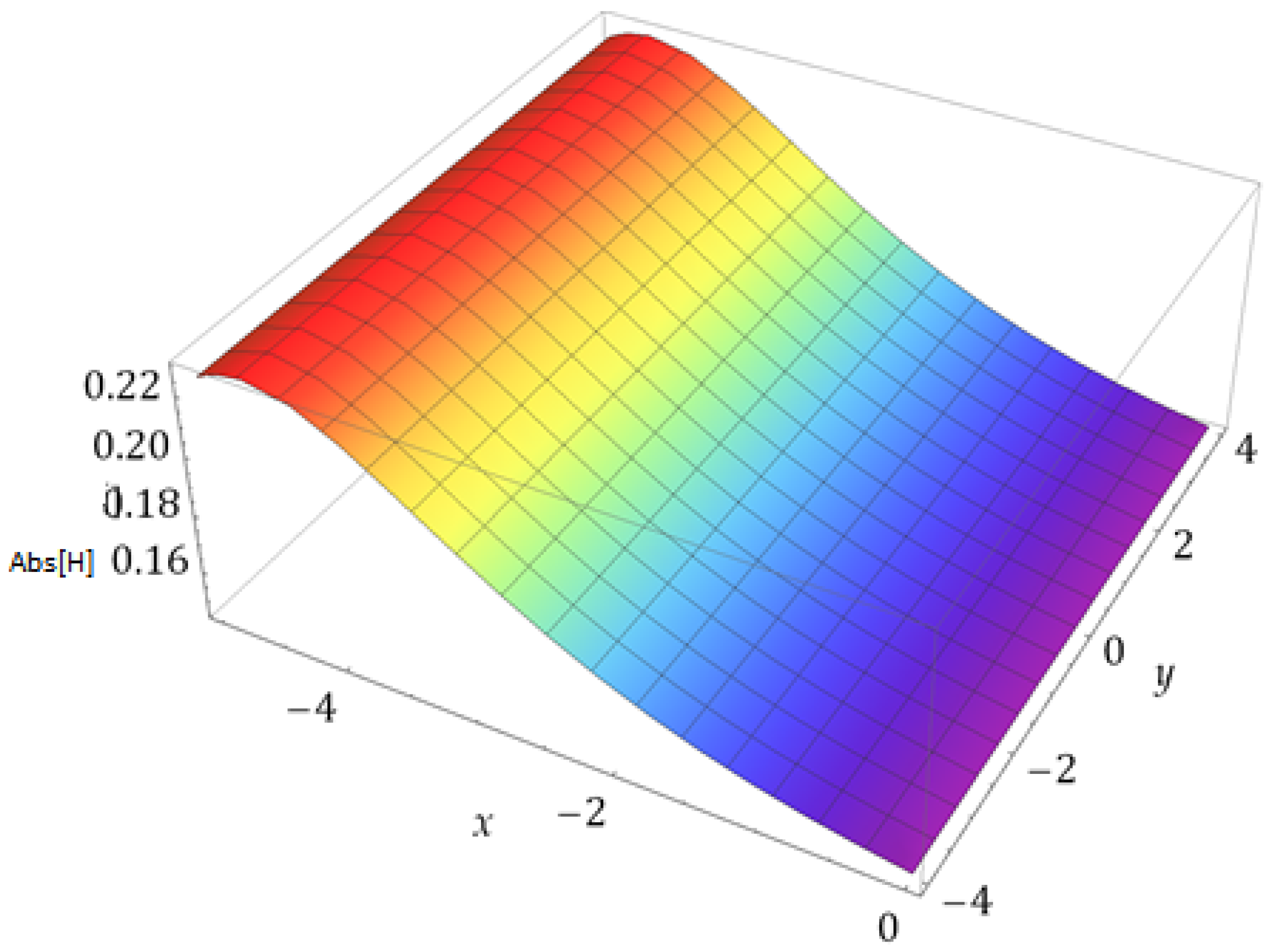
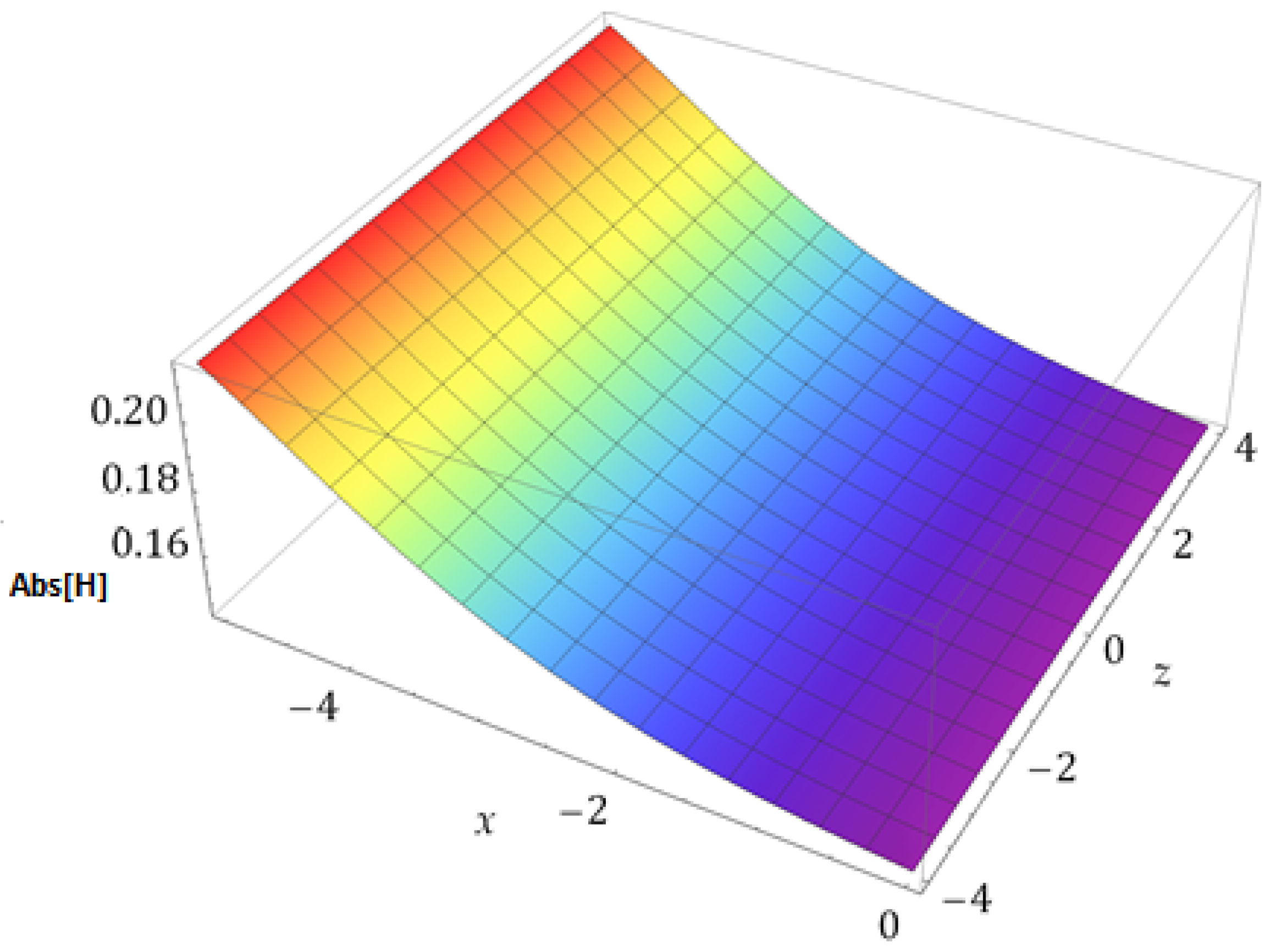
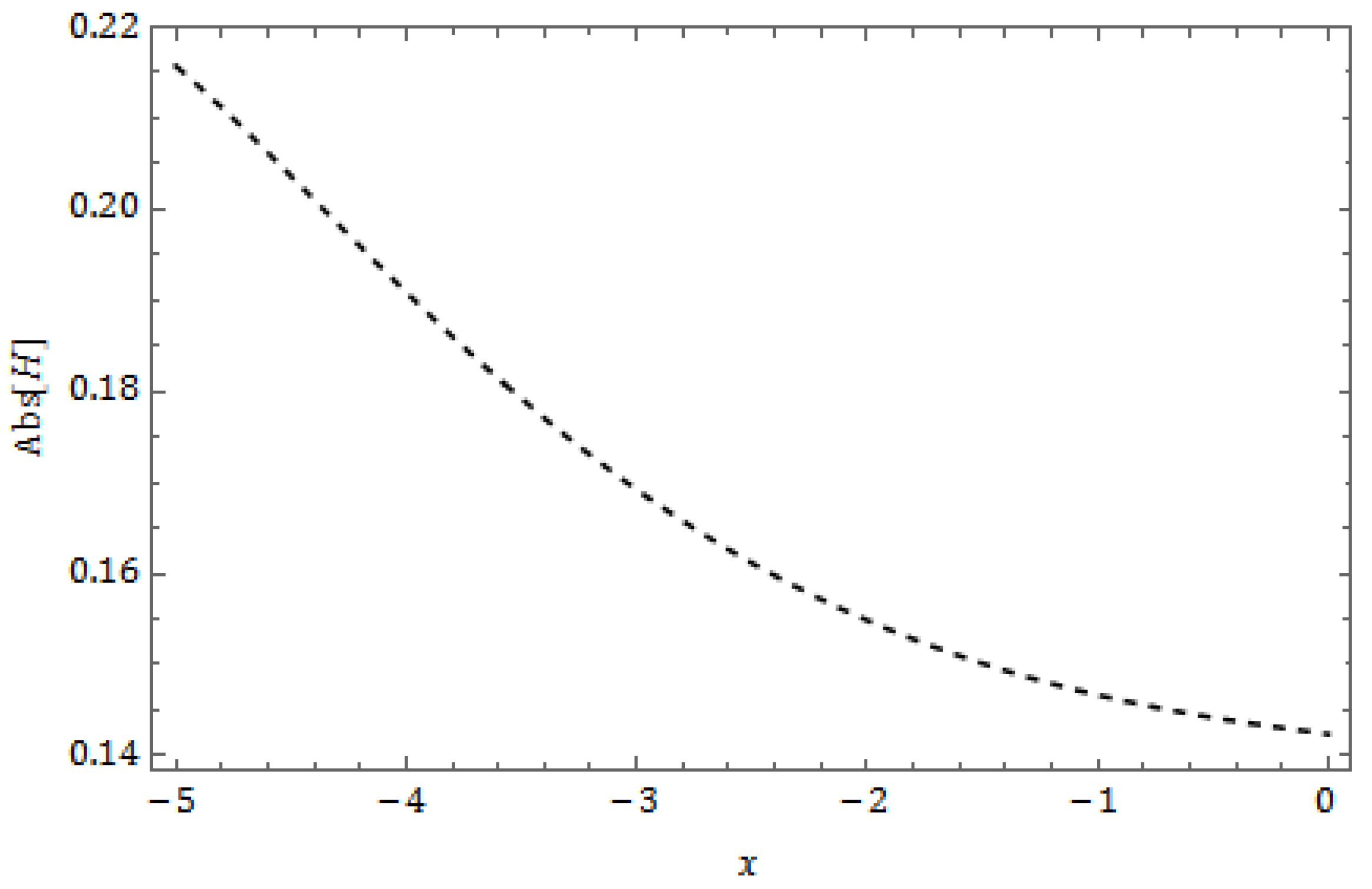
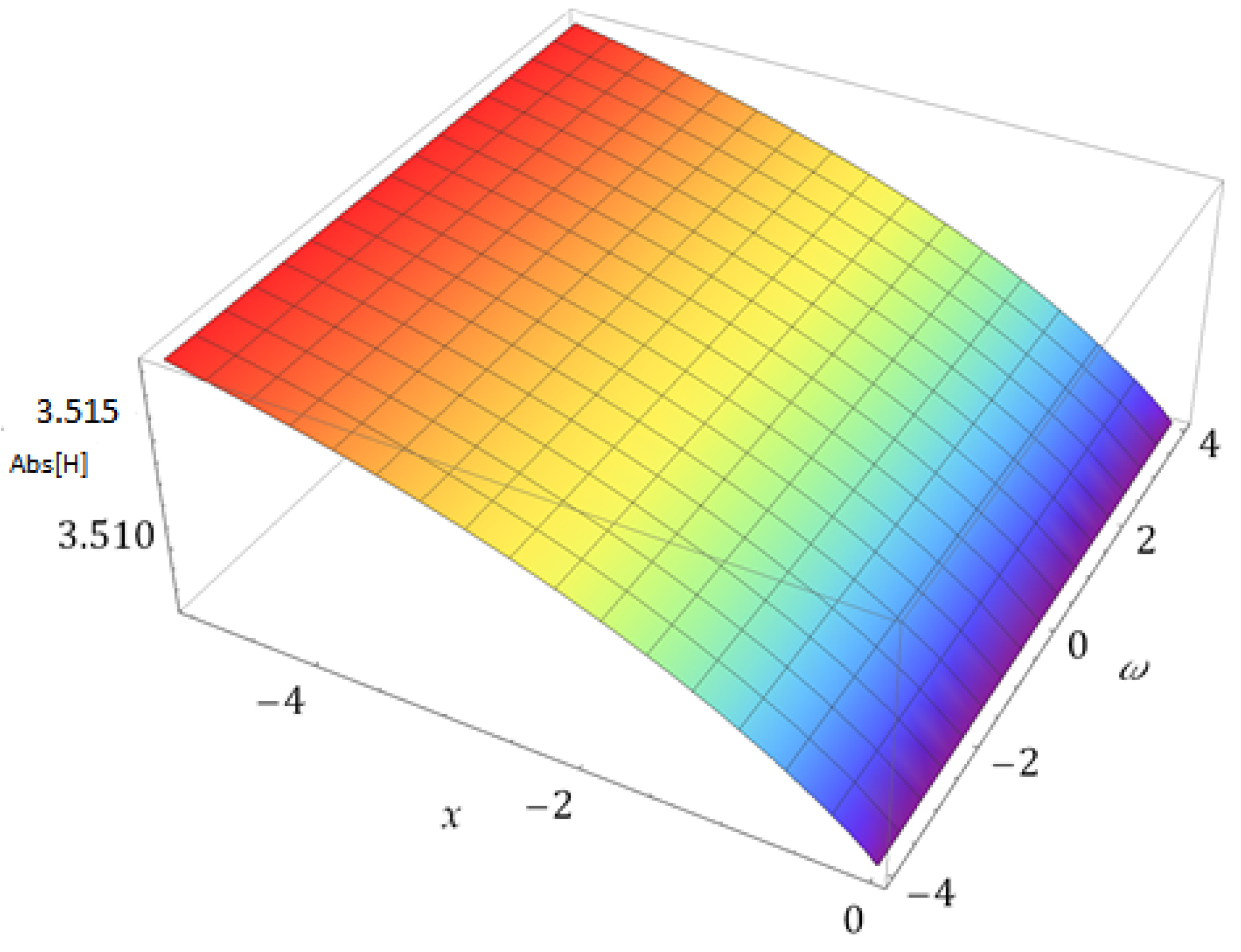
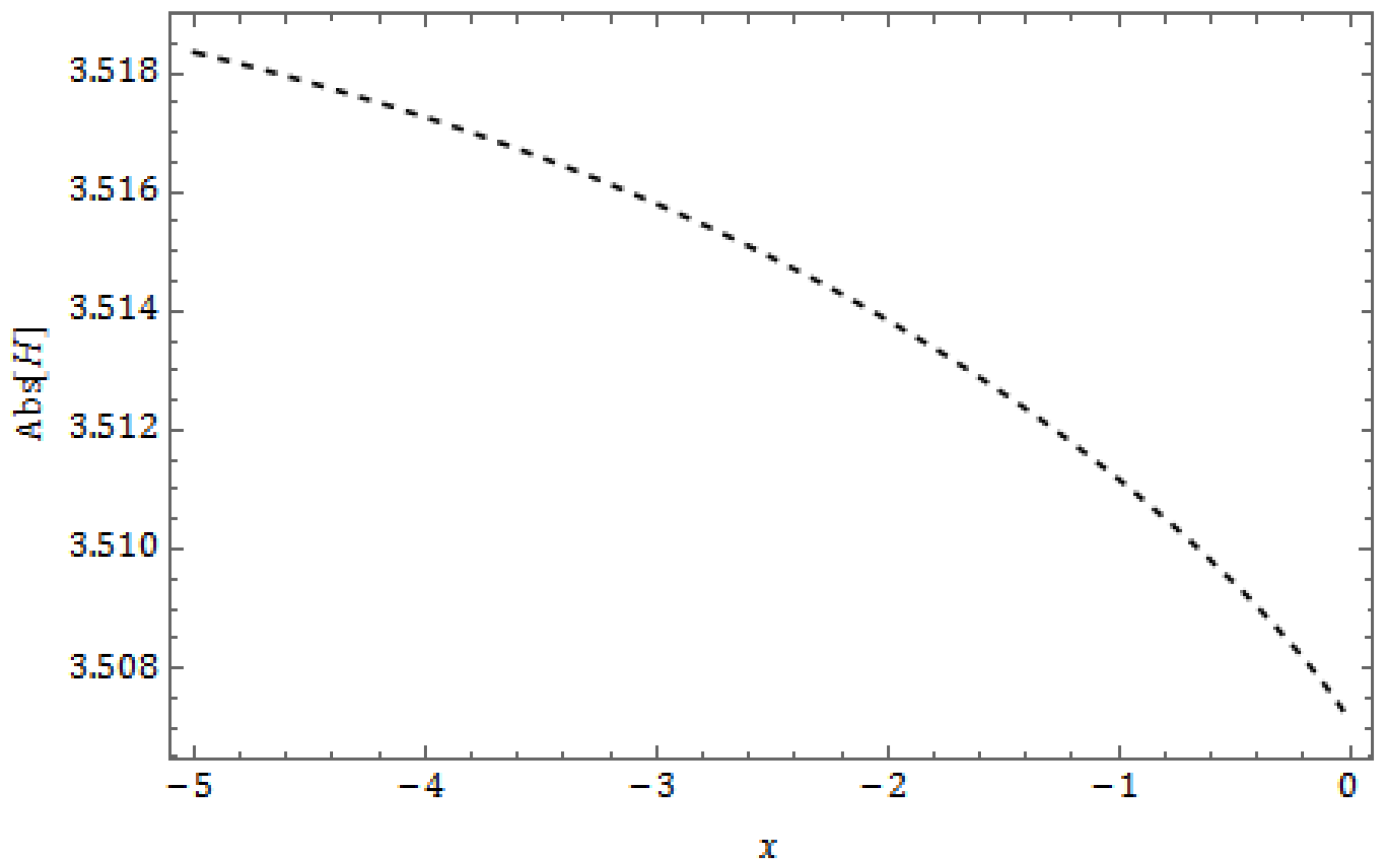
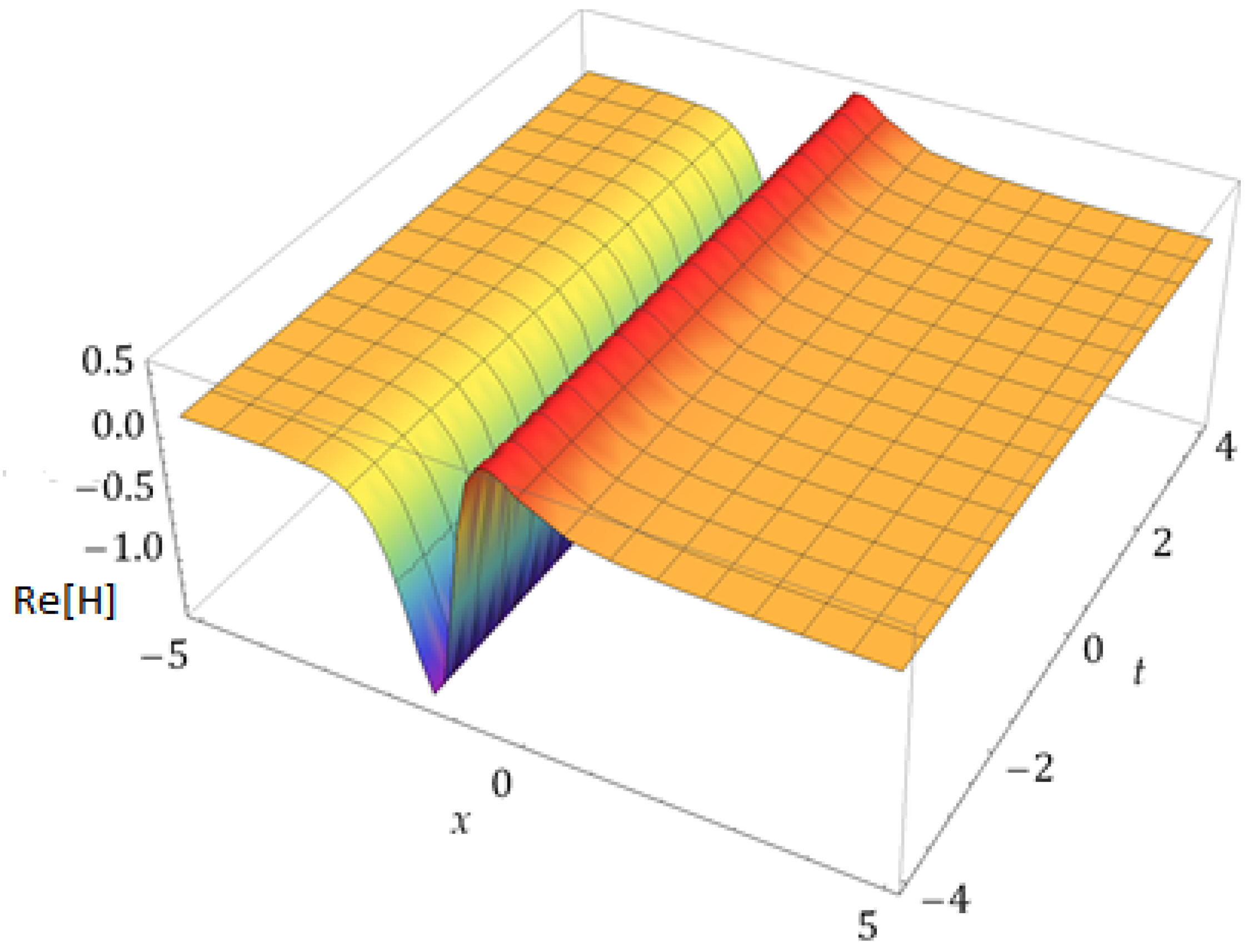
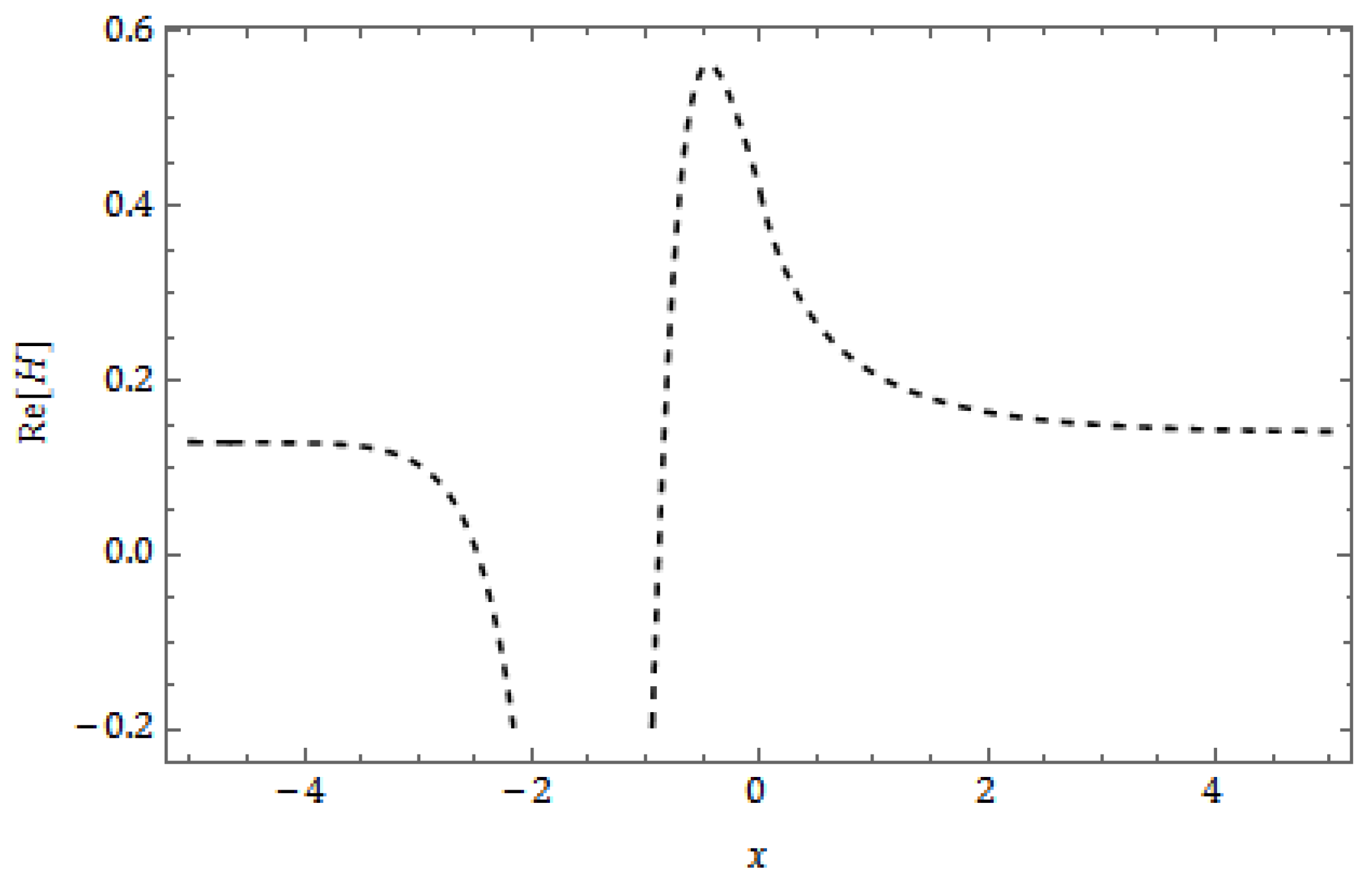
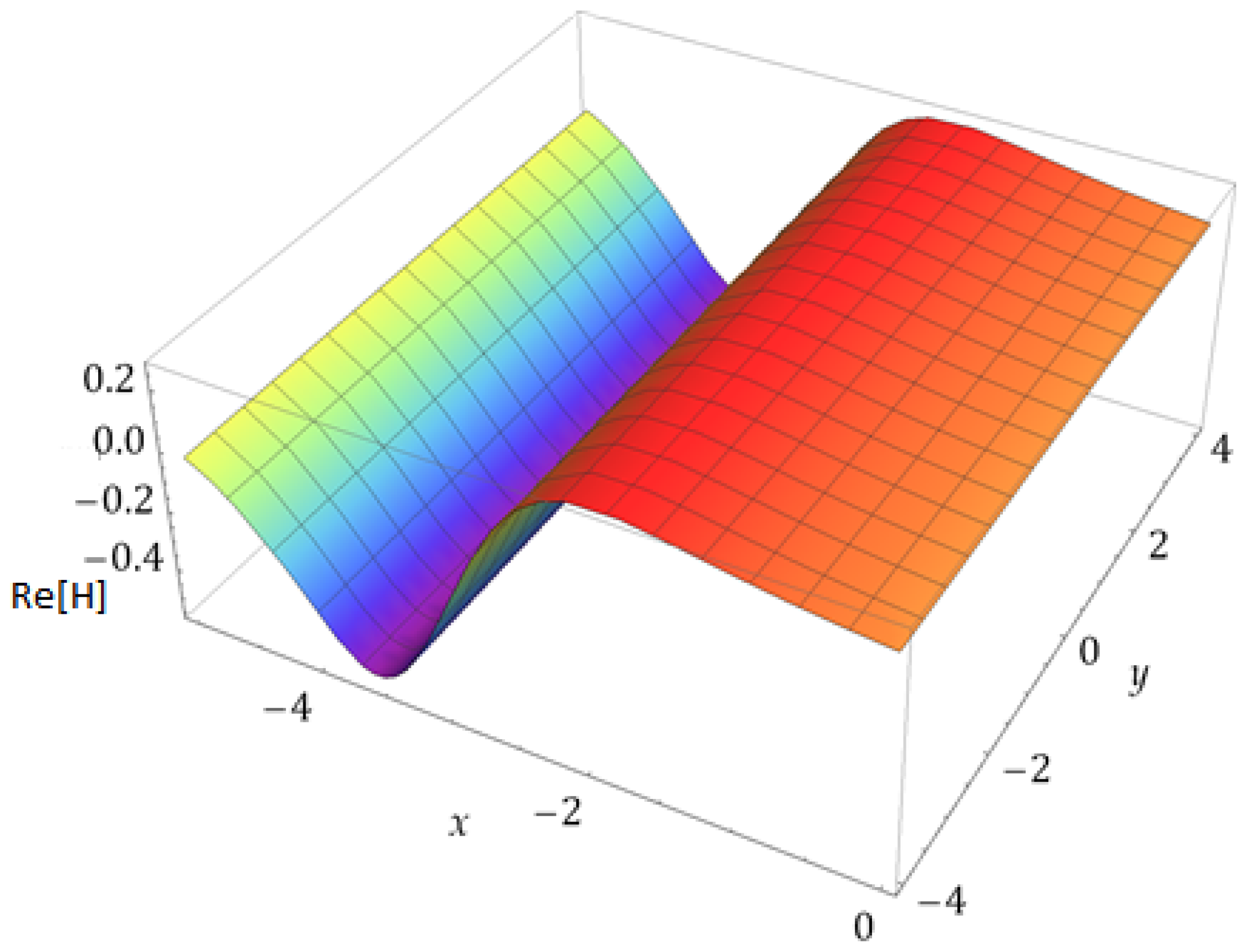
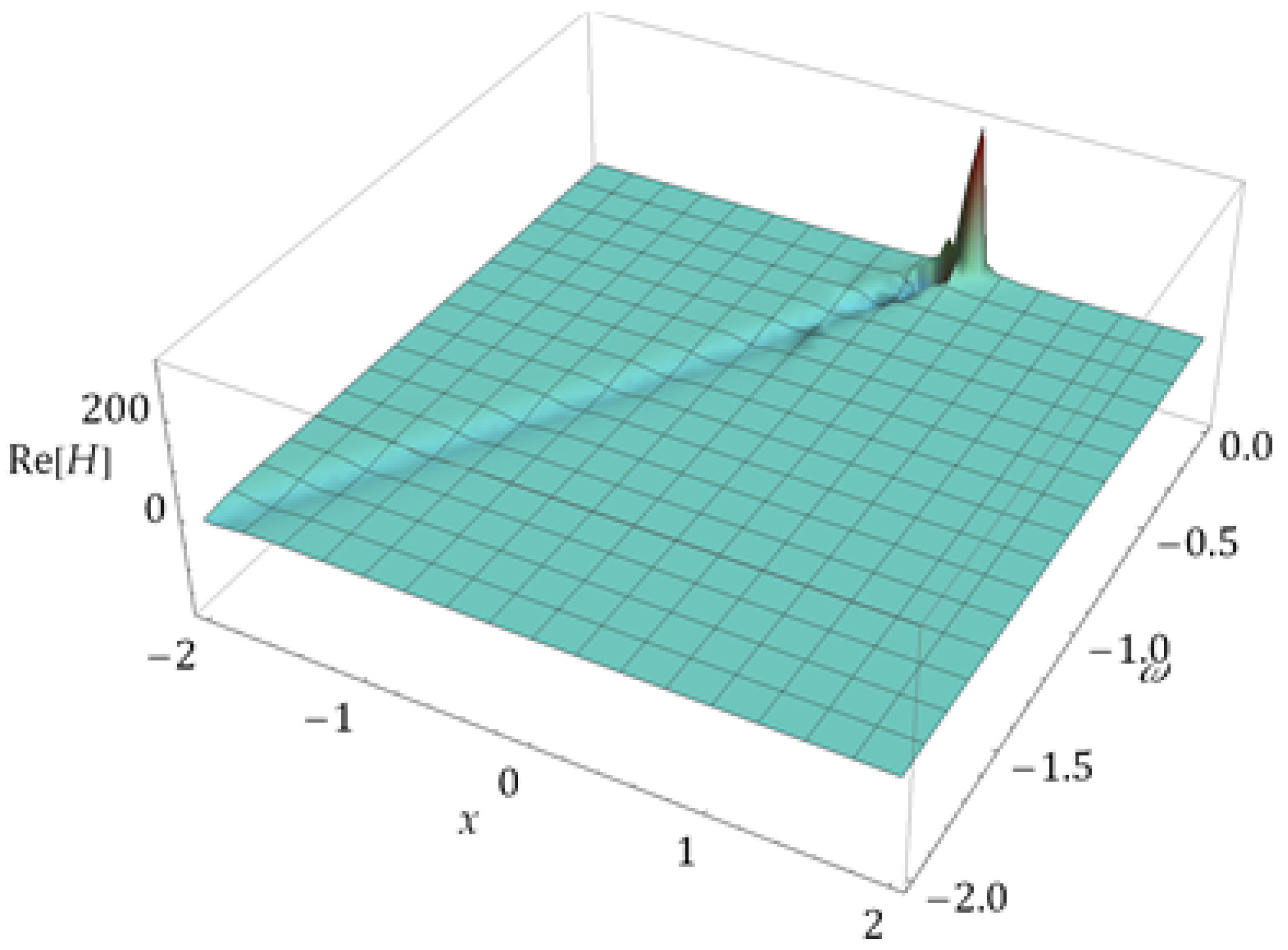
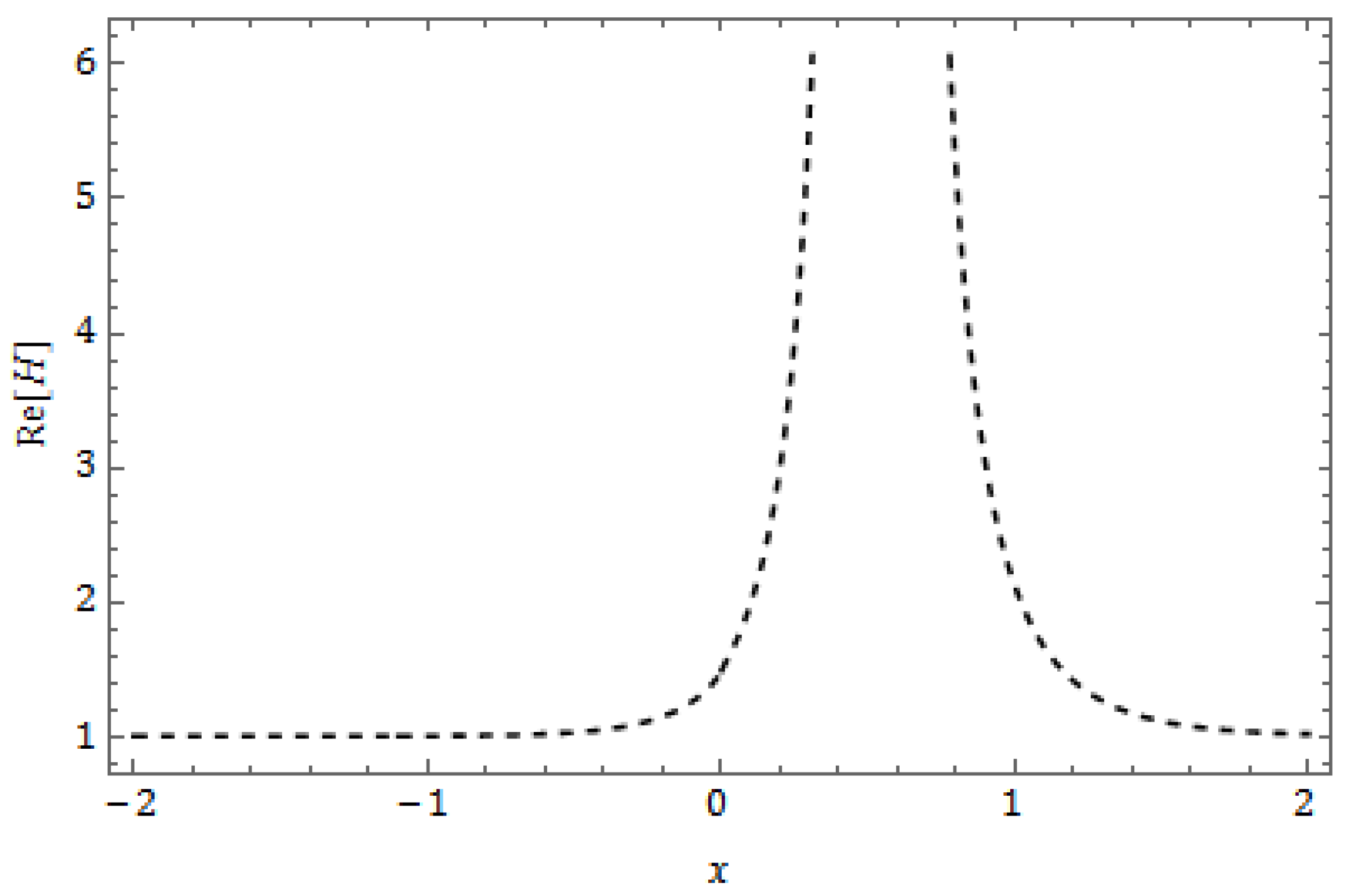
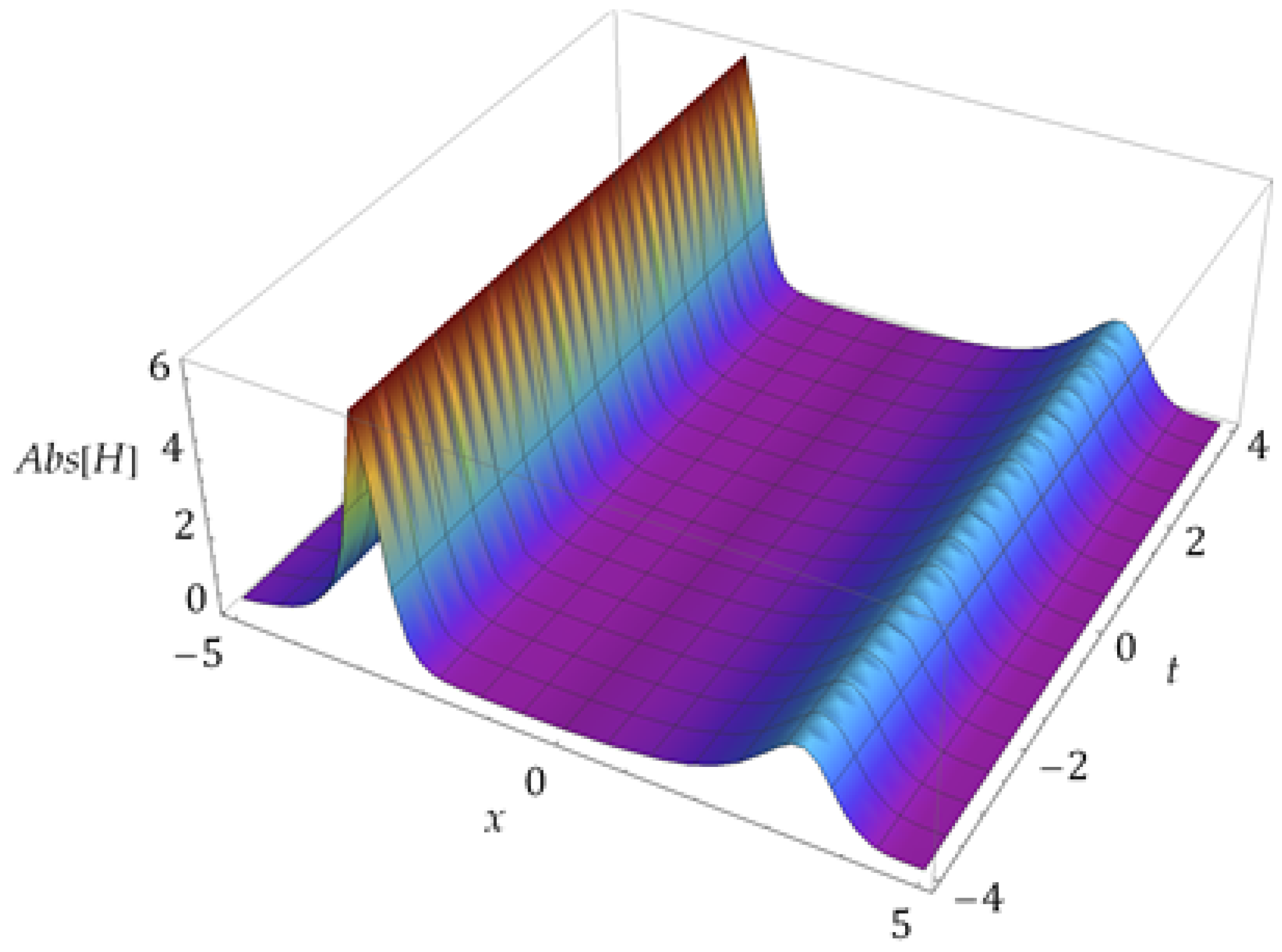
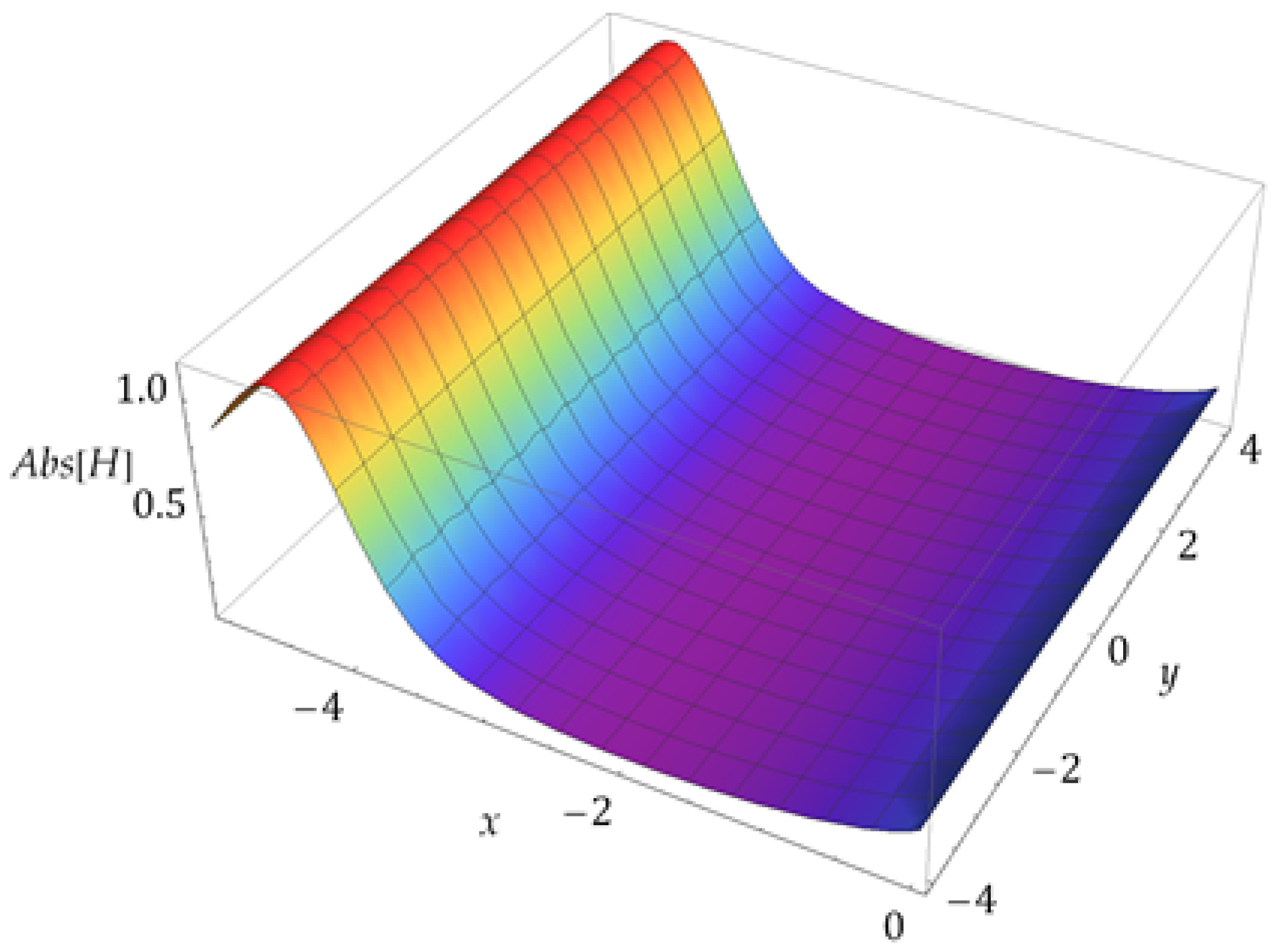
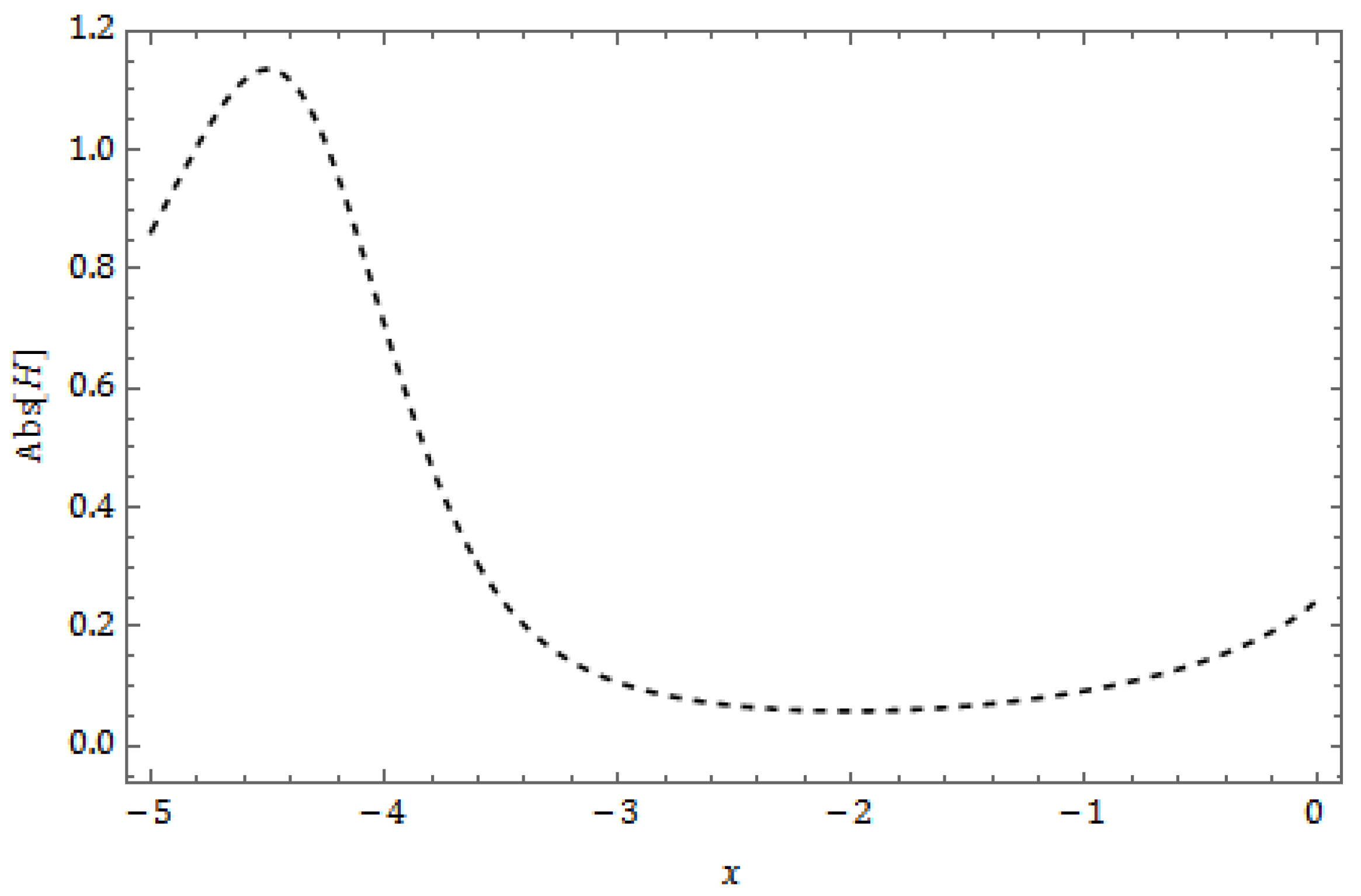
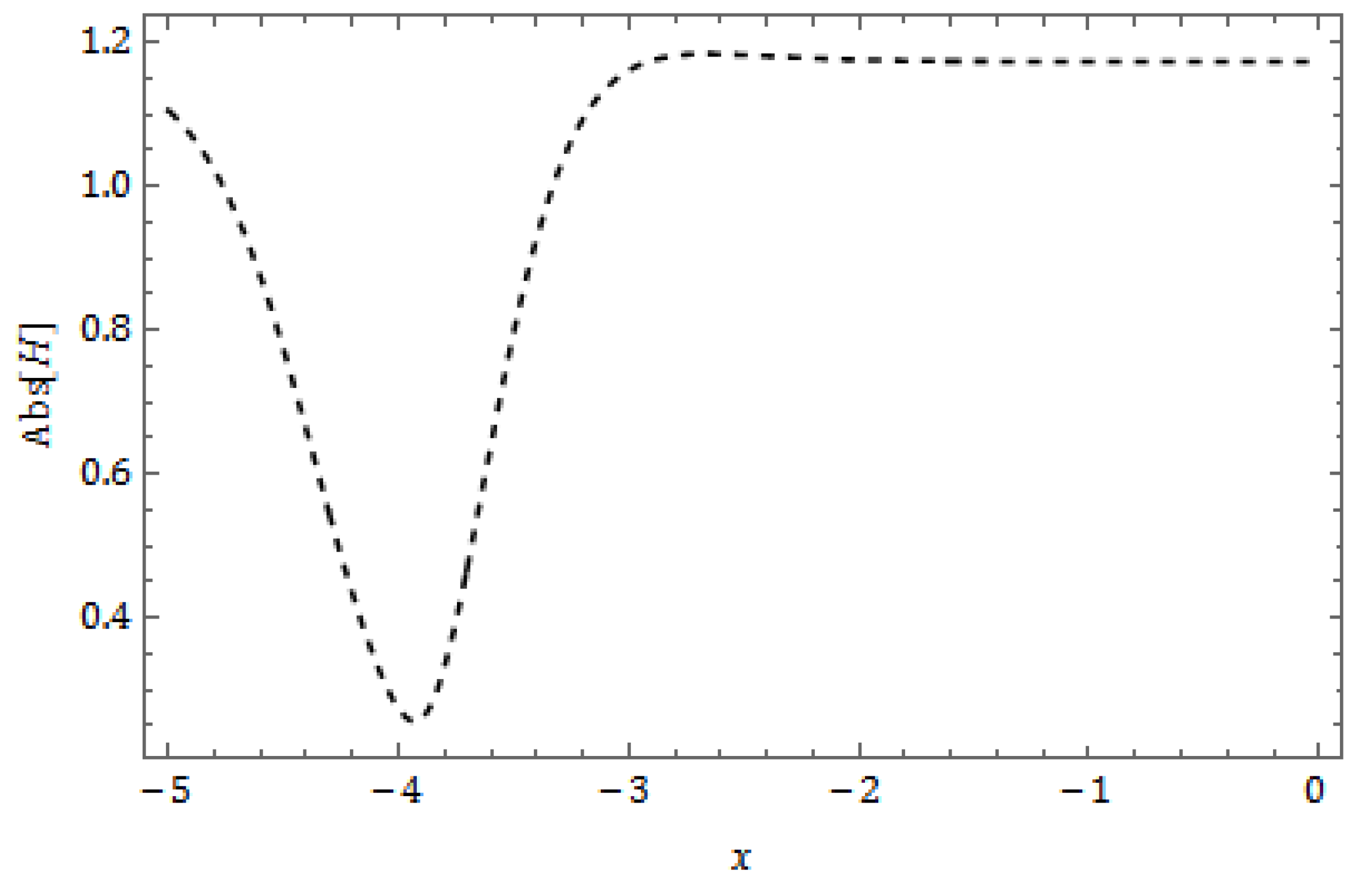
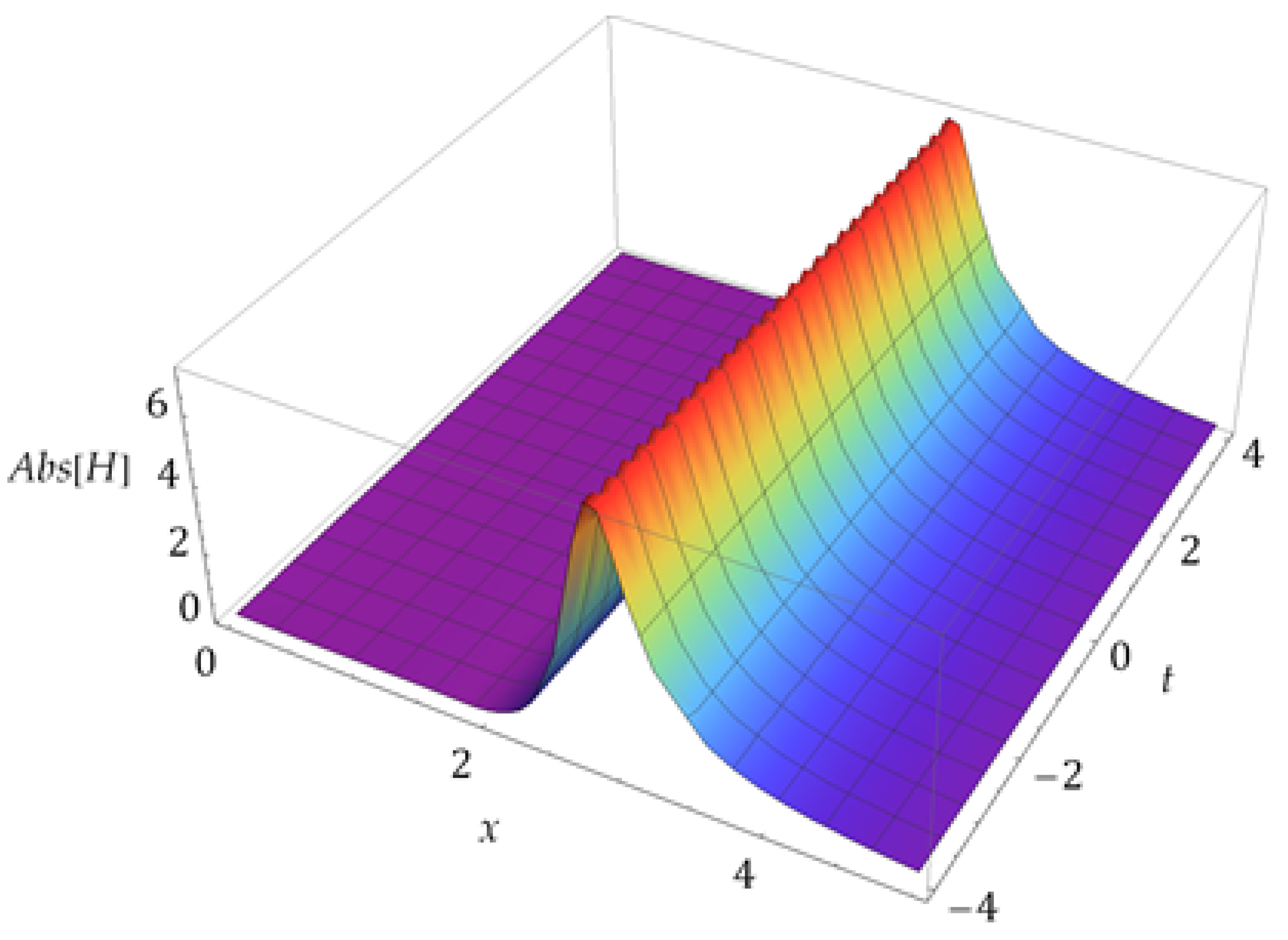
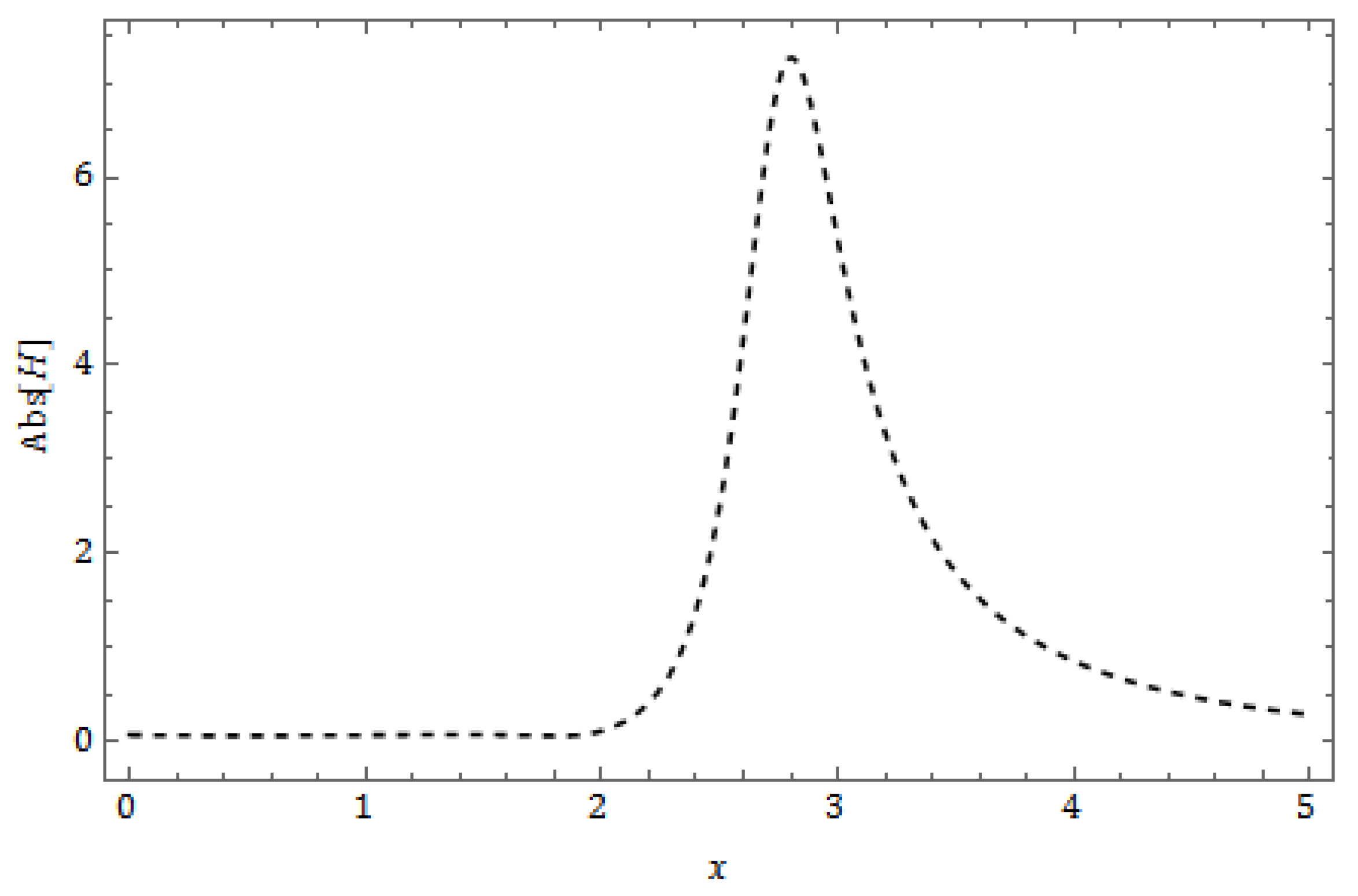
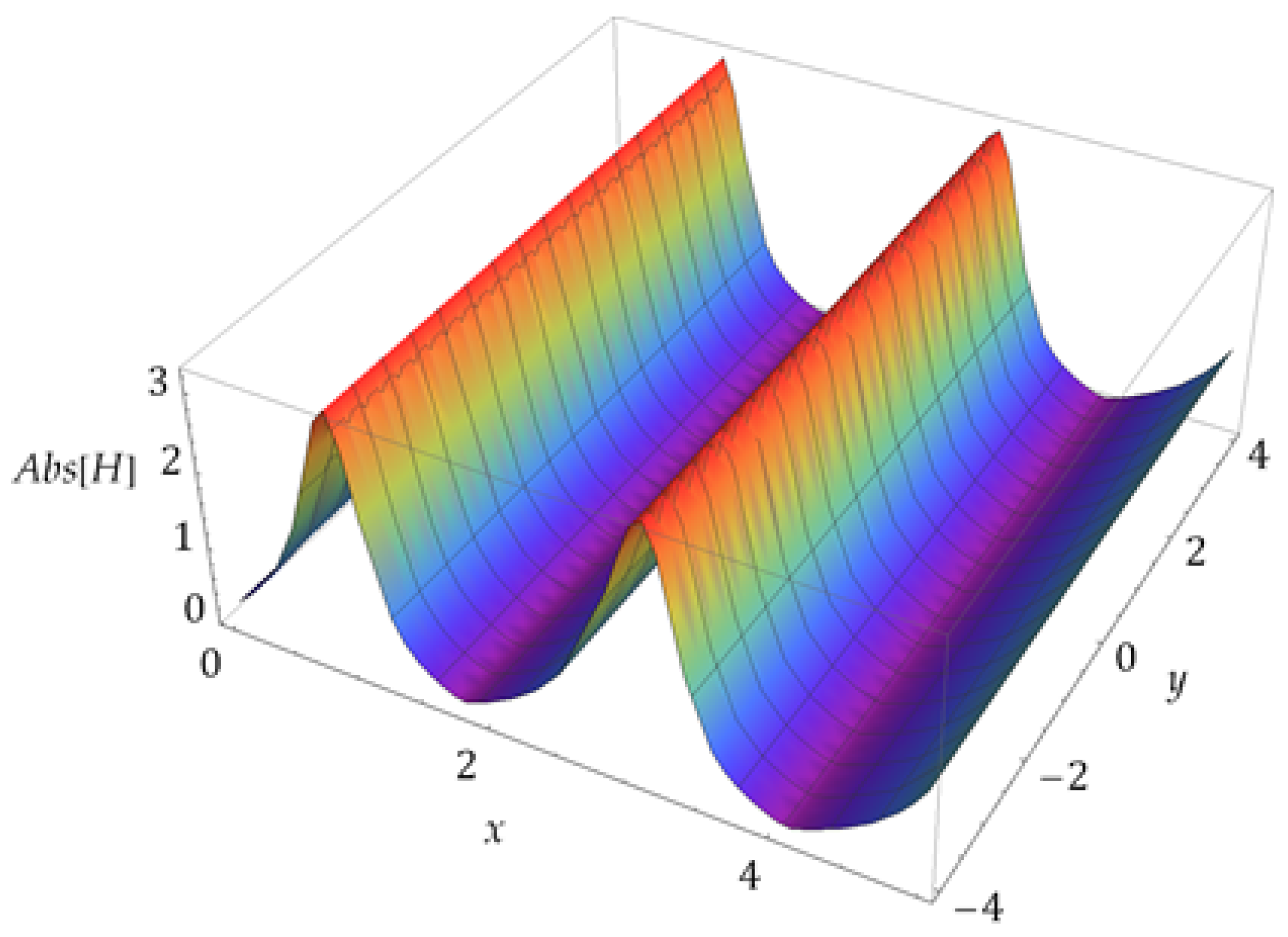
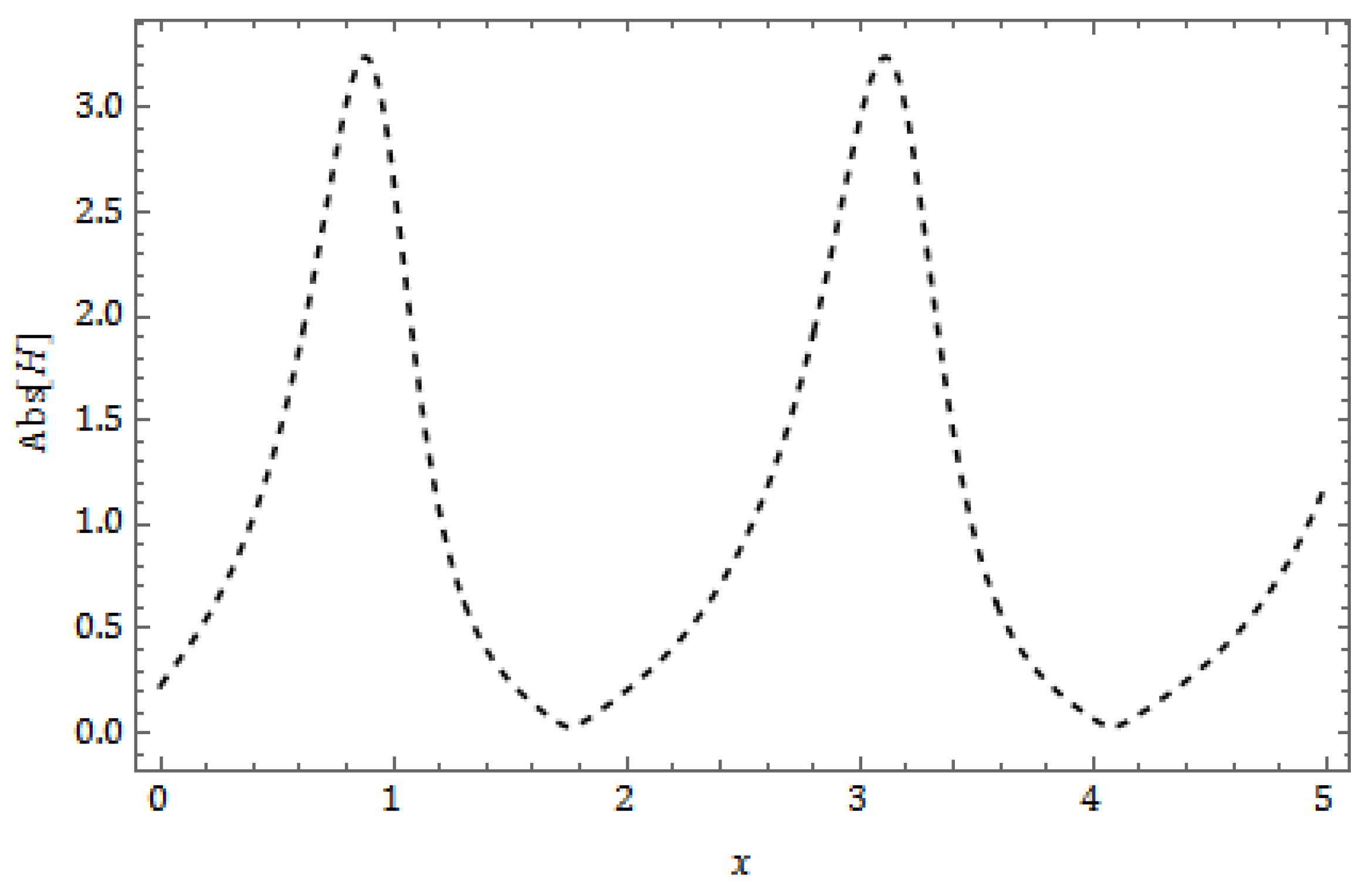
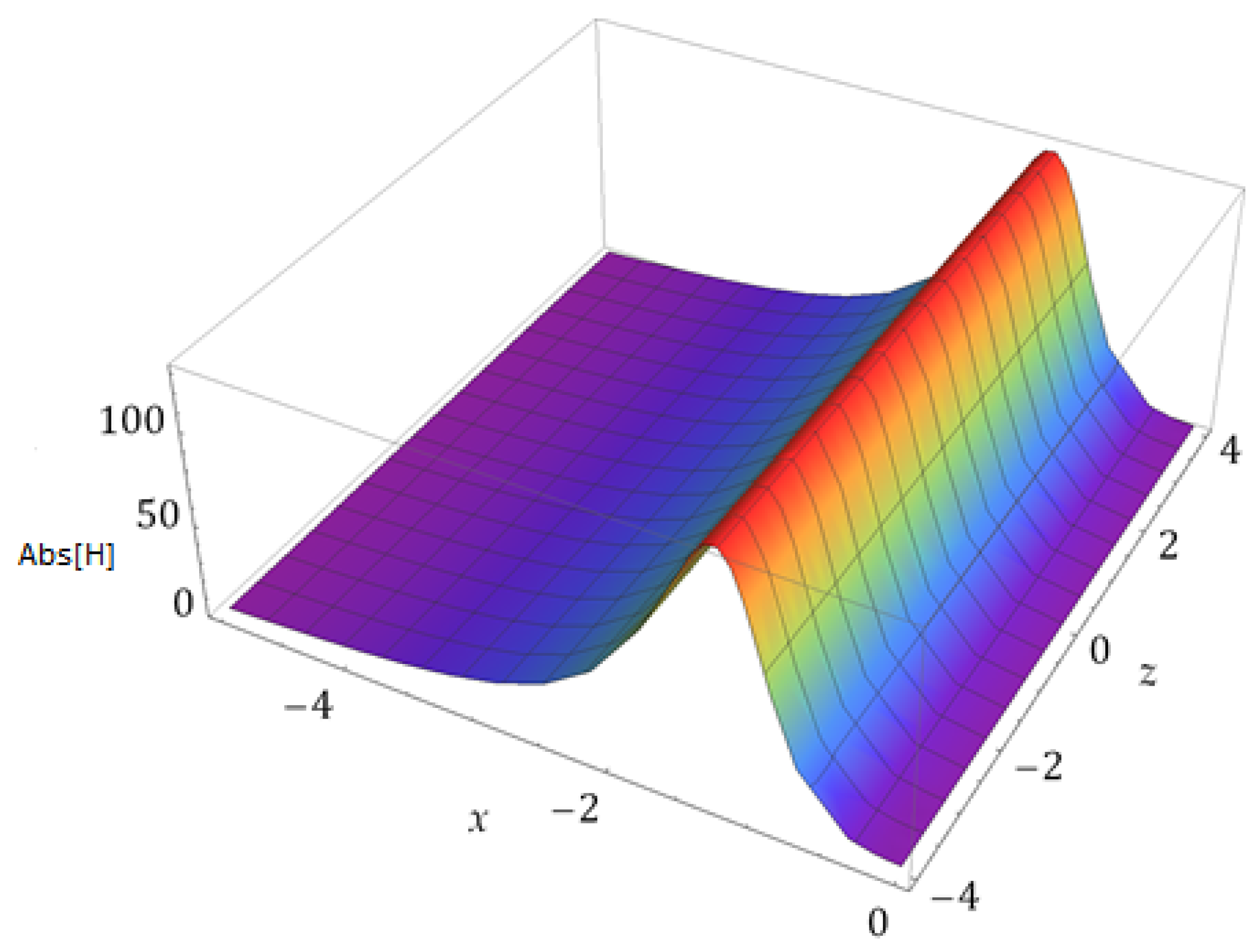
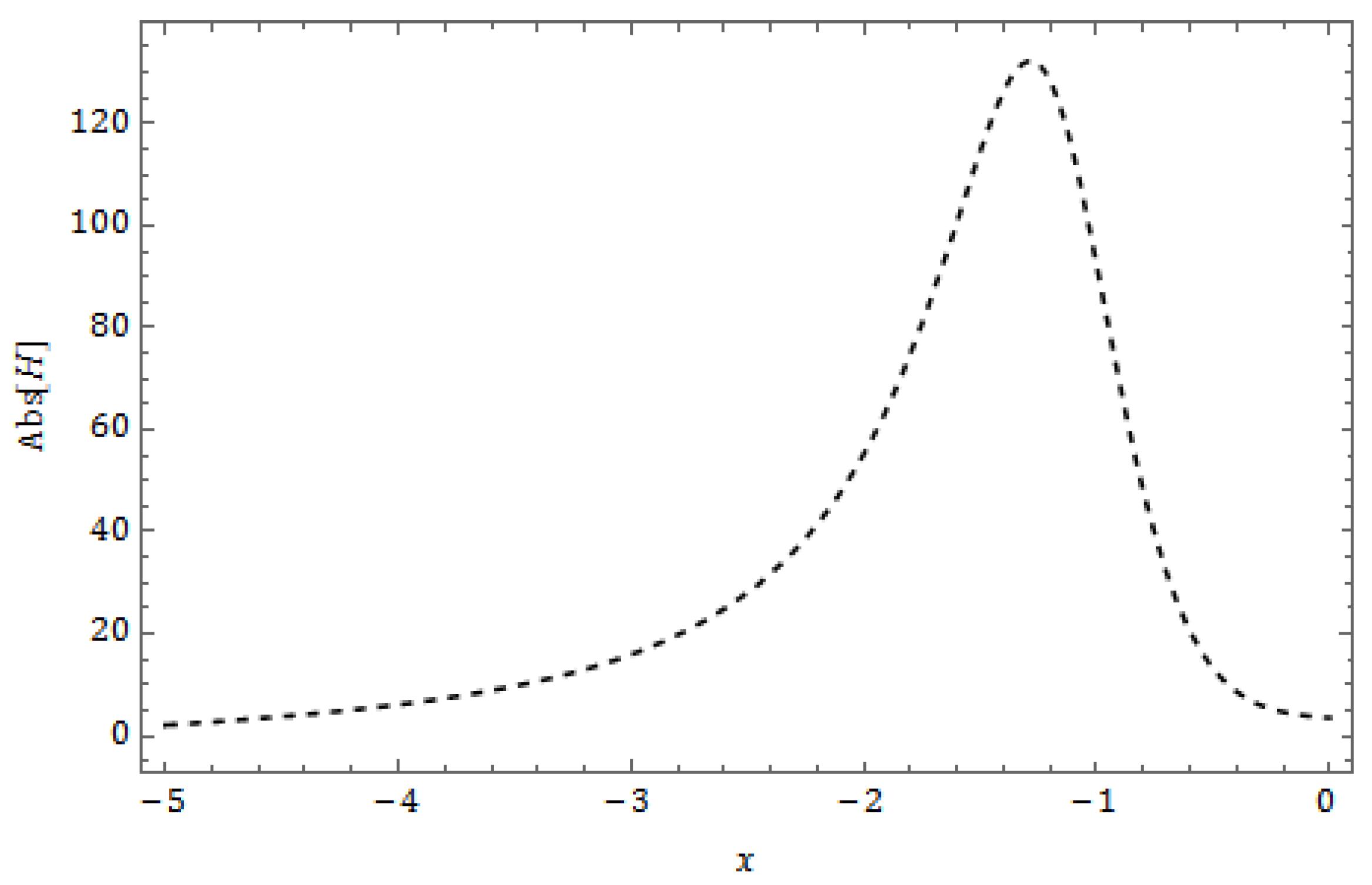
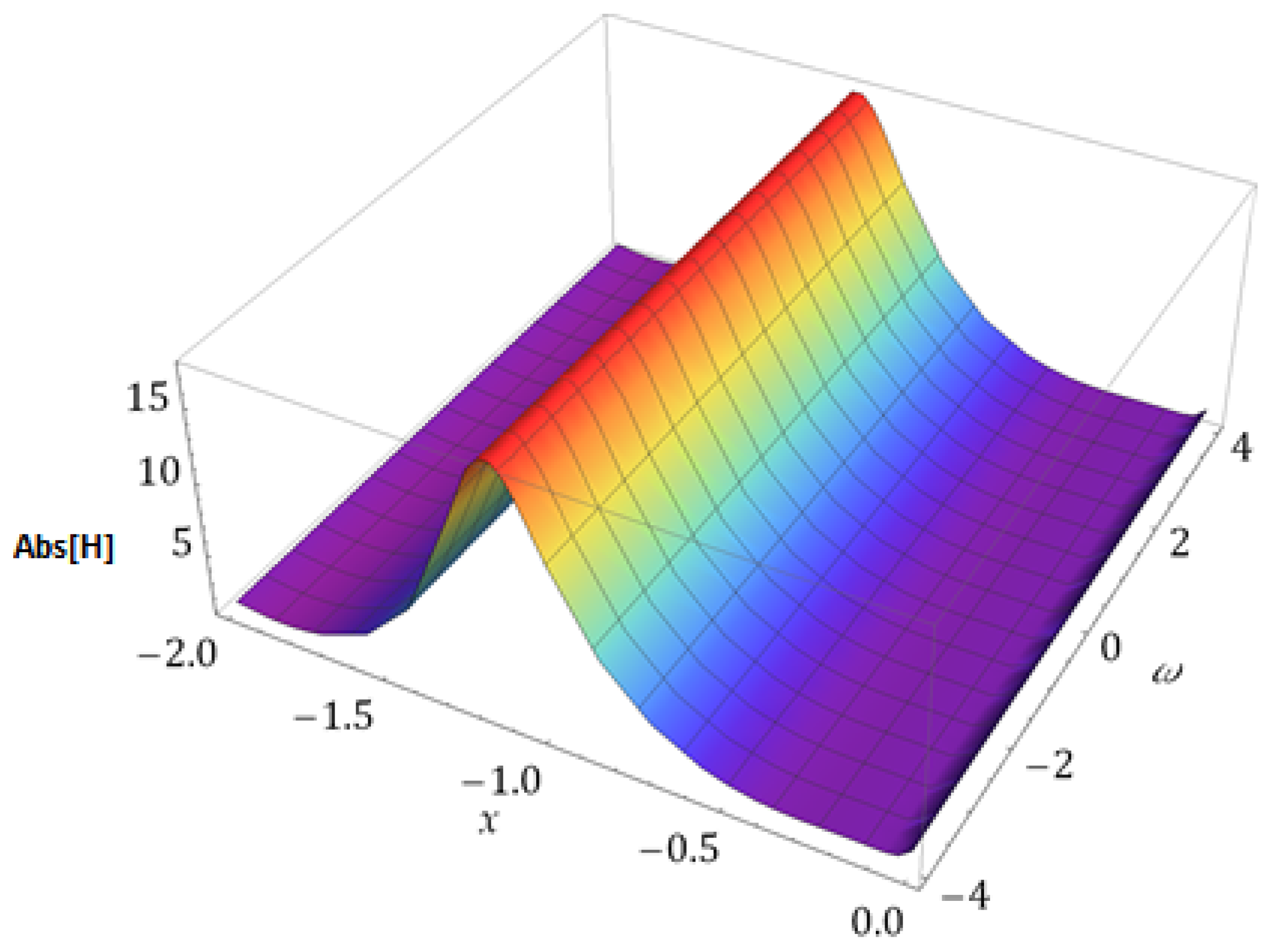
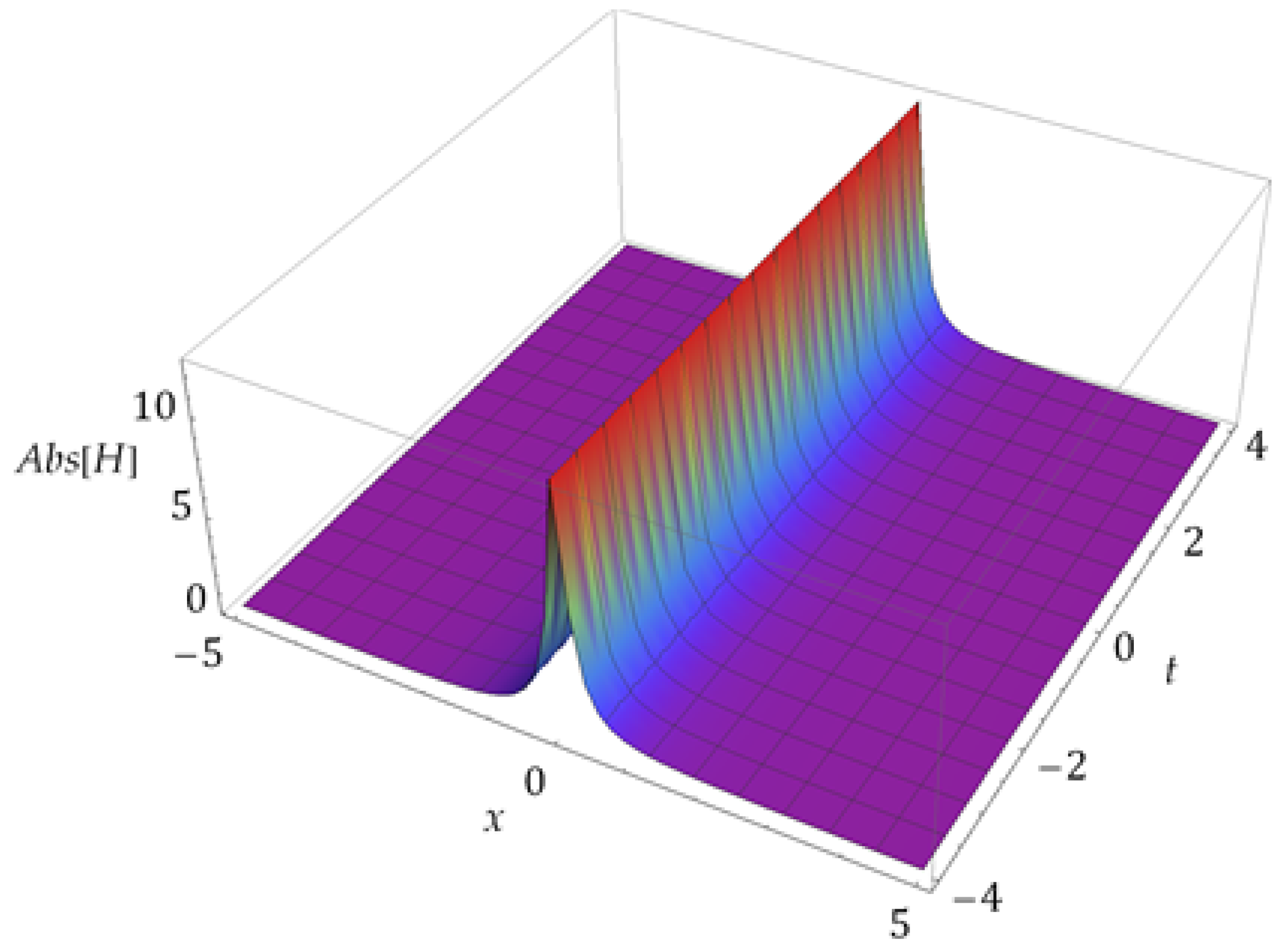
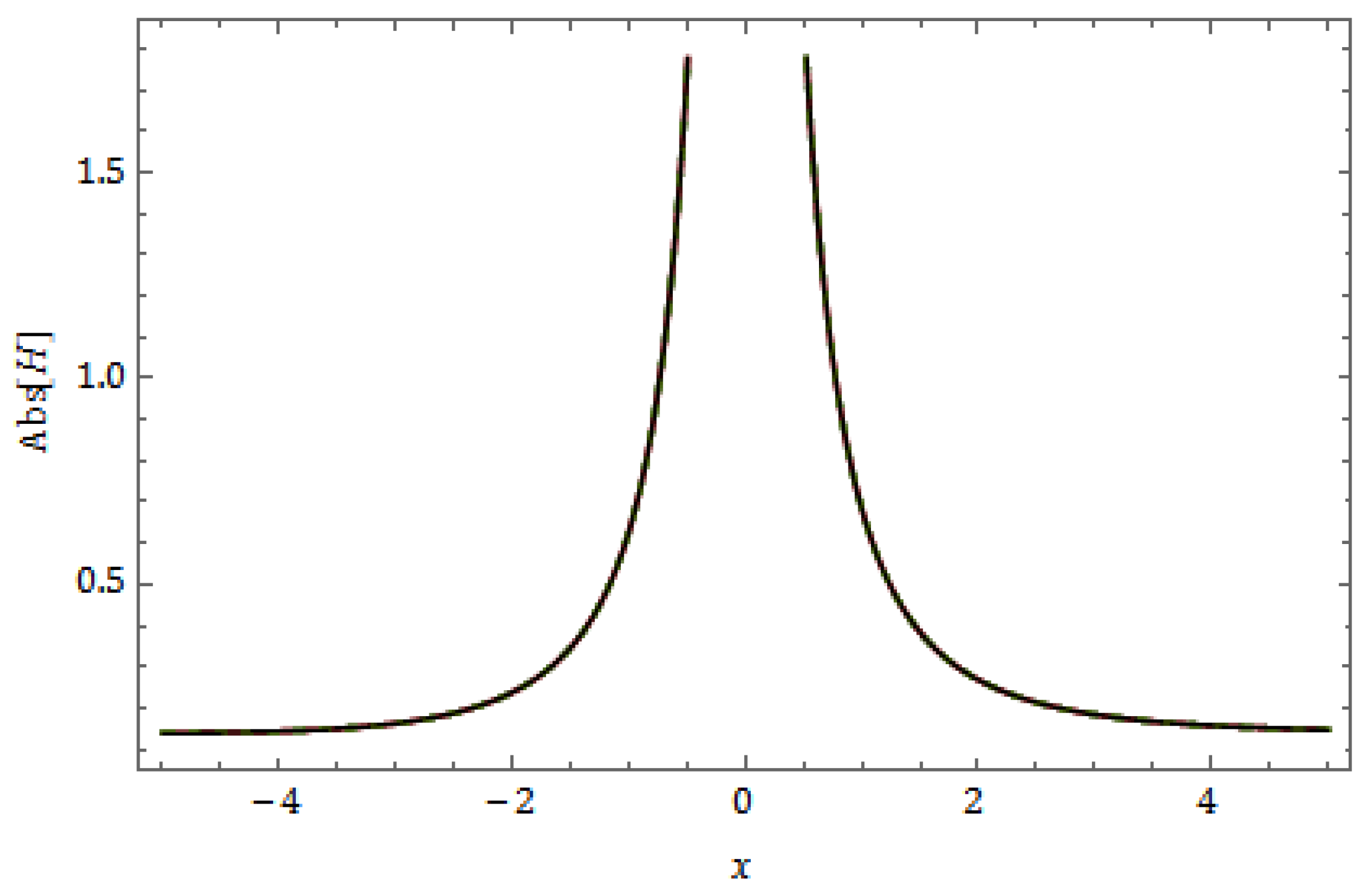
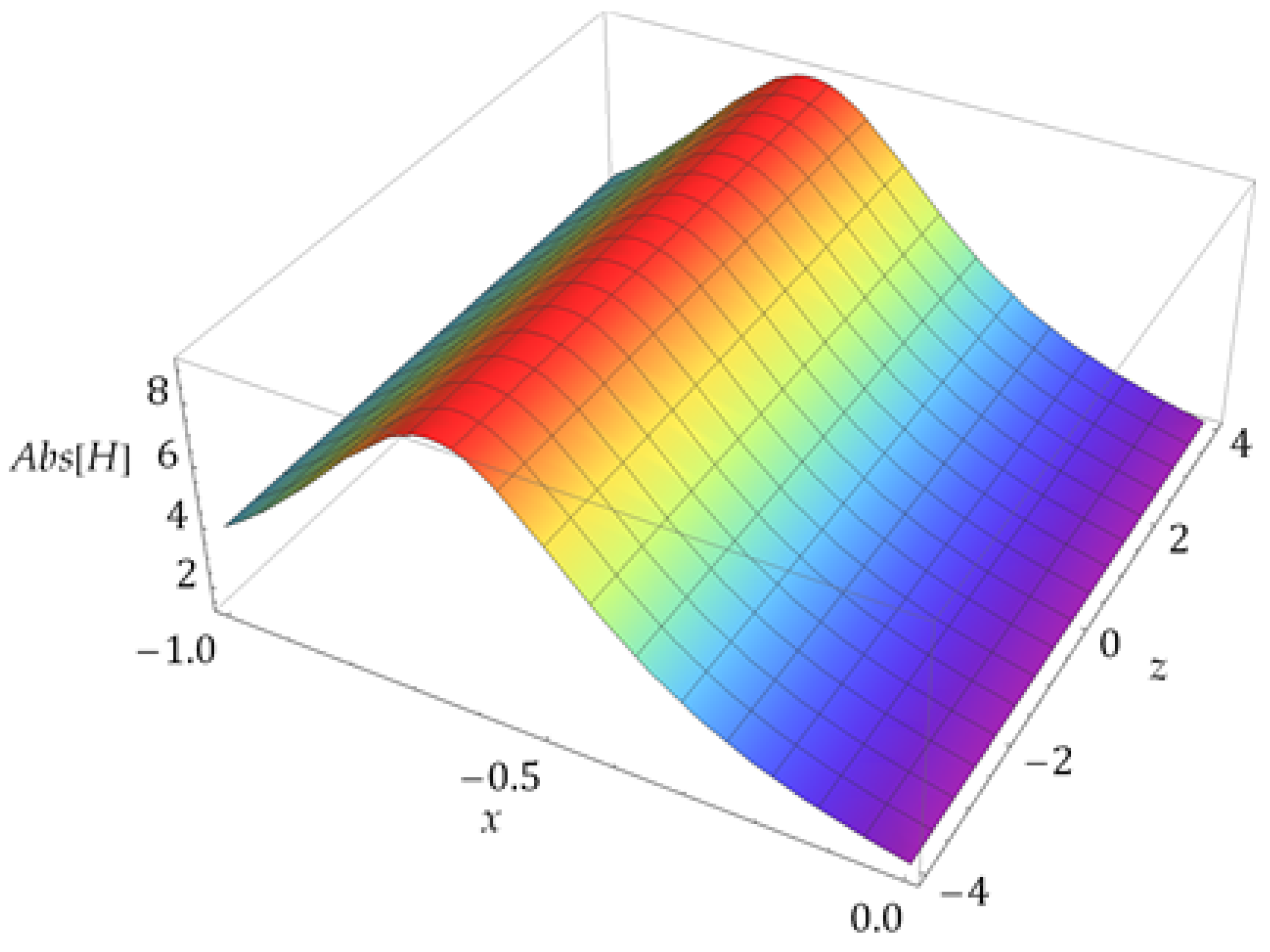
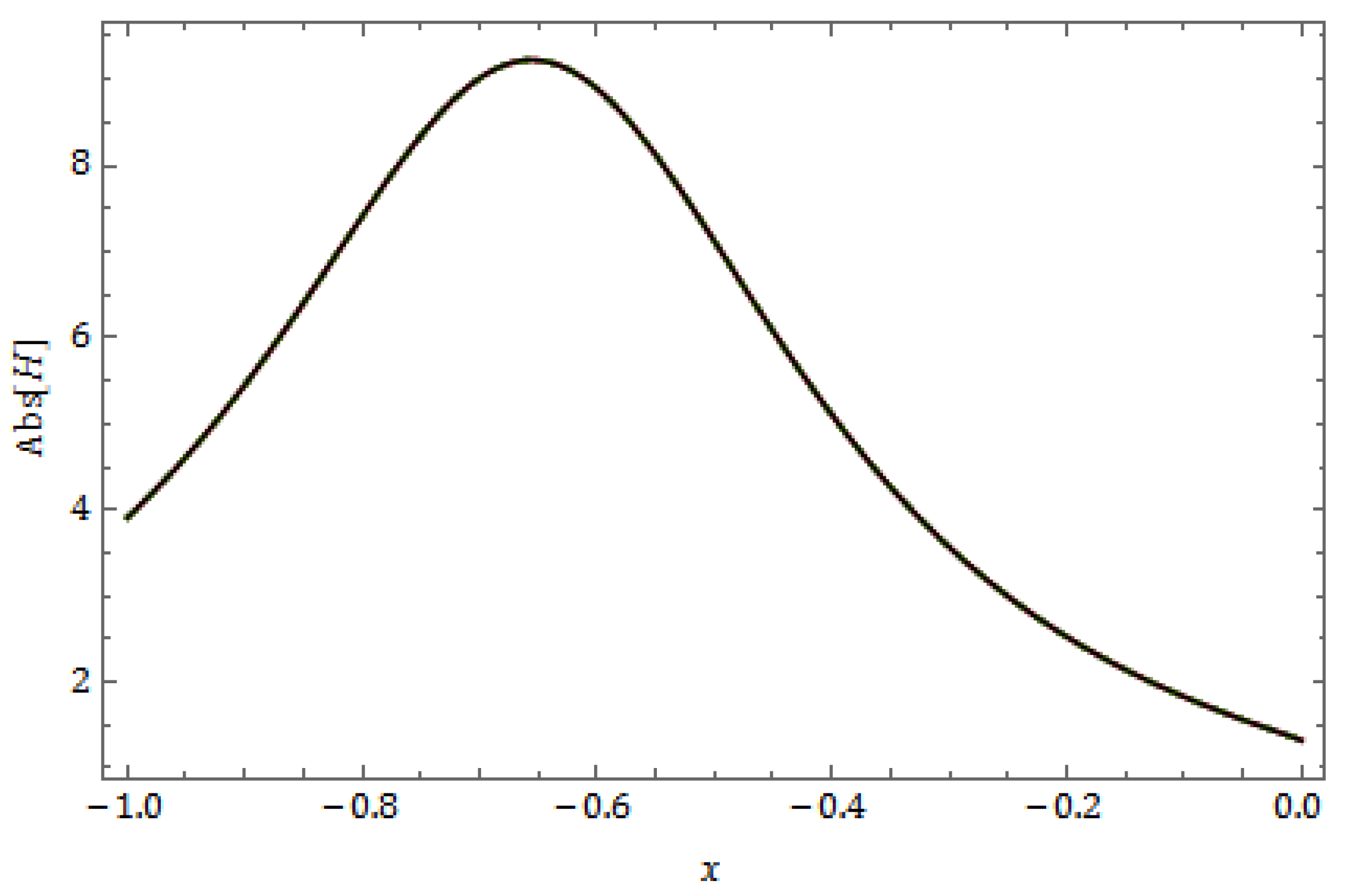
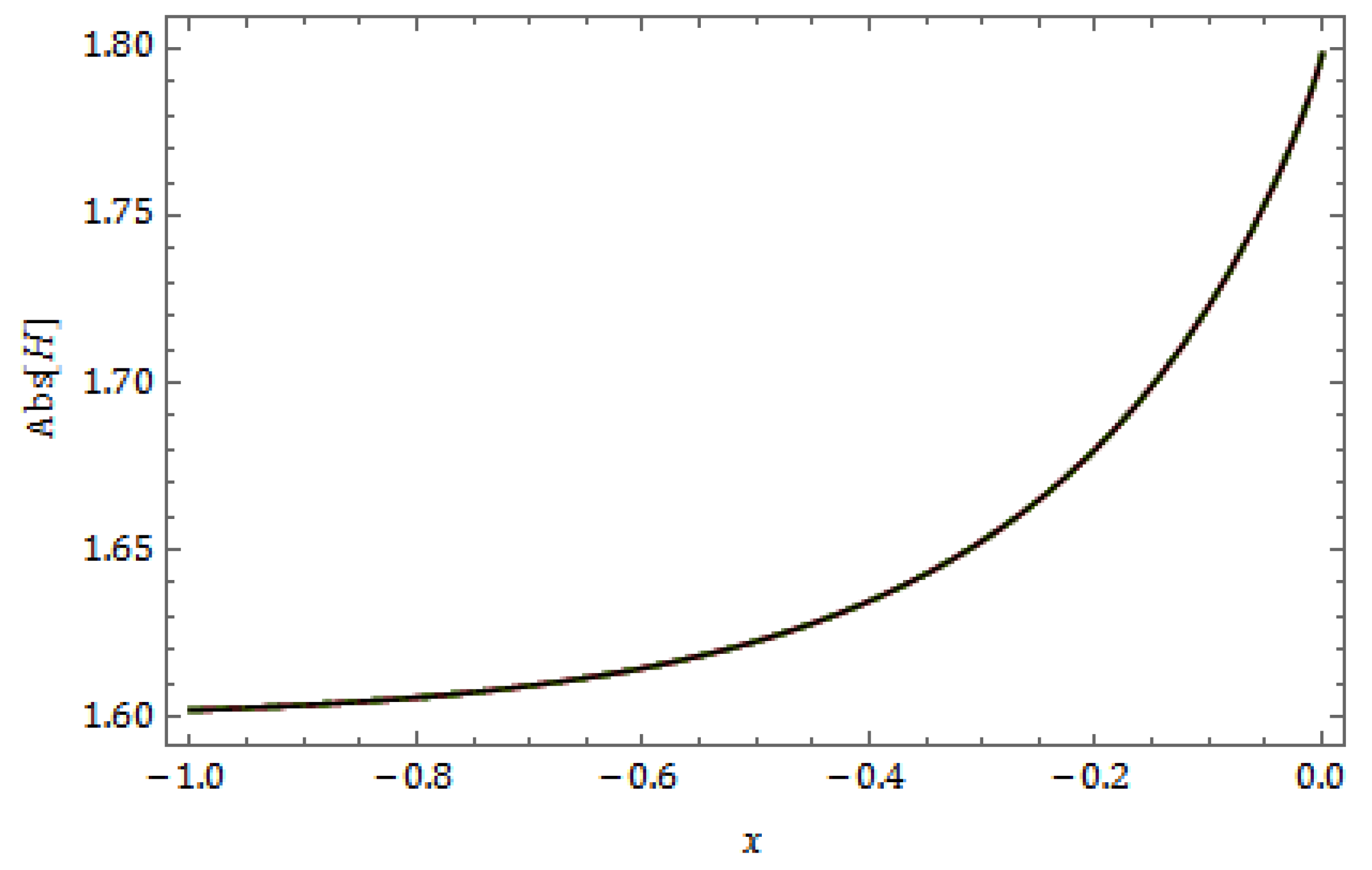
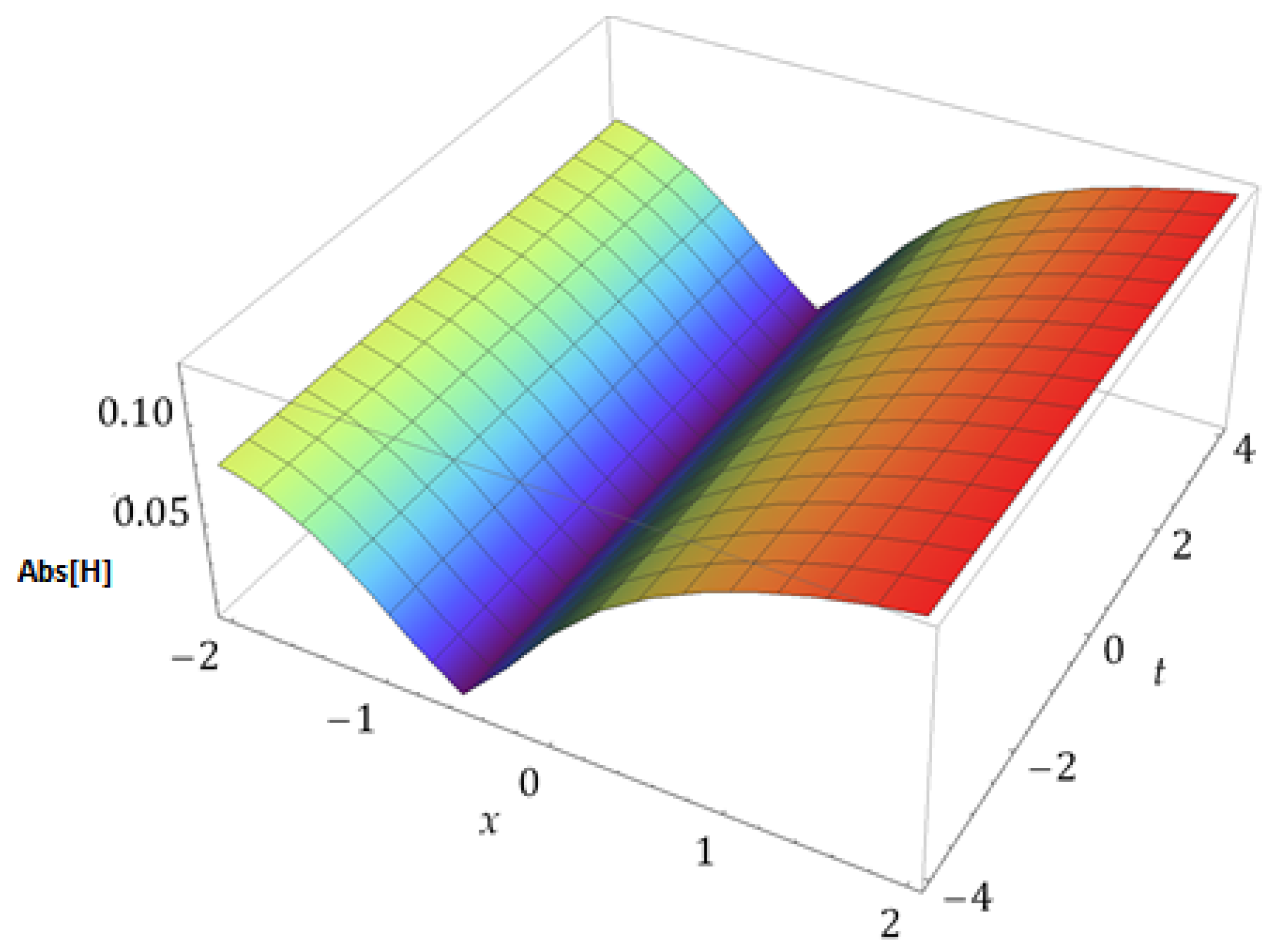
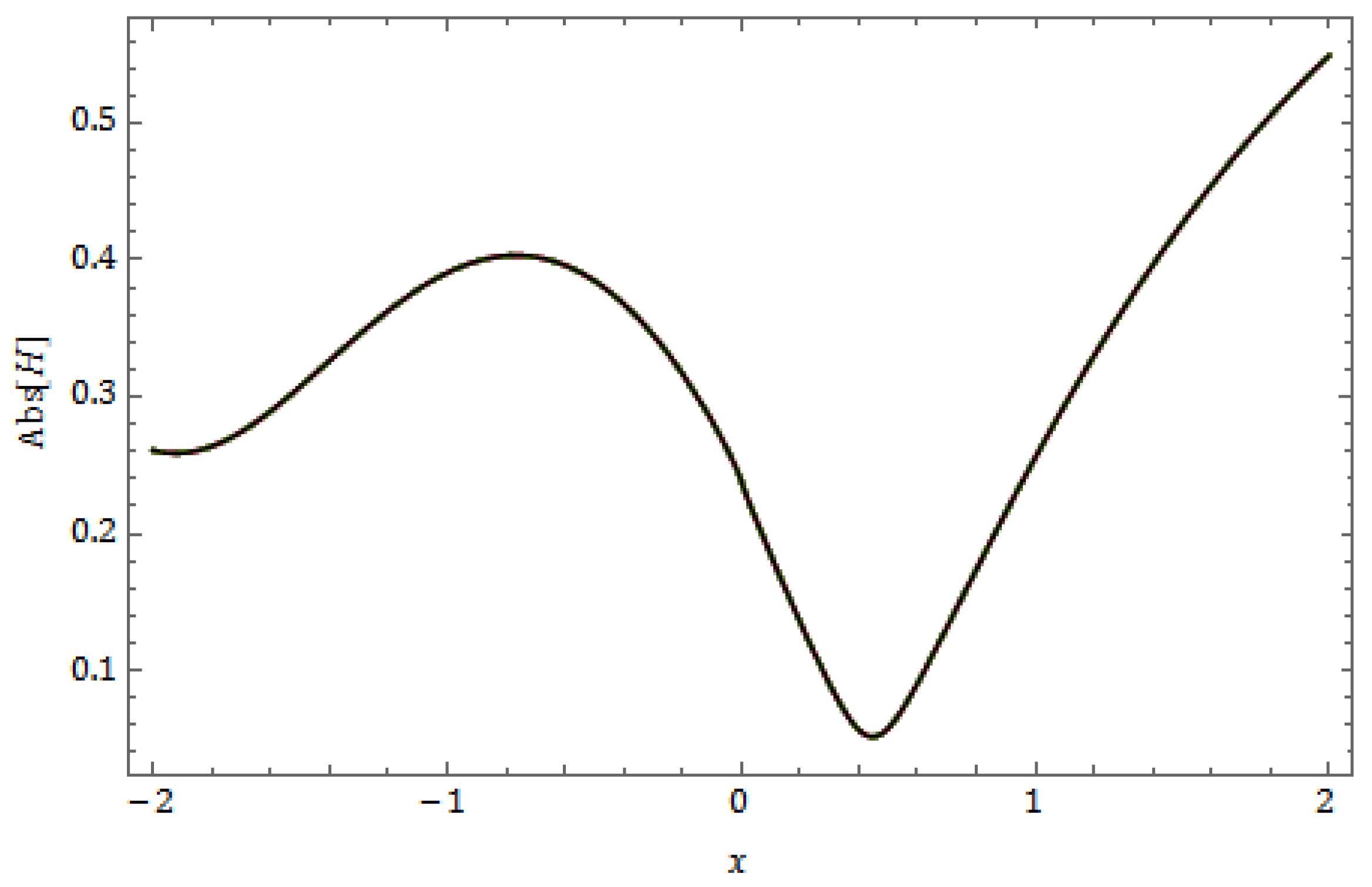
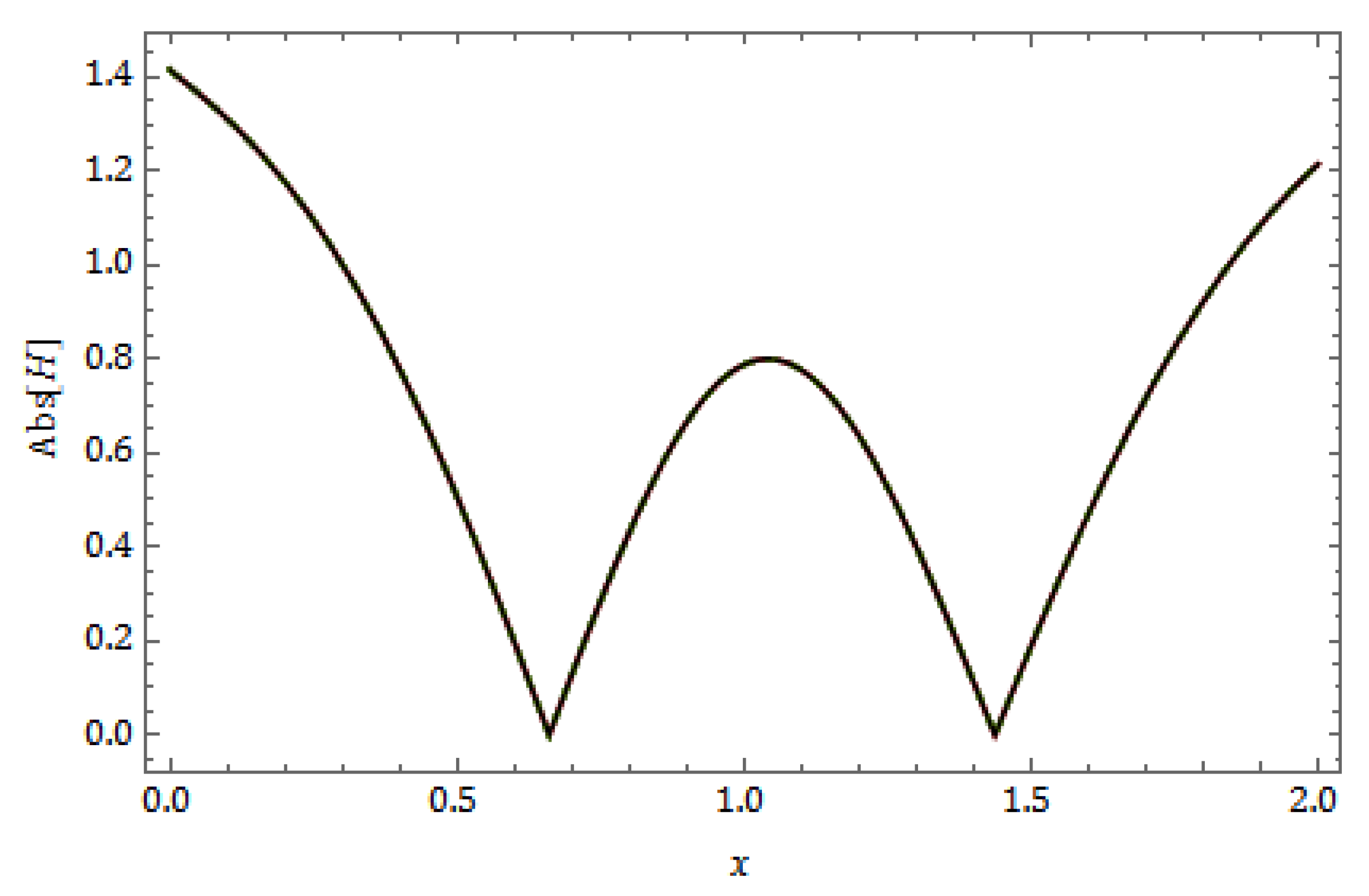
4. Physical Behavior of Solitons
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Akter, S.; Hossain, M.D.; Uddin, M.F.; Hafez, M.G. Collisional Solitons Described by Two-Sided Beta Time Fractional Korteweg-de Vries Equations in Fluid-Filled Elastic Tubes. Adv. Math. Phys. 2023, 2023, 9594339. [Google Scholar] [CrossRef]
- Uddin, M.F.; Hafez, M.G.; Hwang, I.; Park, C. Effect of space fractional parameter on nonlinear ion acoustic shock wave excitation in an unmagnetized relativistic plasma. Front. Phys. 2022, 9, 766. [Google Scholar] [CrossRef]
- Hafez, M.G.; Iqbal, S.A.; Akther, S.; Uddin, M.F. Oblique plane waves with bifurcation behaviors and chaotic motion for resonant nonlinear Schrodinger equations having fractional temporal evolution. Results Phys. 2019, 15, 102778. [Google Scholar] [CrossRef]
- Uddin, M.F.; Hafez, M.G.; Hammouch, Z.; Rezazadeh, H.; Baleanu, D. Traveling wave with beta derivative spatial-temporal evolution for describing the nonlinear directional couplers with metamaterials via two distinct methods. Alex. Eng. J. 2021, 60, 1055–1065. [Google Scholar] [CrossRef]
- Uddin, M.F.; Hafez, M.G. Interaction of complex short wave envelope and real long wave described by the coupled Schrödinger–Boussinesq equation with variable coefficients and beta space fractional evolution. Results Phys. 2020, 19, 103268. [Google Scholar] [CrossRef]
- Uddin, M.F.; Hafez, M.G.; Iqbal, S.A. Dynamical plane wave solutions for the Heisenberg model of ferromagnetic spin chains with beta derivative evolution and obliqueness. Heliyon 2022, 8, e09199. [Google Scholar] [CrossRef] [PubMed]
- Uddin, M.F.; Hafez, M.G. Optical Wave Phenomena in Birefringent Fibers Described by Space-Time Fractional Cubic-Quartic Nonlinear Schrödinger Equation with the Sense of Beta and Conformable Derivative. Adv. Math. Phys. 2022, 2022, 7265164. [Google Scholar] [CrossRef]
- Ahmad, I.; Jalil, A.; Ullah, A.; Ahmad, S.; De la Sen, M. Some new exact solutions of (4+1)-dimensional Davey–Stewartson-Kadomtsev–Petviashvili equation. Results Phys. 2023, 45, 106240. [Google Scholar] [CrossRef]
- Sulaiman, T.A.; Yel, G.; Bulut, H. M-fractional solitons and periodic wave solutions to the Hirota-Maccari system. Mod. Phys. Lett. B 2019, 33, 1950052. [Google Scholar] [CrossRef]
- Sousa, J.V.d.A.C.; Capelas de Oliveira, E. A new truncated M-fractional derivative type unifying some fractional derivative types with classical properties. Int. J. Anal. Appl. 2018, 16, 83–96. [Google Scholar]
- Fokas, A.S. Integrable nonlinear evolution partial differential equations in 4+2 and 3+1 dimensions. Phys. Rev. Lett. 2006, 96, 190201. [Google Scholar] [CrossRef] [PubMed]
- Akbar, M.A.; Abdullah, F.A.; Gepreel, K.A. The solitonic solutions of finite depth long water wave models. Results Phys. 2022, 37, 105570. [Google Scholar] [CrossRef]
- Talafha, A.M.; Jhangeer, A.; Kazmi, S.S. Dynamical analysis of (4+1)-dimensional Davey Srewartson Kadomtsev Petviashvili equation by employing Lie symmetry approach. Ain Shams Eng. J. 2023, 14, 102537. [Google Scholar] [CrossRef]
- El-Shorbagy, M.A.; Akram, S.; Rahman, M.u. Propagation of solitary wave solutions to (4+1)-dimensional Davey–Stewartson–Kadomtsev–Petviashvili equation arise in mathematical physics and stability analysis. Partial. Differ. Equ. Appl. Math. 2024, 10, 100669. [Google Scholar] [CrossRef]
- Ahmad, S.; Ullah, A.; Ahmad, S.; Saifullah, S.; Shokri, A. Periodic solitons of Davey Stewartson Kadomtsev Petviashvili equation in (4+1)-dimension. Results Phys. 2023, 50, 106547. [Google Scholar] [CrossRef]
- Junjua, M.D.; Mostafa, A.M.; AlQahtani, N.F.; Bekir, A. Impact of truncated M-fractional derivative on the new types of exact solitons to the (4+1)-dimensional DSKP model. Mod. Phys. Lett. B 2024, 2024, 2450313. [Google Scholar] [CrossRef]
- Ullah, M.S.; Abdeljabbar, A.; Roshid, H.O.; Ali, M.Z. Application of the unified method to solve the Biswas—Arshed model. Results Phys. 2022, 42, 105946. [Google Scholar] [CrossRef]
- Nandi, D.C.; Ullah, M.S.; Ali, M.Z. Application of the unified method to solve the ion sound and Langmuir waves model. Heliyon 2022, 8, e10924. [Google Scholar] [CrossRef] [PubMed]
- Wang, X.; Javed, S.A.; Majeed, A.; Kamran, M.; Abbas, M. Investigation of exact solutions of nonlinear evolution equations using unified method. Mathematics 2022, 10, 2996. [Google Scholar] [CrossRef]
- Shahen, N.H.M.; Rahman, M.M. Dispersive solitary wave structures with MI Analysis to the unidirectional DGH equation via the unified method. Partial. Differ. Equ. Appl. Math. 2022, 6, 100444. [Google Scholar]
- Alam, L.M.B.; Jiang, X. Exact and explicit traveling wave solution to the time-fractional phi-four and (2+1) dimensional CBS equations using the modified extended tanh-function method in mathematical physics. Partial. Differ. Equ. Appl. Math. 2021, 4, 100039. [Google Scholar] [CrossRef]
- Zahran, E.H.M.; Khater, M.M.A. Modified extended tanh-function method and its applications to the Bogoyavlenskii equation. Appl. Math. Model. 2016, 40, 1769–1775. [Google Scholar] [CrossRef]
- Raslan, K.R.; Ali, K.K.; Shallal, M.A. The modified extended tanh method with the Riccati equation for solving the space-time fractional EW and MEW equations, Chaos. Solitons Fractals 2017, 103, 404–409. [Google Scholar] [CrossRef]


















Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alsharidi, A.K.; Junjua, M.-u.-D. Kink, Dark, Bright, and Singular Optical Solitons to the Space–Time Nonlinear Fractional (41)-Dimensional Davey–Stewartson–Kadomtsev–Petviashvili Model+. Fractal Fract. 2024, 8, 388. https://doi.org/10.3390/fractalfract8070388
Alsharidi AK, Junjua M-u-D. Kink, Dark, Bright, and Singular Optical Solitons to the Space–Time Nonlinear Fractional (41)-Dimensional Davey–Stewartson–Kadomtsev–Petviashvili Model+. Fractal and Fractional. 2024; 8(7):388. https://doi.org/10.3390/fractalfract8070388
Chicago/Turabian StyleAlsharidi, Abdulaziz Khalid, and Moin-ud-Din Junjua. 2024. "Kink, Dark, Bright, and Singular Optical Solitons to the Space–Time Nonlinear Fractional (41)-Dimensional Davey–Stewartson–Kadomtsev–Petviashvili Model+" Fractal and Fractional 8, no. 7: 388. https://doi.org/10.3390/fractalfract8070388






