A New Approach to Designing Multi-Element Planar Solar Concentrators: Geometry Optimization for High Angular Selectivity and Efficient Solar Energy Collection
Abstract
1. Introduction
2. Theoretical Model of Unit Cells
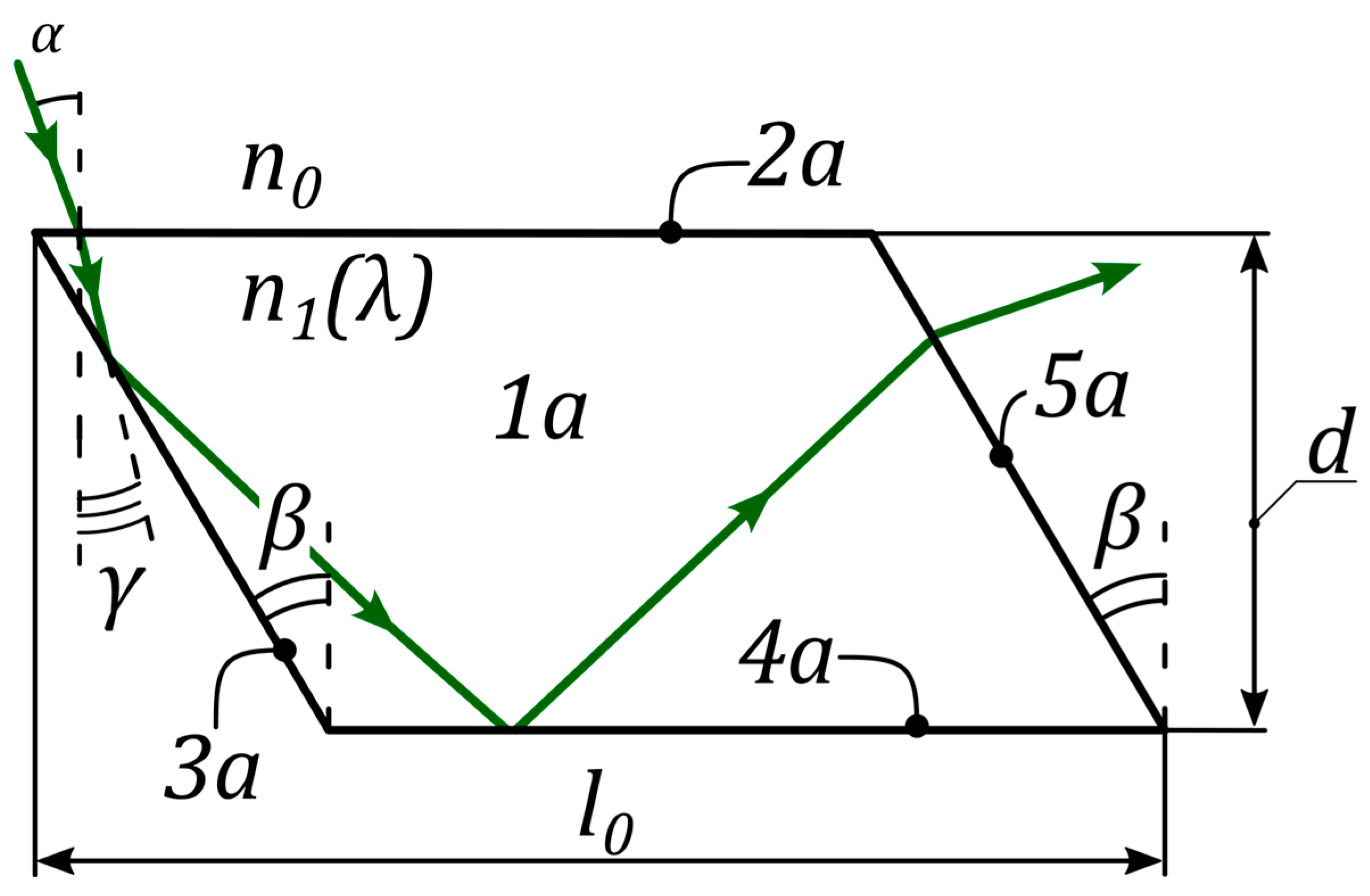
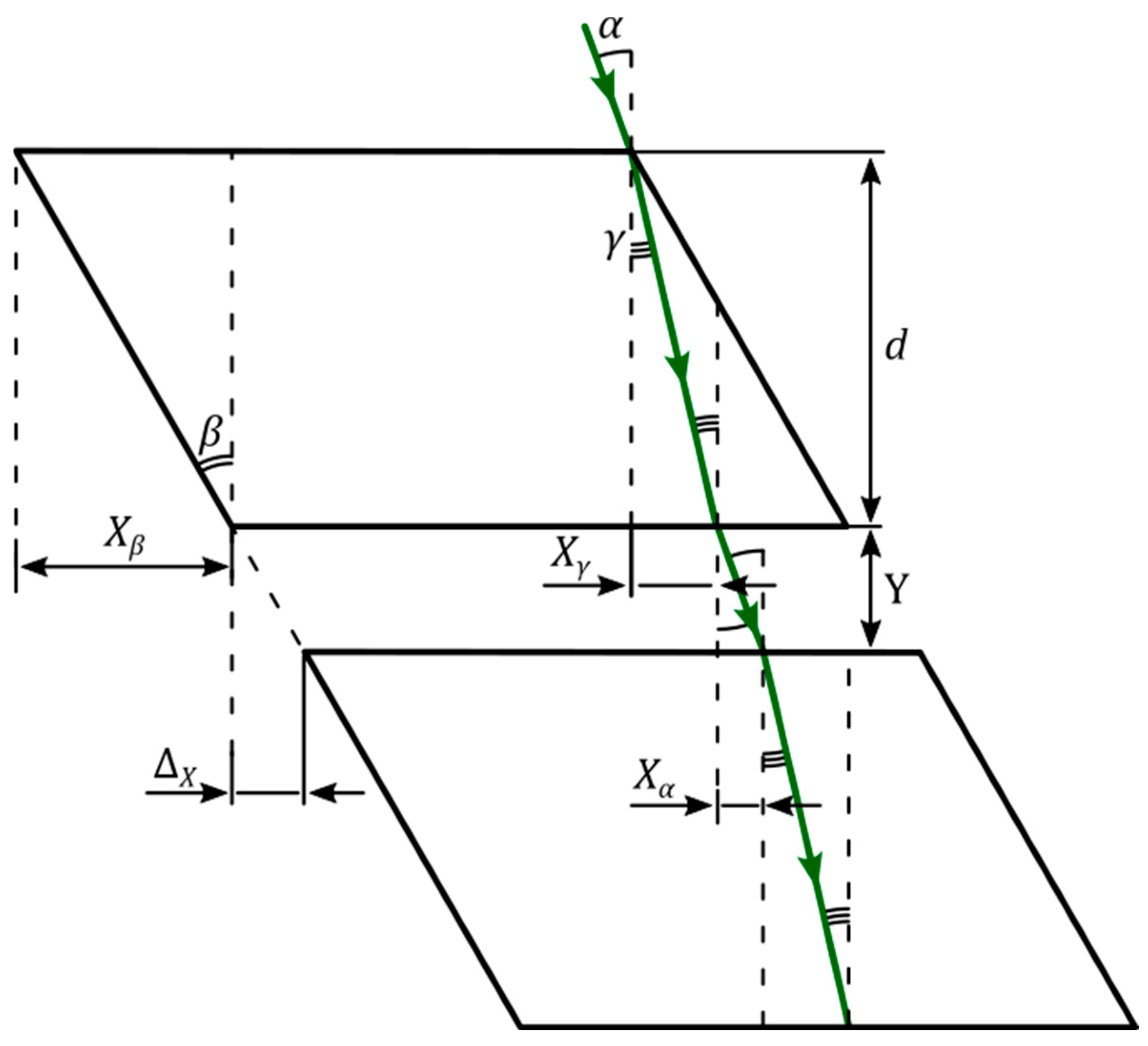
- Refraction at the Receiving Surface: Electromagnetic waves emitted by the Sun strike the receiving surface (2a) of the plates (1a) at an angle . Upon entering the material, the waves are refracted according to Snell’s law due to the difference in refractive indices between the external medium and the material of the concentrator cell , where represents the refractive index of the cell material.
- Propagation and First Reflection: As illustrated in Figure 2, the refracted electromagnetic wave propagates at an angle toward the nearest end surface (3a) of the corresponding plate (1a). At this surface, the wave undergoes TIR, adhering to the principles of geometric optics.
- Subsequent Reflections and Propagation: Following the first reflection, the electromagnetic wave travels along the plate (1a) toward the surface (4a), where it is again reflected via TIR. This reflection directs the wave toward the opposite end surface (5a), ensuring its continued propagation within the cell.
3. Theoretical Model of Solar Concentrator
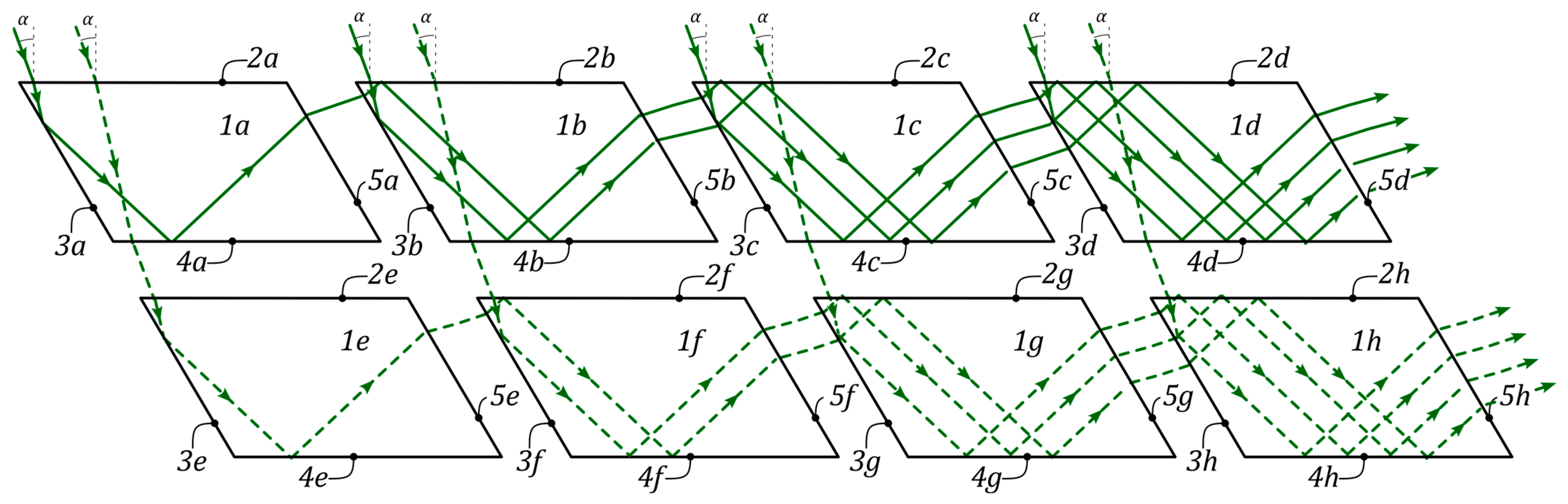
- Wave Propagation Between Cells: As depicted in Figure 9, an electromagnetic wave exits the first plate (1a) and propagates through the air to the adjacent cell (1b) in the row.
- Refraction at the Next Cell: The wave enters the end surface (3b) of the plate (1b) and is refracted due to the difference in refractive indices and .
- Reflection Within the Cell: The wave propagates along plate (1b) toward the receiving surface (2b), where it undergoes TIR toward the opposite surface (4b).
- Second Reflection and Propagation: The wave is reflected again from the surface (4b) via TIR and propagates toward the end surface (5b).
- Repetition Across Cells: The wave exits plate (1b), and the process repeats for each subsequent cell in the row. The propagation concludes at the exit of the final cell, after which the wave travels to the radiation receiver (not shown in Figure 9).
- Waves Incident on Intermediate Cells: If waves strike the receiving surfaces (2a or 2b) of plates (1a and 1b) that are not at the row’s beginning, the process described above repeats for each respective cell.
- Waves Missing End Surfaces: Waves that strike the receiving surfaces (2a and 2d) but, due to refraction, miss the end surfaces (3a and 3d) exit through surfaces (4a and 4d) and propagate to the next row of plates (1e and 1h). The process repeats for each cell in the new row.
- Waves Passing Through Multiple Rows: Waves that do not strike the end surfaces of any plate pass through the rows until they reach the last row, where they finally interact with the end surfaces, repeating the described process.
4. Discussion
- Light Funnel/Homogenizer (Method 1): This method achieves a GCR of 1.5 with an AA range of 0° to 20° [3]. While it offers moderate concentration, its limited angular range restricts its applicability in environments with varying solar angles.
- Flat Mold (Method 2): With a GCR of 2 and an AA of 0° to 1° [4], this method provides higher concentration but is highly sensitive to alignment, making it impractical for most real-world applications.
- V-Shaped Trough (Method 3): This design achieves a GCR of 2 and an AA of 0° to 20° [5]. Although it offers a wider angular range than Method 2, its concentration efficiency remains relatively low.
- Wedge Prism (Method 4): This method achieves a higher GCR of 3.77 with an AA of 0° to 50° [6]. Its broader angular range makes it more versatile, but its concentration efficiency is still limited compared to more advanced designs.
- Compound Parabolic Concentrator (Method 5): With a GCR of 4 and an AA of 0° to 20° [8], this method offers improved concentration but remains constrained by its narrow angular range.
- Linear Fresnel Reflector (Method 6): This design achieves a GCR ranging from 6 to 15, with an AA of 0° to 1° [10]. While it provides high concentration, its extreme sensitivity to alignment limits its practicality.
- Parabolic Shape (Method 7): This method achieves a GCR of 70 with an AA of 0° to 0.26° [12]. Although it offers exceptional concentration, its extremely narrow angular range makes it unsuitable for applications requiring flexibility in solar tracking.
- Fresnel Lens (Method 8): With a GCR of 1000 and an AA of 0° to 1.3° [13], this method provides the highest concentration among the compared technologies. However, its narrow angular range and complexity in manufacturing and alignment pose significant challenges.
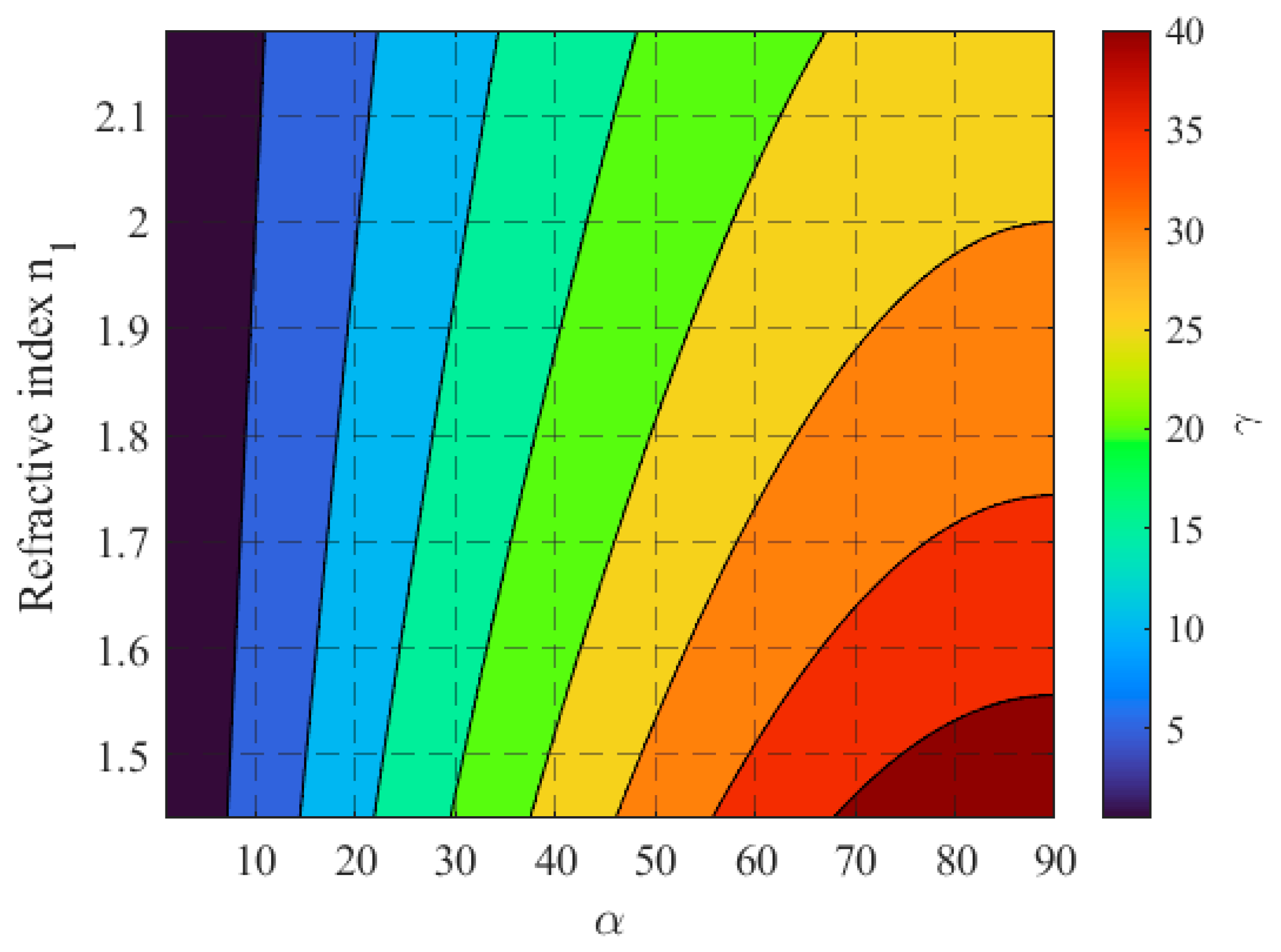
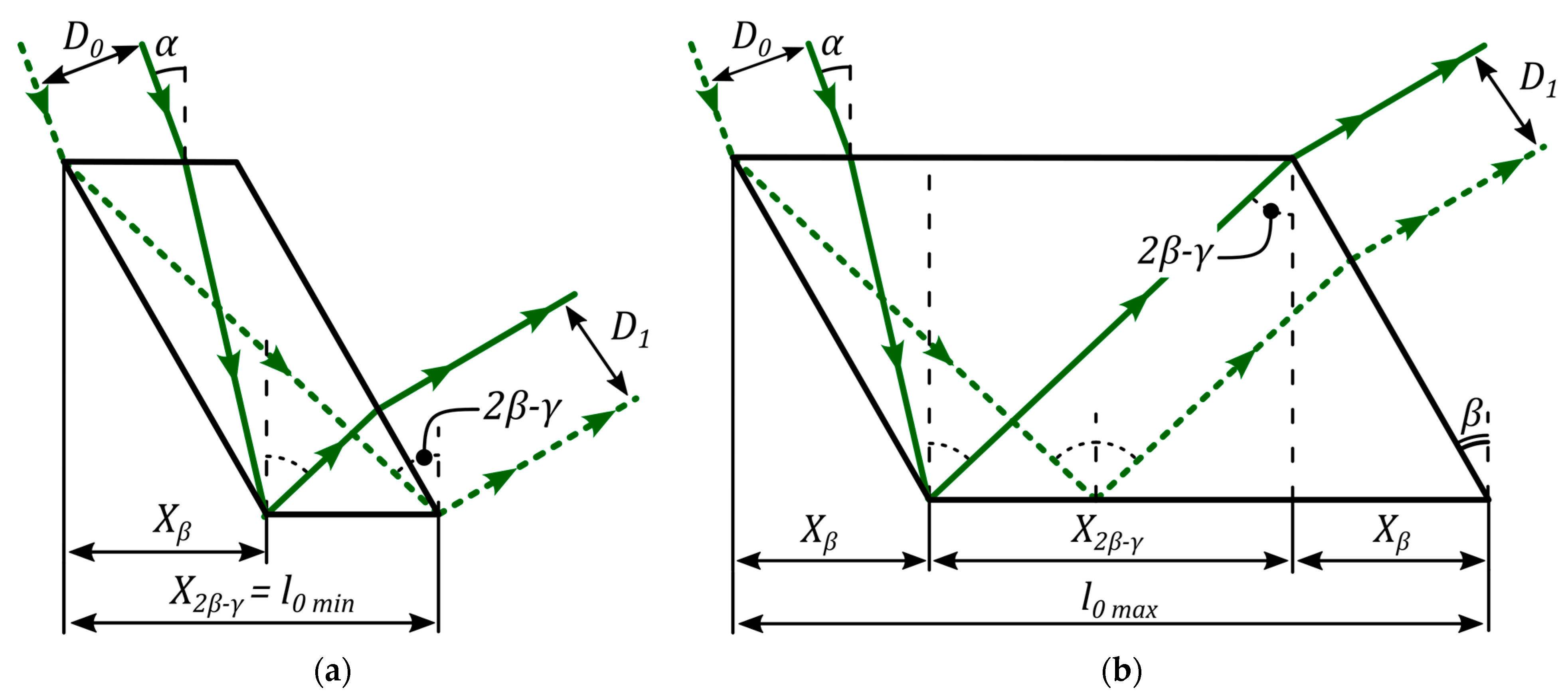
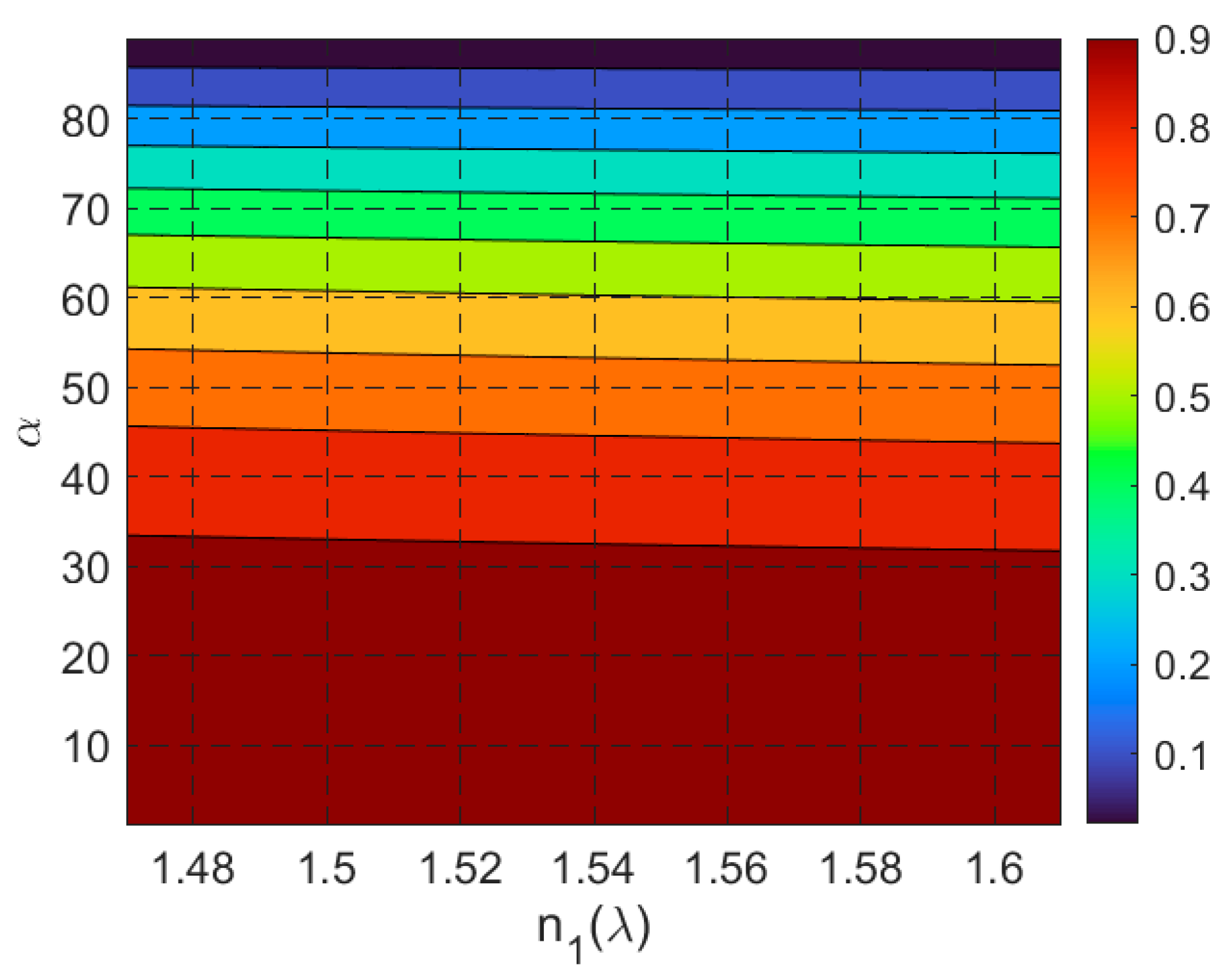
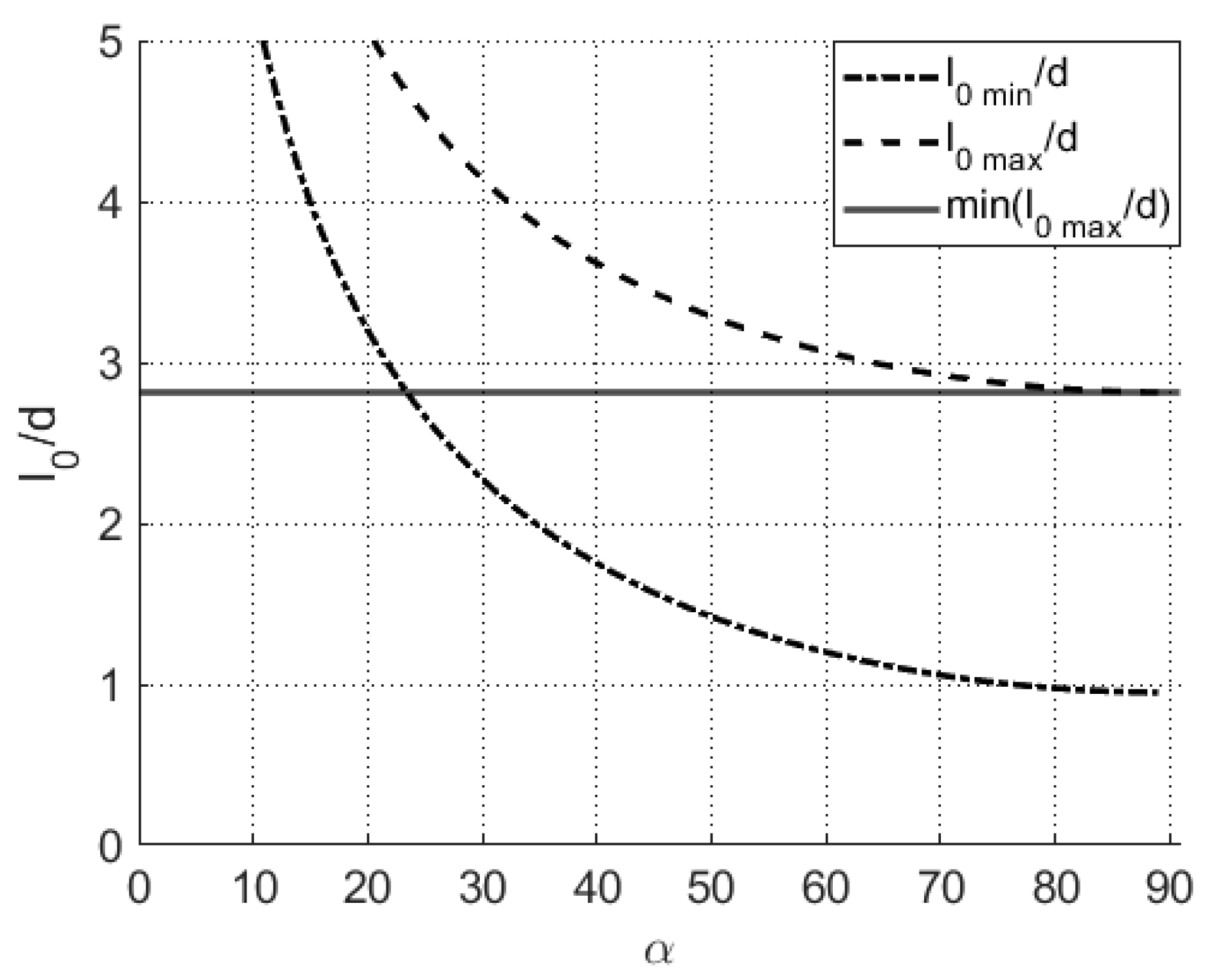
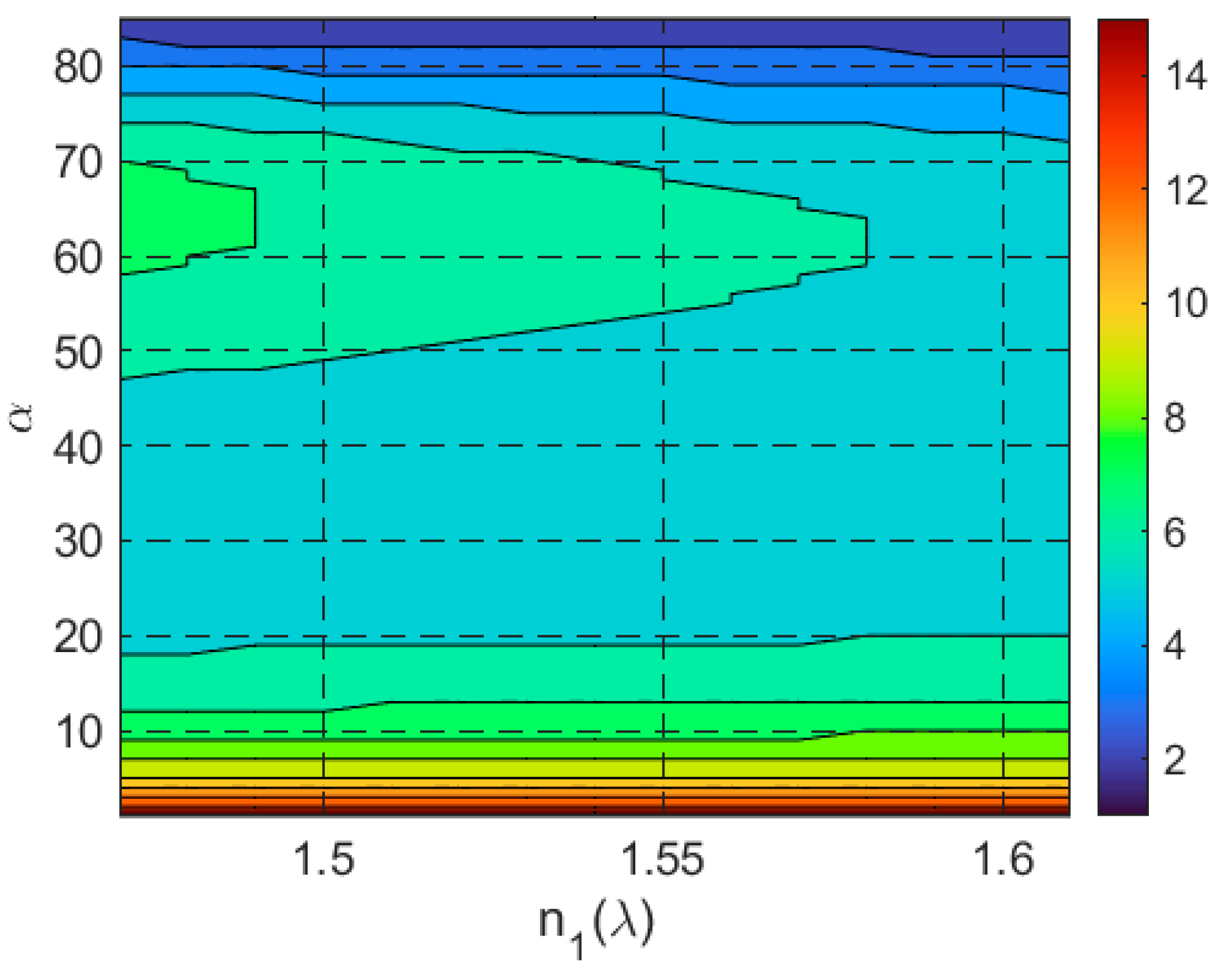
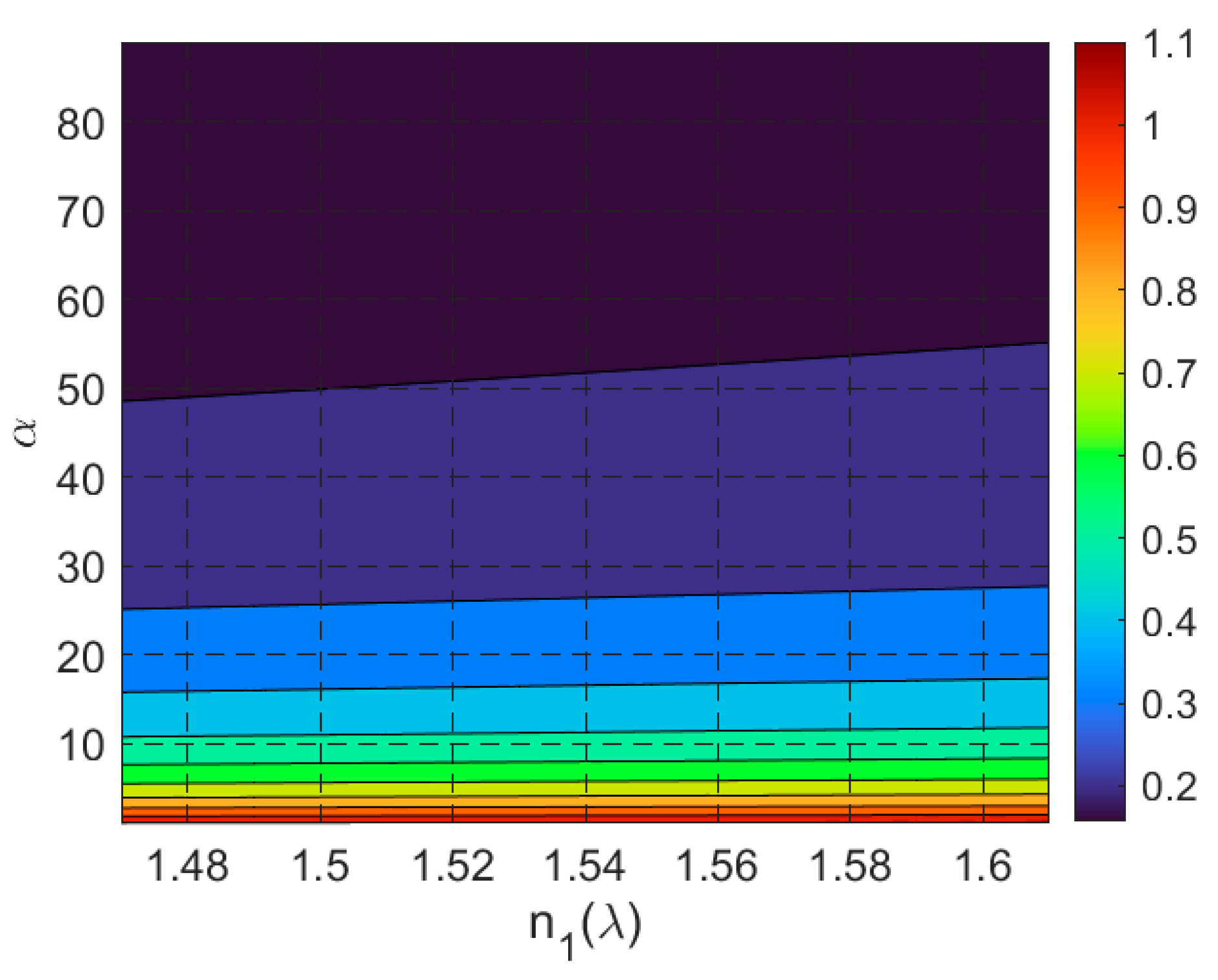
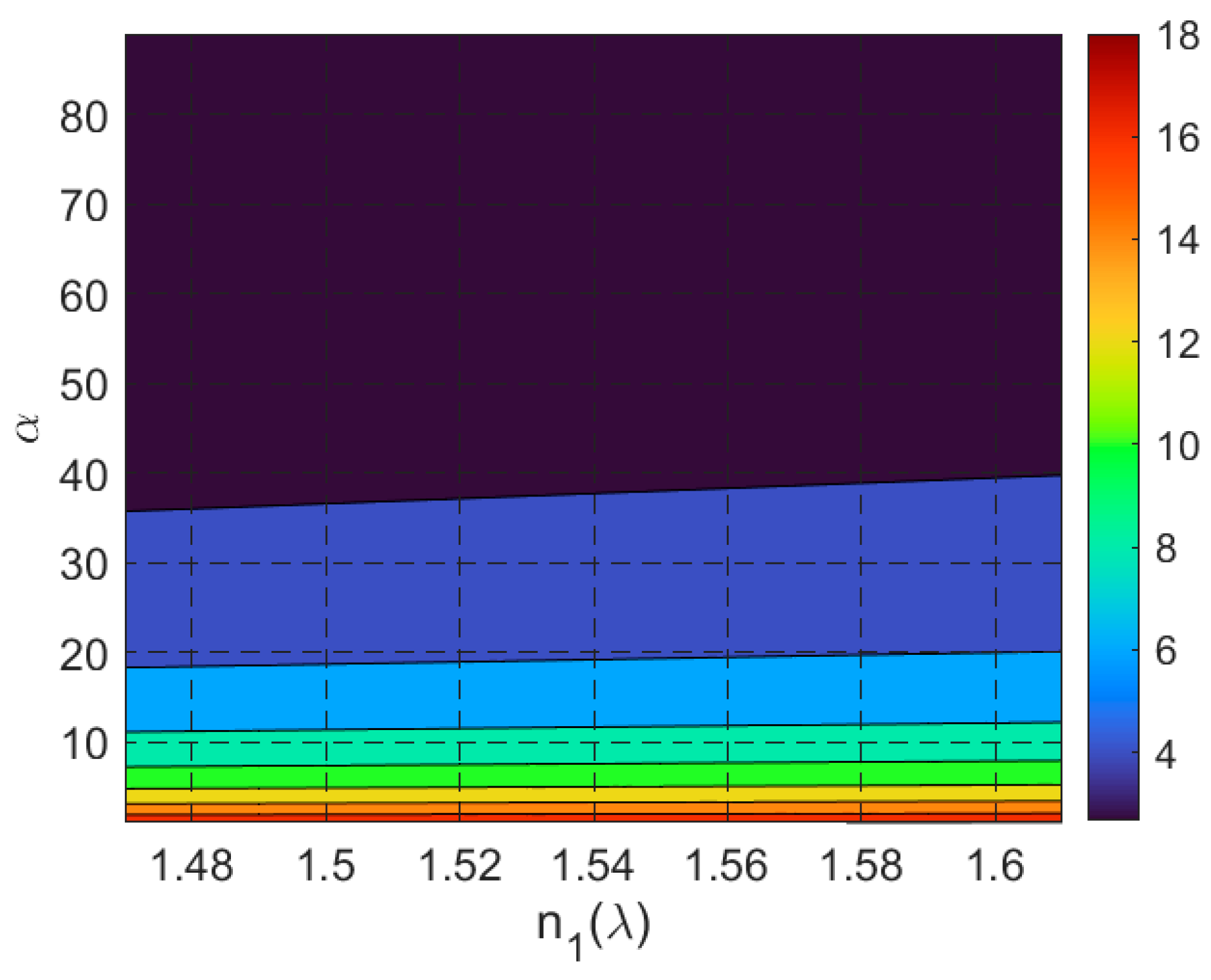
- Proposed Planar Solar Concentrator: The proposed design achieves a GCR of 3.45 with an AA of 23° to 90° (excluding boundaries). This represents a significant improvement in angular range compared to most existing methods, making it highly adaptable to varying solar angles without requiring precise alignment. While its GCR is lower than that of parabolic or Fresnel lens-based systems, its broad AA and simplicity in design and assembly make it a promising solution for practical applications, particularly in environments where solar tracking is impractical or cost-prohibitive.
- wide range of incident angles (23° to 90°), making it suitable for stationary installations and regions with significant seasonal variations in solar elevation.
- Simplified Design and Assembly: The use of identical plane-parallel plates with a fixed inclination angle () and a length-to-thickness ratio of simplifies manufacturing and assembly processes.
- Material Efficiency: The design minimizes the use of optical materials, reducing both cost and weight while maintaining performance.
- Scalability: The modular nature of the design allows for easy scaling by adjusting the number of rows () and cells per row ().

5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| GCR | Geometrical concentration ratio |
| AA | Acceptance angle |
| TIR | Total internal reflection |
References
- Ballif, C.; Haug, F.-J.; Boccard, M.; Verlinden, P.J.; Hahn, G. Status and perspectives of crystalline silicon photovoltaics in research and industry. Nat. Rev. Mater. 2022, 7, 926. [Google Scholar] [CrossRef]
- Sharma, V.K.; Singh, R.; Gehlot, A.; Buddhi, D.; Braccio, S.; Priyadarshi, N.; Khan, B. Imperative Role of Photovoltaic and Concentrating Solar Power Technologies towards Renewable Energy Generation. Int. J. Photoenergy 2022, 13, 3852484. [Google Scholar] [CrossRef]
- Shanks, K.; Senthilarasu, S.; Mallick, T.K. Optics for concentrating photovoltaics: Trends, limits and opportunities for materials and design. Renew. Sustain. Energy Rev. 2016, 60, 394–407. [Google Scholar] [CrossRef]
- Ma, X.; Zheng, H.; Liu, S. A Review on Solar Concentrators with Multi-surface and Multi-element Combinations. J. Daylighting 2019, 6, 80–96. [Google Scholar] [CrossRef]
- Xie, W.; Dai, Y.; Wang, R.; Sumathy, K. Concentrated solar energy applications using Fresnel lenses: A review. Renew. Sustain. Energy Rev. 2011, 15, 2588–2606. [Google Scholar] [CrossRef]
- Khamooshi, M.; Salati, H.; Egelioglu, F.; Hooshyar Faghiri, A.; Tarabishi, J.; Babadi, S. A Review of Solar Photovoltaic Concentrators. Int. J. Photoenergy 2014, 958521, 17. [Google Scholar] [CrossRef]
- Fu, L.; Leutz, R.; Annen, H.P. Secondary optics for Fresnel lens solar concentrators. In Proceedings of the SPIE OPTICAL ENGINEERING + APPLICATIONS, San Diego, CA, USA, 1–5 August 2010. [Google Scholar]
- Soule, D.E. Hybrid Solar Energy Generating System. U.S. Patent 4,700,013, 13 October 1987. [Google Scholar]
- Tremblay, R. Electromagnetic Wave Concentrator. U.S. Patent US4505264A, 27 December 1983. [Google Scholar]
- Miller, O.E.; McLeod, J.H.; Sherwood, W.T. Thin Sheet Plastic Fresnel Lenses of High Aperture. J. Opt. Soc. Am. 1951, 41, 807–814. [Google Scholar] [CrossRef]
- Pociask-Bialy, M. Polyethylene Protective Coating with Anti-Reflective Properties for Silicon Photovoltaic Cells. Materials 2023, 16, 4004. [Google Scholar] [CrossRef]
- Price, H.; Lupfert, E.; Kearney, D.; Zarza, E.; Cohen, G.; Gee, R.; Mahoney, R. Advances in Parabolic Trough Solar Power Technology. J. Sol. Energy Eng. ASME 2002, 124, 109–125. [Google Scholar] [CrossRef]
- Buljan, M.; Mendes-Lopes, J.; Benítez, P.; Miñano, J.C. Recent trends in concentrated photovoltaics concentrators’ architecture. J. Photon. Energy 2014, 4, 040995. [Google Scholar] [CrossRef]
- Weber, K.J.; Everett, V.; Deenapanray, P.N.K.; Franklin, E.; Blakers, A.W. Modeling of static concentrator modules in-corporating lambertian or v-groove rear reflectors. Sol. Energy Mater. Sol. Cells 2006, 90, 1741–1749. [Google Scholar] [CrossRef]
- Yu, Y.; Liu, N.; Li, G.; Tang, R. Performance comparison of CPCs with and without exit angle restriction for concentrating radiation on solar cells. Appl. Energy 2015, 155, 284–293. [Google Scholar] [CrossRef]
- Yu, Y.; Tang, R. Diffuse reflections within CPCs and its effect on energy collection. Sol. Energy 2015, 120, 44–54. [Google Scholar] [CrossRef]
- Tang, R.; Liu, X. Optical performance and design optimization of V-trough concentrators for photovoltaic applications. Sol. Energy 2011, 85, 2154–2166. [Google Scholar] [CrossRef]
- Canavarro, D.; Chaves, J.; Collares-Pereira, M. Manuel New second-stage concentrators (XX SMS) for parabolic primaries; Comparison with conventional parabolic trough concentrators. Sol. Energy 2013, 92, 98–105. [Google Scholar] [CrossRef]
- Murphree, Q. Point focusing double parabolic trough concentrator. Sol. Energy 2001, 70, 85–94. [Google Scholar] [CrossRef]
- Canavarro, D.; Chaves, J.; Collares-Perreira, M. New Optical Designs for Large Parabolic Troughs. Energy Procedia 2014, 49, 1279–1287. [Google Scholar] [CrossRef]
- Collares-Pereira, M.; Gordon, J.; Rabl, A.; Winston, R. High concentration two-stage optics for parabolic trough solar collectors with tubular absorber and large rim angle. Sol. Energy 1991, 47, 457–466. [Google Scholar] [CrossRef]
- El Gharbi, N.; Derbal-Mokrane, H.; Bouaichaoui, S.; Said, N. A comparative study between parabolic trough collector and linear Fresnel reflector technologies. Energy Procedia 2011, 6, 565–572. [Google Scholar] [CrossRef]
- Abbas, R.; Montes, M.J.; Piera, M.; Martínez-Val, J.M. Solar radiation concentration features in Linear Fresnel Reflector arrays. Energy Convers. Manag. 2012, 54, 133–144. [Google Scholar] [CrossRef]
- Abbas, R.; Muñoz-Antón, J.; Valdés, M.; Martinez-Val, J. High concentration linear Fresnel reflectors. Energy Convers. Manag. 2013, 72, 60–68. [Google Scholar] [CrossRef]
- Selvanayagam, B.F.; Krishnamoorthy, S.; Gowtham, S.; Balaji, N. A review of parabolic collector with shell and tube heat exchanger. Mater. Today Proc. 2022, 66, 1382–1388. [Google Scholar] [CrossRef]
- Mills, D.R.; Morrison, G.L. Compact Linear Fresnel Reflector solar thermal powerplants. Sol. Energy 2000, 68, 263–283. [Google Scholar] [CrossRef]
- Omer, S.; Infield, D. Design and thermal analysis of a two stage solar concentrator for combined heat and thermo-electric power generation. Energy Convers. Manag. 2000, 41, 737–756. [Google Scholar] [CrossRef]
- Tang, R.; Wang, J. A note on multiple reflections of radiation within CPCs and its effect on calculations of energy collection. Renew. Energy 2013, 57, 490–496. [Google Scholar] [CrossRef]
- Yu, X.; Su, Y.; Zheng, H.; Riffat, S. A study on use of miniature dielectric compound parabolic concentrator (dCPC) for daylighting control application. Build. Environ. 2011, 74, 75–85. [Google Scholar] [CrossRef]
- Chaoqing, F.; Hongfei, Z.; Rui, W.; Xu, Y.; Yuehong, S. A novel solar multifunctional PV/T/D system for green building roofs. Energy Convers. Manag. 2015, 93, 63–71. [Google Scholar]
- Li, X.; Dai, Y.; Li, Y.; Wang, R. Comparative study on two novel intermediate temperature CPC solar collectors with the U-shape evacuated tubular absorber. Sol. Energy 2013, 93, 220–234. [Google Scholar] [CrossRef]
- Benítez, P.; Miñano, J.C.; Zamora, P.; Mohedano, R.; Cvetkovic, A.; Buljan, M.; Chaves, J.; Hernández, M. High performance Fres-nel-based photovoltaic concentrator. Opt. Express 2010, 26, A25–A40. [Google Scholar] [CrossRef]
- Pham, T.T.; Vu, N.H.; Shin, S. Novel Design of Primary Optical Elements Based on a Linear Fresnel Lens for Concentrator Photovoltaic Technology. Energies 2019, 12, 1209. [Google Scholar] [CrossRef]
- Fabian, L.; Karl, F.; Cédric, L.; Jérôme, L.; Donat, R.; Tanguy, T.; Serge, H. Flat Fresnel doublets made of PMMA and PC: Combining low cost production and very high concentration ratio for CPV. Opt. Express 2011, 19, A280–A294. [Google Scholar]
- Waritanant, T.; Boonruang, S.; Chung, T.-Y. High angular tolerance thin profile solar concentrators designed using a wedge prism and diffraction grating. Sol. Energy 2013, 87, 35–41. [Google Scholar] [CrossRef]
- Hughes, M.; Maher, C.; Borca-Tasciuc, D.A.; Polanco, D.; Kaminski, D. Performance comparison of wedge-shaped and planar luminescent solar concentrators. Renew. Energy 2013, 52, 266–272. [Google Scholar] [CrossRef]
- Lin, H.-H.; Yang, W.-H. Light Concentration Module. U.S. Patent US20140196785A1, 24 October 2013. [Google Scholar]
- Ramachandran, A.M.; Thampi, A.S.; Singh, M.; Asok, A. A comprehensive review on optics and optical materials for planar waveguide-based compact concentrated solar photovoltaics. Results Eng. 2022, 16, 100665. [Google Scholar] [CrossRef]
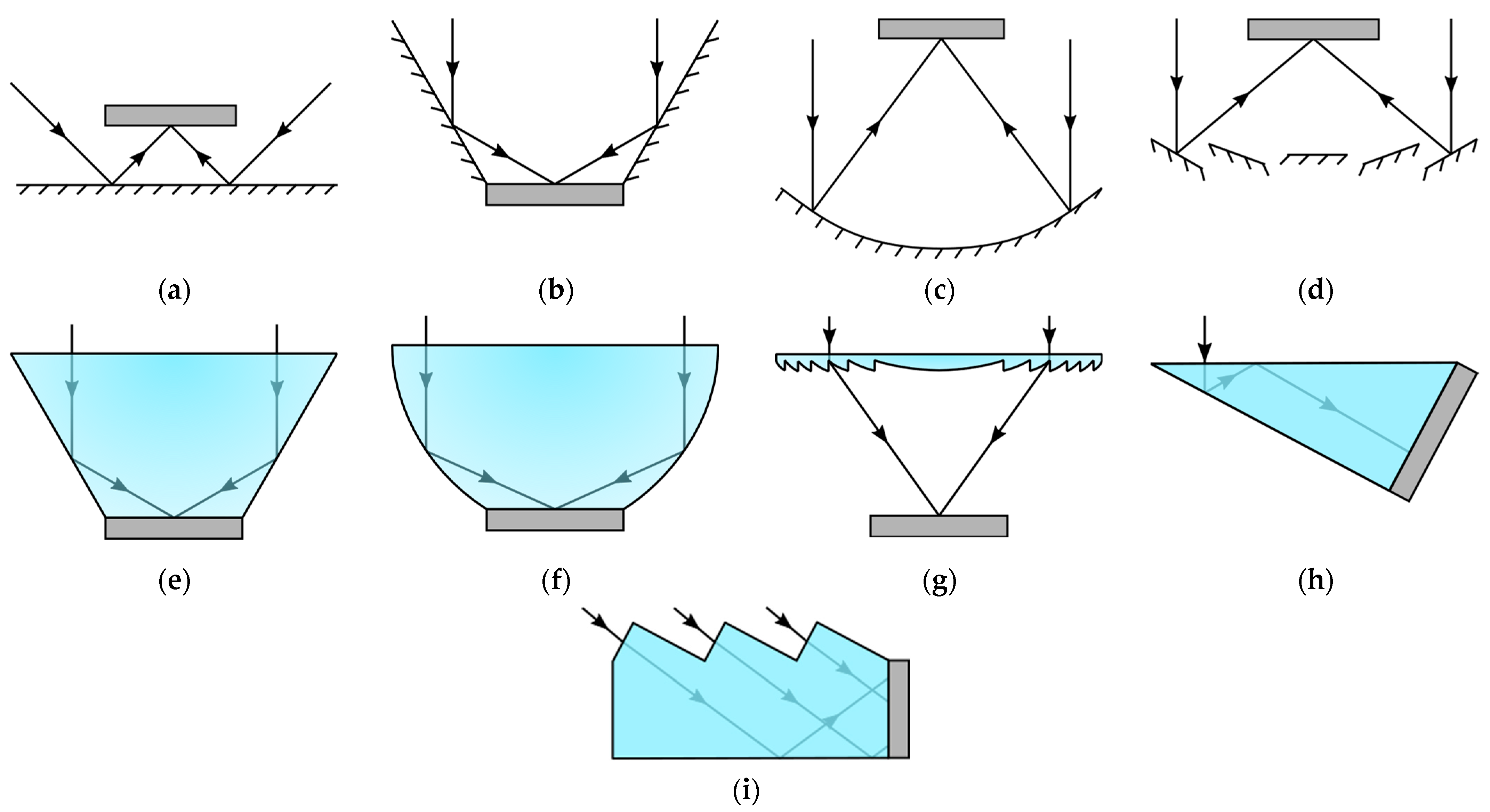



| Type | Operation Mechanism | GCR (arb. Units) | AA (°) |
|---|---|---|---|
| Flat reflector | Reflective coating | 2 | 1 |
| V-trough | Reflective coating | 2 | 20 |
| Parabolic dish/trough | Reflective coating | 70 | 0.26 |
| Linear Fresnel reflector | Reflective coating | 6–15 | 1 |
| Light funnel/ homogenizer | Refractive and Total internal reflection (TIR) | 1.5 | 20 |
| Compound parabolic concentrator | Refractive and TIR | 4 | 20 |
| Fresnel lens | Refractive | 1000 | 1.3 |
| Wedge prism | Refractive and TIR or reflective coating | 3.77 | 50 |
| Wedge prism with planar waveguide | Refractive and TIR or reflective coating | – | 45 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Stsepuro, N.; Kovalev, M.; Podlesnykh, I.; Kudryashov, S. A New Approach to Designing Multi-Element Planar Solar Concentrators: Geometry Optimization for High Angular Selectivity and Efficient Solar Energy Collection. Optics 2025, 6, 6. https://doi.org/10.3390/opt6010006
Stsepuro N, Kovalev M, Podlesnykh I, Kudryashov S. A New Approach to Designing Multi-Element Planar Solar Concentrators: Geometry Optimization for High Angular Selectivity and Efficient Solar Energy Collection. Optics. 2025; 6(1):6. https://doi.org/10.3390/opt6010006
Chicago/Turabian StyleStsepuro, Nikita, Michael Kovalev, Ivan Podlesnykh, and Sergey Kudryashov. 2025. "A New Approach to Designing Multi-Element Planar Solar Concentrators: Geometry Optimization for High Angular Selectivity and Efficient Solar Energy Collection" Optics 6, no. 1: 6. https://doi.org/10.3390/opt6010006
APA StyleStsepuro, N., Kovalev, M., Podlesnykh, I., & Kudryashov, S. (2025). A New Approach to Designing Multi-Element Planar Solar Concentrators: Geometry Optimization for High Angular Selectivity and Efficient Solar Energy Collection. Optics, 6(1), 6. https://doi.org/10.3390/opt6010006








