Application of Hardening State Parameter Constitutive Model for Prediction of Overconsolidated Soft Clay Behavior Due to Embankment Loading
Abstract
:1. Introduction
2. Objectives of the Study
3. HASP Constitutive Soil Model
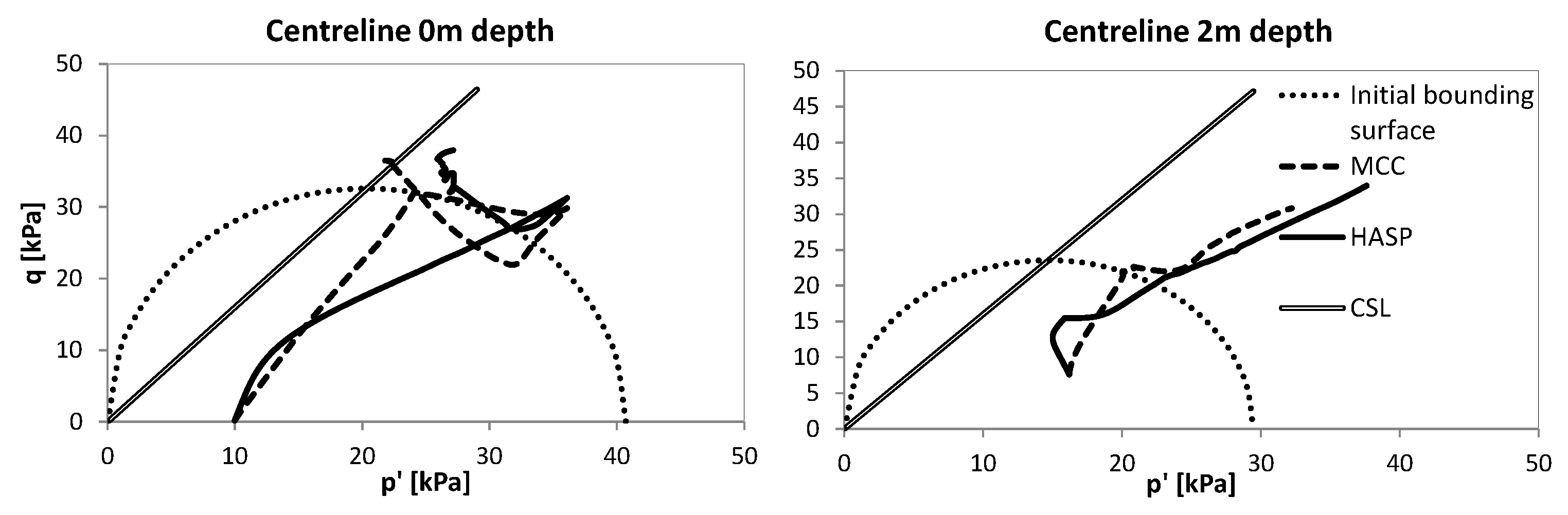
4. Comparison of HASP and MCC Models
5. Finite Element Modelling of the Two Embankments
5.1. Saga Highway Embankment
5.2. Teven Road Trial Embankment
5.3. Numerical Models
5.4. Material Model Parameters
6. Results and Discussion
6.1. Embankment A
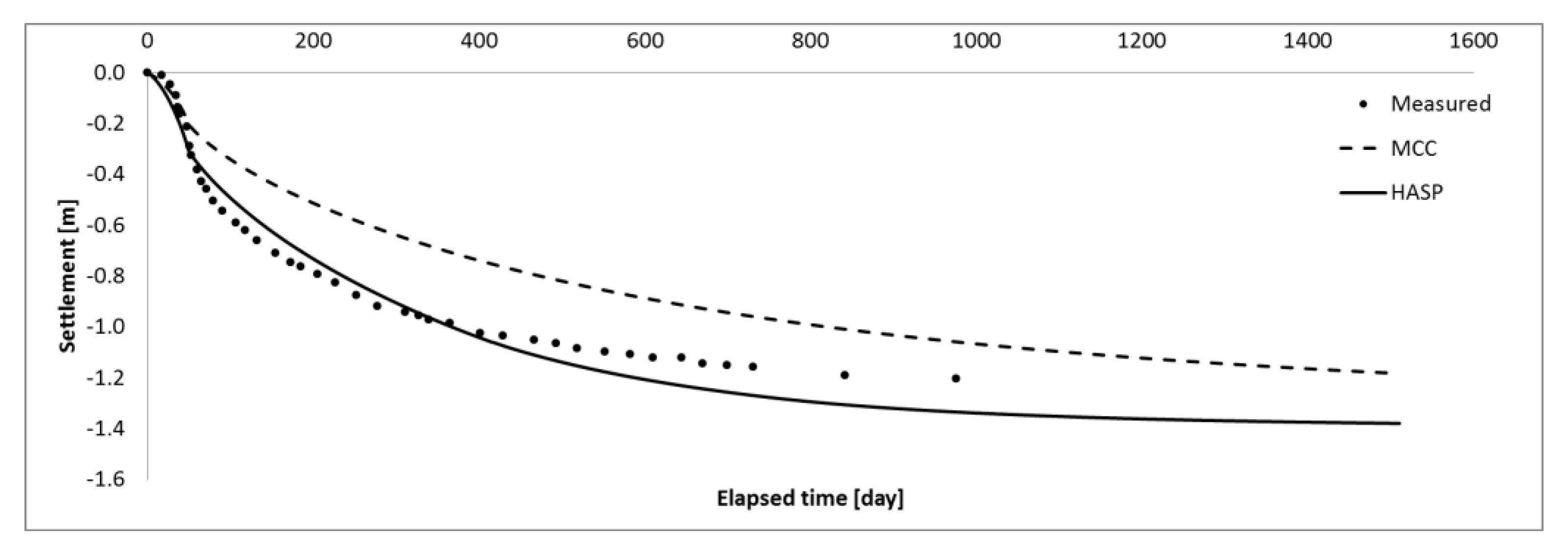
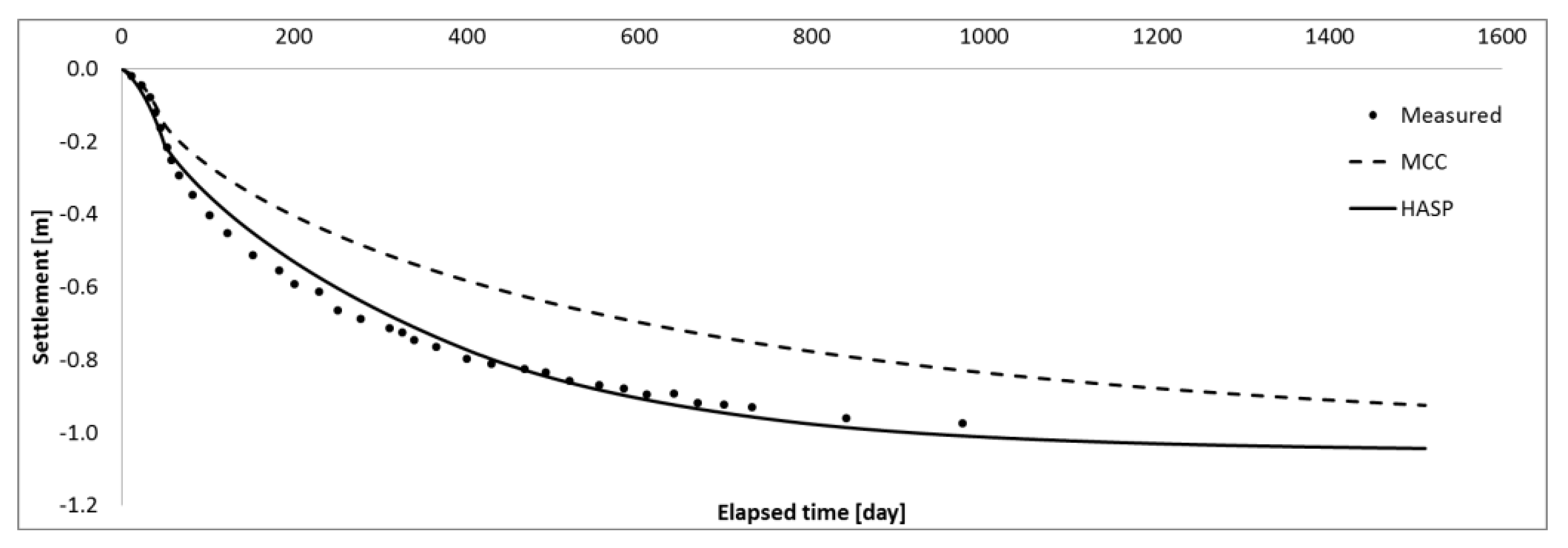
6.1.1. Settlement Predictions
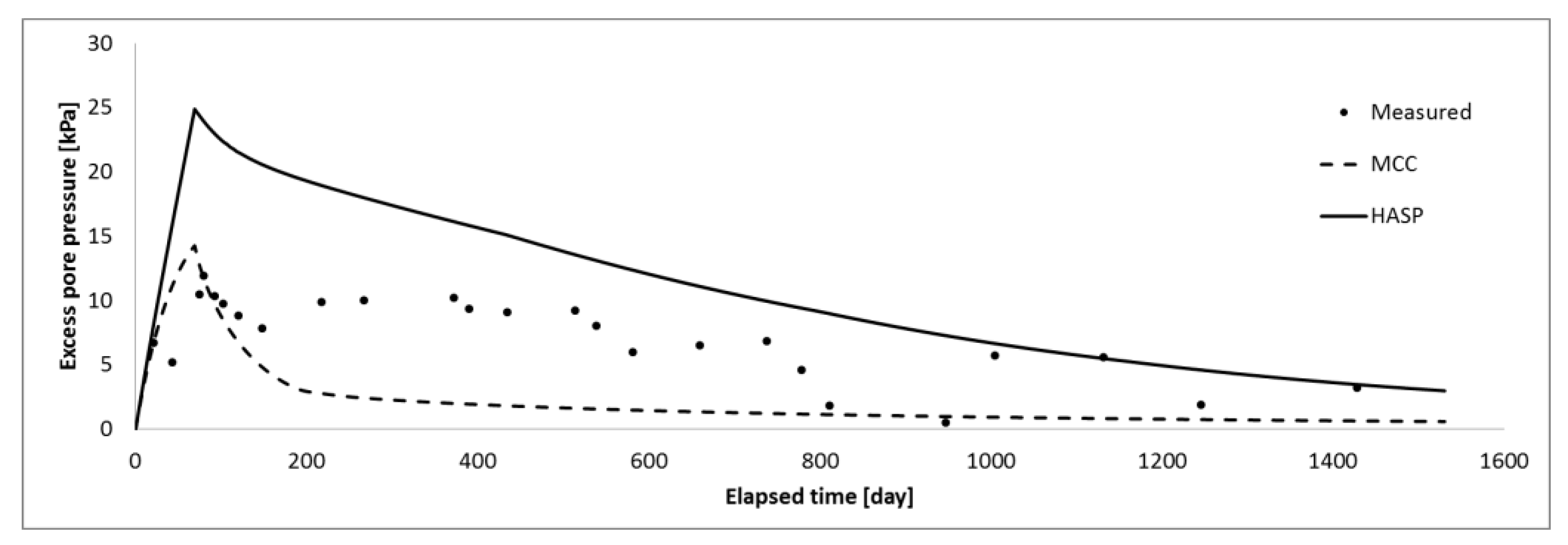
6.1.2. Excess Pore Pressure Predictions
6.2. Embankment B
6.2.1. Settlement Predictions
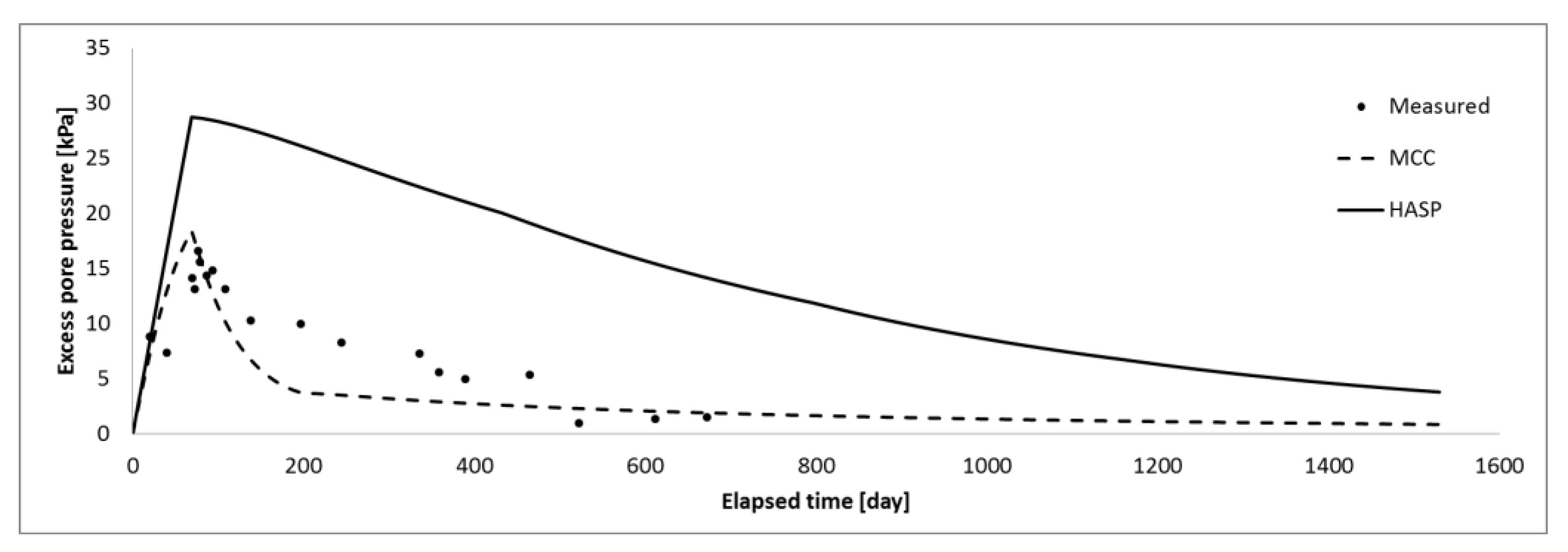
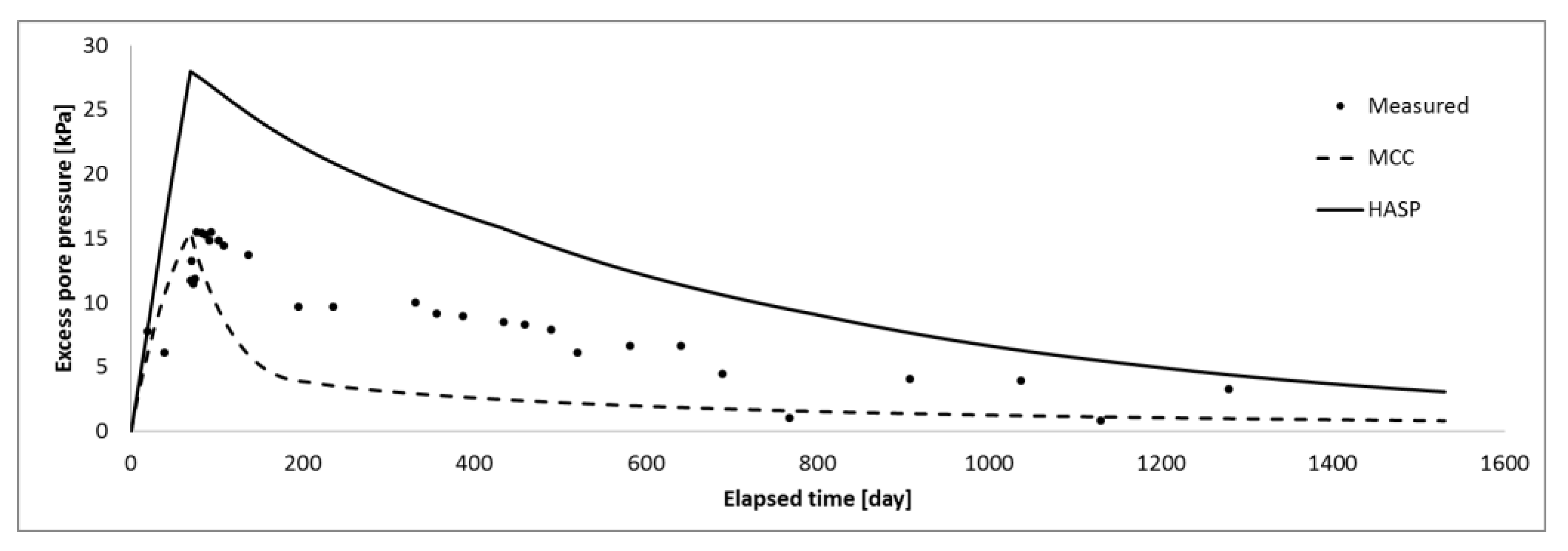
6.2.2. Excess Pore Pressure Predictions
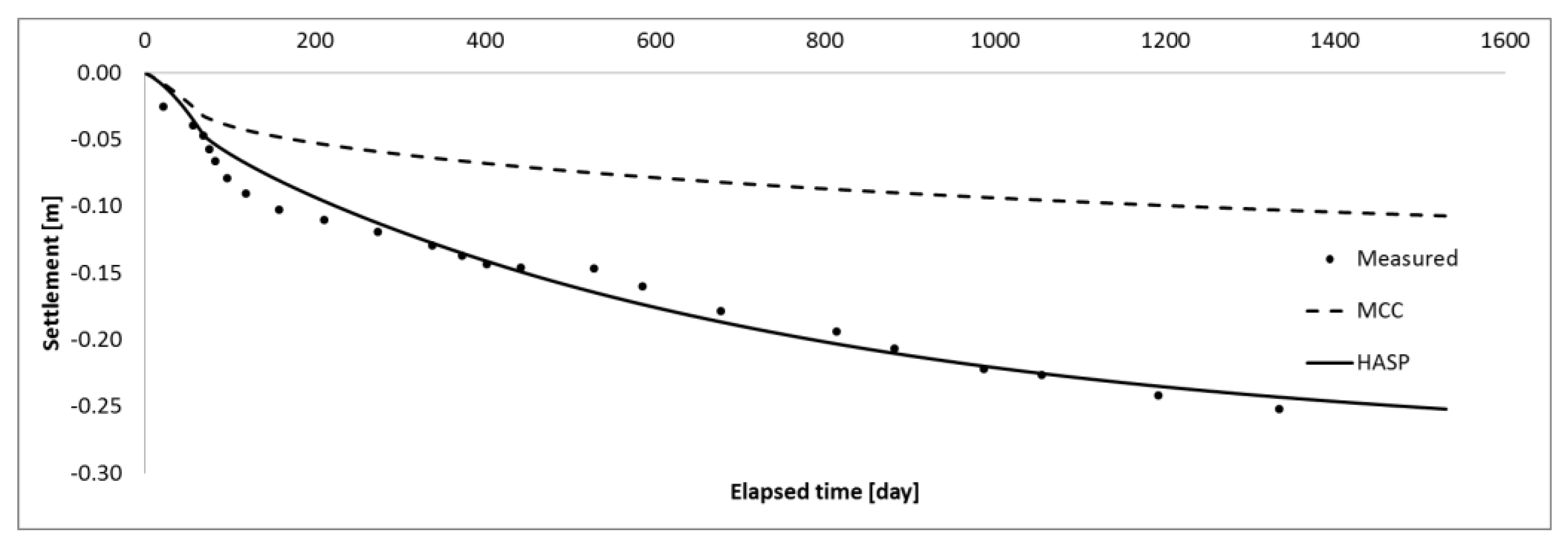
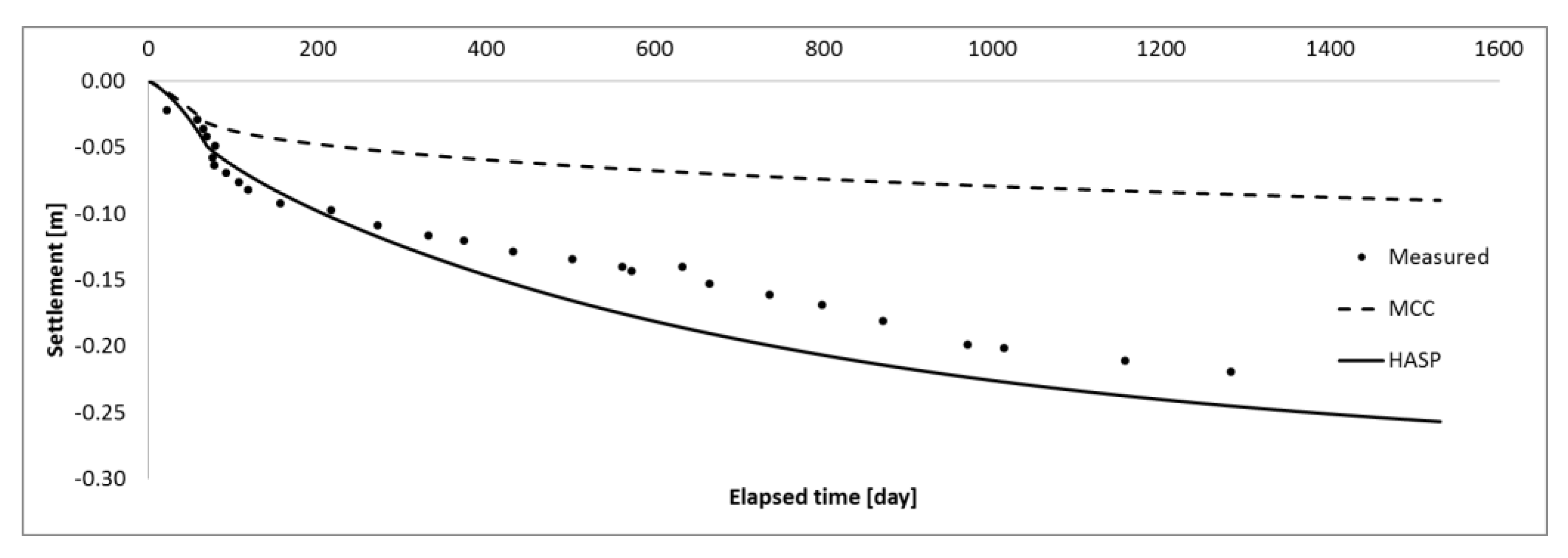
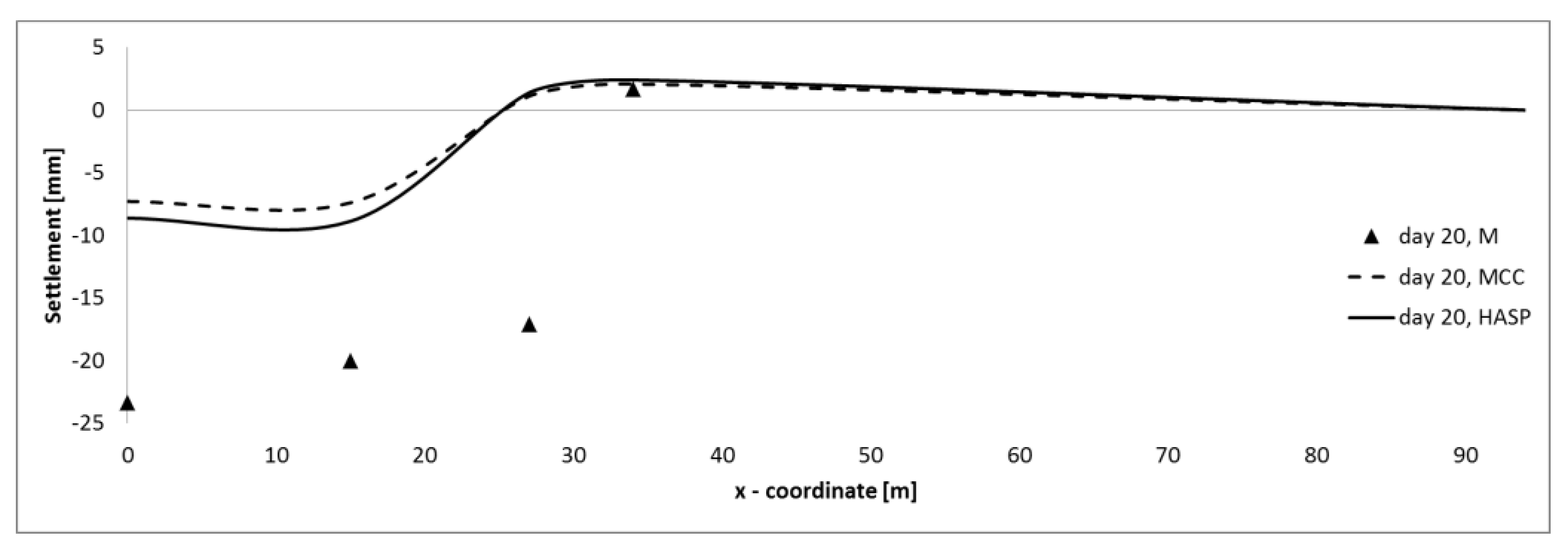
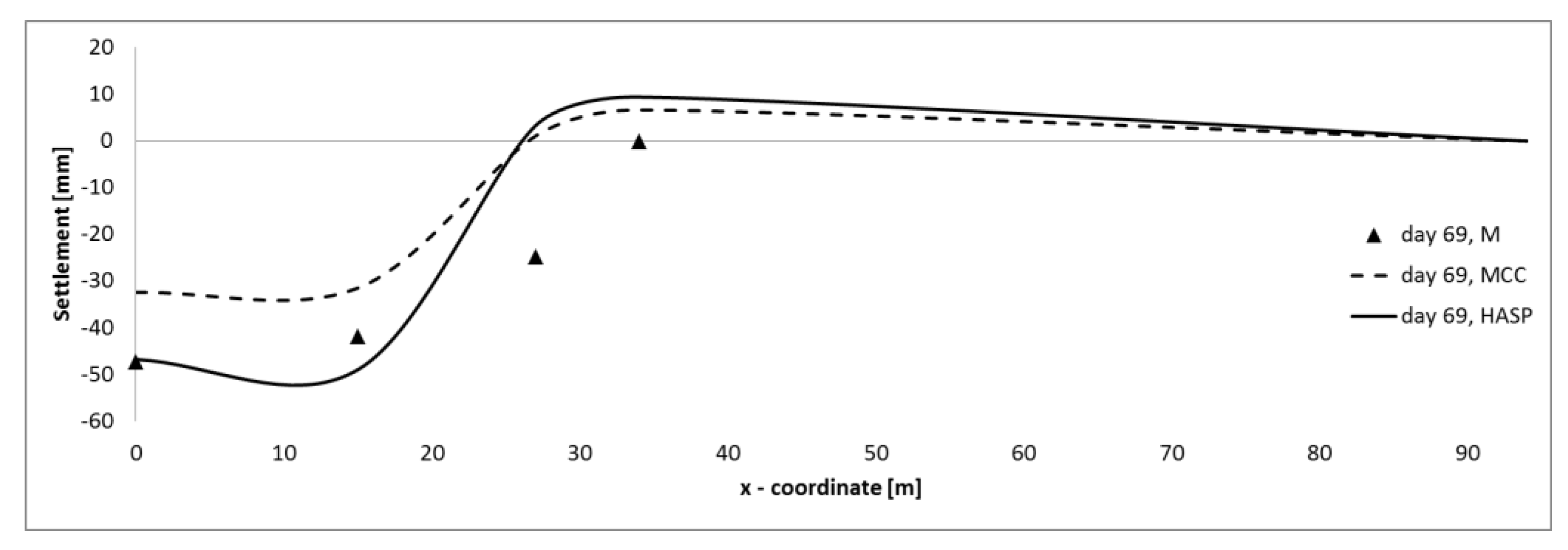
6.2.3. Settlement of the Ground Surface
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Notation
| constant | |
| dilatancy | |
| det | determinant |
| increment of plastic shear strain | |
| increment of plastic volumetric strain | |
| dilatancy at peak stress ratio | |
| void ratio | |
| initial void ratio | |
| hydraulic conductivity of soil in horizontal direction | |
| initial value of hydraulic conductivity of soil | |
| hydraulic conductivity of soil in vertical direction | |
| mean effective stress on yield surface | |
| mean effective stress on bounding surface | |
| size of yield surface—hardening parameter | |
| size of bounding surface—hardening parameter | |
| triaxial deviatoric stress on yield surface | |
| triaxial deviatoric stress on bounding surface | |
| elastic shear modulus | |
| second invariant of the deviatoric stress tensor | |
| third invariant of the deviatoric stress tensor | |
| elastic bulk modulus | |
| coefficient of at-rest earth pressure for normally consolidated soil | |
| coefficient of at-rest earth pressure for overconsolidated soil | |
| gradient of critical state line in plane | |
| gradient of critical state line in plane in triaxial compression | |
| maximum stress ratio | |
| gradient of critical state line in plane in triaxial extension | |
| reference specific volume for on virgin compression line | |
| OCR | overconsolidation ratio |
| POP | preoverburden pressure |
| overconsolidation ratio | |
| X, Y, Z | constants |
| stress ratio | |
| Lode’s angle | |
| slope of an swelling line (URL) in plane | |
| slope of the virgin compression line (VCL) in plane | |
| Poisson’s ratio | |
| specific volume | |
| parameter in hardening rule which controls the influence of plastic shear strains on hardening parameter | |
| friction angle in triaxial compression | |
| friction angle in triaxial extension | |
| hardening coefficient | |
| reference specific volume for on critical state line | |
| state parameter for current stress point | |
| state parameter for conjugate stress point |
References
- Rezania, M.; Ngujen, H.; Zanganeh, H.; Taiebat, M. Numerical analysis of Ballina test embankment on a soft structured clay foundation. Comput. Geotech. 2018, 93, 61–74. [Google Scholar] [CrossRef]
- Ming, D.; Lin, G.; Mingfeng, L.; Tian, J. The pore pressure generation and deformation of overconsolidated soft marine clay considering initial static shear effect. Mar. Georesour. Geotechnol. 2022, 40, 922–935. [Google Scholar] [CrossRef]
- Parry, R. Overconsolidation in soft clay deposits. Geotechnique 1970, 20, 442–446. [Google Scholar] [CrossRef]
- Zdravković, L.; Potts, D.M.; Bodas Freitas, T. Extending the life of existing infrastructure. In Proceedings of the XVII ECSMGE-2019, Reykjavik, Iceland, 1–6 September 2019. [Google Scholar] [CrossRef]
- Leroueil, S.; Magnan, J.; Tevenas, F. Embankments on Soft Clays, 1st ed.; Ellis Horwood: New York, NY, USA, 1990; 360p. [Google Scholar]
- Chai, J.; Igaya, Y.; Hino, T.; Carter, J. Finite element simulation of an embankment on soft clay—Case Study. Comput. Geotech. 2012, 48, 117–126. [Google Scholar] [CrossRef]
- Roscoe, K.H.; Burland, J.B. On the generalized stress-strain behavior of wet clay. In Engineering Plasticity; Cambridge University Press: Cambridge, UK, 1968; pp. 535–609. [Google Scholar]
- Huang, W.; Fityus, S.; Bishop, D.; Smith, D.; Sheng, D. Finite-element parametric study of the consolidation behaviour of a trial embankment on soft clay. Int. J. Geomech. 2006, 6, 328–341. [Google Scholar] [CrossRef]
- Karstunen, M.; Wiltafsky, C.; Krenn, H.; Scharinger, F.; Schweiger, H.F. Modelling the behavior of an embankment on soft clay with different constitutive models. Int. J. Numer. Anal. Methods Geomech. 2006, 30, 953–982. [Google Scholar] [CrossRef]
- Karstunen, M.; Krenn, H.; Wheeler, S.J.; Koskinen, M. Effect of anisotropy and destructuration on the behavior of Murro test embankment. Int. J. Geomech. 2005, 5, 87–97. [Google Scholar] [CrossRef]
- Naatanen, A.; Vepsalainen, P.; Lojander, M. Finite element calculations on Haarajoki test embankment. In Proceedings of the Fourth European Conference on Numerical Methods in Geotechnical Engineering Numge98, Udine, Italy, 14–16 October 1998. [Google Scholar]
- Tashiro, M.; Noda, T.; Inagaki, M.; Nakano, M.; Asaoka, A. Prediction of settlement in natural deposited clay ground with risk of large residual settlement due to embankment loading. Soils Found. 2011, 51, 133–149. [Google Scholar] [CrossRef]
- Zdravković, L.; Potts, D.M.; Hight, D.W. The effect of strength anisotropy on the behavior of embankments on soft ground. Geotechnique 2002, 52, 447–457. [Google Scholar] [CrossRef]
- Venda Oliveira, P.J.; Lemos, L.J.L.; Coelho, P. ALF. Behavior of an atypical embankment on soft soil: Field observations and numerical simulation. J. Geotech. Geoenviron. Eng. ASCE 2010, 136, 35–47. [Google Scholar] [CrossRef]
- Kim, J.; Yun, S.K.; Im, E.S.; Kang, G. A case study for the behavior of consolidated settlement considering overconsolidated layer. KSCE J. Civ. Eng. 2022, 26, 4302–4316. [Google Scholar] [CrossRef]
- Zhiwei, G.; Zhao, J.; Yin, Z.Y. Dilatancy relation for overconsolidated clay. Int. J. Geomech. 2017, 17, 793–799. [Google Scholar] [CrossRef]
- Gallikova, Z.; Rehman, Z. Appraisal of the hypoplastic model for the numerical prediction of high-rise building settlement in Neogene clay based on real-scale monitoring data. J. Build. Eng. 2022, 50, 104152. [Google Scholar] [CrossRef]
- Vesterberg, B.; Andersson, M. Settlement and pore pressure behavior and predictions of test embankments on an organic clay. J. Geotech. Eng. 2022, 16, 1049–1067. [Google Scholar] [CrossRef]
- Karstunen, M.; Amavasai, A. Best Soil: Soft Soil Modelling and Parameter Determination, Research Report for Big Project A2015-06; Chalmers University of Technology: Gothenburg, Sweden, 2017; p. 84. [Google Scholar]
- Nallathamby, S.; Rezania, M. The comparison of modelling inherent and evolving anisotropy on the behavior of a full-scale embankment. J. Geotech. Eng. 2017, 11, 343–354. [Google Scholar] [CrossRef]
- Venda Oliveira, P.J.; Lemos, L.J.L. Numerical predictions of the behavior of soft clay with two anisotropic elastoplastic models. Comput. Geotech. 2011, 38, 598–611. [Google Scholar] [CrossRef]
- Whittle, A.; Kavvadas, M. Formulation of MIT-E3 constitutive model for overconsolidated clays. J. Geotech. Eng. 1994, 120, 173–198. [Google Scholar] [CrossRef]
- Venda Oliveira, P.J.; Araujo Santos, L.M.; Almeida e Sousa, J.N.V.; Lemos, L.J.L. Effect of initial stiffness on the behaviour of two geotechnical structures: An embankment and a tunnel. Comput. Geotech. 2021, 136, 104181. [Google Scholar] [CrossRef]
- Potts, D.L.; Zdravković, L. Finite Element Analysis in Geotechnical Engineering—Theory, 1st ed.; Thomas Telford Publishing: London, UK, 1999; 440p. [Google Scholar]
- Grammatikopoulou, A.L.; Zdravković, L.; Potts, D.M. The effect of the yield and plastic potential deviatoric surfaces on the failure height of an embankment. Geotechnique 2007, 57, 795–806. [Google Scholar] [CrossRef]
- Freitas, T.; Bodas, M.; Potts, D.M.; Zdravković, L. A time dependent constitutive model for soil with isotach viscosity. Comput. Geotech. 2011, 38, 809–820. [Google Scholar] [CrossRef]
- Jocković, S.; Vukićević, M. Bounding surface model for overconsolidated clays with new state parameter formulation of hardening rule. Comput. Geotech. 2017, 83, 16–29. [Google Scholar] [CrossRef]
- Been, K.; Jefferies, M.G. A state parameter for sands. Geotechnique 1982, 35, 99–112. [Google Scholar] [CrossRef]
- Jocković, S.; Vukićević, M. Critical state constitutive model for overconsolidated clays—HASP model. In Proceedings of the XVI Danube—European Conference on Geotechnical Engineering, Skopje, North Macedonia, 7–9 June 2018. [Google Scholar]
- Jocković, S.; Vukićević, M. Validation and implementation of HASP constitutive model for overconsolidated clays. Build. Mater. Struct. 2018, 61, 91–109. [Google Scholar] [CrossRef]
- Potts, D.M.; Zdravković, L. Some Pitfalls when Using Modified Cam Clay; Imperial College: London, UK, 2000; pp. 14p. [Google Scholar]
- Van Eekelen, H.A.M. Isotropic yield surfaces in three dimensions for use in soil mechanics. Int. J. Numer. Anal. Methods Geomechani. 1980, 4, 89–101. [Google Scholar] [CrossRef]
- Zdravković, L.; Taborda, D.; Potts, D.M.; Abadias, D.; Burd, H.; Byrne, B.; Gavin, K.; Houlsby, G.; Jardine, R.; Martin, C.; et al. Finite-element modelling of laterally loaded piles in a stiff glacial clay till at Cowden. Geotechnique 2020, 70, 999–1013. [Google Scholar] [CrossRef]
- Nova, R.; Wood, D.M. A constitutive model for sand in triaxial compression. Int. J. Numer. Anal. Methods Geomechani. 1979, 3, 255–278. [Google Scholar] [CrossRef]
- Yao, Y.P.; Hou, W.; Zhou, A.N. UH model:three-dimensional unified hardening model for overconsolidated clays. Geotechnique 2009, 59, 451–469. [Google Scholar] [CrossRef]
- PLAXIS Manuals. A Constitutive Model for Overconsolidated Clay Based on the Hardening State Parameter. Available online: https://communities.bentley.com/products/geotech-analysis/w/wiki/46246/oc-clay---plaxis-udsm (accessed on 13 January 2023).
- Kojić, M.; Slavković, R.; Grujić, N.; Vukićević, M. Implicit stress integration algorithm for Modified Cam Clay material. Theoretical and Appl. Mech. 1994, 20, 95–119. [Google Scholar]
- Kojić, M.; Bathe, K.J. Inelastic Analysis of Solids and Structures, 1st ed.; Springer-Verlag: Berlin/Heidelberg, Germany, 2003; 414p. [Google Scholar]
- Schofield, A.; Wroth, P. Critical State Soil Mechanics, 1st ed.; Cambridge University: Cambridge, UK, 1968; pp. 228p. [Google Scholar]
- PLAXIS Manuals. Available online: https://communities.bentley.com/products/geotech-analysis/w/wiki/46137/manuals---plaxis (accessed on 13 January 2023).
- Zdravković, L.; Potts, D.M.; Jackons, C. Numerical Study of the effect of preloading on undrained bearing capacity. Int. J. Geomech. 2013, 3, 1–10. [Google Scholar] [CrossRef]
- Chai, J.-C.; Miura, N. Investigation on some factors affecting vertical drain behavior. J. Geotech. Geoenvironmental Eng. ASCE 1999, 125, 216–226. [Google Scholar] [CrossRef]
- Tavenas, F.; Tremblay, M.; Larouche, G.; Lerouei, I.S. In situ measurement of permeability in soft clays. In Proceedings of the ASCE Special Conference on Use of In-Situ Test in Geotechnical Engineering, Blacksburg, VA, USA; 1986. [Google Scholar]
- Jaky, J. The coefficient of earth pressure at rest. J. Soc. Hung. Arch. Eng. 1944, 78, 355–358. [Google Scholar]
- Wood, D.M. Soil Behavior and Critical State Soil Mechanics, 1st ed.; Press Syndicate of the University of Cambridge: Cambridge, UK, 1990; 461p. [Google Scholar]


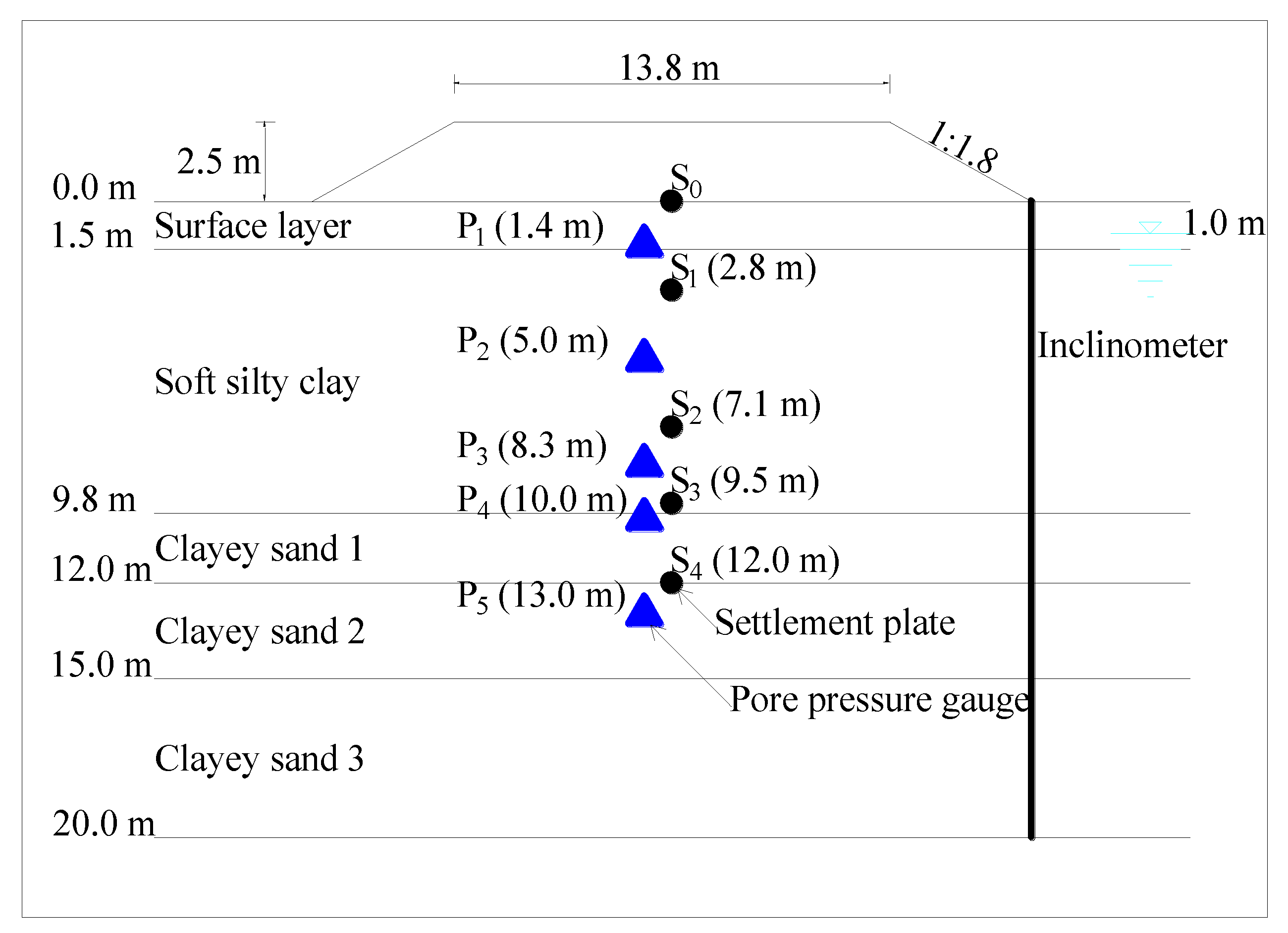
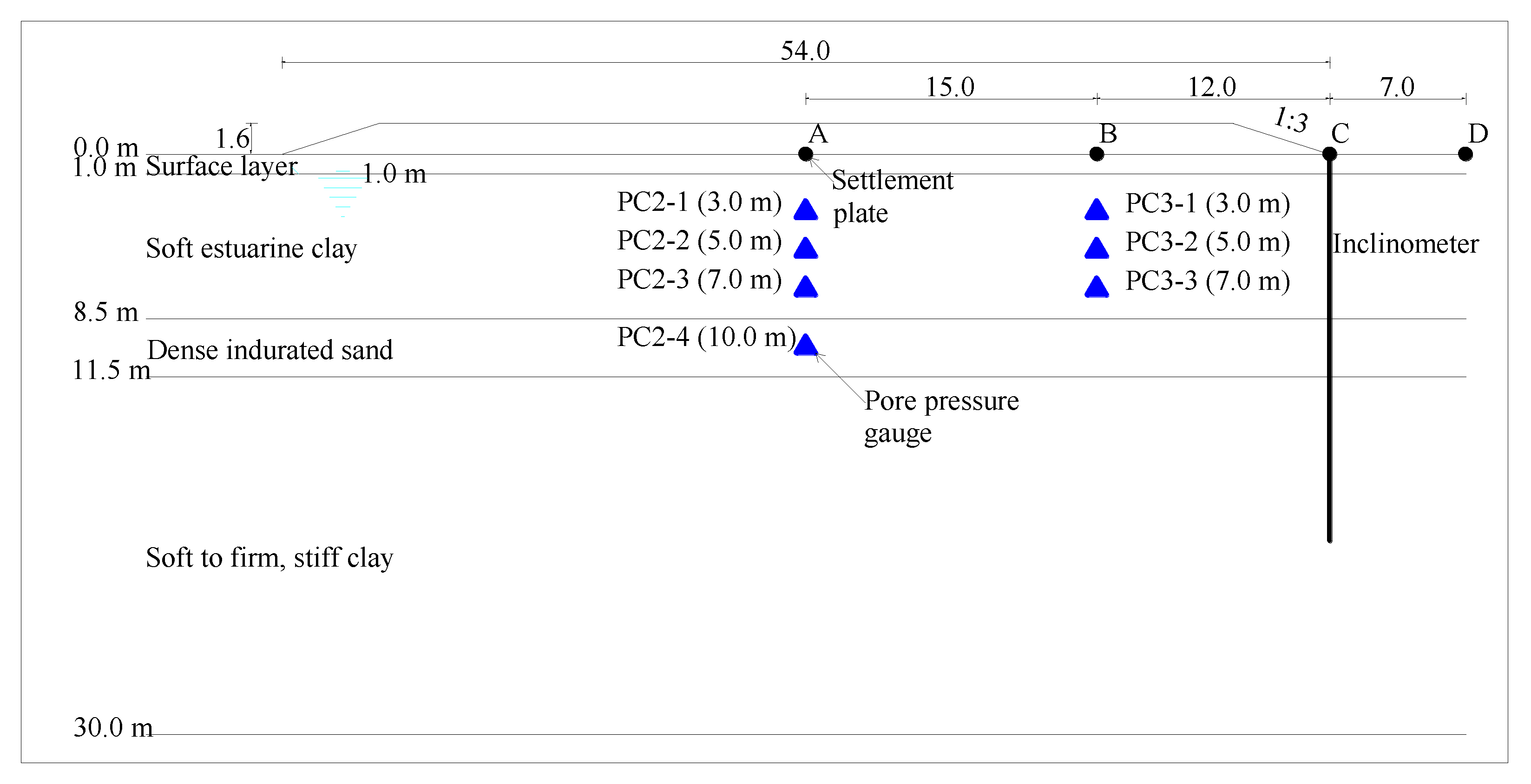
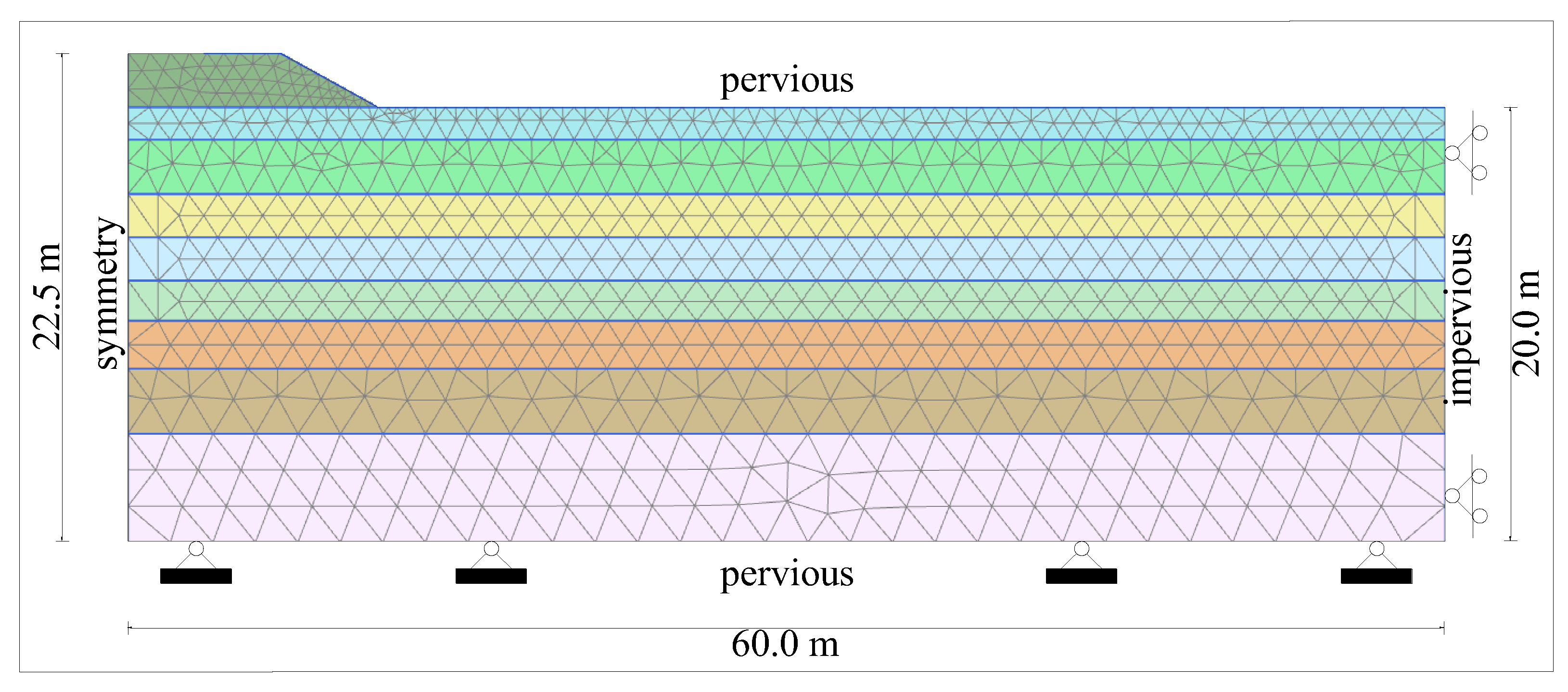




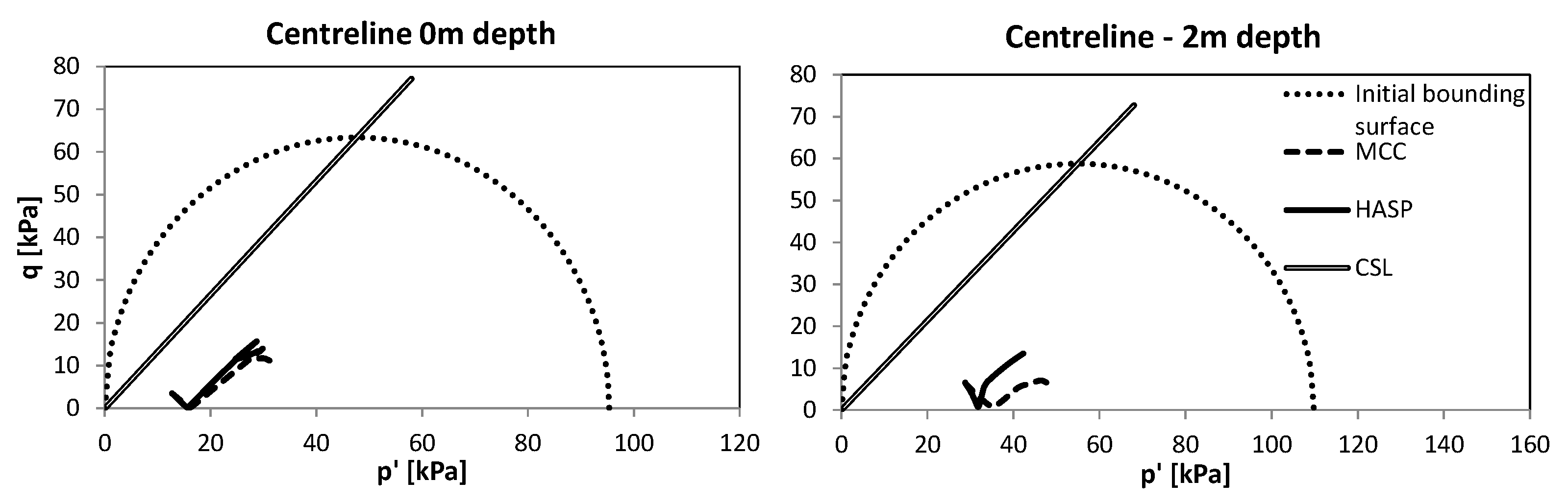



| Soil Strata | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| 0.0–1.5 | Surface clay layer | 16.0 | 0.15 | 1.50 | 40 | 0.51 | 1.48 | 6 | 9.1 | 0.6 |
| 1.5–4.0 | Soft silty clay | 13.1 | 0.15 | 3.71 | 15 | 0.51 | 0.73 | 10.4 | 15.6 | 1.48 |
| 4.0–6.0 | 19.9 | 2.88 | 10 | 0.62 | 17.3 | 25.9 | 1.15 | |||
| 6.0–8.0 | 14.1 | 2.72 | 5 | 0.55 | 16.5 | 24.7 | 1.09 | |||
| 8.0–9.8 | 15.7 | 1.91 | 10 | 0.58 | 7 | 10.6 | 0.76 | |||
| 9.8–12.0 | Clayey Sand | 18.0 | 0.15 | 0.80 | 0 | 0.43 | 2500 | 2500 | 0.32 | |
| 12.0–15.0 | 18.0 | 0.80 | 2500 | 2500 | 0.32 | |||||
| 15.0–20.0 | 19.0 | 0.70 | 2500 | 2500 | 0.32 | |||||
| Embankment | 19.0 | 0.30 | 0.80 | 0 | 0.43 | very high | - | |||
| Soil Strata | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| 0.0–1.0 | Surface clay layer | 18.94 | 0.3 | 0.80 | 101 | 0.45 | 1.41 | 60.48 | 120.96 | 0.32 |
| 1.0–2.5 | Soft clay | 15.08 | 0.33 | 2.29 | 98 | 0.55 | 1.16 | 1.43 | 2.86 | 0.92 |
| 2.5–3.5 | 41 | 0.81 | ||||||||
| 3.5–8.5 | 27 | 0.68 | ||||||||
| 8.5–11.5 | Dense sand | 19.8 | 0.28 | 0.65 | 0 | 0.50 | 864 | 1728 | 0.26 | |
| 11.5–15.0 | Firm Clay | 15.99 | 0.30 | 1.69 | 6 | 0.53 | 0.55 | 0.6 | 1.2 | 0.68 |
| 15.0–20.0 | 4 | 0.54 | ||||||||
| 20.0–30.0 | 0 | 0.53 | ||||||||
| Embankment | 18.0 | 0.30 | 0.5 | 0 | 0.53 | very high | - | |||
| Soil Strata | MCC Parameters | HASP Parameters | ||||
|---|---|---|---|---|---|---|
| 0.0–1.5 | Surface clay layer | 0.025 | 0.25 | 1.6 | 39.17 | |
| 1.5–4.0 | Soft silty clay | 0.107 | 1.07 | 1.6 | 39.17 | |
| 4.0–6.0 | 0.119 | 1.19 | ||||
| 6.0–8.0 | 0.084 | 0.84 | ||||
| 8.0–9.8 | 0.066 | 0.66 | ||||
| 9.8–12.0 | Clayey sand | MC parameters: | ||||
| 12.0–15.0 | MC parameters: | |||||
| 15.0–20.0 | MC parameters: | |||||
| Embankment | MC parameters: | |||||
| Soil Strata | MCC Parameters | HASP Parameters | ||||
|---|---|---|---|---|---|---|
| 0.0–1.0 | Surface clay layer | 0.011 | 0.078 | 1.33 | 33 | 40.29 |
| 1.0–2.5 | Soft clay | 0.02 | 0.587 | 1.07 | 27 | 29.95 |
| 2.5–3.5 | ||||||
| 3.5–8.5 | ||||||
| 8.5–11.5 | Dense sand | MC parameters: | ||||
| 11.5–15.0 | Firm clay | 0.009 | 0.134 | 1.11 | 28 | 31.41 |
| 15.0–20.0 | ||||||
| 20.0–30.0 | ||||||
| Embankment | MC parameters: | |||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Obradović, N.; Jocković, S.; Vukićević, M. Application of Hardening State Parameter Constitutive Model for Prediction of Overconsolidated Soft Clay Behavior Due to Embankment Loading. Appl. Sci. 2023, 13, 2175. https://doi.org/10.3390/app13042175
Obradović N, Jocković S, Vukićević M. Application of Hardening State Parameter Constitutive Model for Prediction of Overconsolidated Soft Clay Behavior Due to Embankment Loading. Applied Sciences. 2023; 13(4):2175. https://doi.org/10.3390/app13042175
Chicago/Turabian StyleObradović, Nikola, Sanja Jocković, and Mirjana Vukićević. 2023. "Application of Hardening State Parameter Constitutive Model for Prediction of Overconsolidated Soft Clay Behavior Due to Embankment Loading" Applied Sciences 13, no. 4: 2175. https://doi.org/10.3390/app13042175
APA StyleObradović, N., Jocković, S., & Vukićević, M. (2023). Application of Hardening State Parameter Constitutive Model for Prediction of Overconsolidated Soft Clay Behavior Due to Embankment Loading. Applied Sciences, 13(4), 2175. https://doi.org/10.3390/app13042175







