Self-Oscillating Converter Based on Phase Tracking Closed Loop for a Dynamic IPT System

Abstract

The coupling of converters with resonant networks poses significant challenges for frequency tracking and power control in inductive power transfer (IPT) systems. This paper presents an implementation method that addresses these issues by dividing the system’s operation into two distinct states: self-oscillating and power-injecting. Based on these states, a phase-closed loop is constructed. Within this closed loop, the phase tracking unit detects and tracks frequency drift, while the power regulating unit incorporates an integrator and adopts a control variable to adjust the output power by modifying the duration of the power injecting state. Meanwhile, the oscillating unit operates in the self-oscillating state. Operating in this manner, the system achieves self-oscillation and demonstrates the capability to effectively track and compensate for system variations within a single cycle. A verification prototype has been constructed, and it demonstrates that the converter within it completely decoupled from the resonant network. Experimental results validate that altering the control variable solely affects the duration of the power-injecting state, allowing for independent control of the output power. When the control variable changes from 2.0 V to 3.5 V, the output power changes from 178 W to 519 W while the self-oscillating state remains unchanged. Furthermore, the system accurately tracks frequency changes, even under significant variations in the coupling coefficient or load, without compromising the power injection state. When the air gap changes from 3 cm to 12 cm, the duration of the self-oscillating state changes from 22.1 μs to 26.3 μs, while the power injecting state remains unchanged. This approach exhibits a robust performance, particularly suitable for dynamic IPT systems sensitive to parameter variations.

1. Introduction

Inductive power transfer (IPT) is a technology that enables power transmission through a magnetic flux without physical contact. It has found widespread applications in various fields, including electric vehicles, mobile phones, and medical transplantation [1,2,3]. However, IPT systems are classified as loosely coupled systems compared to tightly coupled systems. In an IPT system, the primary and secondary coils form a loosely coupled transformer, resulting in poor magnetic coupling between the two sides. This leads to power reduction, decreased efficiency, and increased VA capacity of the converter.

To address this issue, extensive research has been conducted. Mainstream solutions involve incorporating a resonant network (compensation network) into a series between the converter and primary circuit, as well as the secondary circuits and load circuit [3,4,5]. The resonant network primarily consists of reactive elements, with capacitors being the main component. Resonant networks are categorized into nine basic types based on the series or parallel connection of capacitors [6], with four (S-S, S-P, P-S and P-P) being extensively studied and considered to be mainstream topologies [7,8,9].

However, the introduction of a resonant network in an IPT system introduces coupling and mutual interference between the converter and the resonant network during operation. To ensure the stability of the IPT system, it is necessary for the converter frequency to align with the resonant network frequency, thus ensuring a zero phase angle impedance (ZPA) in the primary circuit [10]. Additionally, IPT systems experience the problem of frequency bifurcation [11]. Due to the possibility of misalignment, an uncertainty between the loosely coupled transformer and resonant network occurs, which leads to frequency shifts and bifurcation [12,13], therefore, frequency tracking is necessary.

One common approach is to insert a controlled impedance network on the primary and secondary sides to match any changes that may occur [14,15,16,17]. These controllable impedance networks typically employ self-switching reactive elements, such as self-switching capacitors [1,14]. However, this tracking method has a disadvantage in that it cannot cover the entire frequency range due to the limited number of switches available. Another approach is to utilize a phase-locked loop (PLL) to construct a frequency loop for accurate frequency tracking [18,19,20]. However, the drawback of PLL is that it introduces circuit complexity and may have tracking delay.

The self-oscillating technique is widely employed in various applications, including induction heating, and has also been used in IPT system. This technique utilizes a sensor and sampling circuit to track the zero crossing of the primary current. The switches are then triggered at the zero crossing point to generate self-excited oscillation. By employing this method, the converter and resonant network frequencies can be synchronized, ensuring accurate frequency tracking [21,22,23,24,25]. Nevertheless, in these self-oscillation applications, it is crucial for the converter frequency to align with the resonant network frequency. Consequently, some coupling between the converter and the resonant network still exists, requiring the operation of switches at the zero crossing point. This, in turn, amplifies the complexity of the control strategy. Furthermore, due to the inherent delay introduced by the sensor, sampling and shaping circuits, the actual switching point may deviate from the zero crossing, resulting in the loss of soft switching opportunities.

In practice applications, it is necessary to regulate the voltage and power to achieve the desired output. Conventional solutions involve using a DC/DC converter in either the primary or secondary sides to control the bus voltage and load impedance [26,27] or regulating phase shift to control power flow [28,29]. However, inserting a DC/DC converter into the system introduces additional losses, which can result in decreased overall efficiency. On the other hand, the phase-shifting technology may cause the switching point to deviate from the zero crossing, leading to the loss of the soft switching condition.

In the literature [30,31], a quasi-resonant control strategy is presented that divides an energy transfer period into two states: the resonant state and the energy-independent injection state. This control strategy provides an effective scheme for power control. During the resonant state, the converter is isolated from the resonant network, allowing the system to resonate freely. This resonant state lasts for less than one cycle, enabling frequency tracking during this period. In the energy injection state, the resonant network is isolated from the converter, and the power source directly injects energy into the primary inductor. This decouples the converter from the resonant network, allowing for the independent regulation of power by adjusting the duration of the energy injection state. The limitation of the literature [30,31] lies in the complexity of the system control strategy and the absence of a closed-loop scheme.

In this paper, the objective is to find a way to eliminate the coupling between the converter and the resonant network, allowing them to operate independently, and reducing the problems caused by coupling. To accomplish this, this paper proposes a variable structure approach that divides a converter into three fundamental operating states: switch-on state, blocking state, and diode-on state, based on their functions within an IPT system.

In these three states, the blocking state serves to achieve quasi-resonance within the system and is employed for tracking changes in system frequency. The switch-on state is utilized to inject power and adjust the output power. The diode state maintains soft switching conditions for the converter when transitioning between the blocked and switched states.

Furthermore, these three states can be combined into two distinct states: quasi-resonant and power injection states, which are independent of each other.

Building upon this foundation, this paper introduces a phase-closed loop control topology based on the quasi-resonant and power injection modes. In this closed-loop system, the phase of the quasi-resonant state acts as a feedback signal, triggering the self-oscillation of the system. This means that the system can achieve self-sustained oscillation without the need for external control. Additionally, within this closed-loop, a control variable is employed to regulate the duration of the power injection mode for power adjustment. To facilitate clear understanding, we refer to the quasi-resonant state as the self-oscillating state, while the power injection state retains its original designation.

For a better understanding,

Table 1. The comparison of the decoupling ability and power regulation effect between this work and the literature.

Ref.	Compensation Network	Closed-Loop	Feedback Signal	Resonant Mode	Coupling between Converter and Network	Power Control Strategy
[20]	S-S	Yes	Primary side Current	Perfect resonance	Strong	—
[23]	S-S	Yes	Primary side Current	Perfect resonance	Strong	Regulating phase
[25]	S-CC	Yes	Current from current transformer	Perfect resonance	Strong	Regulating phase
[26]	S-S	Yes	Load voltage	Perfect resonance	Strong	Additional converter
[28]	LCC-S	Yes	Load voltage and current	Perfect resonance	Strong	Regulating phase
[31]	P-S	No	—	quasi-resonant	No	Regulating the duration of energy injection state
This work	P-P	Yes	Voltage from primary side	quasi-resonant	No	Regulating the duration of energy injection state

briefly compares the coupling between existing converters and resonant networks, as well as the power regulation techniques.

Adopting a closed-loop control strategy in prior studies has essentially solved the problem of frequency tracking. However, the coupling between the converter and the resonant network persists, requiring switching points at current zero crossings, which complicates system control. Additionally, power regulation in prior studies still relies on techniques like phase shifting or adding DC/DC converters, whose drawbacks have been discussed extensively in much of the literature.

In document [31], a strategy of time-sharing energy injection and free resonance is adopted based on the quasi-resonant mode to address the coupling and power regulation issues. However, it falls short in establishing closed-loop control and providing a comprehensive solution.

To address these shortcomings, this paper proposes dividing the converter’s operation into three modes: blocking, diode conduction, and switch conduction. These modes allow for dividing the energy transmission period into two states: free resonance and power injection. This approach achieves decoupling between the converter and the resonant network and enables power adjustment by controlling the energy injection time. With closed-loop control, it exhibits favorable dynamic characteristics and power adjustment capabilities.

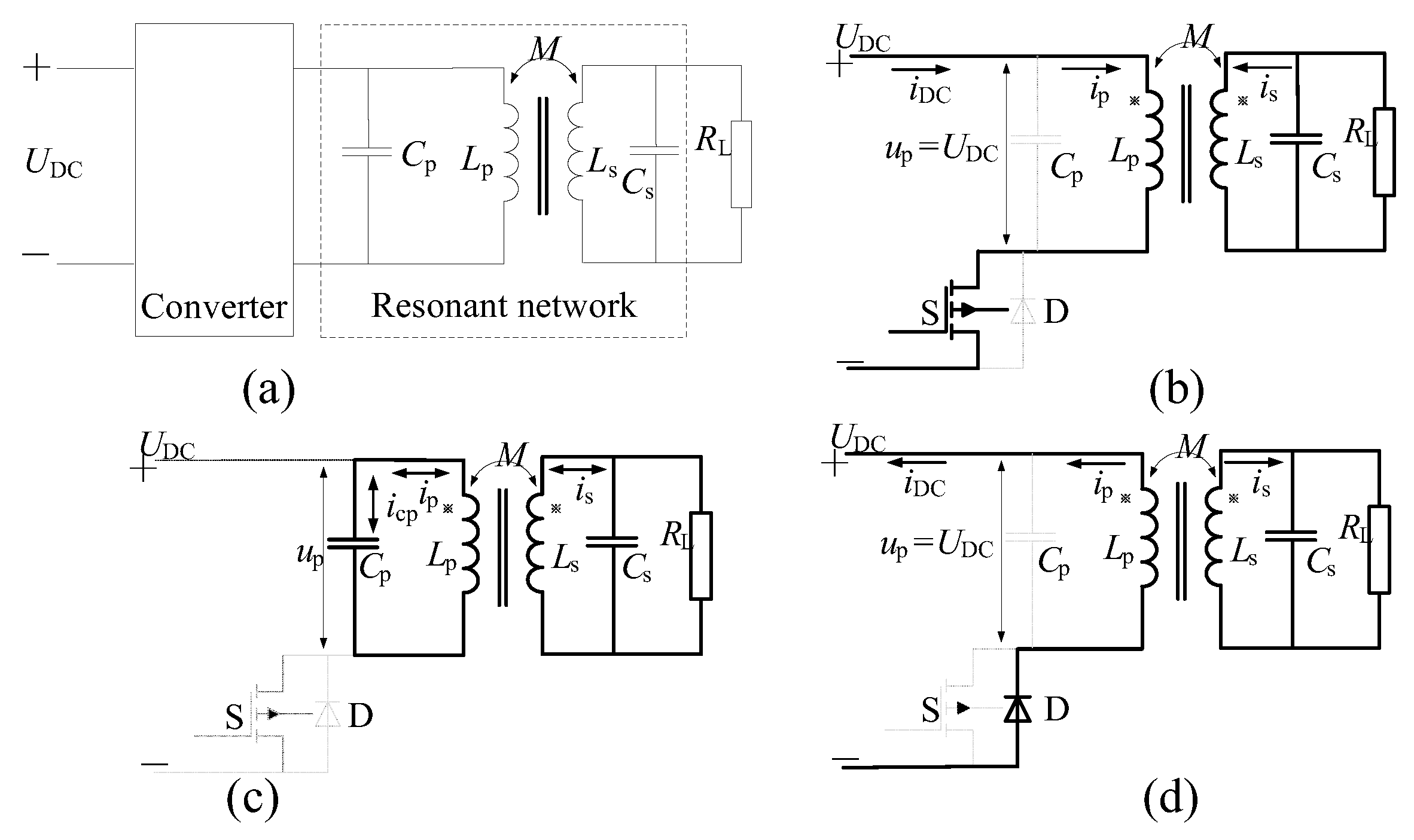
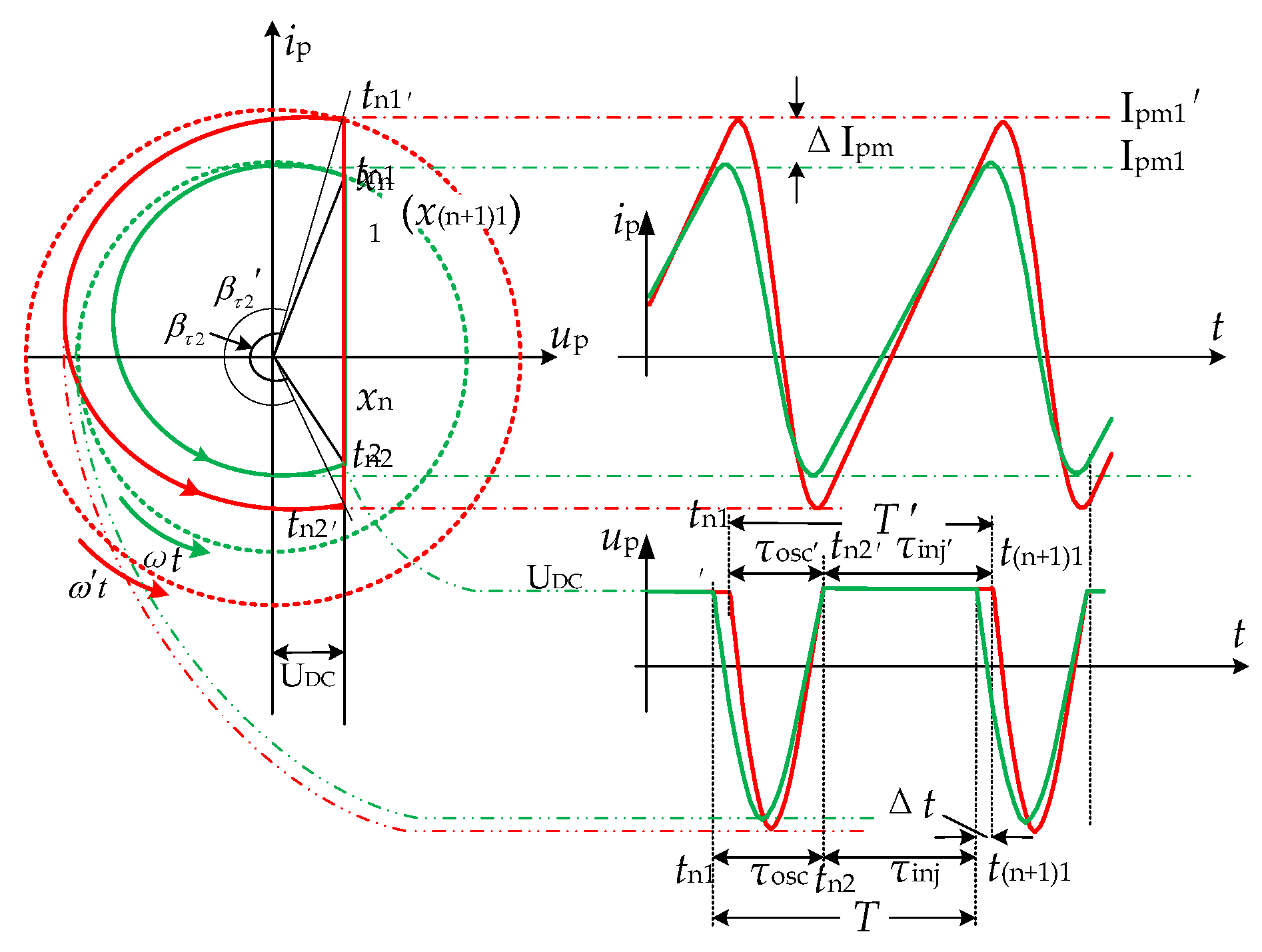
2. Variable Structure Analysis of an IPT System

Figure 1a depicts an IPT charging system with a converter in the primary side and a resonant network (compensation network) on both the primary and secondary sides. In Figure 1a, the U_DC represents the DC source that supplies power to the system, and R_L stands for load. C_p and L_p constitute the primary resonant network, while C_s and L_s constitute the secondary resonant network. Mutual inductance, M, establishes a magnetic link between the primary and the secondary to transmit power. Furthermore, L_p and L_s together form a loosely coupled transformer. It is evident that this IPT system belongs to P–P compensation topology.

It is widely recognized that the converter can be constructed using various topologies such as full-bridge, half-bridge, or single switch configurations. These topologies employ semiconductor switches, with each switch being equipped with a body diode. Consequently, during the operation of an IPT system, the converter can be categorized into three states based on its working structure: the switch-on state, the blocking state, and the diode-on state.

To visually illustrate these three states, Figure 1b–d employ a single switch converter as an example. In this context, S represents a semiconductor device, such as an IGBT, while D signifies the body diode associated with S.

Figure 1b illustrates the behavior of the switch-on state of a converter. During this state, the switch S is activated to deliver U_DC to L_p, generating a primary inductor current i_p with the slope of K_E, injecting power into the system, and the value of K_E can be determined as follows:

$$K_{\mathrm{E}}=\frac{U_{\mathrm{DC}}}{L_{\mathrm{p}}}$$

(1)

In the switch-on state, due to the connection of U_DC to L_p, the voltage across C_p is clamped at U_DC, and there is current flowing into the secondary coil.

Figure 1c depicts the behavior of the blocking state, where both switches and diodes are deactivated, leading to the isolation of U_DC from the resonant network. During this state, the resonant network initiates oscillation with an angular frequency ω. This angular frequency is solely determined by the system parameters, such as C_p, L_p and secondary reflected impedance, as the resonant network is no longer influenced by the converter.

It is important to note that C_p is connected in parallel with the output of the converter, ensuring that the voltage across C_p does not exceed U_DC. As a result, the oscillation of the system in the blocking state is incomplete.

Figure 1d illustrates the behavior of the diode-on state. In this state, the diode D is activated, allowing U_DC to be reconnected to L_p. These activated diodes provide a pathway for the current i_p to flow back to U_DC. Similar to the switch-on state, the voltage across C_p remains clamped at U_DC during the diode-on state.

While we employ a single-switch converter in the analysis of these three states, in fact, it is worth noting that these three states are also applicable to the half-bridge and full-bridge converter mentioned earlier.

If the loosely coupled transform in Figure 1 is replaced by the T model, the resonant network can be represented by the equivalent circuit shown in Figure 2. In this circuit, L_pk and L_sk denote the leakage inductances of the primary and secondary, respectively, while L_M represents the mutual inductance. The relationship between these parameters can be expressed as follows:

$$\{\begin{array}{c}{L}_{\mathrm{M}}=M\\ L_{\mathrm{pk}}=L_{\mathrm{p}}-M\\ L_{\mathrm{sk}}=L_{\mathrm{s}}-M\\ k=\frac{M}{\sqrt{L_{\mathrm{p}}{L}_{\mathrm{s}}}}\end{array}$$

(2)

In Equation (2), k represents the coupling coefficient, which indicates the efficiency of energy transferring from the primary to secondary side. For an IPT system, the coupling coefficient k is typically weak. As a result, only a portion of the power can be transmitted to the secondary side, and a significant amount of energy remains stored in leakage inductance L_pk. This energy needs to be returned to U_DC during a power transfer period.

Based on the characteristics of the resonant network in Figure 2, the operational reality can be described as follows: during the switching on state, U_DC injects power into the primary inductor L_p; during the blocking state, the injected power excites the resonant network, causing it to oscillate; and during the diode-on state, the residual energy stored in leakage inductance L_pk is returned to U_DC.

In Figure 2, capacitor C_p, inductors L_pk, and L_M form a compensation topology. In this compensation network, the power injected into the mutual inductance L_M is transmitted to the secondary side. Meanwhile, the power remaining in leakage inductance L_pk is utilized to sustain the diode-on state and subsequently returned to the power source U_DC.

A characteristic of the compensation network is its ability to harness the energy stored in the leakage inductance to sustain the diode-on state. This bestows a substantial soft-switching margin upon the converter. Consequently, the diode-on state can be employed to establish an isolated transition zone between the blocking state and the switch-on state. Moreover, by designing a repeating converter operational cycle as follows: blocking state → diode-on state → switch-on state → blocking state, and by using some hardware circuits, we can construct a self-oscillating closed loop for an IPT system, as shown in Figure 3. With the diode-on state acting as a buffer, the system designed by this method has a wide soft switching margin.

In this self-oscillating loop, the blocking state facilitates free oscillation, the switch-on state implements power injection, and the diode-on state contributes to the soft switch behavior. By employing this approach, the decoupling between the converter and the resonant network can be achieved while also realizing self-oscillation of the system.

Figure 4 provides a vector trajectory diagram and curves of this self-oscillating loop, demonstrating the dynamic behavior of i_p, u_p. To illustrate the relationship between the switch S and i_p, u_p, the control signal of S, v_g, is also depicted in Figure 4.

In Figure 4, the left side displays the vector trajectory diagram. The dotted circle represents the oscillating vector trajectory, while the solid black line represents the actual running vector trajectory of i_p, u_p. Assuming that the system has achieved stability in the nth period, it can be seen that the vector trajectory forms a closed curve divided into three segments: τ₁, τ₂, and τ₃, respectively. t_n0 denotes the starting point of the switch-on state, t_n1 is the starting point of blocking state, and t_n2 is the starting point of the diode-on state.

Referring to Figure 1 and Figure 4, during the τ₁ segment, [t_n0, t_n1], the vector trajectory is in the switch-on state, with u_p clamped at U_DC. As a result, the vector trajectory in this segment is a straight line parallel to the i_p axis, where u_p = U_DC. It starts from i_p = 0 and extends to the oscillating circle at t_n1. The phase angle occupied by τ₁ is represented by β_τ1.

During the τ₂ segment, [t_n1, t_n2], the system enters the blocking state, and the oscillation begins. However, due to attenuation, the vector trajectory deviates from the oscillating circle and follows a decaying circular curve. The phase angle occupied by τ₂ is represented by β_τ2.

During the τ₃ segment, [t_n2, t_(n+1)0], the system is in the diode-on state, similar to τ₁, with u_p clamped at U_DC. Consequently, the vector trajectory in this segment appears as a parallel line parallel to axis i_p, where u_p = U_DC. The phase angle occupied by τ₃ is represented by β_τ3.

In Figure 4, the right side presents the curves of i_p, u_p, and the relationship between vector trajectory and these curves is indicated by dot-dash lines. Referring to Figure 1, the following is an analysis of i_p, u_p curves in different states:

[t_n0, t_n1]: This interval represents the switch-on state, τ₁. The control signal v_g has been at a high level, indicating that switch S has been turned on and U_DC supplied to L_p. The current i_p increases linearly from zero with slope K_E, while u_p remains clamped at U_DC. At t_n1, when i_p reaches i_p (t_n1), v_g is pulled to a low level, turning off switch S, and the system exits the switch-on state. As the voltage of C_p is equal to U_DC, switch S is turned off with the zero voltage switch (ZVS) condition.

[t_n1, t_n2]: This interval represents the blocking state. During this interval, the system experiences oscillation at the angular frequency ω, causing in-sinusoidal variations in both u_p and i_p. Since the phase angle occupied by oscillation segment, β_τ2, is greater than π but less than 2π, the i_p exhibits two amplitudes, I_pm1, I_pm2, within this interval. Between these two amplitudes, i_p passes through a zero crossing.

After t_n1, the capacitor voltage u_p starts to decrease from its maximum value, U_DC. It then reaches its lowest point at the zero crossing of the current, i_p. Subsequently, as u_p begins to rise again, it reaches U_DC once more at t_n2. When u_p reaches U_DC, diode D is turned on, clamping u_p to U_DC again. Consequently, the system loses the oscillation condition and exit the blocking state at t_n2. At t_n2, the current i_p reaches value of i_p (t_n2).

[t_n2, t_(n+1)0]: This interval represents the diode-on state. After t_n2, the current i_p increase linearly from i_p (t_n2) with a slope of k_E, indicating that the energy stored in the leakage inductor L_pk flows back to power source during this interval. Assuming that the switch S has been turned on before i_p reaches its zero crossing, as shown in Figure 4, the system will automatically transition from the diode-on state to the switch-on state at the zero crossing of i_p, denoted as point t_(n+1)0. Therefore, after t_(n+1)0, the system will exit from the diode-on state and enter the switch-on state again, initiating a new power injection process.

Although the converter mentioned above is divided into three states, Figure 4 shows that the vector trajectories of the switch-on state and the diode-on state are the same line segment parallel to i_p axis. Additionally, as depicted in Figure 1, in both states, U_DC is directly connected to L_p, and the voltage of C_p is clamped at U_DC. That is to say, the function of both states is to maintain the power flow in or out of L_p. Therefore, these two states can be collectively referred to as power injecting states, and the durations τ₁ and τ₃ can be combined into τ_inj = τ₁ + τ₃. Similarly, the blocking state can also be referred to as a self-oscillating state, and the duration τ₂ can be denoted as τ_osc.

The value of τ_osc depends on the phase angle β_τ2 and the angular frequency ω, which are determined by real-time parameters. Therefore, frequency tracking can be achieved by monitoring τ_osc and implementing appropriate compensation. On the other hand, the power injected from U_DC to L_p is determined by the duration of power injection state, τ_inj. That is to say, τ_inj can be used as a control variable to regulate the system power.

Figure 5 is utilized to illustrate the impact of parameter changes on τ_osc and τ_inj during the operation of the proposed IPT system. When parameters are modified, the system follows a new vector trajectory, resulting in corresponding alterations in the curves of i_p, u_p. In Figure 5, the trajectories and curves with unchanged parameters are represented in green, while those after the changes are depicted in red. By comparing the green and red voltage curve, it can be observed that changes in system parameters result in a shift in the start time of the oscillation state from t_n1 to t_n1’, a shift in the end time from t_n2 to t_n2’, a change in the angular frequency from ω to ω’ and a change in the phase angle from β_τ2 to β_τ2’. These changes cause a shift in the duration of the oscillating state from τ_osc to τ_osc’.

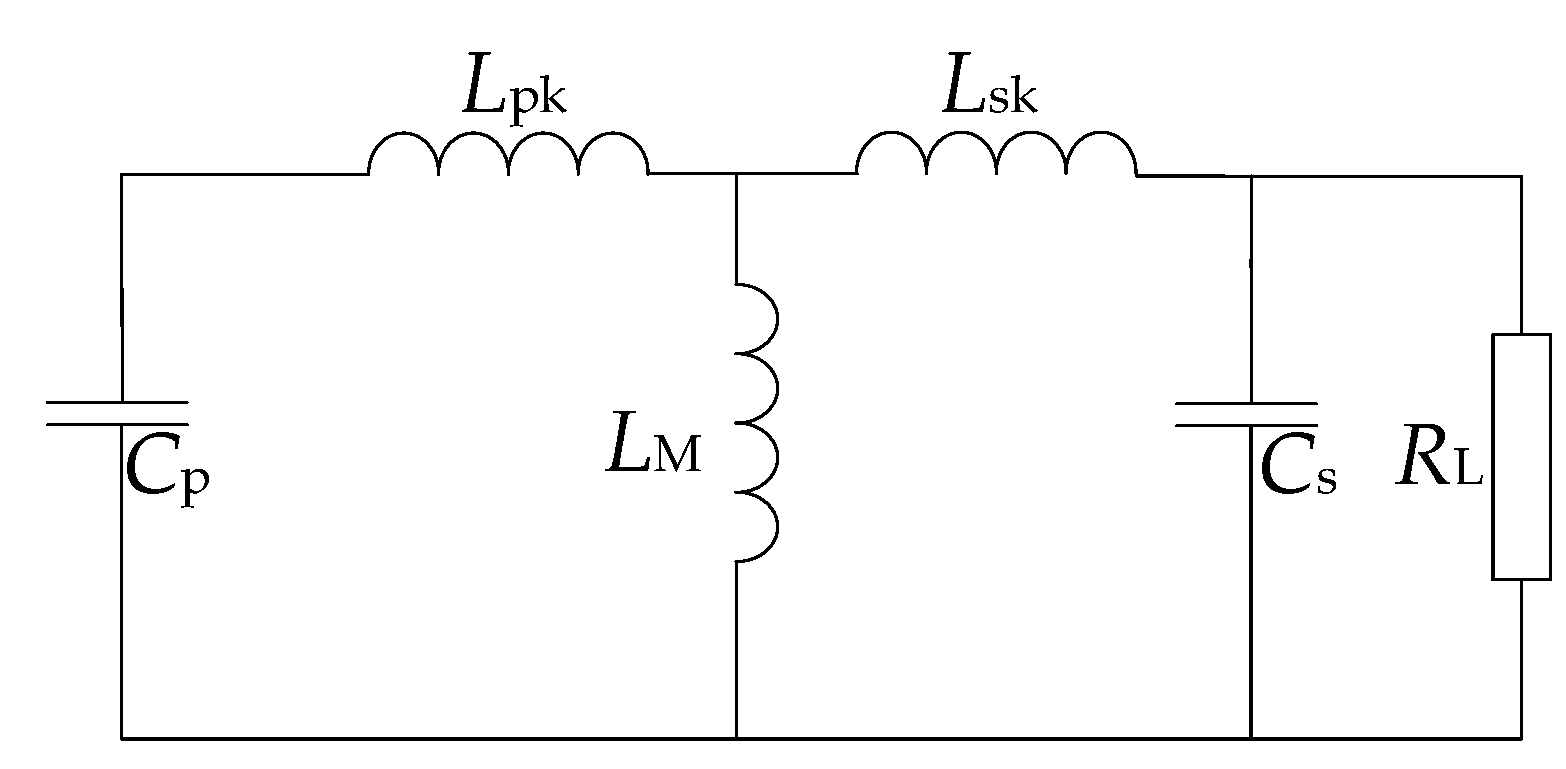
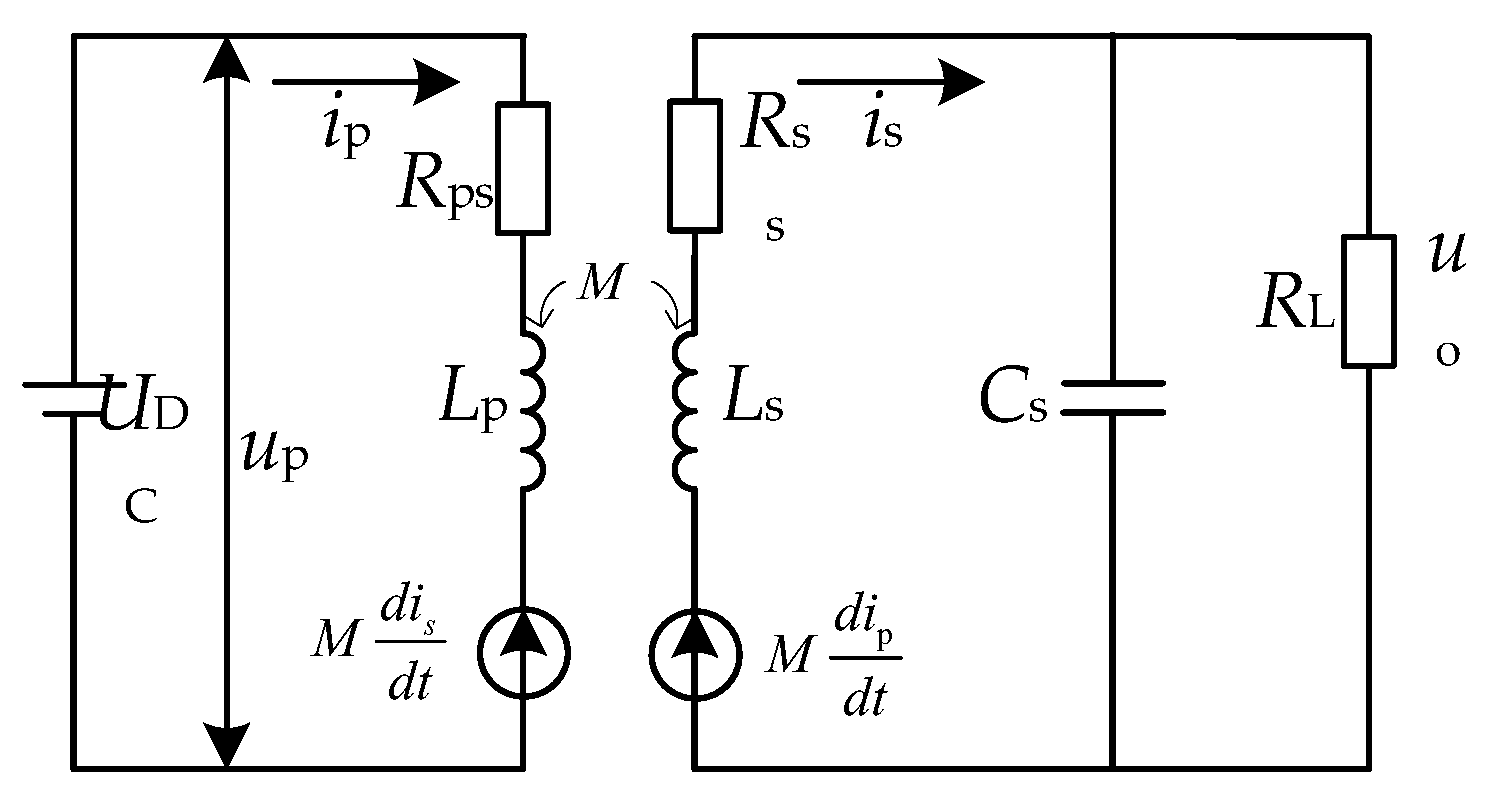
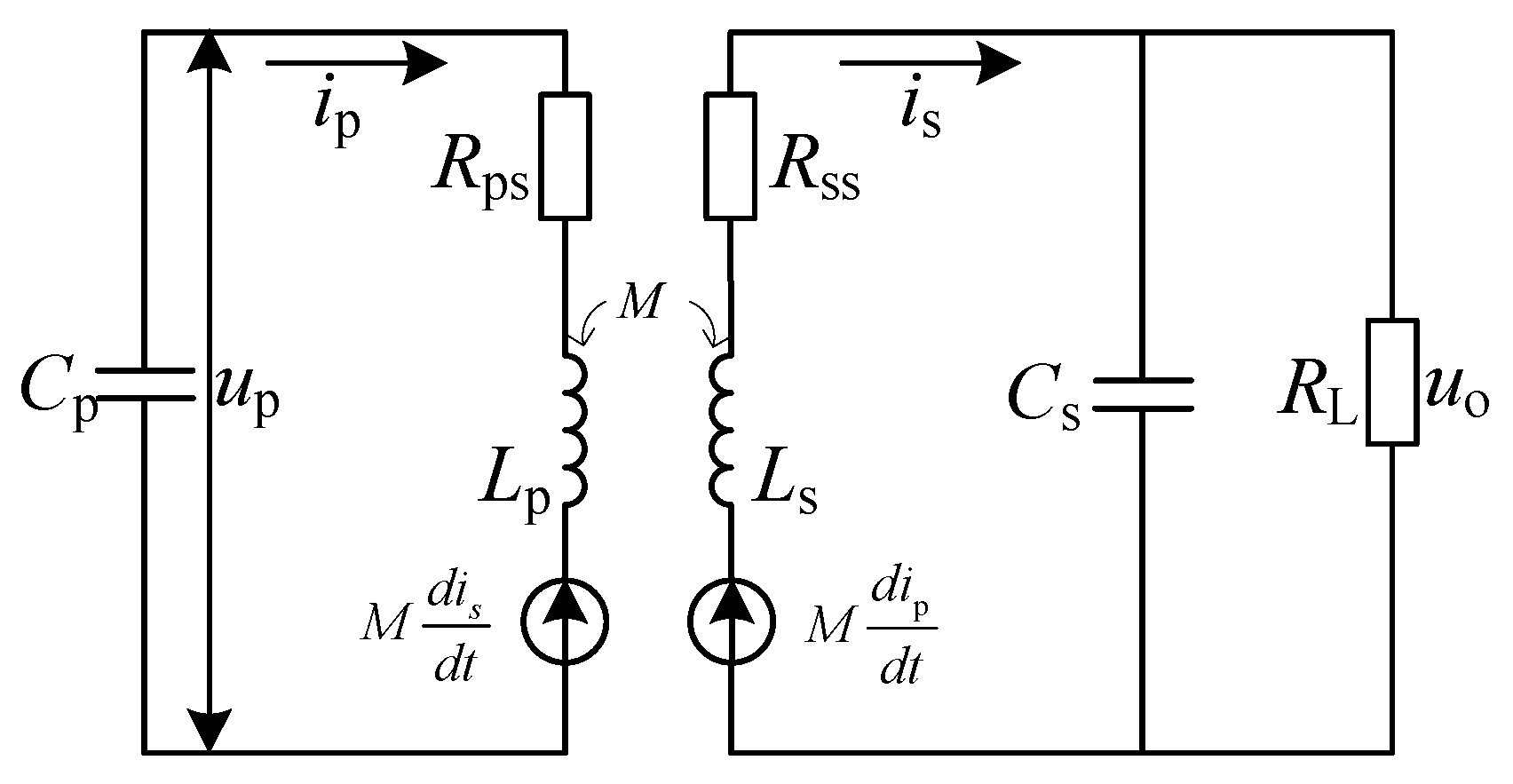
3. Mathematical Model

Figure 6 illustrates a schematic diagram of the power injecting model.

On the primary side, the U_DC directly supplies to L_p. Taking into account the copper resistance of the coil, R_ps and R_ss denote the loss resistances of the primary and secondary inductors, respectively. L_p and L_s act as power transfer channels, creating a loosely coupled transformer between the primary and secondary sides. The symbol M represents the mutual inductance of the transformer. Additionally, the controlled voltage M × di_p/dt signifies the impact of the secondary current on the primary side, while M × di_s/dt represents the effect of the primary current on the secondary side.

Figure 7 depicts the schematic diagram of the oscillating state model. In this model, the power source U_DC illustrated in Figure 6 is replaced with the capacitor C_p, while the remaining components remain unchanged as depicted in Figure 6.

If a power electronic system exhibits multiple structures periodically during each stabilization period, these structures are determined by the actual state of the circuit. Meanwhile, these structures possess their own linear dynamics, and the system can be modeled using a general state space representation [32]:

$$\{\begin{array}{cc}{x}^{\prime }=A_{i}x+B_{i}u& i=\mathrm{osc},\mathrm{inj}\\ y=Cx& \end{array}$$

(3)

where, A is the characteristic matrix, B is the input matrix, C is the output matrix and i represents the serial number of each structures. Respectively, x represents the state variable, with x = [u_p, i_p, i_s, u₀]^T; u denotes the input variable, with u = [U_DC]; and y represents the output variable.

Based on Figure 6 and Figure 7, the characteristic matrix and input matrix of the power-injecting and self-oscillating states can be written as Equations (4) and (5).

$$\begin{array}{l}{A}_{\mathrm{inj}}=\left[\begin{array}{cccc}-1& 0& 0& 0\\ 0& -\frac{L_{\mathrm{s}}{R}_{\mathrm{ps}}}{\mathsf{\Delta }}& -\frac{M{R}_{\mathrm{ss}}}{\mathsf{\Delta }}& -\frac{M}{\mathsf{\Delta }}\\ 0& -\frac{M{R}_{\mathrm{ps}}}{\mathsf{\Delta }}& -\frac{L_{\mathrm{p}}{R}_{\mathrm{ss}}}{\mathsf{\Delta }}& -\frac{L_{\mathrm{p}}}{\mathsf{\Delta }}\\ 0& 0& \frac{1}{C_{\mathrm{s}}}& -\frac{1}{C_{\mathrm{s}}{R}_{\mathrm{L}}}\end{array}\right]\\ B_{\mathrm{inj}}={\left[\begin{array}{cccc}1& \frac{L_{\mathrm{s}}}{\mathsf{\Delta }}& \frac{M}{\mathsf{\Delta }}& 0\end{array}\right]}^T\end{array}$$

(4)

$$\begin{array}{l}{A}_{\mathrm{osc}}=\left[\begin{array}{cccc}0& -\frac{1}{C_{\mathrm{p}}}& 0& 0\\ \frac{L_{\mathrm{s}}}{\mathsf{\Delta }}& -\frac{L_{\mathrm{s}}{R}_{\mathrm{ps}}}{\mathsf{\Delta }}& -\frac{M{R}_{\mathrm{ss}}}{\mathsf{\Delta }}& -\frac{M}{\mathsf{\Delta }}\\ \frac{M}{\mathsf{\Delta }}& -\frac{M{R}_{\mathrm{ps}}}{\mathsf{\Delta }}& -\frac{L_{\mathrm{p}}{R}_{\mathrm{ss}}}{\mathsf{\Delta }}& -\frac{L_{\mathrm{p}}}{\mathsf{\Delta }}\\ 0& 0& \frac{1}{C_{\mathrm{s}}}& -\frac{1}{C_{\mathrm{s}}{R}_{\mathrm{L}}}\end{array}\right]\\ B_{\mathrm{osc}}={\left[\begin{array}{cccc}0& 0& 0& 0\end{array}\right]}^T\end{array}$$

(5)

In the time domain, when the variable t is used as the time variable for each state running process, then, the solution of Equation (3) represents the time function that describes the system behavior [33]:

$$\begin{array}{cc}x(t)={\mathsf{\Phi }}_i(t-t_0)x_0+A_i^{-1}({\mathsf{\Phi }}_i(t-t_0)-I)B_{i}u& i=\mathrm{osc},\mathrm{inj}\end{array}$$

(6)

where, x₀ represents the initial value at the beginning of each state at time t₀, with x₀ = x(t₀). I denotes the identity matrix. Φ(t) is defined as Φ(t) = exp{A_it}, i = inj, osc.

Referring back to Figure 5 to discuss the contents of the green line, in the proposed IPT system, as described earlier, there exist two states durations, namely τ_osc and τ_inj, respectively. Therefore, as depicted in Figure 5, a power transfer period T can be defined as follows:

$$T={\tau }_{\mathrm{osc}}+{\tau }_{\mathrm{inj}}$$

(7)

Let us begin by analyzing the interval [t_n1, t_n2], which represents the oscillating state duration. According to Equation (5), in this interval, the input matrix B is a zero matrix. Therefore, Equation (6) can be rewritten as follows:

$$\begin{array}{cc}x(t)={\mathsf{\Phi }}_{\mathrm{osc}}(t-t_{\mathrm{n}1})x_{\mathrm{n}1}& t_{\mathrm{n}1}\le t<\end{array}{t}_{\mathrm{n}2}$$

(8)

Then, let us analyze the interval [t_n2, t_(n+1)1], which represents the power injecting state duration. According to Equation (4), Equation (6) can be rewritten as follows:

$$\begin{array}{cc}x(t)={\mathsf{\Phi }}_{\mathrm{inj}}(t-t_{\mathrm{n}2})x_{\mathrm{n}2}+A_{\mathrm{inj}}^{-1}({\mathsf{\Phi }}_{\mathrm{inj}}(t-t_{\mathrm{n}2})-I)B_{\mathrm{inj}}u& t_{\mathrm{n}2}\le t_{(\mathrm{n}+1)1}\end{array}$$

(9)

Referring to Figure 5, by substituting τ_osc and τ_inj into Equations (8) and (9), the state variables x_n1 and x_n2 at the end of the power injecting state and the oscillating state can be obtained, respectively. x_n1 and x_n2 can be derived as follows:

$$\{\begin{array}{cc}{x}_{\mathrm{n}1}={\mathsf{\Phi }}_{\mathrm{inj}}({\tau }_{\mathrm{inj}})x_{\mathrm{n}2}+A_{\mathrm{inj}}^{-1}({\mathsf{\Phi }}_{\mathrm{inj}}({\tau }_{\mathrm{inj}})-I)B_{\mathrm{inj}}u& t_{\mathrm{n}2}\le t_{(\mathrm{n}+1)1}\\ x_{\mathrm{n}2}={\mathsf{\Phi }}_{\mathrm{osc}}({\tau }_{\mathrm{osc}})x_{\mathrm{n}1}& t_{\mathrm{n}1}\le t<t_{\mathrm{n}2}\end{array}$$

(10)

By combining the two equations in Equation (10), x_n1 can be expressed as follows:

$$x_{\mathrm{n}1}={(I-{\mathsf{\Phi }}_{\mathrm{inj}}({\tau }_{\mathrm{inj}}){\mathsf{\Phi }}_{\mathrm{osc}}({\tau }_{\mathrm{osc}}))}^{-1}{A}_{\mathrm{inj}}^{-1}({\mathsf{\Phi }}_{\mathrm{inj}}({\tau }_{\mathrm{inj}})-I)B_{\mathrm{inj}}u$$

(11)

Referring to the vector trajectory shown in Figure 4, if we assume that x has already stabilized at t_n1 with a value of x_n1, then, after a duration of one period T, x will repeatedly return to x_n1 at t_(n+1)1. In other words, x_n1 will be equal to x_(n+1)1, indicating that x_n1 is a fixed point. By applying the fixed point mapping calculation, and considering the end-time point of power injection state t_n1, the capacitor voltage of u_p is clamped at U_DC. Therefore, the end value of the power injecting stat can be obtained as C × x_n1 = U_DC, where the output matrix C = [1,0,0,0]. As a result, Equation (11) can be modified as follows:

$$\{\begin{array}{c}{U}_{\mathrm{DC}}=C{(I-{\mathsf{\Phi }}_{\mathrm{inj}}({\tau }_{\mathrm{inj}}){\mathsf{\Phi }}_{\mathrm{osc}}({\tau }_{\mathrm{osc}}))}^{-1}{A}_{\mathrm{inj}}^{-1}({\mathsf{\Phi }}_{\mathrm{inj}}({\tau }_{\mathrm{inj}})-I)B_{\mathrm{inj}}{U}_{\mathrm{DC}}\\ C=[\begin{array}{cccc}1& 0& 0& 0\end{array}]\end{array}$$

(12)

Taking a power transfer period T as a known variable and Substituting τ_inj = T − τ_osc into Equation (12), Equation (12) can be modified as:

$$\{\begin{array}{c}{U}_{\mathrm{DC}}=C{(I-{\mathsf{\Phi }}_{\mathrm{inj}}(T-{\tau }_{\mathrm{osc}}){\mathsf{\Phi }}_{\mathrm{osc}}({\tau }_{\mathrm{osc}}))}^{-1}{A}_{\mathrm{inj}}^{-1}({\mathsf{\Phi }}_{\mathrm{inj}}(T-{\tau }_{\mathrm{osc}})-I)B_{\mathrm{inj}}{U}_{\mathrm{DC}}\\ C=[\begin{array}{cccc}1& 0& 0& 0\end{array}]\end{array}$$

(13)

After determining the value of T, the oscillating and injecting durations, τ_osc and τ_inj, can be obtained by solving Equation (13). The expressions for τ_osc and τ_inj as functions of T can be written as follows:

$$\{\begin{array}{c}{\tau }_{\mathrm{osc}}=f(T)\\ {\tau }_{\mathrm{inj}}=T-f(T)\end{array}$$

(14)

By utilizing Equation (14), the duration of τ_osc and τ_inj can be calculated. By substituting these calculated results into Equations (10) and (11), the fixed points, x_n1 and x_n2, can be expressed as functions in the following manner:

$$\{\begin{array}{c}{x}_{\mathrm{n}1}=F_{\mathrm{inj}}({\tau }_{\mathrm{osc}},{\tau }_{\mathrm{inj}})\\ x_{\mathrm{n}2}=F_{\mathrm{osc}}({\tau }_{\mathrm{osc}},x_{\mathrm{n}1})\end{array}$$

(15)

By substituting Equation (15) into Equation (8), the time domain solution of the state variable of the oscillating state can be obtained:

$$\begin{array}{cc}x(t)={\mathsf{\Phi }}_{\mathrm{osc}}(t-t_{\mathrm{n}1})F_{\mathrm{inj}}({\tau }_{\mathrm{osc}},{\tau }_{\mathrm{inj}})& t_{\mathrm{n}1}\le t<\end{array}{t}_{\mathrm{n}2}$$

(16)

By substituting Equation (15) into Equation (9), the time domain solution of the state variable of the power injecting state can be obtained:

$$\begin{array}{cc}x(t)={\mathsf{\Phi }}_i(t-t_{\mathrm{n}2})F_{\mathrm{osc}}({\tau }_{\mathrm{osc}},x_{\mathrm{n}1})+A_{\mathrm{inj}}^{-1}({\mathsf{\Phi }}_{\mathrm{inj}}(t-t_{\mathrm{n}2})-I)B_{\mathrm{inj}}u& t_{\mathrm{n}2}\le t_{(\mathrm{n}+1)1}\end{array}$$

(17)

According to Equation (3), if we define C_up = [1, 0, 0, 0] and C_ip = [0, 1, 0, 0], the time domain expression of the voltage u_p and current i_p can be obtained as follows:

$$\begin{array}{cc}\{\begin{array}{c}{u}_{\mathrm{p}}(t)=C_{u\mathrm{p}}{\mathsf{\Phi }}_{\mathrm{osc}}(t-t_{\mathrm{n}1})F_{\mathrm{inj}}({\tau }_{\mathrm{osc}},{\tau }_{\mathrm{inj}})\\ i_{\mathrm{p}}(t)=C_{i\mathrm{p}}{\mathsf{\Phi }}_{\mathrm{osc}}(t-t_{\mathrm{n}1})F_{\mathrm{inj}}({\tau }_{\mathrm{osc}},{\tau }_{\mathrm{inj}})\end{array}& t_{\mathrm{n}1}\le t<\end{array}{t}_{\mathrm{n}2}$$

(18)

If we define C_uo = [0,0,0,1], the output power can be obtained as follows:

$$P_o=\frac{1}{T}{\displaystyle {\int }_0^T\frac{{(C_{u\mathrm{o}}{\mathsf{\Phi }}_{\mathrm{osc}}(t-t_{\mathrm{n}1})F_{\mathrm{inj}}({\tau }_{\mathrm{osc}},{\tau }_{\mathrm{inj}}))}^2}{R_{\mathrm{L}}}dt}$$

(19)

To verify whether the models in Figure 6 and Figure 7 and the associated theoretical analysis are consistent with Figure 4, as well as to confirm the accuracy of the proposed self-oscillating IPT system, Equations (3)–(18) were simulated and tested using MATLAB R2016b. The parameters used for the validation process are listed in

Table 2. The main simulation parameters.

Parameter	Design Value	Parameter	Design Value
UDC	100 V	Lp, Ls	100 μH, 100 μH
M	50 μH	Cp, Cs	0.3 μF, 0.3 μF
Rps, Rss	0.07 Ω, 0.07 Ω	T	40 μs

.

Figure 8 illustrates the calculation results, where the voltage curve u_p and current curve i_p align with Figure 4 and Figure 5, respectively. This confirms the accuracy of the proposed theoretical model. The calculation results indicate that the system enters the oscillating state at 20 μs, and remains in that state until 40 μs, resulting in a duration of 20 μs.

Subsequently, the system transitions to the power injecting state at 40 μs and exits this state at 60 μs, with a duration of 20 μs. During the onset of the oscillating state, the current value i_p is 15.1 A. Following a 2 μs delay, i_p reaches its maximum value of 15.7 A (I_pm1). Similarly, prior to the system exiting the oscillating state, i_p reaches its negative maximum of −10.3 A (I_pm2). After a 4 μs delay, when the system exits the oscillating state at 40 μs, i_p measures −8.5 A.

Figure 9 illustrates the impact of the coupling coefficient k on the curves of i_p and u_p with a fixed value of T = 40 μs. As depicted in the figure, with k varying from 0.5 to 0.1, the starting time of the oscillating state advances by approximately 5 μs, while the amplitude of i_p decreases by approximately 6A. For a more detailed analysis of the effect of k on the oscillating state duration, τ_osc, and the amplitude, I_pm1, please refer to Figure 10.

For a more detailed analysis of the effect of coupling coefficient k on the duration of the oscillating state, τ_osc, and the amplitude of the primary current i_p, I_pm1, Figure 10 illustrates the relationship between τ_osc and I_pm1 as a function of k. The figure demonstrates that the τ_osc decreases with increasing values of k. This is attributed to the fact that a higher coupling coefficient results in a smaller leakage inductance L_pk for the loosely coupled transformer, consequently leading to a shorter oscillation period. Furthermore, it should be noted that an increase in the coupling coefficient also corresponds to an amplified amplitude of the primary current i_p. This can be attributed to the enhanced power transmission to the secondary side as k increases. Therefore, on the primary side, a greater amount of power needs to be injected during the power injecting state duration.

Clearly, the calculated results of Figure 9 and Figure 10 precisely correspond to those of Figure 4 and Figure 5, providing further evidence of the accuracy of the proposed theoretical models.

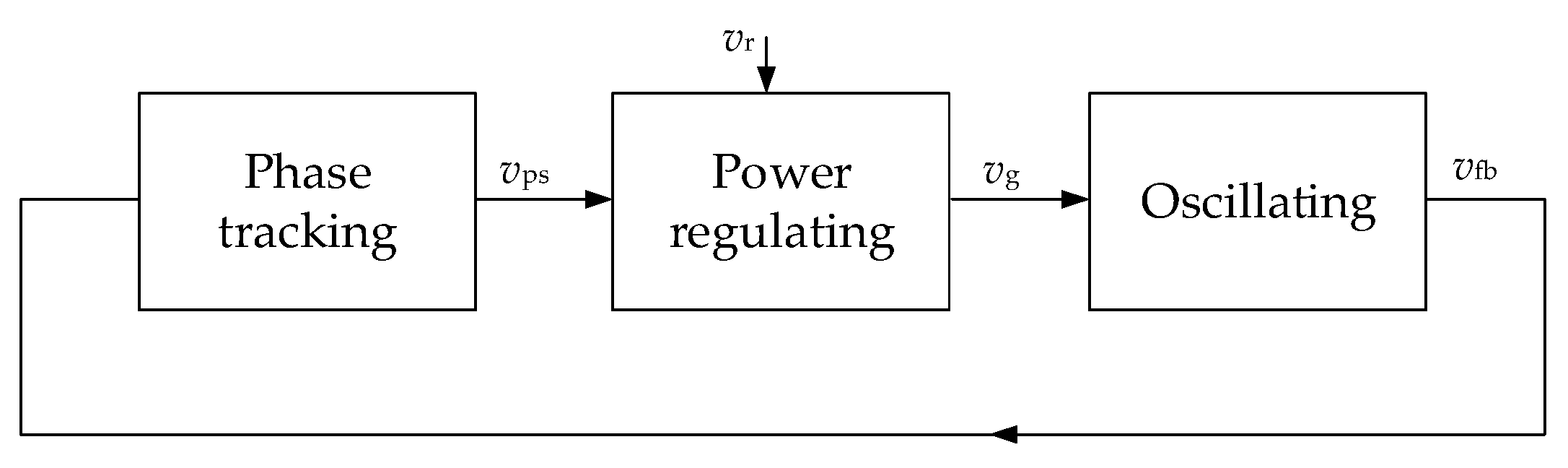
4. Control Method

As analyzed in Section 2, achieving oscillation phase tracing and power injection duration control are the key objectives of the proposed IPT system. Therefore, this chapter focuses on the methods employed to fulfill these requirements. Figure 11 illustrates the circuit diagram of the dynamic IPT system studied in this paper.

In this IPT system, a closed-loop phase tracking circuit is utilized to track the phase and trigger self-oscillation. This self-oscillating circuit consists of three components: phase tracking unit, power regulating unit, and oscillating unit. With this closed-loop control, the system achieves self-oscillation.

4.1. The Oscillating Unit

The oscillating unit adopts a topology that includes a single switch converter and an oscillating network. The converter employs an IGBT as switch S, while the oscillating network consists of the primary capacitor C_p, and inductor L_p. As depicted in Figure 1, the oscillation unit can alternate between the oscillating state and the power injecting state by controlling the switch S. To acquire the phase information of the system oscillation, a voltage divider comprising R_s1 and R_s2 is utilized as a sensor to monitor the voltage across switch S, denoted as u_ds. The expression for u_ds is:

$$u_{\mathrm{ds}}=(U_{\mathrm{DC}}-u_{\mathrm{p}})$$

(20)

The output of the sensor, v_fb, can be calculated using the following formula:

$$v_{\mathrm{fb}}=\frac{R_{\mathrm{s}2}}{R_{\mathrm{s}1}+R_{\mathrm{s}2}}{u}_{\mathrm{ds}}$$

(21)

4.2. Phase Tracking Unit

The phase tracking unit comprises R_d, D_d and comparator A₁, which is employed for tracking phase changes. R_d and D_d are responsible for generating a comparison threshold voltage v_d of 0.7 V, which is applied to the negative terminal of A₁. The feedback signal v_fb is then fed to the positive terminal of A₁ and compared with v_d. The output of A₁, v_ps, can be expressed as follows:

$$v_{\mathrm{ps}}=\{\begin{array}{cc}0& v_{\mathrm{fb}}<v_{\mathrm{d}}\\ U_{\mathrm{C}}& v_{\mathrm{fb}}>v_{\mathrm{d}}\end{array}$$

(22)

Based on Figure 4, and Equation (21), the signal v_fb is a sinusoidal pulse sequence in which pulse width signifies the duration of the oscillating state, τ_osc (phase angle β_τ2). Consequently, the duration of the high level output of v_ps, aligns with the duration of the oscillation state, τ_osc. Notably, according to Equation (22), the high-level duration of v_ps can be automatically adjusted to track τ_osc (phase angle β_τ2), thereby facilitating the phase tracking within the system.

A phase tracking process can be demonstrated using Figure 12. In the figure, the curves before the phase change are highlighted in green, while the curves after the phase change are marked in red. Suppose a phase shift occurs due to some reason, this results in a change in the width of v_fb from τ_osc to τ_osc’ as the phase transitions from β_τ2 to β_τ2’. By comparing it with the threshold v_d, the width of v_ps also tracks this change, shifting from τ_osc to τ_osc’. As shown in Figure 12, this method enables the system to promptly track the phase shift.

4.3. Power Injecting Unit

The power regulating unit comprises a comparator A₂ and an integrator. The integrator is composed of a resistor R_int and capacitor C_int. The control signal, v_ps, is connected to the input of the integrator, as depicted in Figure 13. This configuration demonstrates how the integrator operates under the control of output of comparator A₁, v_ps. To simplify the analysis, the output of A₁ is considered equivalent to a transistor push–pull structure.

Referring to Figure 13, the control process of v_ps for the integrator can be described as follows:

(1)

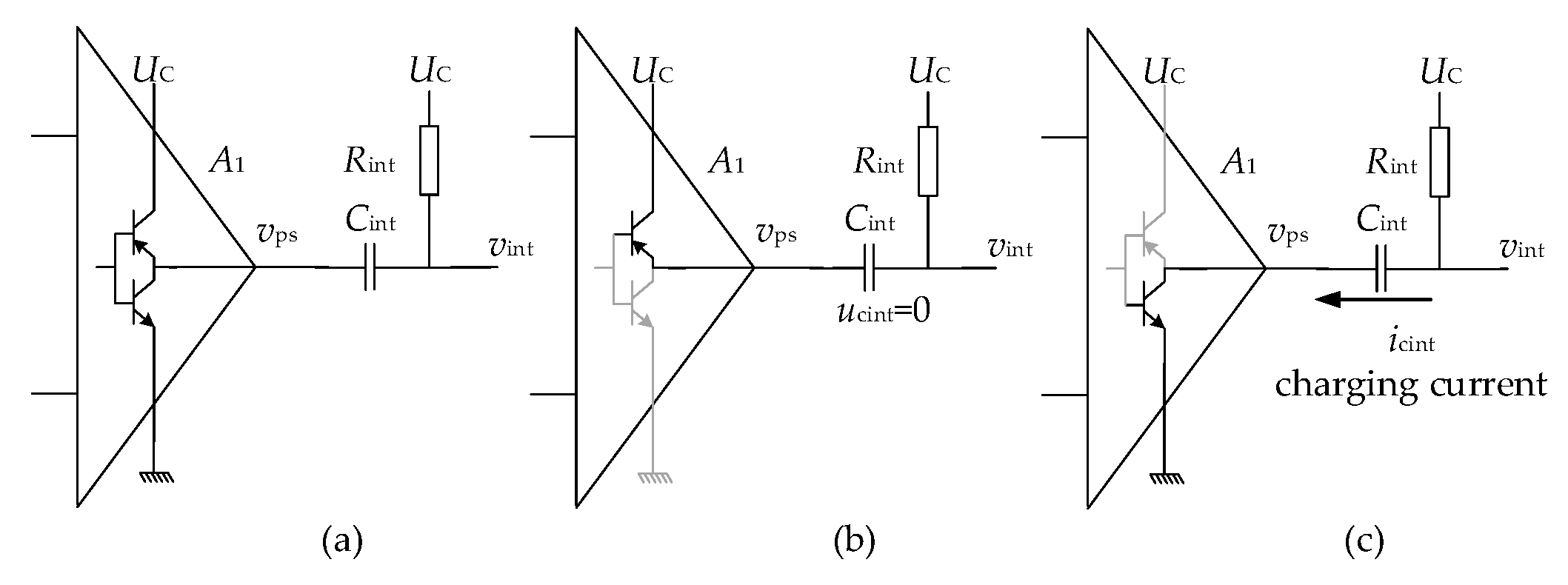
When v_ps is at a high level, the upper switch of the push–pull structure is turned on, as depicted in Figure 13b. In this case, both ends of the capacitor C_int are connected to U_c, resulting in zero voltage across C_int. Consequently, the integrator ceases its operation, and the integrator output v_int = U_c.

(2)

As v_ps is lowered, the lower switch of the push–pull structure is activated, as shown in Figure 13c. In this scenario, the input of the integrator is connected to the ground, initiating the charging of capacitor C_int through R_int by U_c. As a result, the output of the integrator, v_int, begins to increase from zero. The expression for v_int can be represented as follows:

$$v_{\mathrm{int}}=\frac{1}{C_{\mathrm{int}}}{\displaystyle {\int }_{t_0}^t\frac{U_{\mathrm{C}}}{R_{\mathrm{int}}}}dt$$

(23)

In Equation (23), t₀ represents the time at which the integrator begins its operation. Assuming that the moment v_ps is pulled to low level corresponds to the time when the integrator starts working, then t₀ can be set to 0. In this case, v_int can be expressed as follows:

$$v_{\mathrm{int}}=\frac{1}{C_{\mathrm{int}}}{\displaystyle {\int }_0^t\frac{U_{\mathrm{C}}}{R_{\mathrm{int}}}}dt=\frac{U_{\mathrm{C}}}{R_{\mathrm{int}}{C}_{\mathrm{int}}}t$$

(24)

The output of the integrator, v_int, is then compared with a threshold v_r in comparator A₂, and the comparison result v_g can be expressed as follows:

$$v_{\mathrm{g}}=\{\begin{array}{cc}{U}_{\mathrm{C}}& v_{\mathrm{int}}<v_{\mathrm{r}}\\ 0& v_{\mathrm{int}}>v_r\end{array}$$

(25)

The control signal v_g is sent to the oscillating unit to control the switch S, turning it on or off. It is evident that by controlling the duration of v_g at the high level, the output power of the system can be regulated. Since v_int is a signal that starts at zero and rises linearly, according to Equation (25), the duration of v_g is determined by the threshold v_r. Hence, v_r can be used as a variable to control the system power.

Figure 14 illustrates the principle of utilizing v_r to control the system power. In the figure, the green and red lines represent the waveforms before and after the change in v_r, respectively. Figure 14 demonstrates that when the power control variable is increased from v_r to v_r’, the end time of power injecting state extends from t_(n+1)1 to t_(n+1)1’, and the duration of power injecting extends from τ_inj to τ_inj’.

As depicted in Figure 14, with the increase in τ_inj, the amplitudes of i_p also increase from I_pm1 and I_pm2 to I_pm1’ and I_pm2’, respectively, indicating an increase in the power injected into the system. Conversely, if v_r is decreased, the power injected into the system will be reduced accordingly.

Hereto, the challenges to tracking the oscillation phase and controlling the duration of power injection have been successfully resolved. To provide a clearer representation, Figure 11 is depicted as a block diagram in Figure 15, illustrating the operational process of the proposed IPT system.

Initially, the signal v_fb, which carries the oscillating network information, is directed to the phase tracking unit. This unit detects the duration of the oscillating state, τ_osc, and generates the output signal v_ps.

Subsequently, v_ps serves as a trigger signal to initiate the power regulating unit. By utilizing the control variable v_r, the power regulating unit determines the duration of the power injecting state, τ_inj, and generates the control signal v_g.

Ultimately, the control signal v_g is transmitted to the oscillating unit, which seamlessly transitions between the self-oscillating state and the power injecting state, thereby completing the self-oscillating closed loop of the system.

Figure 16 presents the curves of crucial signals during each operation of the proposed IPT system, elucidating the temporal relationship between these signals. This visualization aims to enhance comprehension of the system’s working principle.

5. Validate Prototypes and Experiment

A verification platform was built to verify the theoretical analysis of the proposed dynamic IPT system, as shown in Figure 17, which includes a prototype made according to Figure 11. In the experimental platform, an oscilloscope (ROHDE&SCHWARZ RTB2004, Munich, Germany) and a power analyzer (YOKOGAWA WT500, Tokyo, Japan) are hired to monitor the working waveform and analyze the system power, respectively. A resistance group consisting of four resistors and switches is used to provide different load resistor. In the experiment, the main parameters of the experimental platform are listed in

Table 3. The main parameters of the verify prototype.

Parameter	Value	Parameter	Value
UDC, Uc	100–200 V, 5 V	Comparator	LM339
Rint, Cint	50 k, 20 nF	Rd, Dd	1 k, 1N4148
IGBT	H30R1602	RL	10–50 Ω

, and L_p, L_s, C_p, Cs can be obtained from Table 2.

The primary and secondary coils used in the experimental platform are made of a 3 mm diameter Leeds wire wound 18 turns, and a cross-shaped ferrite core was installed behind the coil. Both the coils are tested by a digital electric bridge (VICTOR 4091C LCR, Shenzhen, China). According to the test results, the relationship of the coupling coefficient k between the coils and the air gap d can be obtained, as shown in Figure 18.

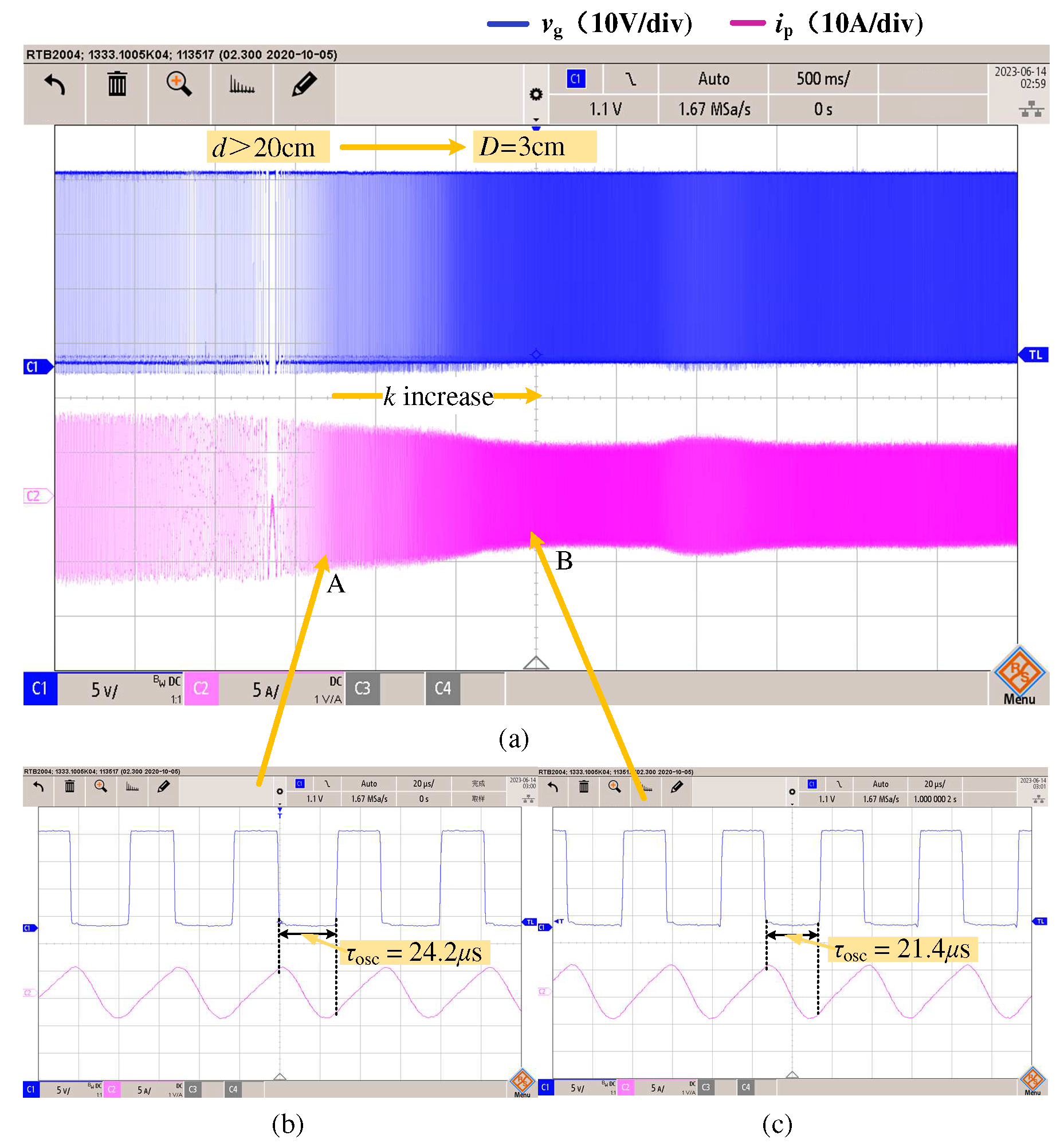
5.1. Self-Oscillation Characteristic Verification

The curves of i_p, i_cp, i_DC, and v_ds were obtained from the experiment, as depicted in Figure 19. Here, v_ds represents the voltage across switch S, given by Equation (20), where it is used to represent u_p. The following phenomena can be observed in this experiment:

(1)

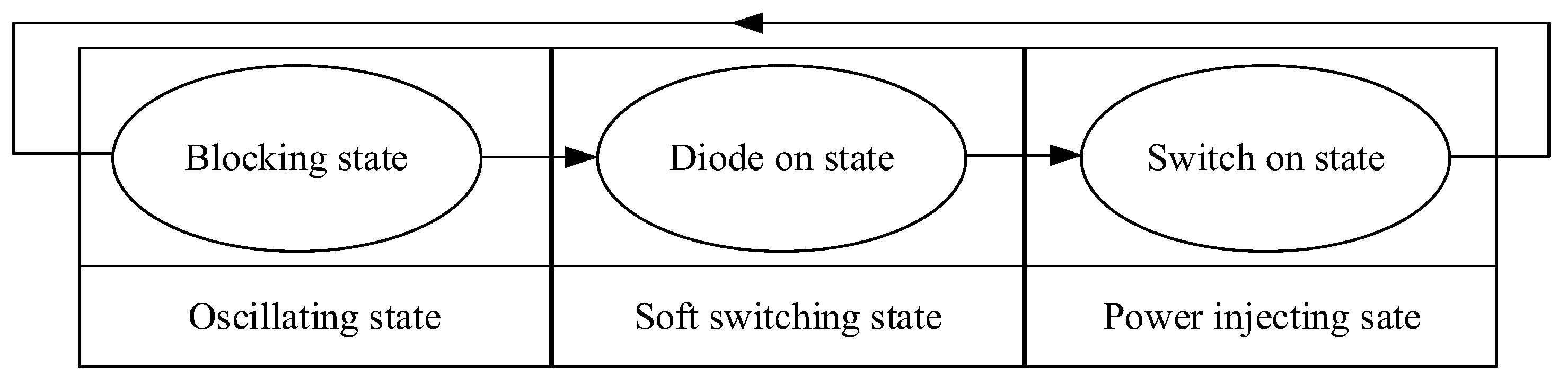
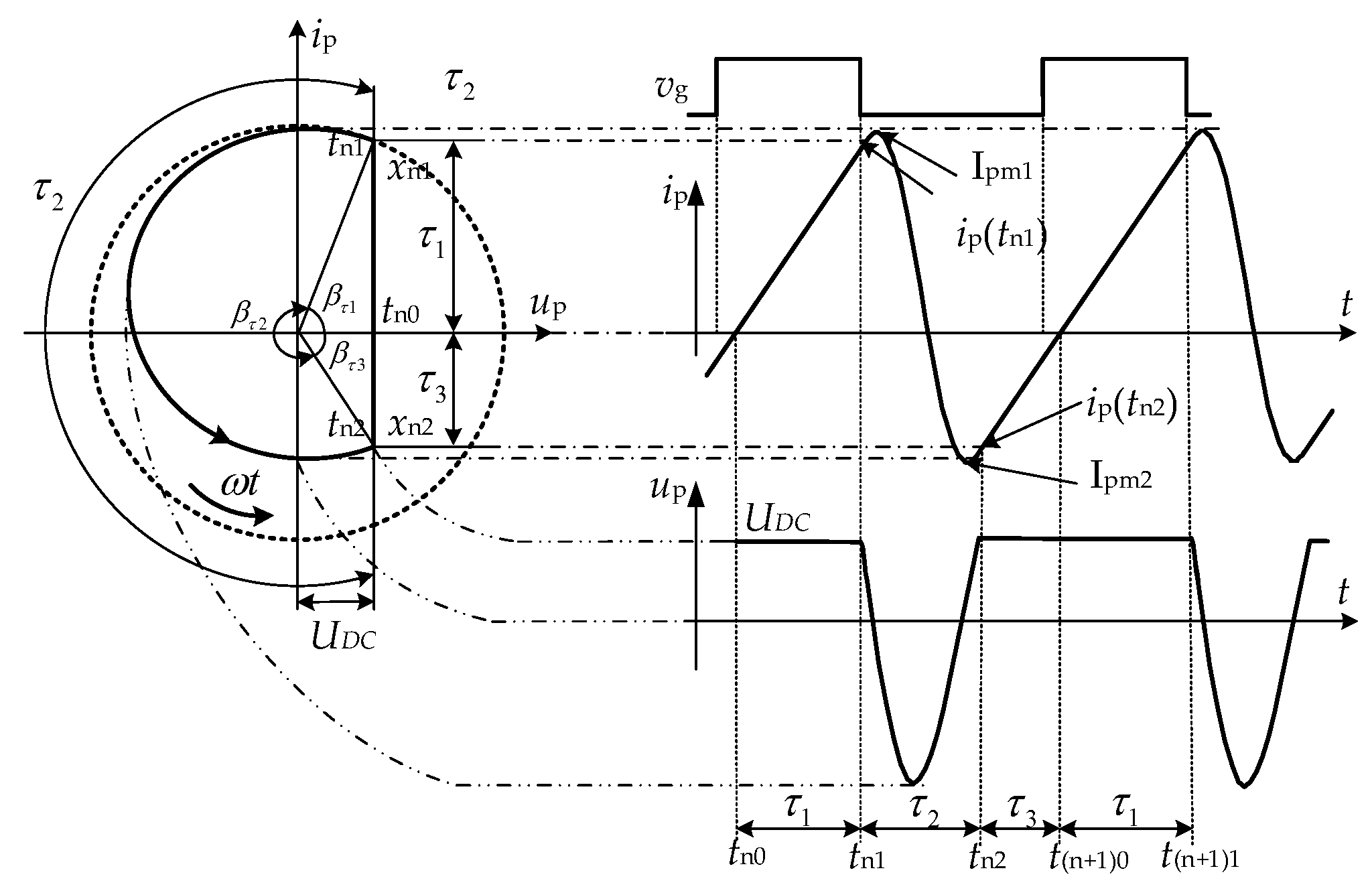
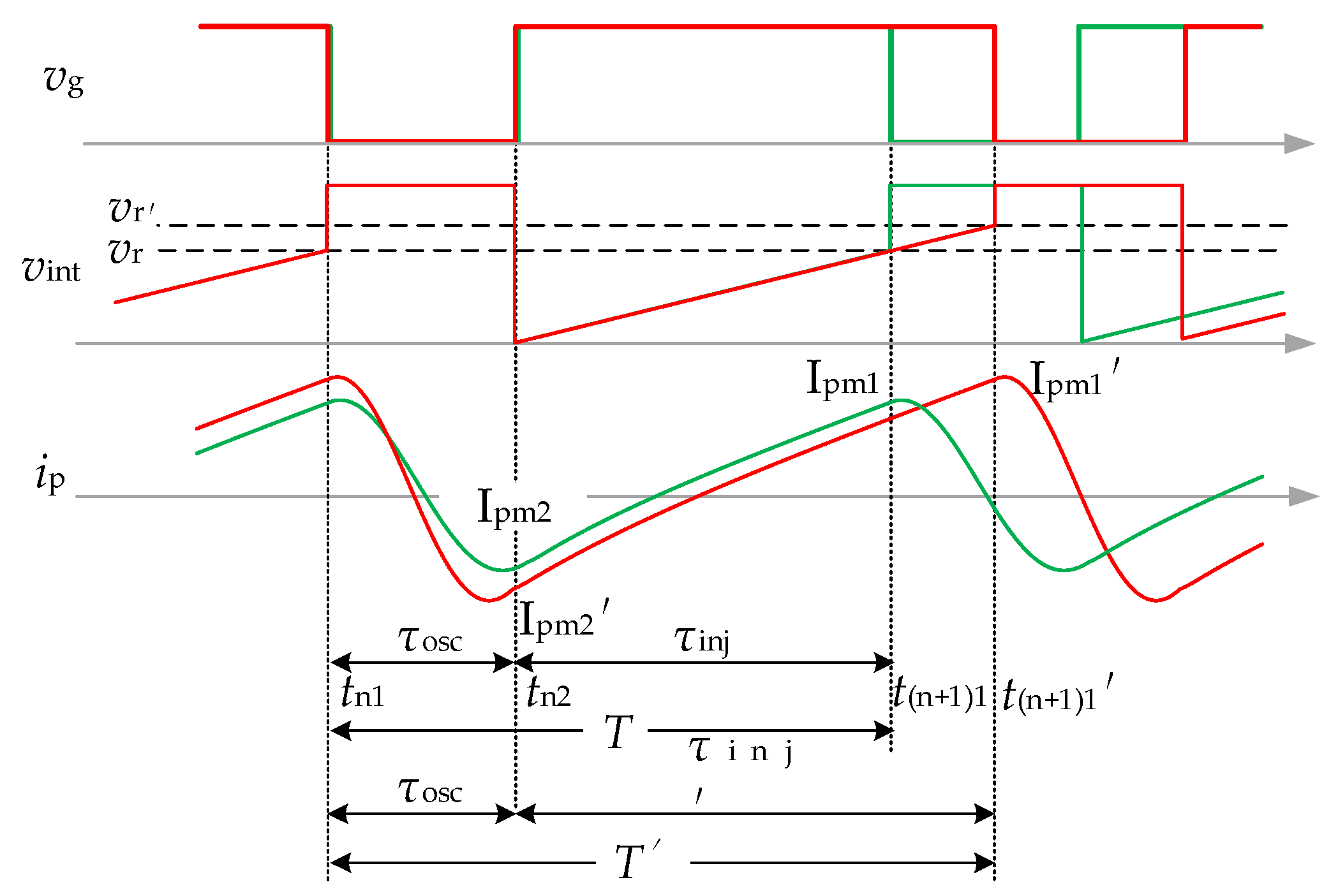
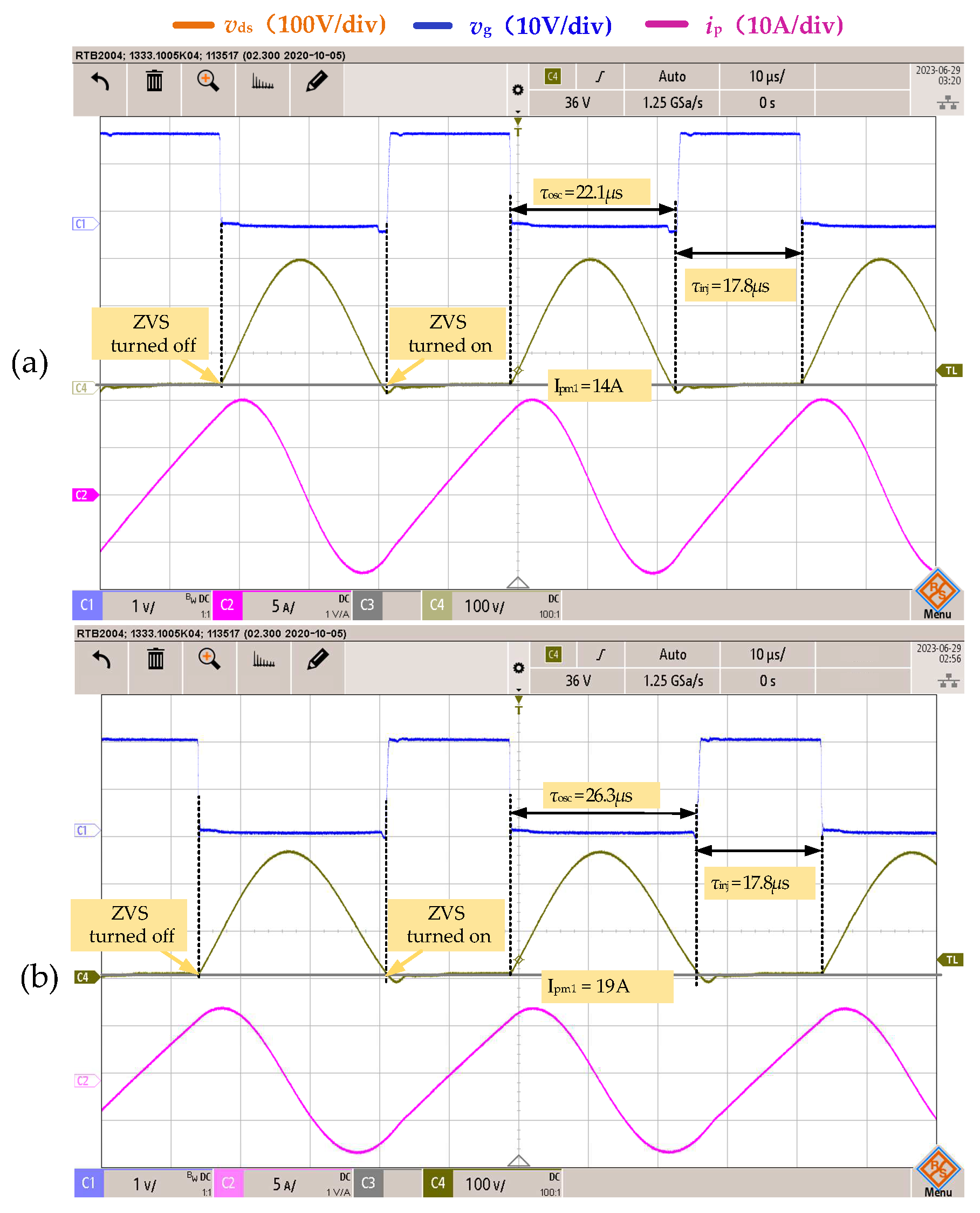
The system operates in a closed loop, following the sequence of the blocking (self-oscillating) state, τ₂, the diode-on state τ₃, and the switch-on state τ₁, as described in Figure 4. This demonstrates that the system is capable of self-oscillation, with the oscillation process repeating in the oscillating state, τ_osc, and the power injecting state, τ_inj.

(2)

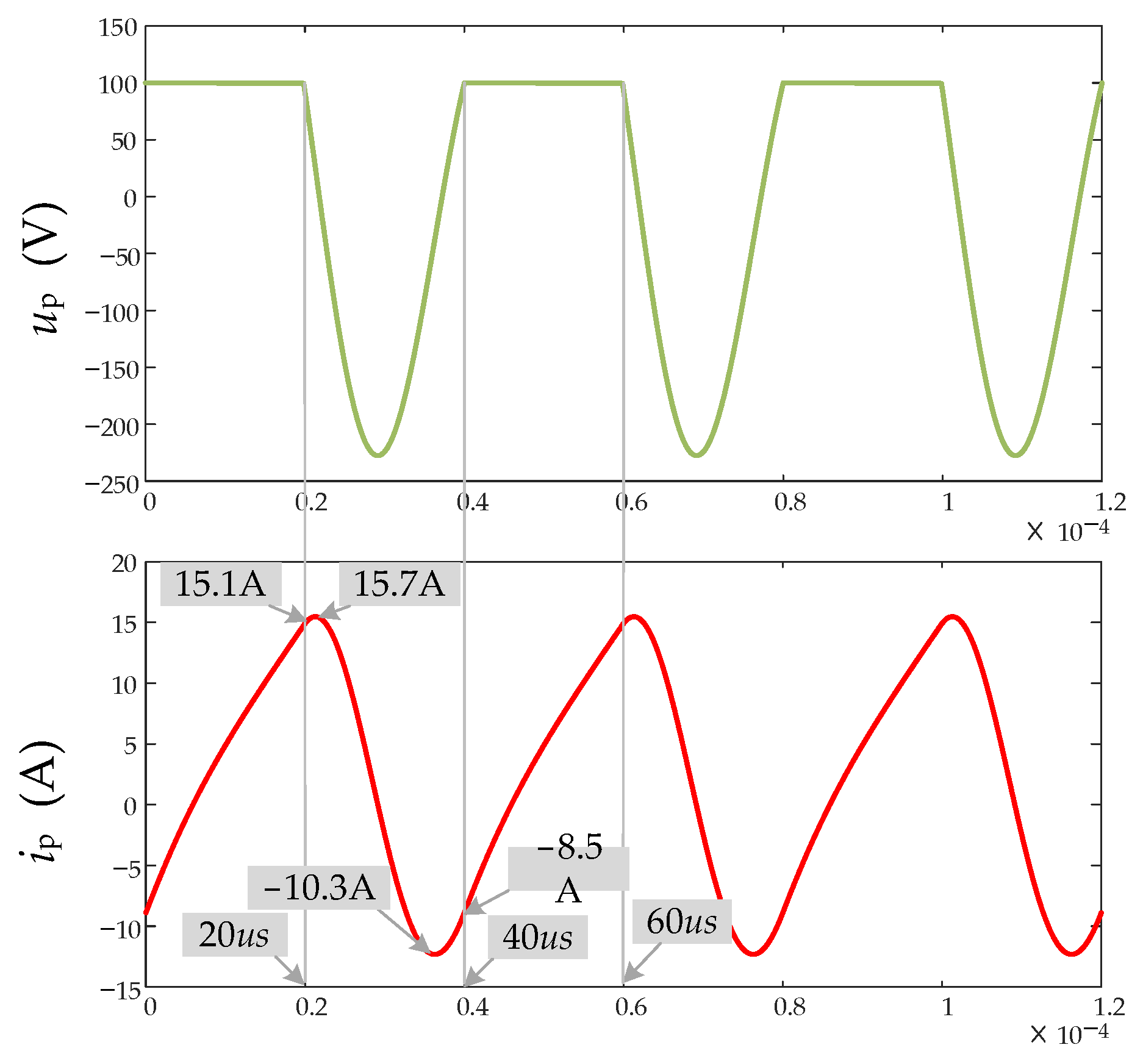
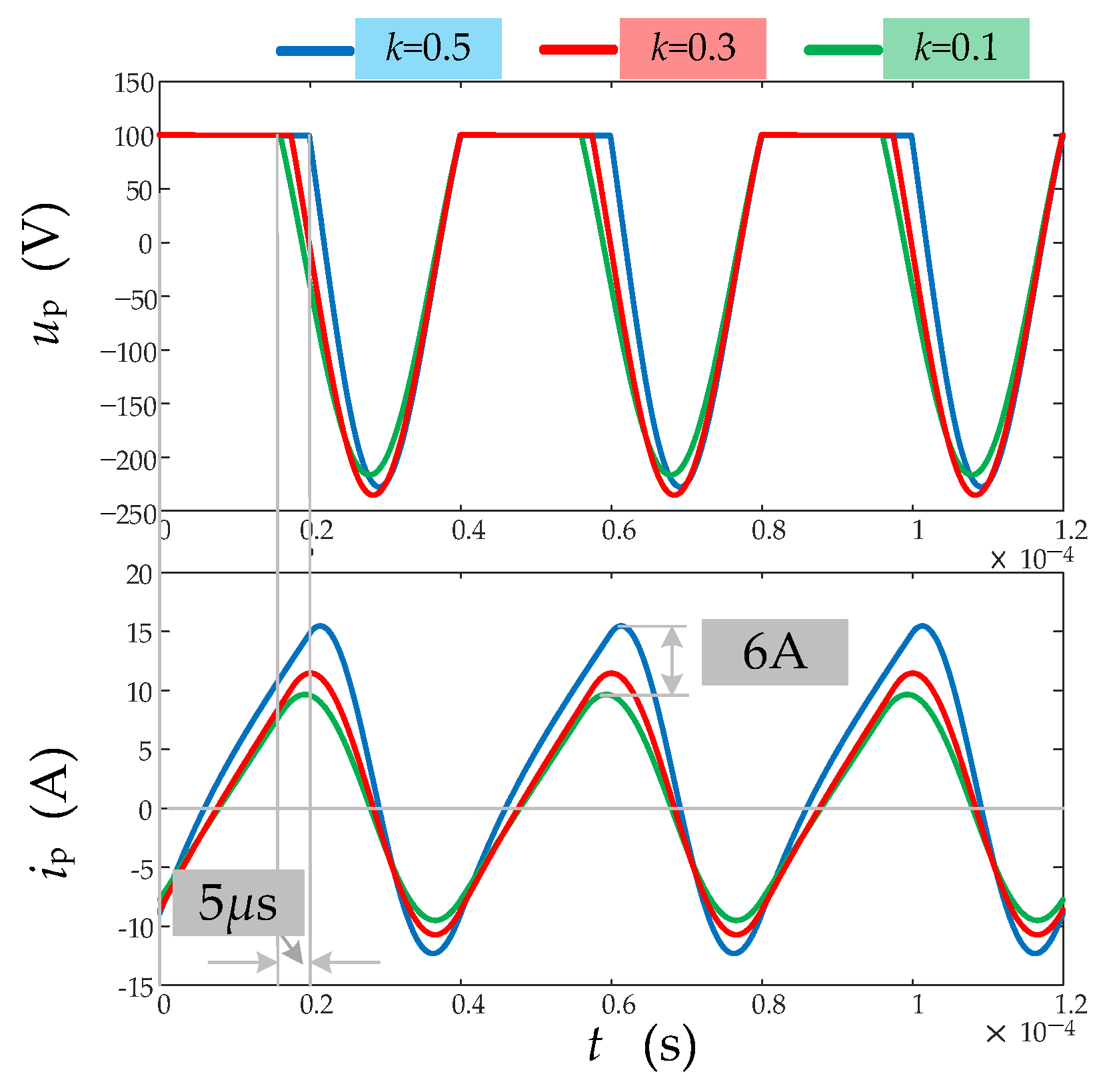
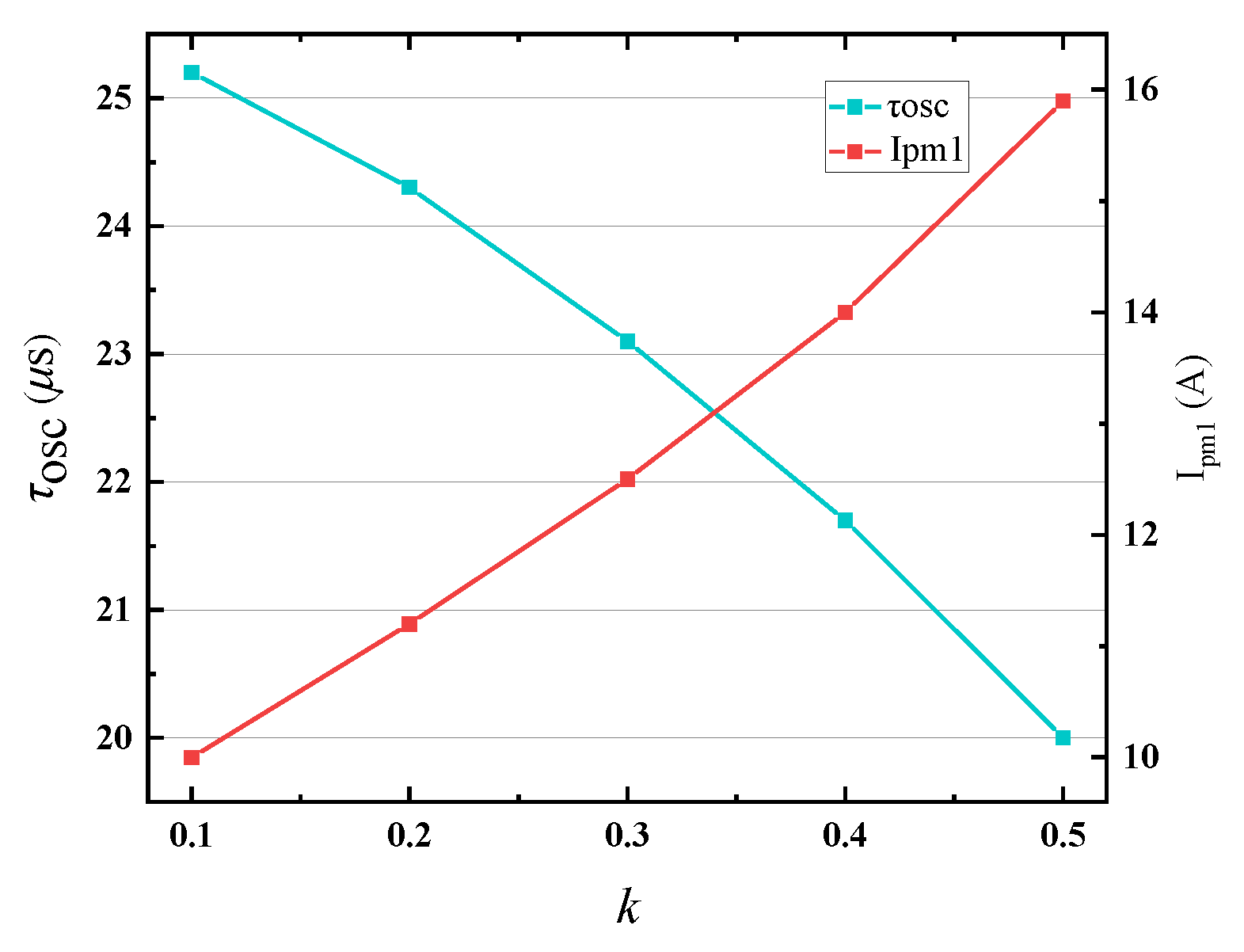
During the oscillating state, i_DC is equal to zero, indicating that the oscillating network is isolated from U_DC. The capacitor C_p and inductor L_p form a resonant tank and initiate oscillation. The capacitor voltage, v_ds (u_p), capacitor current, i_cp, and the inductor current, i_p, exhibit sinusoidal changes, with i_cp and i_p being equal. Clearly, the oscillation is sustained for less than one cycle. The oscillation state duration τ_osc being 22 μs, slightly larger than the simulation results shown in Figure 8. This deviation may be caused by the deviation of component parameters.

(3)

In the diode-on state, i_DC is negative, indicating that the current i_p flows back to U_DC through the diode. In the switch-on state, i_DC is positive, indicating that U_DC injects a current into the inductor L_p. Combining the diode-on state, τ₃, and the switch-on state, τ₁, into the power injecting state τ_inj, it can be observed that during the power injecting state, i_p linearly increases from negative to positive with a rise slope of 1 × 10⁶ A/s, consistent with the calculated result from Equation (1). Additionally, the capacitor current, i_cp, is zero during the power injecting state, implying that the capacitor voltage is clamped to U_DC during these two states.

This experimental results are consistent with the theoretical analysis presented in Figure 1 and Figure 4. Additionally, the experimental results closely correspond to the simulation result depicted in Figure 8, thus validating the accuracy of the model established in Section 3.

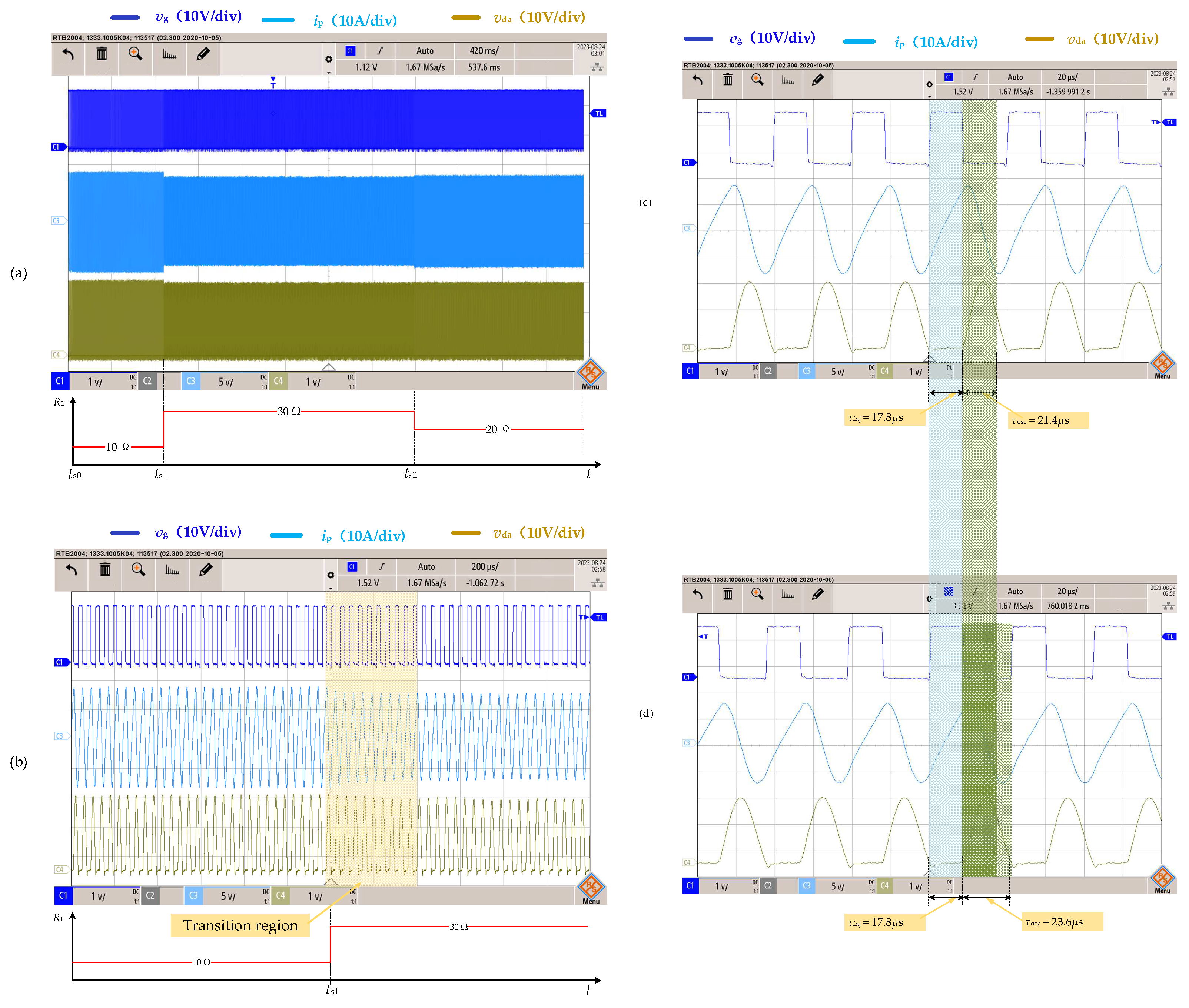
5.2. Power Regulating Verification

Figure 20 exhibits the experimental curves of u_ds, v_g, v_int and i_p, serving as a means to assess the performance of the IPT system outlined in Figure 11, as well as the impact of the control variable v_r on the system’s output power regulation. Specifically, in subfigure (a), with v_r set to 2.5 V, and in subfigure (b), with v_r set to 3.5 V. The experimental results yield the following observations:

(1)

The experimental curves are exactly the same as the principle curves shown in Figure 16, which indicates that the system can realize self-oscillation according to the order of the self-oscillating state and power injecting state.

(2)

The power injecting state duration can only be controlled by the control variable v_r. The comparison between (a) and (b) shows that when v_r increases from 2.5 V to 3.5 V, the power injection duration τ_inj increases from 24 μs to 36 μs, and the amplitude I_pm1 of the current i_p increases from 14 A to 20 A, indicating that the power can be adjusted by controlling v_r.

It is noted that when the control variable v_r adjusts the power injecting state duration, the self-oscillating state duration τ_osc is not affected and is maintained at 22 μs. This shows that the power injection process is decoupled from the self-oscillation process.

Figure 21 illustrates the relationship between output power, efficiency, and the control variable v_r. This experimental result demonstrates a monotone positive correlation between output power and v_r. The reason for this correlation is that the proposed IPT system’s power injection process is decoupled from the self-oscillation process. The power is solely regulated by the control variable v_r. This characteristic simplifies the design of output power regulation and provides a convenient technical implementation for voltage, current, or power closed-loop control within the system.

There is a discrepancy between the calculated output power value and the experimental value shown in Figure 21. This deviation occurs because the phase tracking unit employs the forward voltage v_d of diode D, 0.7 V, as a threshold, as illustrated in Figure 16. Consequently, the detected value of the self-oscillation state duration is smaller than the actual value, resulting in the experimental output power value being lower than the value calculated using Equation (19). Nevertheless, as the output power increases, the influence of the self-oscillating state duration diminishes, bringing the experimental output power value closer to the calculated value.

It can be observed that in Figure 21, the efficiency at v_r = 2.5 V and 3.0 V reaches an optimal value of 89.2%, while it decreases to 83.5% and 87.6% at v_r = 2 V and 3.5 V, respectively. This phenomenon occurs because the power is regulated by adjusting the transmission period, which can lead the system to deviate from the optimal equivalent impedance.

5.3. Phase Tracking and Soft Switch Condition Verification

Figure 22 illustrates the experimental curves of i_p, u_ds and the control signal v_g of switch S, providing evidence of the phase tracking characteristic of the proposed IPT system. The results reveal that when d = 3 cm, the self-oscillating state lasts for τ_osc = 22.1 μs, and the power injecting state persists for τ_inj = 17.8 μs. With an increase in d = 12 cm, the duration of the self-oscillating state extends to 26.3 μs, while the power injecting state remains unchanged at τ_inj = 17.8 μs.

This experiment clearly demonstrates the system’s excellent good phase (frequency) tracking capability and confirms that the self-oscillation method proposed in this paper does not interfere with each other between the self-oscillating state and the power injecting state. Significantly, it can be observed that, despite the variation in the self-oscillating state duration, the switch S effectively tracks the change and remains at the ZVS soft switching point.

5.4. Dynamic Characteristics Verification

To test the dynamic characteristics and robustness of the system, an experiment was conducted involving the shifting of the coil’s position. The procedure for the experiment is as follows: initially, the secondary coil was positioned more than 20 cm away from the primary coil. Subsequently, the secondary coil was rapidly moved to a position just 3 cm away from the primary coil. The experimental results are represented by the curves of v_g and i_p, which were captured by the oscilloscope and depicted in Figure 23.

Figure 23a illustrates the curves obtained at a scale of 500 ms/div, which is used to depict the overall dynamic process in the experiment. On the other hand, (b,c) display the curves unfolded at a scale of 20 μs/div, captured at different time points from (a), aiming to present detailed information in dynamic experiments. The experimental results demonstrate that the system can accurately track the phase change caused by the alteration of coupling coefficient. Throughout the experiment, as the coil distance varies, the duration of the oscillating state automatically adjusts from 24.2 μs to 21.4 μs.

Furthermore, an experiment on sudden load tolerance was conducted. In this experiment, the load steps from 10 Ω to 30 Ω, and then returned to 20 Ω. Figure 24a depicts the experimental results obtained at a scale of 500 ms/div, demonstrating that all the curves effectively track the changes in load.

Figure 24b provides an expanded of Figure 24a at time point t_s1, offering a detailed examination of the transition from 10 Ω to 30 Ω. The revealed details indicate that the curve transition remarkably smooth, taking only 10 cycles to complete.

Moving on to Figure 24c,d, it further amplifies the data from Figure 24b, this time at a scale of 20 μs/div. Specifically, Figure 24c focuses on the R_L = 10 Ω scenario, while Figure 24d looks at R_L = 30 Ω. Notably, the power injection time remaining at the self-oscillation time increases from 21.4 μs to 23.6 μs. This outcome suggests that the load change solely impacts the resonant angular frequency of the self-oscillation, highlighting the decoupling of the power injection process from the self-oscillation process.

These experimental findings substantiate the effectiveness of the proposed closed loop self-oscillating structure, which exhibits the ability to track parameter changes in one period while showcasing remarkable robustness of the system.

6. Conclusions

To address the challenges associated with frequency tracking and power control in conventional IPT system, which arise due to the coupling between the converter and the resonant network, this study proposes a method for implementing an IPT system based on a phase-closed loop. This method divides a power transfer process into two distinct states: the self-oscillating state and power injecting state, and ensures their independence from each other. The effectiveness of this method was verified through simulation and experiments, which led to the following conclusions:

(1)

The working process of a converter can be divided into three distinct states: the switch-on state, blocking state, and diode-on state. This division allows for decoupling of the resonant network from the converter. By combining the switch-on state and diode-on state into power injecting state, and considering the blocking state as oscillating state, the working process of an IPT system consists of two state sequences: power injecting and oscillating states. By controlling the duration of the power injecting state, the output power can be adjusted independently. Furthermore, by detecting the duration of the self-oscillating state, changes in frequency can be accurately tracked and compensated for. The diode-on state’s soft switching characteristics enable a smooth transition between the power injecting and oscillating states.

(2)

The proposed phase-closed loop, comprising the phase tracking unit, power regulating unit, and oscillating unit, proves to be effective. This closed loop enables the system to switch between the power injecting state and oscillating state under soft switching condition.

(3)

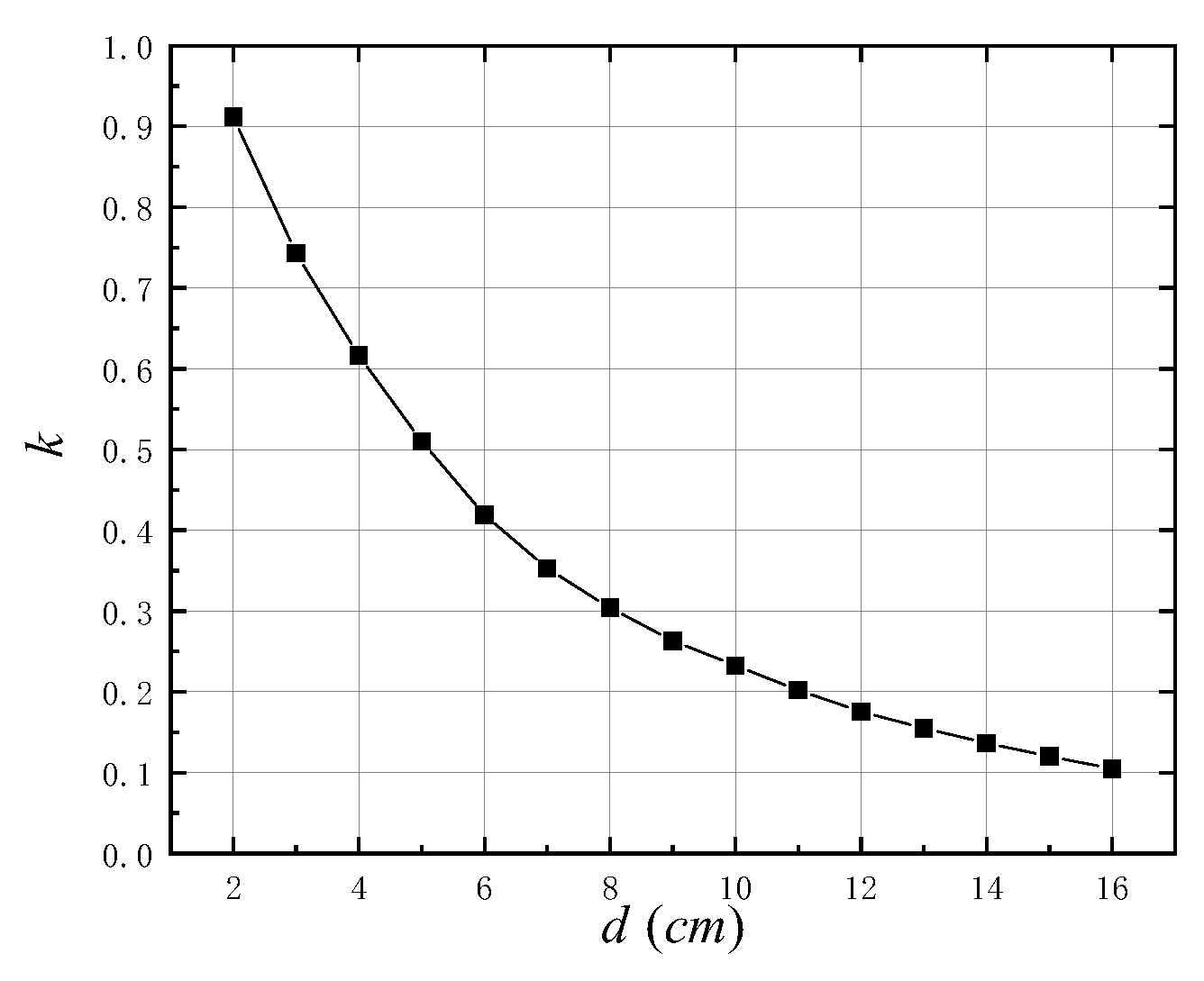
Experimental results demonstrate that the phase tracking unit accurately tracks frequency drift caused by system parameters, indicating excellent frequency tracking characteristics. Furthermore, the experiments show that the system exhibits robust frequency tracking, even under conditions of a large coupling coefficient and maximum speed change.

(4)

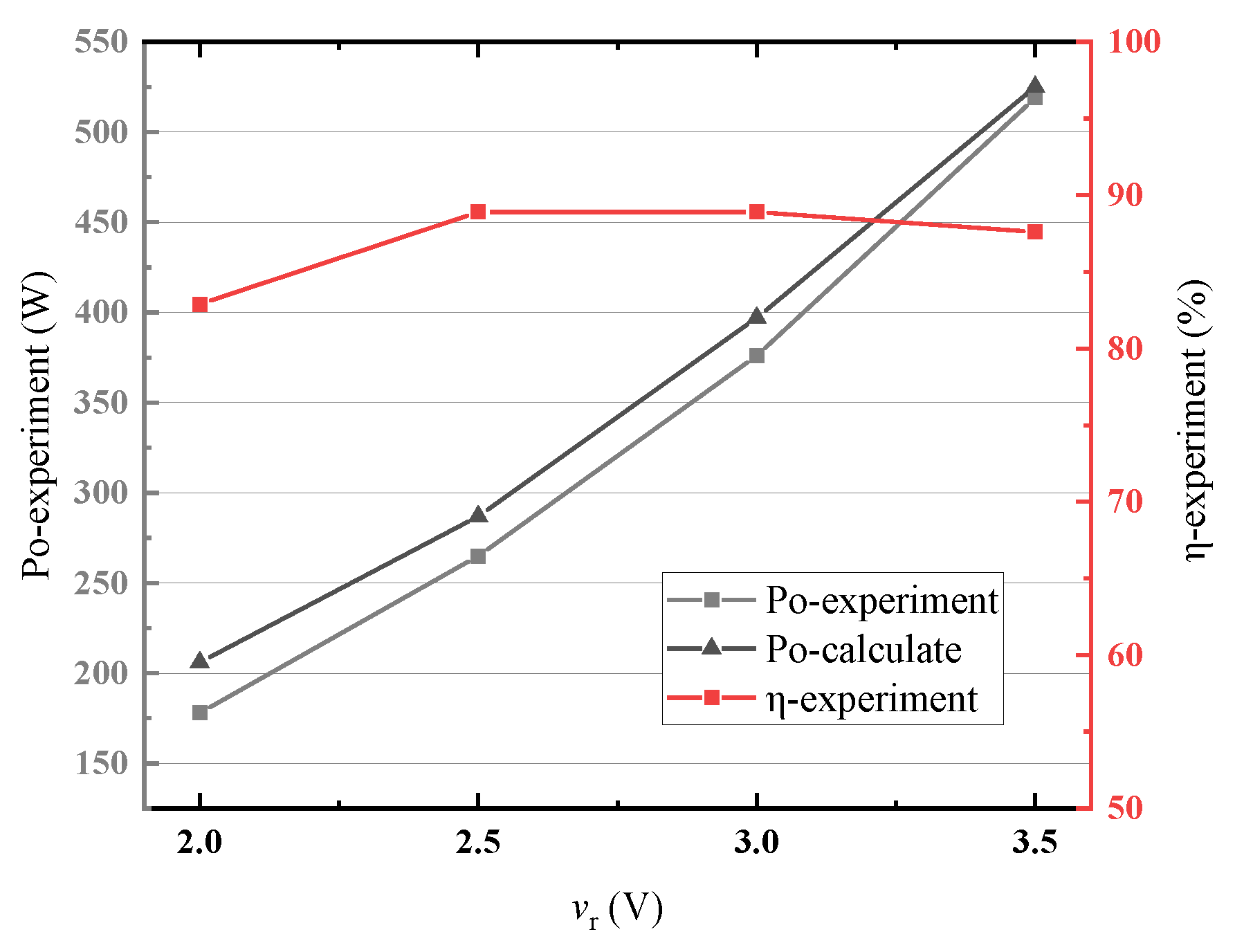
The proposed power control method utilizing an integrator is proved to be effective. With the power injecting process decoupling from the oscillating process, power regulation only requires manipulation of the control variable v_r. The experimental results indicate that the output power monotonically increases with the control variables v_r. This power regulation characteristic simplifies and enhances the reliability of designing an IPT system control strategy.